JAC Class 10 Maths Solutions Chapter 1 Real Numbers Ex 1.4

Jharkhand Board JAC Class 10 Maths Solutions Chapter 1 Real Numbers Ex 1.4 Textbook Exercise Questions and Answers.

JAC Board Class 10 Maths Solutions Chapter 1 Real Numbers Exercise 1.4

Question 1.
Without actually performing the long division, state whether the following rational numbers will have a terminating decimal expansion or a non-terminating repeating decimal expansion:
1. \(\frac{13}{3125}\)
2. \(\frac{17}{8}\)
3. \(\frac{64}{455}\)
4. \(\frac{15}{1600}\)
5. \(\frac{29}{343}\)
6. \(\frac{23}{2^3 5^2}\)
7. \(\frac{129}{2^2 5^7 7^5}\)
8. \(\frac{6}{15}\)
9. \(\frac{35}{50}\)
10. \(\frac{77}{210}\)
Solution:
1. \(\frac{13}{3125}\)
Here, the denominator q(5⁵) is of the form 2ⁿ5^m with n = 0 and m = 5.
Hence, the decimal expansion of \(\frac{13}{3125}\) is terminating.

2. \(\frac{17}{8}\) = \(\frac{17}{2^3}\)
Here, the denominator q(2³) is of the form 2ⁿ5^m with n = 3 and m = 0.
Hence, the decimal expansion of \(\frac{17}{8}\) terminating.

3. \(\frac{64}{455}=\frac{64}{5 \times 7 \times 13}\)
Here, the denominator q(5 × 7 × 13) is not of the form 2ⁿ5^m, where n and m are non-negative integers.
Hence, the decimal expansion of \(\frac{64}{455}\) is non-terminating repeating.

4. \(\frac{15}{1600}=\frac{3}{320}=\frac{3}{2^6 \times 5^1}\)
Here, the denominator q(2⁶ × 5¹) is of the form 2ⁿ5^m with n = 6 and m = 1.
Hence, the decimal expansion of \(\frac{15}{1600}\) is terminating.

5. \(\frac{29}{343}=\frac{29}{7^3}\)
Here, the denominator q(7³) is not of the form 2ⁿ5^m, where n and m are non-negative integers.
Hence, the decimal expansion of \(\frac{29}{343}\) is non-terminating repeating.

6. \(\frac{23}{2^3 5^2}\)
Here, the denominator q(2³5²) is of the form 2ⁿ5^m with n = 3 and m = 2.
Hence, the decimal expansion of \(\frac{23}{2^3 5^2}\) is terminating.

7. \(\frac{129}{2^2 5^7 7^5}\)
Here, the denominator q (2² × 5⁷ × 7⁵) is not of the form 2ⁿ5^m, where n and m are non-negative integers. Hence, the decimal expansion of \(\frac{129}{2^2 5^7 7^5}\) is non-terminating repeating.

8. \(\frac{6}{15}=\frac{2 \times 3}{3 \times 5}=\frac{2}{5^1}\)
Here, the denominator q(5¹) is of the form 2ⁿ5^m with n = 0 and m = 1.
Hence, the decimal expansion of \(\frac{6}{15}\) is terminating.

9. \(\frac{35}{50}=\frac{5 \times 7}{2 \times 5 \times 5}=\frac{7}{2^1 \times 5^1}\)
Here, the denominator q(2¹ × 5¹) is of the form 2ⁿ5^m with n = 1 and m = 1.
Hence, the decimal expansion of \(\frac{35}{50}\) is terminating.

10. \(\frac{77}{210}=\frac{7 \times 11}{2 \times 3 \times 5 \times 7}=\frac{11}{2 \times 3 \times 5}\)
Here, the denominator q(2 × 3 × 5) is not of the form 2²ⁿ5^m, where n and m are non-negative integers.
Hence, the decimal expansion of \(\frac{77}{210}\) is non-terminating repeating.

Question 2.
Write down the decimal expansions of those rational numbers in Question 1 above which have terminating decimal expansions.
Solution:

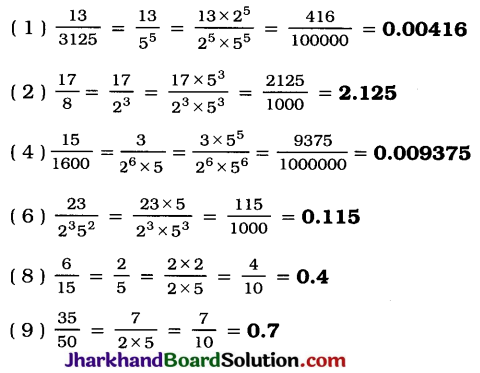
Question 3.
The following real numbers have decimal expansions as given below. In each case, decide whether they are rational or not. If they are rational, and of the form \(\frac{p}{q}\), what can you say about the prime factors of q?
1. 43.123456789
2. 0.120120012000120000…
3. \(43 . \overline{123456789}\)
Solution:
1. The given number 43.123456789 is a rational number as its decimal expansion is terminating
Since the decimal expansion of the given number is terminating, in the \(\frac{p}{q}\) form of the number, the prime factors of q will only be 2 or 5 or both. (Theorem 1.5)

2. The given number 0.120120012000120000…. is an irrational number as its decimal expansion is non-terminating non-repeating.

3. The given number \(43 . \overline{123456789}\) is a rational number as its decimal expansion is an non-terminating repeating.
Since the decimal expansion of the given number is non-terminating repeating, in the \(\frac{p}{q}\) form of the number, the prime factors of q will contain at least one prime other than 2 and 5. (Converse of Theorem 1.7)