Jharkhand Board JAC Class 10 Maths Solutions Chapter 10 Circles Ex 10.2 Textbook Exercise Questions and Answers.
JAC Board Class 10 Maths Solutions Chapter 10 Circles Exercise 10.2
Question 1.
From a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. The radius of the circle is
(A) 7 cm
(B) 12 cm
(C) 15 cm
(D) 24.5 cm
Solution :

OQ² = TO² – TQ²
= 25² – 24²
= (25 + 24) (25 – 24)
= 49 × 1 = 49
OQ = \(\sqrt{49}\) = 7 cm.
![]()
Question 2.
In the figure, if TP and TQ are the two tangents to a circle with centre O so that ∠POQ = 110°, then ∠PTQ is equal to
(A) 60°
(B) 70°
(C) 80°
(D) 90°
Solution :
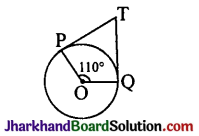
POQT is a cyclic quadrilateral because \(\hat{\mathbf{P}}\) + \(\hat{\mathbf{O}}\) = 180°.
∴ \(\hat{\mathbf{O}}\) + \(\hat{\mathbf{T}}\) = 180°
latex]\hat{\mathbf{O}}[/latex] = 110°.
latex]\hat{\mathbf{T}}[/latex] = 180° – 110° = 70°.
Question 3.
If tangents PA and PB from a point P to a circle with centre O are inclined to each other at an angle of 80°, then ∠POA is equal to
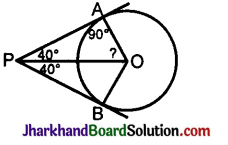
A) 50°
B) 60°
C) 70°
D) 80°
Solution :
In ΔPAO, P\(\hat{A}\)O = 90° A\(\hat{P}\)O = 40°
∴ A\(\hat{O}\)P = 180° – 90° – 40° = 50°.
![]()
Question 4.
Prove that the tangents drawn at the ends of a diameter of a circle are parallel.
Solution :
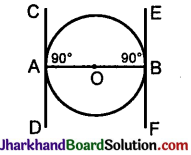
Data: O is the centre of the circle.
AB is a diameter. CD and EF are tangents drawn at A and B.
To prove: CD || EF.
Proof: O\(\hat{A}\)C = 90°
O\(\hat{B}\)E = 90°
B\(\hat{A}\)C + A\(\hat{B}\)E = 180°
These are co-interior angles.
Radius is ⊥ to the tangent at the point of contact
Question 5.
Prove that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
Solution :
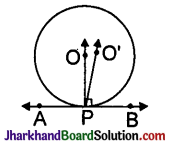
Let us consider a circle with centre O.
Let AB be a tangent which touches the circle at P.
We have to prove that the line perpendicular to AB at P passes through the centre O.
We shall prove this by contradiction method.
Let us assume that the perpendicular to AB at P does not pass through centre O.
Let it pass through another point O’. Join OP and O’P.
As the perpendicular to AB at P passes through O’, therefore,
∠O’PB = 90° ……..(1)
O is the centre of the circle and P is the point of contact. We know that the line joining the centre and the point of contact to the tangent of the circle are perpendicular to each other.
∴ ∠OPB = 90° ……..(2)
Comparing equations (1) and (2), we obtain
∠O’PB = ∠OPB ….(3)
From the figure, it can be observed that,
∠O’PB < ∠OPB ………..(4)
Therefore, ∠O’PB = ∠OPB is not possible. It is only possible when the line O’P coincides with OP. Therefore, the perpendicular to AB at P passes through the centre O.
![]()
Question 6.
The length of a tangent from a point A at a distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
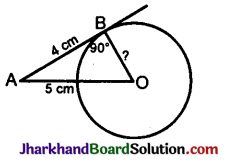
Solution :
A\(\hat{B}\)O = 90° (The radius is perpendicular to the tangent at the point of contact)
BO² + AB² = AO²
BO² = AO² – AB²
= 5² – 4²
= 25 – 16 = 9
BO = \(\sqrt{9}\) = 3 cm.
Hence, radius of the circle is 3 cm.
Question 7.
Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
Solution :
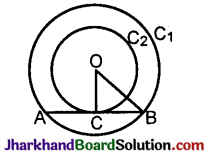
O is the centre of the concentric circles C1 and C2.
AB is a chord of the bigger circle C1.
AB is a tangent to circle C2.
OC = 3 cm, OB = 5 cm.
To find AB.
Proof: In ΔOCB,
O\(\hat{C}\)B = 90° (The tangent AB to C2 is ⊥ to the radius CO drawn from the point of contact C)
In ΔOCB, O\(\hat{C}\)B = 90°
OC² + CB² = OB²
3² + CB² = 5²
CB² = 5² – 3²
= 25 – 9 = 16
CB = 4 cm.
In circle C1 AB is a chord. OC ⊥ AB.
∴ AC = CB = 4 cm. The ⊥ drawn to a chord from the centre bisects the chord.
AB = AC + CB
= 4 + 4 = 8 cm.
![]()
Question 8.
A quadrilateral ABCD is drawn to circumscribe a circle. Prove that AB + CD = AD + BC.
Solution :
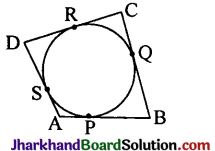
A, B, C and D are external points. Tangents drawn to the circle from these points are equal.
AP = AS ….(1) (Tangents from A)
BP = BQ ….(2) (Tangents from B)
CR = CQ ….(3) (Tangents from C)
DR = DS ….(4) (Tangents from D)
Adding the results
AP + BP + CR + DR = AS + BQ+CQ + DS
AB + CD = AS + DS + BQ +CQ
∴ AB + CD = AD + BC.
Question 9.
In the figure, XY and X’Y’ are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersecting XY at A and X’Y’ at B. Prove that ∠AOB = 90°.
Solution :
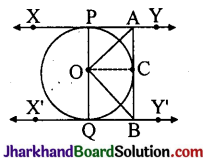
Given: XY || X’Y’.
XPA, X’QY’ and AB are the tangents.
To prove: ∠AOB = 90°
Construction: Join OC.
Proof: In ΔOAP and ΔOAC
AP = AC (Theorem 4.2)
OA = OA (Common)
OP = OC (Radii of circle)
AOAP AOAC (S.S.S. congruency)
Since XY || X’Y’
∠PAB + ∠QBA = 180° (Co-interior angles)
\(\frac{1}{2}\)∠PAB + \(\frac{1}{2}\)∠QBA = 90°
∴ ∠OAB + ∠OBA = 90°
In ΔAOB, ∠AOB = 180° – (∠OAB + ∠OBA)
= 180° – 90° = 90°
∴ ∠AOB = 90°.
The line joining the external point to the centre, bisects the angle between the tangents.
Question 10.
Prove that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line-segment joining the points of contact at the centre.
Solution :
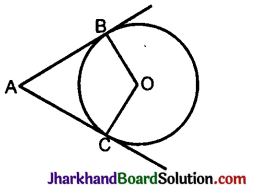
Data: O is the centre of the circle.
AB and AC are tangents drawn from A.
To prove: B\(\hat{\mathbf{A}}\)C + B\(\hat{\mathbf{O}}\)C = 180°.
Proof: AB is a tangent. BO is the radius drawn from the point of contact B.
∴ O\(\hat{\mathbf{B}}\)A = 90°
AC is a tangent. CO is the radius drawn from the point of contact C.
∴ O\(\hat{\mathbf{C}}\)A = 90°
∴ O\(\hat{\mathbf{B}}\)A + O\(\hat{\mathbf{C}}\)A = 90°.
∴ B\(\hat{\mathbf{O}}\)C + B\(\hat{\mathbf{A}}\)C = 180° [Sum of angles of a quadrilateral is 360°]
![]()
Question 11.
Prove that the parallelogram circumscribing a circle is a rhombus.
Solution :
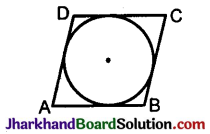
Data: ABCD is a circumscribed parallelogram.
AB = CD, AD = BC
To prove: ABCD is a rhombus.
AB = BC – CD – DA
Proof: ABCD is a circumscribed quadrilateral.
∴ AB + CD = AD + BC (Proved in problem 8)
AB = DC, AD = BC (Opposite sides of the parallelogram)
AB + AB = AD + AD
2AB = 2AD
AB = AD
AB = CD, AD = BC
AB = BC = CD = DA. [Since adjacent sides of a parallelogram are equal]
∴ ABCD is a rhombus.
Question 12.
A triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 8 cm and 6 cm respectively. Find the sides AB and AC.
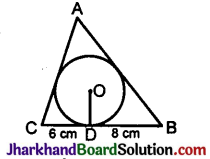
Solution :
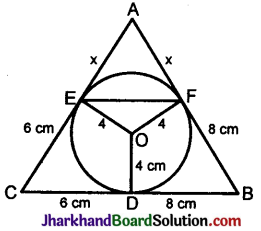
In the adjoining figure, the circle touches AB at F, BC at D, CA at E.
AE = AF = x. (Tangents to the circle from A)
Tangent BF = Tangent BD = 8 cm
Tangent CE = Tangent CD = 6 cm
AB = AF + FB = (x + 8) cm
AC = AE + EC = (x + 6) cm
CB = CD + DB = 6 + 8 = 14 cm.
2S(perimeter of AABC) = AB + BC + CA
= (x + 8) + (6 + 8) + (x + 6).
= 2x + 28
S = x + 14
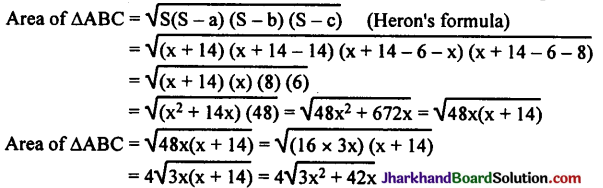
Area of ΔABC = \(\frac{1}{2}\) × base × altitude
= \(\frac{1}{2}\) × 14 × 4 = 28 cm².
Area of ΔOAB = \(\frac{1}{2}\) × 4(x + 8) = 2(x + 8)
Area of ΔOAC = \(\frac{1}{2}\) × 4(x + 6) = 2(x + 6)
Area of ΔOBC = \(\frac{1}{2}\) × 4 × 14 = 28 cm.
Total area = Area of ΔOBC + Area of ΔOAB + Area of ΔOAC
= 28 + 2x + 12 + 2x + 16
∴ 4x + 56 = 4(x + 14)
∴ 4\(\sqrt{3 x^2+42 x}\) = 4(x + 14)
\(\sqrt{3 x^2+42 x}\) = x + 14
Squaring both sides 3x² + 42x = (x + 14)² = x² + 28x + 196
= 2x² + 145 – 196 = 0 x² + 7x – 98 = 0
∴ (x + 14) (x – 7) = 0 x = – 14 or x = 7
AC = x + 8
= 7 + 8
= 15 cm.
AC = x + 6
= 7 + 6
= 13 cm.
BC = 6 + 8
= 14 cm.
![]()
Question 13.
Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
Solution :
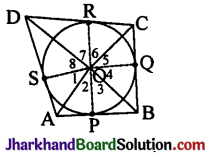
Construction: Join OP, OQ, OR and OS.
Proof: The two tangents drawn from an external point to a circle subtend equal angles at the centre.
∴ ∠1 = ∠2, ∠3 = ∠4, ∠5 = ∠6 and ∠7 = ∠8.
Now, ∠1 + ∠2 + ∠3 + ∠4 + ∠5 + ∠6 + ∠7 + ∠8 = 360° (Sum of angles subtended at a point)
2(∠2 + ∠3 + ∠6 + ∠7) = 360° and
2(∠1 + ∠8 + ∠4 + ∠5) = 360°
⇒ (∠2 + ∠3) + (∠6 + ∠7) = 180°
(∠1 + ∠8) + (∠4 + ∠5) = 180°
∴ ∠AOB + ∠COD = 180° and ∠AOD + ∠BOC = 180°.