JAC Class 10 Maths Solutions Chapter 7 Coordinate Geometry Ex 7.2

Jharkhand Board JAC Class 10 Maths Solutions Chapter 7 Coordinate Geometry Ex 7.2 Textbook Exercise Questions and Answers.

JAC Board Class 10 Maths Solutions Chapter 7 Coordinate Geometry Exercise 7.2

Question 1.
Find the coordinates of the point which divides the join of (- 1, 7) and (4, – 3) in the ratio 2 : 3.
Answer:
Let P(x, y) be the required point which divides the join of (- 1, 7) and (4, – 3) in the ratio 2 : 3.
Then, using the section formula, we get
x = \(\frac{2(4)+3(-1)}{2+3}\) = \(\frac{8-3}{5}\) = 1 and
y = \(\frac{2(-3)+3(7)}{2+3}\) = \(\frac{-6+21}{5}\) = 3
Thus, the coordinates of the point which divides the join of (- 1, 7) and (4, – 3) in the ratio 2 : 3 are (1, 3).

Question 2.
Find the coordinates of the points of trisection of the line segment joining (4, – 1) and (- 2, – 3).
Answer:
Let A (4, – 1) and B (- 2, – 3) be the given points and let P and Q be the points of trisection of AB.

Then, AP = PQ = QB
Hence, P divides AB in the ratio 1 : 2.
Therefore, the coordinates of P, by applying the section formula, are
(\(\frac{1(-2)+2(4)}{1+2}, \frac{1(-3)+2(-1)}{1+2}\)) = (2, – \(\frac{5}{3}\))
Now, Q divides AB in the ratio 2 : 1.
Therefore, the coordinates of Q, by applying the section formula, are
(\(\frac{2(-2)+1(4)}{2+1}, \frac{2(-3)+1(-1)}{2+1}\)) = (0, – \(\frac{7}{3}\))
Thus, the coordinates of the points of trisection of the line segment joining (4, – 1) and (- 2, – 3) are (2, – \(\frac{5}{3}\)) and (0, – \(\frac{7}{3}\)).

Alternative method for Q :
Here, Q is the midpoint of the line segment joining P(2, – \(\frac{5}{3}\)) and B(- 2, – 3). Therefore, by applying the midpoint formula, the coordinates of Q are
(\(\frac{2+(-2)}{2}\), \(\frac{-\frac{5}{3}+(-3)}{2}\)) = (0, – \(\frac{7}{3}\))

Question 3.
To conduct Sports Day activities, in your rectangular shaped school ground ABCD, lines have been drawn with chalk powder at a distance of 1m each. 100 flower pots have been placed at a distance of 1 m from each other along AD, as shown in the given figure. Niharika runs \(\frac{1}{4}\)th the distance AD on the 2nd line and posts a green flag. Preet runs \(\frac{1}{5}\)th the distance AD on the eighth line and posts a red flag. What is the distance between both the flags? If Rashmi has to post a blue flag exactly halfway between the line segment joining the two flags, where should she post her flag?

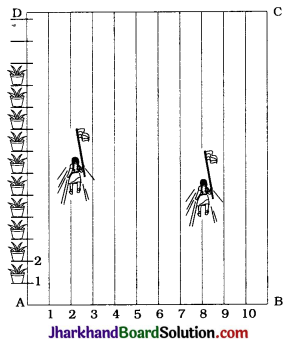
Let us consider rectangle ABCD as coordinate plane with A as the origin.
Then, AD = 100 m and each lane number will indicate its distance on the x-axis. Niharika \(\frac{1}{4}\)th the distance AD.
∴ Distance covered by Niharika = \(\frac{1}{4}\) × 100 m
= 25 m
Niharika runs on the second line.

Hence, the coordinates of the point N where Niharika posts the green flag are (2, 25). Following the same notations, the coordinates of the point P where Preet posts the red flag are (8, 20).
To find the distance (in metres) between the two flags, we have to find the length of join of N (2, 25) and P(8, 20).

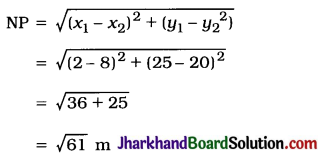
Now, Rashmi has to post a blue flag exactly halfway between the green flag and the red flag.
So, we have to find the midpoint R of the join of N (2, 25) and P(8, 20).

Then, the coordinates of R, using the midpoint formula, are
(\(\frac{2+8}{2}, \frac{25+20}{2}\)) = (5, 22.5)
This suggest that Rashmi should post the blue flag on the fifth line at distance of 22.5 m from AB.
Thus, the distance between the green flag and the red flag is \(\sqrt{61}\)m and Rashmi should post the blue flag on the 5th line at distance 22.5 m from AB.

Question 4.
Find the ratio in which the line segment joining the points (- 3, 10) and (6, – 8) is divided by (- 1, 6).
Answer:
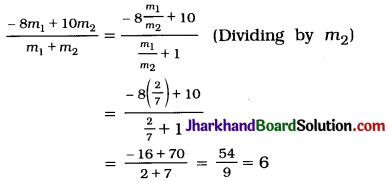
Let P(- 1, 6) divide internally the line segment joining the points A(- 3, 10) and B (6, – 8) in the ratio m₁ : m₁.
Using the section formula, we get

Comparing the x-coordinates on both the sides, we get
∴ – 1 = \(\frac{6 m_1-3 m_2}{m_1+m_2}\)
∴ – m₁ – m< sub>2 = 6m₁ – 3m₂
∴ 7m₁ = 2m₂
∴ \(\frac{m_1}{m_2}=\frac{2}{7}\)
∴ m₁ : m₂ = 2 : 7
Then, to verify the y-coordinate,

Thus, the point (- 1, 6) divides the line segment joining the points (- 3, 10) and (6, – 8) in the ratio 2 : 7.

Question 5.
Find the ratio in which the line segment joining A(1, 5) and B (- 4, 5) is divided by the x-axis. Also find the coordinates of the point of division.
Answer:
Let the ratio in which the line segment joining A(1, – 5) and B(- 4, 5) is divided by the x-axis be k : 1.
Then, by the section formula, the coordinates of the point which divides AB in the ratio k : 1 are
(\(\frac{k(-4)+1(1)}{k+1}, \frac{k(5)+1(-5)}{k+1}\)), i.e. (\(\frac{1-4 k}{k+1}\), \(\frac{5 k-5}{k+1}\))
Now, the point of division is on the x-axis.
Hence, its y-coordinate is 0.
∴ \(\frac{5 k-5}{k+1}\) = 0
∴ k = 1
Hence, the required ratio k : 1 is 1 : 1.
Now, the coordinates of the point of division

Thus, the x-axis divides the line segment joining A(1, – 5) and B(- 4, 5) in the ratio 1 : 1 at the point (-\(\frac{3}{2}\), 0).

Question 6.
If (1, 2), (4, y), (x, 6) and (3, 5) are the vertices of a parallelogram taken in order, find x and y.
Answer:
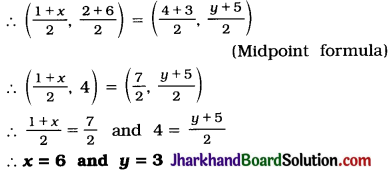
Let A(1, 2), B(4, y), C(x, 6) and D(3, 5) be the vertices of parallelogram ABCD. As ABCD is a parallelogram, its diagonals AC and BD bisect each other.
∴ Midpoint of AC = Midpoint of BD

Question 7.
Find the coordinates of a point A, where. AB is the diameter of a circle whose centre is (2, – 3) and B is (1, 4).
Answer:
Let the coordinates of A be (x, y).
In a circle, centre is the midpoint of every diameter.
Here, AB is a diameter and (2, – 3) is the centre.
∴ Midpoint of AB = (2, – 3)
∴ (\(\frac{x+1}{2}, \frac{y+4}{2}\)) = (2, – 3)
∴ \(\frac{x+1}{2}\) = 2 and \(\frac{y+4}{2}\) = – 3
∴ x = 3 and y = – 10
Thus, the coordinates of A are (3, -10).

Question 8.
If A and B are (- 2, – 2) and (2, 4). respectively, find the coordinates of P such that AP = \(\frac{3}{7}\)AB and P lies on the line segment AB.
Answer:
A(- 2, – 2) and B (2, – 4) are given points and point P(x, y) lies on line segment AB such that AP = \(\frac{3}{7}\)AB.
Then, PB = AB – AP = AB – \(\frac{3}{7}\)AB = \(\frac{4}{7}\)AB.
Now, \(\frac{AP}{PB}\) = \(\frac{\frac{3}{7} \mathrm{AB}}{\frac{4}{7} \mathrm{AB}}\) = \(\frac{3}{4}\)
Thus, P(x, y) divides AB in the ratio 3 : 4.
Then, by section formula
(x, y) = (\(\frac{3(2)+4(-2)}{3+4}, \frac{3(-4)+4(-2)}{3+4}\))
∴ (x, y) = (-\(\frac{2}{7}\), – \(\frac{20}{7}\))
Thus, the coordinates of P are (-\(\frac{2}{7}\), – \(\frac{20}{7}\))

Question 9.
Find the coordinates of the points which divide the line segment joining A(-2, 2) and B (2, 8) into four equal parts.

Answer:
Let P, Q and R be the points which divide the line segment AB joining A(-2, 2) and B(2, 8) into four equal parts.
Then, Q is the midpoint of AB.
Hence, by midpoint formula, the coordinates of Q are
(\(\frac{-2+2}{2}, \frac{2+8}{2}\)) = (0, 5)
Now, P is the midpoint of Ag.
Hence, by midpoint formula, the coordinates of P are
(\(\frac{-2+0}{2}, \frac{2+5}{2}\)) = (-1, \(\frac{7}{2}\))
Again, R is the midpoint of QB.
Hence, by midpoint formula, the coordinates of R are
(\(\frac{0+2}{2}, \frac{5+8}{2}\)) = (1, \(\frac{13}{2}\))
Thus, the coordinates of the required points are (- 1, \(\frac{7}{2}\)), (0, 5) and (1, \(\frac{13}{2}\)).

Question 10.
Find the area of a rhombus if its vertices are (3, 0), (4, 5), (- 1, 4) and (- 2, – 1) taken in order.
[Hint: Area of a rhombus = \(\frac{1}{2}\) (product of its diagonals)]
Answer:
Let A (3, 0), B(4, 5), C(-1, 4) and D(-2, -1) be the vertices of rhombus ABCD.
Diagonal AC = \(\sqrt{(3+1)^2+(0-4)^2}\)
= \(\sqrt{32}\) = 4 \(\sqrt{2}\) units
Diagonal BD = \(\sqrt{(4+2)^2+(5+1)^2}\)
= \(\sqrt{72}\) = 6\(\sqrt{2}\) units
Now, area of a rhombus
= \(\frac{1}{2}\)(Products of its diagonals)
∴ Area of rhombus ABCD = \(\frac{1}{2}\) × AC × BD
= \(\frac{1}{2}\) × 4\(\sqrt{2}\) × 6\(\sqrt{2}\)
= 24 sq units
Thus, the area of given rhombus with vertices (3, 0), (4, 5), (- 1, 4) and (- 2, – 1) is 24 sq units.