Jharkhand Board JAC Class 9 Maths Solutions Chapter 7 Triangles Ex 7.5 Textbook Exercise Questions and Answers.
JAC Board Class 9th Maths Solutions Chapter 7 Triangles Ex 7.5
Page-133
Question 1.
ABC is a triangle. Locate a point in the interior of ΔABC which is equidistant from all the vertices of ΔABC.
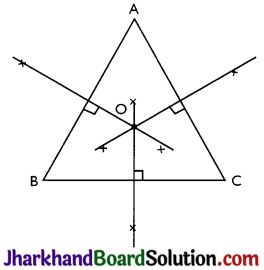
Answer:
In ΔABC, we can find the circumcentre by drawing the perpendicular bisectors of sides AB, BC and CA of this triangle. 0 is the point where these bisectors meet. Therefore, O is the point which is equidistant from all the vertices of ΔABC.
Question 2.
In a triangle locate a point in its interior which is equidistant from all the sides of the triangle.
Answer:
The point which is equidistant from all the sides of a triangle is called the incentre of the triangle. Incentre of a triangle is the intersection point of the angle bisectors of the interior angles of that triangle.

In ΔABC, we can the find the incentre of this triangle by drawing the angle bisectors of the interior angles ∠A, ∠B and ∠C of this triangle. O is the point where these angle bisectors meet. Therefore, O is the point equidistant from all the sides of ΔABC.
![]()
Question 3.
In a huge park people are concentrated at three points (see the given figure)
A: where there are different slides and swings for children,
B: near which a man-made lake is situated.
C: which is near to a large parking and exit.

Answer:
Maximum number of persons can approach the ice-cream parlour if it is equidistant from A, B and C. Now, A, B and C form a triangle. In a triangle, at the circumcentre is the only point that it equidistant from its vertices. So, the ice-cream parlour should be set up at the circumcentre of ΔABC.
Question 4.
Complete the hexagonal and star shaped rangolies (see the given figure) by filling them with as many equilateral triangles of side 1 cm as you can. Count the number of triangles in each case. Which has more triangles?

Answer:
It can be observed that hexagonal-shaped rangoli has 6 equilateral triangles in it.
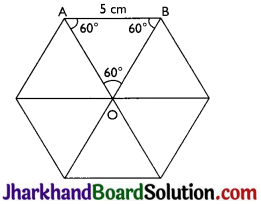
Area of ∆OAB = \(\frac{\sqrt{3}}{4}\) (side)2 = \(\frac{\sqrt{3}}{4}\) (5)2
= \(\frac{\sqrt{3}}{4}\) (25) = \(\frac{25 \sqrt{3}}{4}\) cm2
Area of hexagonal-shaped rangoli
= \(6 \times \frac{25 \sqrt{3}}{4}=\frac{75 \sqrt{3}}{2}\) cm2
Area of equilateral triangle having its side as 1 cm = \(\frac{\sqrt{3}}{4}\) (1)2 = \(\frac{\sqrt{3}}{4}\) cm2
Number of equilateral triangles of 1 cm side that can be filled in this hexagonal-shaped rangoli
= \(\frac{\frac{75 \sqrt{3}}{2}}{\frac{\sqrt{3}}{4}}\) = 150
Star-shaped rangoli has 12 equilateral triangles of side 5 cm in it.

Area of star-shaped rangoli
= \(12 \times \frac{\sqrt{3}}{4} \times(5)^2=75 \sqrt{3}\) cm2
Number of equilateral triangles of 1 cm side that can be filled in this star-shaped rangoli
= \(\frac{75 \sqrt{3}}{\frac{\sqrt{3}}{4}}\) = 300
Therefore, star-shaped rangoli has more equilateral triangles in it.