Jharkhand Board JAC Class 9 Maths Solutions Chapter 14 Statistics Ex 14.4 Textbook Exercise Questions and Answers.
JAC Board Class 9th Maths Solutions Chapter 14 Statistics Ex 14.4
Page-269
Question 1.
The following number of goals were scored by a team in a series of 10 matches :
2, 3, 4, 5,0, 1, 3, 3, 4, 3
Find the mean, median and mode of these scores.
Answer:

For median, we will arrange the given data in ascending order,
0, 1, 2, 3, 3, 3, 3,4, 4,5
Number of observations (n) = 10
Number of observations is even so we will calculate median as,
Median
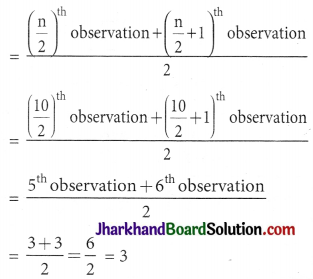
For mode, we will arrange the given data in ascending order, we have
0, 1, 2, 3, 3, 3, 3,4, 4,5
Here, 3 occurs most frequently (4 times)
Mode = 3
![]()
Question 2.
In a mathematics test given to 15 students, the following marks (out of 100) are recorded:
41, 39, 48, 52, 46, 62, 54, 40, 96, 52, 98, 40, 42, 52, 60.
Find the mean, median and mode of this data.
Answer:

For median, we will arrange the given data in ascending order,
39,40, 40, 41, 42,46, 48, 52, 52, 52, 54, 60, 62, 96, 98
Number of observations (n) = 15
Number of observations is odd so we will calculate median as,
Median = \(\left(\frac{\mathrm{n}+1}{2}\right)^{\mathrm{th}}\) observation
= \(\left(\frac{15+1}{2}\right)^{\mathrm{th}}\) observation
= 8th observation = 52
For Mode, we will arrange the given data in ascending order, we have
39, 40, 40, 41, 42,46, 48, 52, 52, 52, 54, 60, 62, 96, 98
Here, 52 occurs most frequently (3 times)
∴ Mode = 52
Question 3.
The following observations have been arranged in ascending order. If the median of the data is 63, find the value of x.
29, 32, 48, 50, x, x + 2, 72, 78, 84, 95
Answer:
Number of observations (n) = 10 (even)
Median
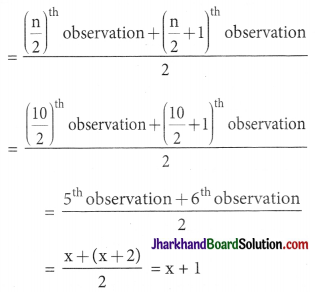
According to question, median = 63
∴ x + 1 = 63 ⇒ x = 63 – 1 = 62
Hence, the value of x is 62.
Question 4.
Find the mode of 14, 25, 14, 28, 18, 17, 18, 14, 23, 22,14, 18.
Answer:
The given data is,
14, 25, 14, 28, 18, 17, 18, 14, 23, 22, 14, 18
Arranging the data in ascending order,
14, 14, 14, 14, 17, 18, 18, 18, 22, 23, 25, 28
Here, 14 occurs most frequently (4 times).
Mode = 14
Question 5.
Find the mean salar y of 60 workers of a factory from the following table:
| Salary (in Rs.) | Number of workers |
| 3000 | 16 |
| 4000 | 12 |
| 5000 | 10 |
| 6000 | 8 |
| 7000 | 6 |
| 8000 | 4 |
| 9000 | 3 |
| 10000 | 1 |
| Total | 60 |
Answer:
| Salary (in Rs.) xi | Number of workers fi | fixi |
| 3000 | 16 | 48000 |
| 4000 | 12 | 48000 |
| 5000 | 10 | 50000 |
| 6000 | 8 | 48000 |
| 7000 | 6 | 42000 |
| 8000 | 4 | 32000 |
| 9000 | 3 | 27000 |
| 10000 | 1 | 10000 |
| \(\sum f_i=60\) = 60 | \(\sum f_i x_i\) = 305000 |
\(\bar{x}(\text { mean })=\frac{\sum f_i x_i}{\sum f_i}=\frac{305000}{60}\)
= ₹ 5083.33
Hence, the mean salary is ₹ 5083.33.
![]()
Question 6.
Give one example of a situation in which
(i) the mean is an appropriate measure of central tendency.
(ii) the mean is not an appropriate measure of central tendency but the median is an appropriate measure of central tendency.
Answer:
(i) Mean marks in a test in mathematics.
(ii) Average beauty