JAC Class 9 Maths Important Questions Chapter 13 Surface Areas and Volumes

Jharkhand Board JAC Class 9 Maths Important Questions Chapter 13 Surface Areas and Volumes Important Questions and Answers.

JAC Board Class 9th Maths Important Questions Chapter 13 Surface Areas and Volumes

Question 1.
Three equal cubes are placed adjacently in a row. Find the ratio of the total surface area of the new cuboid to that of the sum of the surface areas of three cubes.
Solution :
Let the side of each of the three equal cubes be a cm.
Then, surface area of one cube = 6a² cm²
∴ Sum of the surface areas of three cubes = 3 × 6a² = 18 cm²
For new cuboid
length (l) = 3a cm
breadth (b) = a cm
height (h) = a cm
∴ Total surface area of the new cuboid = 2(l × b + b × h + h × l)
= 2[3a × a + a × a + a × 3a]
= 2[3a² + a² + 3a²] = 14a² cm²
∴ Required ratio
= Total surface area of the new cuboid / Sum of the surface areas of three cubes
= \(=\frac{14 \mathrm{a}^2}{18 \mathrm{a}^2}\) = \(\frac {7}{9}\)
= 7 : 9

Question 2.
A classroom is 7 m long, 6.5 m wide and 4 m high. It has one door 3 m × 1.4 m and three windows each measuring 2 m × 1 m. The interior walls are to be colour-washed. The contractor charges ₹ 15 per sq. m. Find the cost of colour washing.
Solution :
l = 7 m, b = 6.5 m and h = 4 m
∴ Area of the walls of the room including door and windows = 2(l + b)h
= 2(7 + 6.5) 4 = 108 m²
Area of door = 3 × 1.4 = 4.2 m
Area of one window = 2 × 1 = 2 m²
∴ Area of 3 windows = 3 × 2 = 6 m²
∴ Area of the walls of the room to be colour washed = 108 – (4.2 + 6)
= 108 – 10.2 = 97.8 m
∴ Cost of colour washing at the rate of ₹ 15 per square metre = ₹ 97.8 × 15 = ₹ 1467

Question 3.
A cylindrical vessel, without lid, has to be tin coated including both of its sides. If the radius of its base is \(\frac {1}{2}\) m and its height is 1.4 m, calculate the cost of tincoating at the rate of ₹ 50 per 1000 cm² (Use π = 3.14)
Solution :
Radius of the base (r) = \(\frac {1}{2}\)m
= \(\frac {1}{2}\) × 100 cm = 50 cm
Height (h) = 1.4 m = 1.4 × 100 cm
= 140 cm.
Surface area to be tin-coated
= 2 (2πh + πr²)
= 2[2 × 3.14 × 50 × 140 + 3.14 × (50)²]
= 2 [43960 + 7850]
= 2(51810)
= 103620 cm²
∴ Cost of tin-coating at the rate of ₹ 50 per 1000 cm²
= \(\frac {50}{1000}\) × 103620 = ₹ 5181.

Question 4.
The diameter of a roller 120 cm long is 84 cm. If its takes 500 complete revolutions to level a playground, determine the cost of levelling it at the rate of ₹ 25 per square metre. (Use π = \(\frac {22}{7}\))
Solution :
diameter of roller = 84 cm
∴ r = \(\frac {84}{2}\) cm = 42 cm
h = 120 cm
Area of the playground levelled in one complete revolution = 2πrh
= 2 × \(\frac {22}{7}\) × 42 × 120 = 31680 cm²
∴ Area of the playground
= 31680 × 500cm² = \(\frac{31680 \times 500}{100 \times 100}\)m²
= 1584 m²
∴ Cost of levelinga at the rate of ₹ 25 per square metre = ₹ 1584 × 25 = ₹ 39600.

Question 5.
How many metres of cloth of 1.1 m width will be required to make a conicaltent whose vertical height is 12 m and base radius is 16 m? Find also the cost of the cloth used at the rate of ₹ 14 per metre.
Solution :
h = 12 m, r = 16 m
∴ l = \(\sqrt{\mathrm{r}^2+\mathrm{h}^2}\)
= \(\sqrt{(16)^2+(12)^2}=\sqrt{256+144}\)
= \(\sqrt{400}\) = 20 m
∴ Curved surface area = πrl
= \(\frac {22}{7}\) × 16 × 20 = \(\frac {7040}{7}\)m²
Width of cloth = 1.1 m
∴ Length of cloth
= \(\frac{7040 / 7}{1.1}=\frac{70400}{77}=\frac{6400}{7}\) m
∴ Cost of the cloth used at the rate of ₹ 14 per metre
= ₹ \(\frac {6400}{7}\) × 14 = ₹ 12800

Question 6.
The surface area of a sphere of radius 5 cm is five times the area of the curved surface of cone of radius 4 cm. Find the height of the cone.
Solution :
Surface area of cone of radius 4 cm = π(4)lcm² where, l cm is the slant height of the cone.
Surface area of sphere of radius 5 cm = π(5)²
According to the question,
4π (5)² = 5[π(4)l]
⇒ l = 5 cm
⇒ \(\sqrt{\mathrm{r}^2+\mathrm{h}^2}\) = 5
⇒ r² + h² = 25
⇒ (4)² + h² = 25
⇒ 16 + h² = 25
⇒ h² = 9
⇒ h = 3
Hence, the height of the cone is 3 cm.

Question 7.
The dimensions of a cinema hall are 100 m, 50 m and 18 m. How many persons can sit in the hall, if each required 150 m³ of air?
Solution :
l = 100 m, b = 50 m, h = 18 m
∴ Volume of the cinema hall = lbh
= 100 × 50 × 18 = 90000 m³
Volume occupied by 1 person = 150 m³
∴ Number of persons who can sit in the hall
= Volume of the hall / Volume occupied by 1 person
= \(\frac {90000}{150}\) = 600
Hence, 600 persons can sit in the hall.

Question 8.
The outer measurements of a closed wooden box are 42 cm, 30 cm and 27 cm. If the box is made of 1 cm thick wood, determine the capacity of the box.
Solution :
Outer dimensions :
l = 42 cm, b = 30 cm, h = 27 cm
Thickness of wood = 1 cm
Inner dimensions :
l = 42 – (1 + 1) = 40 cm
b = 30 – (1 + 1) = 28 cm
h = 27 – (1 + 1) = 25 cm
∴ Capacity of the box = l × b × h = 40 × 28 × 25 = 28000 cm³.

Question 9.
If v is the volume of a cuboid of dimensions a, b and c and s is its surface area, then prove that:
\(\frac{1}{v}=\frac{2}{s}\left(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\right)\)
Solution :
L.H.S. = \(\frac {1}{v}\) = \(\frac {1}{abc}\)
R.H.S. = \(\frac{2}{s}\left(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\right)\)
= \(\frac{2}{2(a b+b c+c a)}\) (\(\frac{b c+c a+a b}{a b c}\))
= \(\frac {1}{abc}\) ……………(ii)
From (i) and (ii), we have,
\(\frac{1}{v}=\frac{2}{s}\left(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\right)\)

Question 10.
The ratio of the volumes of the two cones is 4 : 5 and the ratio of the radii of their bases is 2 : 3. Find the ratio of their vertical heights.
Solution :
Let the radii of bases, vertically heights and volumes of the two cones be r₁, h₁, v₁ and r₂, y₂, v₂ respectively.
According to the question,

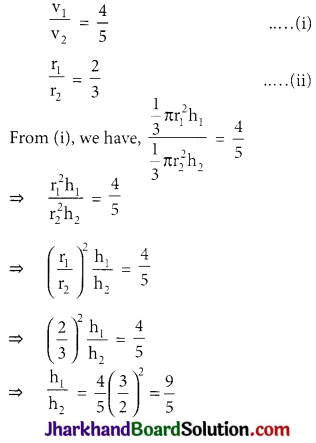
Hence the ratio of their vertical heights is 9 : 5.

Question 11.
If h, c and v be the height, curved surface and volume of a cone, show that
3πvh³ – c²h² + 9v² = 0.
Solution :
Let the radius of the base and slant height of the cone be r and l respectively. Then;
C = curved surface area = πrl
= πr\(\sqrt{\mathrm{r}^2+\mathrm{h}^2}\) ………..(i)
v = volume = \(\frac {1}{3}\)πr²h ………..(ii)
∴ 3πvh³ – c²h² + 9v²
= 3πh³(\(\frac {1}{3}\)πr²h) – π²r²(r² + h²)h² + 9(\(\frac {1}{3}\)πr²h)²
[Using (i) and (ii)]
= π²r²h⁴ – π²r⁴h⁴ – π²r²h⁴ – π²r²h²
= 0.
Hence Proved.

Question 12.
How many balls, each of radius 1 cm, can be made from a solid sphere of lead of radius 8 cm?
Solution :
Volume of the spherical ball of radius 8 cm
= \(\frac {4}{3}\)π × 8³cm³
Also, volume of each smaller spherical ball of radius 1 cm
= \(\frac {4}{3}\)π × 1³cm³
Let n be the number of smaller balls that can be made. Then, the volume of the larger ball is equal to the sum of all the volumes of n smaller balls.
Hence, \(\frac {4}{3}\)π × n = \(\frac {4}{3}\)π × 8³
⇒ n = 8³ = 512
Hence, the required number of balls = 512.

Question 13.
By melting a solid cylindrical metal, a few conical materials are to be made. If three times the radius of the cone is equal to twice the radius of the cylinder and the ratio of the height of the cylinder and the height of the cone is 4 : 3, find the number of cones which can be made.
Solution :
Let R be the radius and H be the height of the cylinder and let r and h be the radius and height of the cone respectively. Then,
3r = 2R
And, H : h = 4 : 3 …………..(i)
\(\frac{\mathrm{H}}{\mathrm{h}}=\frac{4}{3}\)
⇒ 3H = 4h …………..(ii)
Let n be the required number of cones which can be made from the materials of the cylinder. Then, the volume of the cylinder will be equal to the sum of the volumes of n cones. Hence, we have
πR²H = \(\frac {n}{3}\)πr²h
3R²H = nr²h
n = \(\frac{3 R^2 H}{r^2 h}=\frac{3 \times \frac{9 r^2}{4} \times \frac{4 h}{3}}{r^2 h}\) = 9
[From (i) and (ii), R = \(\frac {3r}{2}\) and H = \(\frac {4h}{3}\)]
Hence, the required number of cones is 9.

Question 14.
Water flows at the rate of 10 m per minute through a cylindrical pipe having its diameter as 5 mm. How much time will it take to fill a conical vessel whose diameter of the base is 40 cm and depth 24 cm?
Solution :
Diameter of the pipe = 5 mm
= \(\frac {5}{10}\)cm = \(\frac {1}{2}\)cm
∴ Radius of the pipe = \(\frac{1}{2} \times \frac{1}{2}\) cm
= \(\frac {1}{4}\)cm.
In 1 minute, the length of the water column in the cylindrical pipe = 10 m = 1000 cm.
∴ Volume of water that flows out of the pipe in 1 minute
= π × \(\frac {1}{4}\) × \(\frac {1}{4}\) × 1000 cm³
Also, volume of the cone
= \(\frac {1}{3}\) × π × 20 × 20 × 24 cm³.
Hence, the time needed to fill up this conical vessel
= \(\frac{\frac{1}{3} \pi \times 20 \times 20 \times 24}{\pi \times \frac{1}{4} \times \frac{1}{4} \times 1000}\) minutes
= (\(\frac{20 \times 20 \times 24}{3} \times \frac{4 \times 4}{1000}\))minutes
= \(\frac {256}{5}\) minutes
= 51.2 minutes.
Hence, the required time is 51.2 minutes.

Multiple Choice Questions

Question 1.
The height of a conical tent at the centre is 5 m. The distance of any point on its circular base from the top of the tent is 13 m. The area of the slant surface is:
(a) 144 π sq. m
(b) 130 π sq.m
(c) 156 π sq.m
(d) 169 π sq.m
Solution :
(c) 156 π sq.m

Question 2.
A rectangular sheet of paper 22 cm long and 12 cm broad can be curved to form the lateral surface of a right circular cylinder in two ways. Taking π = \(\frac {22}{7}\), the difference between the volumes of the two cylinders thus formed is :
(a) 200 cm³
(b) 210 cm³
(c) 250 cm³
(d) 252 cm³
Solution :
(b) 210 cm³

Question 3.
The percentage increase in the surface area of a cube when each side is increased two times the original length
(a) 225
(b) 200
(c) 175
(d) 300
Solution :
(d) 300

Question 4.
A cord in the form of a square enclose the area ‘S’ cm. If the same cord is bent into the form of a circle, then the area of the circle is
(a) \(\frac{\pi \mathrm{S}^2}{4}\)
(b) 4πS²
(c) \(\frac{4 \mathrm{~S}^2}{\pi}\)
(d) \(\frac {4S}{π}\)
Solution :
(c) \(\frac{4 \mathrm{~S}^2}{\pi}\)

Question 5.
If ‘l’, ‘B’ and ‘h’ of a cuboids are increased, decreased and increased by 1%, 3% and 2% respectively, then the volume of the cuboid
(a) increases
(b) decreases
(c) increases or decreases depending on original dimensions
(d) can’t be calculated with given data
Solution :
(b) decreases

Question 6.
The radius and height of a cone are each increased by 20%, then the volume of the cone is increased by (a) 20%
(b) 40%
(c) 60%
(d) 72.8%
Solution :
(d) 72.8%

Question 7.
There is a cylinder circumscribing the hemisphere such that their bases are common. The ratio of their volumes is
(a) 1 : 3
(b) 1 : 2
(c) 2 : 3
(d) 3 : 4
Solution :
(d) 3 : 4

Question 8.
Consider a hollow cylinder of inner radius r and thickness of wall t and length l. The volume of the above cylinder is given by
(a) 2πl(r² – l²)
(b) 2πrlt(\(\frac {t}{2r}\) + l)
(c) 2πl(r² + l²)
(d) 2πrl(r + l)
Solution :
(b) 2πrlt(\(\frac {t}{2r}\) + l)

Question 9.
A cone and a cylinder have the same base area. They also have the same curved surface area. If the height of the cylinder is 3 m, then the slant height of the cone (in m) is
(a) 3 m
(b) 4 m
(c) 6 m
(d) 7 m
Solution :
(c) 6 m

Question 10.
A sphere of radius 3 cm is dropped into a cylindrical vessel of radius 4 cm. If the sphere is submerged completely, then the height (in cm) to which the water rises, is
(a) 2.35 cm
(b) 2.30 cm
(c) 2.25 cm
(d) 2.15 cm
Solution :
(c) 2.25 cm