Class 10

Students should go through these JAC Class 10 Maths Notes Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन will seemingly help to get a clear insight into all the important concepts.

JAC Board Class 10 Maths Notes Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन

भूमिका (Introduction) :
पिछली कक्षाओं में हम ठोस आकृतियों जैसे घनाभ, घन, शंकु, बेलन और गोला के पृष्ठीय क्षेत्रफल तथा आयतन के बारे में अध्ययन कर चुके है। इस अध्याय में पिछले ज्ञान का उपयोग करते हुए दो या दो से अधिक ठोस आकृतियों के संयोजन से बनी आकृतियों के पृष्ठीय क्षेत्रफल तथा आयतन का अध्ययन करेंगे। हम ऐसी ठोस आकृतियों के बारे में भी अध्ययन करेंगे जो एक शंकु को उसके आधार के समान्तर एक तल द्वारा काटने पर प्राप्त होते है। उदाहरण के लिए बाल्टी, ग्लास, फ्रिक्शन क्लच आदि।
→ ठोस (Solid) : वस्तुएँ जिनकी अन्तरिक्ष में तीन भुजाएँ अर्थात् (लम्बाई, चौड़ाई, ऊँचाई) हों ठोस कहलाते हैं।
→ आयतन (Volume) : किसी ठोस द्वारा अन्तरिक्ष के घेरे गए स्थान के परिमाण को उसका आयतन कहते हैं।
→ पृष्ठीय क्षेत्रफल (Surface area) : किसी ठोस के सभी पृष्ठों के क्षेत्रफलों के योग को उसका पृष्ठीय क्षेत्रफल कहते हैं।
→ वक्त पृष्ठीय क्षेत्रफल (Lateral surface area) : किसी ठोस के आधार क्षेत्रफलों को छोड़कर शेष बचे सभी पृष्ठों के क्षेत्रफलों के योग को वक्र पृष्ठीय क्षेत्रफल कहते हैं।
→ घनाभ (Cuboid): छ: आयताकार पृष्ठों से घिरी ठोस बन्द आकृति को घनाभ कहते हैं।
→ घन (Cube) : यदि घनाभ की प्रत्येक कोर लम्बाई में समान हो, तो उसे घन कहते हैं।
→ लम्ब वृत्तीय शंकु का छिन्नक (Frustum of a right circular cone) : यदि किसी लम्ब वृत्तीय शंकु को उसके आधार के समान्तर किसी तल द्वारा काटा जाये तो कटने वाले तल तथा आधार के बीच के भाग को शंकु का छिन्नक कहते हैं।

घन (Cube) और घनाभ (Cuboid) :
घनाभ (Cuboid) : यदि समान्तर षट्फलक का प्रत्येक फलक आयत हो, तो उसे घनाभ कहते हैं। घनाभ को आयतफलकी ठोस भी कहते हैं जैसे ईंट, सन्दूक, कमरा आदि घनाभ हैं घनाभ में छः पृष्ठ (फलक), 8 शीर्ष व 12 कोरें होती हैं।
चित्र से स्पष्ट है कि घनाभ के आमने-सामने के फलक परस्पर समान होते हैं।

1. घनाभ के फलक ABCD का क्षेत्रफल = फलक A’B’CD’ का क्षेत्रफल = लम्बाई × चौड़ाई
2. घनाभ के फलक ADD’A’ का क्षेत्रफल = फलक BCC’B’ का क्षेत्रफल = चौड़ाई × ऊँचाई
3. घनाभ के फलक ABB’A’ का क्षेत्रफल = फलक DCC’D’ का क्षेत्रफल = ऊँचाई × ऊँचाई
4. घनाभ का सम्पूर्ण पृष्ठीय क्षेत्रफल = 2 (लम्बाई × चौड़ाई + चौड़ाई × ऊँचाई + ऊँचाई × लम्बाई) वर्ग इकाई
5. घनाभ की चारों दीवारों का क्षेत्रफल = 2 × ऊँचाई (लम्बाई + चौड़ाई) वर्ग इकाई
अथवा
= (ऊँचाई × परिमाप) वर्ग इकाई।

घन (Cube) : यदि घनाभ का प्रत्येक फलक वर्गाकार हो, तो उसे घन कहते हैं। अर्थात् घन की लम्बाई, चौड़ाई और ऊँचाई बराबर होती हैं।
∴ घन का प्रत्येक पृष्ठ वर्गाकार होता है।
घन के एक पृष्ठ का क्षेत्रफल = (भुजा)²
∴ घन के 6 पृष्ठों का क्षेत्रफल = 6(भुजा)²
अतः घन का सम्पूर्ण पृष्ठीय क्षेत्रफल = 6(भुजा)² वर्ग इकाई

घन और घनाभ के विकर्ण (Diagonals of Cube and Cuboid)
घन या घनाभ के समान्तर फलक के दो सम्मुख शीर्षों को मिलाने वाली रेखा विकर्ण कहलाती है अतः घन एवं मैं कुल 4 विकर्ण होते हैं।
1. घनाभ के विकर्ण की लम्बाई =
2. घन के विकर्ण की लम्बाई = भुजा \(\sqrt{3}\) इकाई
घन और घनाभ का आयतन (Volume of Cube and Cuboid)
प्रत्येक ठोस आकृति स्थान घेरती है। अतः ठोस आकृति द्वारा घेरे गये स्थान की माप को आयतन कहा जाता है।
घनाभ का आयतन = (लम्बाई × चौड़ाई × ऊँचाई ) घन इकाई तथा घन का आयतन = (भुजा)³ घन इकाई।

लम्बवृत्तीय बेलन और शंकु :
लम्बवृत्तीय बेलन (Right Circular Cylinder) :
परिभाषा- किसी ‘आयत’ की एक भुजा को स्थिर रखकर उसके परित: आयत को घुमाने पर बने ठोस को लम्बवृत्तीय बेलन कहते हैं।
संलग्न चित्र में आयत OABC की भुजा OA को स्थिर मानकर उसके परितः आयत को घुमाने पर बने लभ्यवृत्तीय बेलन को दिखाया गया है।
स्थिर भुजा OA की लम्बाई को बेलन की ऊँचाई कहते हैं तथा बिन्दु O और A इसके वृत्तीय सिरों के केन्द्र कहलाते हैं। OC अथवा AB इसके वृत्तीय आधार की त्रिज्या हैं। आयत को उसकी किसी एक भुजा के परितः घुमाने पर लम्बवृत्तीय बेलन प्राप्त होता है।

लम्बवृत्तीय बेलन से सम्बन्धित सूत्र
1. बेलन के आधार का क्षेत्रफल = वृत्त का क्षेत्रफल = πr² वर्ग इकाई
2. बेलन के आधार की लम्बाई = वृत्त की परिधि = 2πr इकाई
3. बेलन के वक्रपृष्ठ का क्षेत्रफल (C.S.A.) = आधार की परिधि × ऊँचाई
= 2πrh वर्ग इकाई
4. बेलन का सम्पूर्ण पृष्ठीय क्षेत्रफल (T.S.A.) = वक्रपृष्ठ का क्षेत्रफल + दोनों वृत्तीय आधारों का क्षेत्रफल
= 2πrh + 2πr² = 2πr (h + r) वर्ग इकाई
5. बेलन का आयतन = आधार का क्षेत्रफल × ऊँचाई = πr² × h = πr2h घन इकाई

खोखला बेलन (Hollow Cylinder)- यदि r₁ व r₂ खोखले बेलन की बाह्य और अन्तः त्रिज्याएँ हों तथा ऊँचाई h हो तो :
1. खोखले बेलन के प्रत्येक सिरे का क्षेत्रफल = π(r₁² – r₂²)
2. खोखले बेलन के वक्रपृष्ठ का क्षेत्रफल = बाह्य पृष्ठ का क्षेत्रफल + अन्तः पृष्ठ का क्षेत्रफल
= 2πr₁h + 2πr₂h = 2π(r₁ + r₂)h
3. खोखले बेलन का सम्पूर्ण पृष्ठीय क्षेत्रफल = वक्रपृष्ठ का क्षेत्रफल + 2 × (एक सिरे का क्षेत्रफल)
= 2π(r₁ + r₂)h + 2π(r₁² – r₂²)
= 2π(r₁ + r₂)h + 2π(r₁ + r2) (r₁ – r₂)
= 2π(r₁ + r₂) (h + r₁ – r₂)
4. खोखले बेलन का आयतन = बाह्य बेलन का आयतन – अन्तः बेलन का आयतन
= πr₁²h – πr₂²h = π(r₁² – r₂²)h

लम्बवृत्तीय शंकु (Right Circular Cone) :
परिभाषा- किसी समकोण त्रिभुज की समकोण बनाने वाली दो भुजाओं में से एक को स्थिर मानकर, त्रिभुज को उसके परितः घुमाने पर बने ठोस को लम्बवृत्तीय शंकु कहते हैं।
चित्र में समकोण ΔVOA को भुजा OV के परित: घुमाने पर प्राप्त लम्बवृत्तीय शंकु को दिखाया गया है, जिसका शीर्ष V तथा वृत्तीय आधार का केन्द्र O है।

यहाँ शंकु की ऊँचाई = OV = h
शंकु की तिरछी ऊँचाई = VA = l
शंकु के आधार की त्रिज्या = ∠OAP = α
शंकु का अर्द्ध-शीर्ष कोण
समकोण ΔVOA में, VA² = OV² + OA²
l² = h² + h²
l = \(\sqrt{h^2+r^2}\)
शंकु की तिरछी ऊँचाई (l) = \(\sqrt{h^2+r^2}\)

लम्बवृत्तीय शंकु से सम्बन्धित सूत्र :
1. शंकु का वक्रपृष्ठीय क्षेत्रफल (C.S.A.) = \(\frac{1}{2}\) × वृत्तीय आधार की परिधि × तिरछी ऊँचाई
= \(\frac{1}{2}\) × 2πr × l = πrl
2. शंकु का सम्पूर्ण पृष्ठीय क्षेत्रफल (T.S.A.) = वक्र पृष्ठ + वृत्तीय आधार का क्षेत्रफल
= πrl + πr² = πr(l + r)
3. शंकु का आयतन = \(\frac{1}{3}\) × वृत्तीय आधार का क्षेत्रफल × ऊँचाई = \(\frac{1}{3}\)πr²h
यदि शंकु का अर्द्ध-शीर्ष कोण α हो तो उसकी :
(i) ऊँचाई (h) = r cot α
(ii) तिर्यक ऊँचाई (l) = r cosec α

गोला (Sphere) :
जब किसी वृत्त या अर्द्धवृत्त को उसके व्यास के सापेक्ष परिक्रमण कराया जाता है, तो एक ठोस आकृति प्राप्त होती है जिसे गोला कहते हैं। वृत्त का केन्द्र, त्रिज्या और व्यास गोले के केन्द्र, त्रिज्या और व्यास होंगे।

यदि गोले की त्रिज्या r हो, तो
1. गोले का पृष्ठीय क्षेत्रफल = 4πr²
2. गोले का आयतन = \(\frac{4}{3}\)πr³

यदि अर्द्ध गोले की त्रिज्या r हो, तो
1. अर्द्धगोले का वक्र पृष्ठीय क्षेत्रफल = 2πr²
2. अर्द्धगोले का सम्पूर्ण पृष्ठीय क्षेत्रफल = 2πr² + πr² = 3πr²
3. अर्द्ध गोले का आयतन = \(\frac{2}{3}\)πr³

गोलीय कोश (खोखला गोला) (Spherical shell)- दो संकेन्द्रीय गोलों से सीमाबद्ध आकृति को गोलीय कोश कहते हैं।
यदि गोलीय कोश की बाह्य त्रिज्या r₁ और अन्तः त्रिज्या r₂ हो, तो
1. गोलीय कोश का आयतन = \(\frac{4}{3}\)π(r₁³ – r₂³)
2. गोलीय कोश की मोटाई = r₁ – r₂.

ठोसों के संयोजन का पृष्ठीय क्षेत्रफल :
निम्नांकित चित्रों में बनी आकृतियों पर ध्यान दीजिए:

चित्र (1) में दो घनों को संयुक्त करके एक आयतफलकी बनाया गया है।
चित्र (2) में दी गई आकृति एक बेलन के दोनों सिरों पर समान परिच्छेद क्षेत्रफल के दो शंकु जोड़कर बनाई गई है।
चित्र (3) में दी गई आकृति एक बेलन के दोनों सिरों पर समान परिच्छेद क्षेत्रफल के दो अर्द्धगोलों को संयुक्त कर बनाई गई है।
चित्र (4) में दी गई आकृति समान आधार वाले अर्द्धगोले पर शंकु के संयोजन से बनाई गई है।
नोट : (1) किसी संयुक्त ठोस का सम्पूर्ण पृष्ठीय क्षेत्रफल (T.S.A.) उसके खण्डों के सम्पूर्ण पृष्ठीय क्षेत्रफलों के योग से कम होता है और क्षेत्रफल में यह कमी उस क्षेत्रफल के बराबर होती है जो खण्डित भागों को संयुक्त करने पर विलुप्त हो जाता है।
(2) यदि संयुक्त ठोस के सभी खण्ड वक्र संयुक्त पृष्ठीय हैं, तो
संयुक्त ठोस का सम्पूर्ण पृष्ठीय क्षेत्रफल = संयुक्त ठोस के खण्डों के वक्र पृष्ठों के क्षेत्रफल का योग
तथा संयुक्त ठोस का आयतन = संयुक्त ठोस के खण्डों के आयतनों का योग।

ठोसों के संयोजन का आयतन :
दो ठोसों के संयोजन से बने ठोस आकृति का आयतन वास्तव में दोनों के आयतनों के योग के बराबर होता है।
आयतन से सम्बन्धित सूत्र :
1. घनाभ का आयतन = ल. × चौ. × ॐ.
2. घन का आयतन = भुजा³
3. बेलन का आयतन = πr²h
4. शंकु का आयतन = \(\frac{1}{3}\)πr²h
5. खोखले बेलन का आयतन = π(r₁² – r₂²)h, जहाँ r₁ > r₂
6. गोले का आयतन = \(\frac{4}{3}\)πr³
7. अर्द्धगोले का आयतन = \(\frac{2}{3}\)πr³
8. खोखले गोले (गोलीय कोश) का आयतन = \(\frac{4}{3}\)π(r₁³ – r₂³), जहाँ r₁ > r₂

आयतन सम्बन्धी इकाइयाँ
1 लीटर = 1000 घन सेमी
1000 लीटर = 1 घन मीटर
1 किलो लीटर = 1 घन मीटर
1 घन सेमी = 10 × 10 × 10 = 1000 घन मिमी
1 घन मीटर = 100 × 100 × 100
= 1000000 घन सेमी

एक ठोस का एक आकार से दूसरे आकार में रूपान्तरण :
इस खण्ड में हम एक ठोस को एक रूप से दूसरे रूप में परिवर्तित करते हैं, तो इस पूरी स्थिति में दोनों आकारों का आयतन समान रहता है।
जैसे-जूस की एक बोतल को कुछ गिलासों में समान मात्रा में बाँट दें। अब हम गिलासों की संख्या ज्ञात करना चाहेंगे।
[जूस की बोतल का आयतन] = n[गिलासों का आयतन],
जहाँ गिलासों की संख्या है।

लम्बवृत्तीय शंकु का छिन्नक (Frustum of a Right Circular Cone) :
छिन्नक : यदि किसी लम्बवृत्तीय शंकु को उसके आधार के समान्तर तल द्वारा काटा जाता है, तो वह भाग जो शंकु के आधार तथा काटने वाले तल के बीच है, छिन्नक कहलाता है।

चित्र (i) में लम्बवृत्तीय शंकु VAB को उसके वृत्तीय आधार के समान्तर एक तल द्वारा काटा जाता है जिसका केन्द्र O तथा व्यास AB है। शीर्ष / वाले भाग को हटा दिया जाता है, जैसा कि चित्र (ii) में दिखाया गया है। शेष हुआ भाग ABB ‘A’ चित्र (iii) में दिखाया गया है, यह शंकु VAB का छिन्नक है वृत्तीय पृष्ठ AOB तथा A’O’B’ छिन्नक के वृत्तीय सिरे कहलाते हैं।
अतः लम्बवृत्तीय शंकु के छिन्नक के दो असमान वृत्तीय आधार होते हैं और एक वक्र पृष्ठ होता है।
छिन्नक की ऊँचाई: छिन्नक की ऊँचाई या मोटाई दोनों वृत्तीय आधारों के बीच की लम्बीय दूरी होती है।
छिन्नक की ऊँचाई OO’ = VO – VO’ (चित्र iii)
छिन्नक की तिर्यक ऊंचाई : छिन्नक के दोनों वृत्तीय आधारों पर एक ही दिशा में खींची समान्तर त्रिज्याओं के बाहरी बिन्दुओं को मिलाने वाले रेखाखण्ड की लम्बाई को छिन्नक की तिर्यक ऊँचाई कहते हैं।
चित्र में, छिन्नक ABA’B’ की तिर्यक ऊँचाई AA’ या BB’ हैं।
स्पष्ट है कि AA’ = VA – VA’
तथा BB’ = VB – VB’
अतः छिन्नक की तिर्यक ऊँचाई शंकुओं VAB और VA’B’ की तिर्यक ऊँचाइयों के अन्तर के बराबर होती है।

लम्बवृत्तीय शंकु के छिन्नक का आयतन और पृष्ठीय क्षेत्रफल :
शंकु के छिन्नक का आयतन = \(\frac{1}{3}\)π(r₁² – r₁ r₂ + r₂²)h
शंकु के छिन्नक का वक्र पृष्ठीय क्षेत्रफल = π(r₁ + r₂)l

शंकु के छिन्नक का सम्पूर्ण पृष्ठीय क्षेत्रफल = वक्र पृष्ठ का क्षेत्रफल + वृत्तीय आधारों का पृष्ठीय क्षेत्रफल
= π(r₁ + r₂)l + πr₁² + πr₁²
= π[(r₁ + r₂)l + r₁² + r₂²]
शंकु के छिन्नक की (तिर्यक) ऊँचाई = \(\sqrt{\left(r_1-r_2\right)^2+h^2}\)

Jharkhand Board JAC Class 10 Maths Important Questions Chapter 2 बहुपद Important Questions and Answers.

JAC Board Class 10th Maths Important Questions Chapter 2 बहुपद

लयूत्तरात्मक / निबन्धात्मक प्रश्न :

प्रश्न 1.
बहुपद ax² + bx + c के आलेखों की आकृतियाँ, चित्र में दी गयी हैं। प्रत्येक आकृति में a, b और c के चिह्न ज्ञात कीजिए:

हल :
(i) बहुपद y = ax² + bx + c का परवलय नीचे की ओर खुलता है।
∴ a < 0 होगा तथा परवलय का शीर्ष प्रथम चतुर्थांश में है। –\(\frac{b}{2a}\) > 0 ⇒ – b < 0 ⇒ b > 0
परलवय y-अक्ष को बिन्दु P पर प्रतिच्छेद करता है। y-अक्ष पर x = 0 होता हैं। x = 0 का मान समीकरण y = ax² + bx + c में रखने पर हम y = c प्राप्त करते हैं। अतः P के निर्देशांक (o, c) हैं। ∵ P, y- अक्ष की धनात्मक दिशा में है । अतः
∴ c > 0.
∴ a < 0, b > 0 तथा c > 0

(ii) दिशा है : y = ax² + bx + c परवलय ऊपर की ओर खुलता है। अत: a > 0 हैं। परवलय के शीर्ष चौथे चतुर्थाश में हैं।
∴ – \(\frac{b}{2a}\) > 0 ⇒ b < 0 ⇒ b < 0
परवलय y-अक्ष को बिन्दु p पर प्रतिच्छेद करता है। x = 0, अत: y = ax 2 + bx + c में x = 0 रखने पर y = c प्राप्त होता हैं।
∴ p के निर्देशांक (0, c) हैं।
∵ बिन्दु P, OY’ पर स्थित है। अतः c < 0 होगा। a > 0, b < 0 ओर c <0

(iii) बहुपद y = ax² + bx + c का परवलय ऊपर की ओर खुलता है। अतः a > 0 है।
परवलय का शीर्ष OX पर स्थित है।
∴ – \(\frac{b}{2a}\) > 0 ⇒ – b > 0 ⇒ b < 0 परवलय y = ax² + bx + c Y-अक्ष को बिन्दु P पर प्रतिच्छेद करता है जो OY पर स्थित है। समीकरण y = ax² + bx + c में x = 0 रखने पर, y = c प्राप्त होता है। अत: बिन्दु P के निर्देशांक (0, c) हैं। ∵ P, OY अक्ष पर स्थित है। ∴ c > 0 होगा।
∴ a > 0, b < 0 और c > 0.

(iv) परवलय y = ax² + bx + c नीचे की ओर खुलता है। अत: a < 0 है।
परवलय का शीर्ष (\(\frac{-b}{2a}\), \(\frac{-D}{4a}\))OX’ पर स्थित है।
∴ – \(\frac{b}{2a}\) < 0 ⇒ b > 0
परवलय y = ax² + bx + c, Y-अक्ष को P(0, c) पर प्रतिच्छेद करता है जो OY’ पर है। अतः c < 0 होगा।
∴ a < 0, b > 0 तथा c < 0.

प्रश्न 2.
यदि बहुपद p(x) = 2x² + 5x + k के शून्यक α तथा β, सम्बन्ध α² + β² + αβ = \(\frac{21}{4}\) को सन्तुष्ट करते हैं, तो k का मान ज्ञात कीजिए।
हल :
∵ αβ बहुपद p(x) = 2x² + 5x + k के शून्यक हैं।
∴ α + β = \(\frac{-5}{2}\)
तथा α × β = \(\frac{k}{2}\) ………(1)
α² + β² + αβ = \(\frac{21}{4}\) (दिया है)
⇒ α² + β² + 2αβ – αβ = \(\frac{21}{4}\) (αβ जोड़ने तथा घटाने पर)
⇒ (α + β)² – αβ = \(\frac{21}{4}\)
⇒ (\(\frac{-5}{2}\))² – \(\frac{k}{2}\) = \(\frac{21}{4}\)
[समी. (1) का प्रयोग करने पर]
⇒ \(\frac{25}{4}-\frac{k}{2}\) = \(\frac{21}{4}\)
\(\frac{25}{4}-\frac{21}{4}\) = \(\frac{k}{2}\)
\(\frac{4}{4}\) = \(\frac{k}{2}\)
\(\frac{k}{2}\) = 1
⇒ k = 2
अतः k = 2

प्रश्न 3.
यदि α और β द्विघात बहुपद f(x) = x² – px + g के शून्यक हैं तो निम्न के मान ज्ञात कीजिए :
(i) α² + β²
(ii) \(\frac{1}{α}=\frac{1}{β}\)
हल :
∵ α और β बहुपद f(x) = x² – px + q के शून्यक हैं।
∴ शून्यकों का योग (α + β) = – (\(\frac{-p}{1}\)) = p
शून्यकों का गुणनफल (αβ) = \(\frac{q}{1}\) = q

(i) α² + β² = (α + β)² – 2αβ
= p² – 2q

(ii) \(\frac{1}{α}=\frac{1}{β}\) = \(\frac{\alpha+\beta}{\alpha \beta}\) = \(\frac{p}{q}\)

प्रश्न 4.
यदि α, β द्विघात बहुपद f(x)= 2x² – 5x + 7 के शून्यक हों तो एक बहुपद ज्ञात कीजिए जिसके शून्यक 2α + 3β और 3α + 2β हों।
हल
∵ α और β द्विघात बहुपद f(x) = 2x² – 5x + 7 के शून्यक हैं।
∴ α + β = -(\(\frac{-5}{2}\)) = \(\frac{5}{2}\) और αβ = \(\frac{7}{2}\)
माना अभीष्ट बहुपद के शून्यकों के योग व गुणनफल को S व P से व्यक्त करें तो :
S = (2α + 3β) + (3α + 2β)
= 5(α + β) = 5 × \(\frac{5}{2}=\frac{25}{2}\)
या P = (2α + 3β) × (3α + 2β)
या P = 6(α² + β²) + 13αβ
या P = 6α² + 6β² + 12αβ + αβ
या P = 6 (α + β)² + αβ
या P = 6(\(\frac{5}{2}\))² + \(\frac{7}{2}\)
या P = 6 × \(\frac{25}{4}+\frac{7}{2}=\frac{75}{2}+\frac{7}{2}\)
∴ P = \(\frac{75+7}{2}=\frac{82}{2}\) = 41
अत: अभीष्ट बहुपद g(x) निम्न होगा :
g(x) = k(x² – Sx + P)
⇒ g (x) = k(x² – \(\frac{25}{2}\)x + 41)
जहाँ k एक अशून्य वास्तविक संख्या है।

प्रश्न 5.
बहुपद f(x) = 3x³ + ax² + 4x + b का एक गुणनखण्ड (x + 2) है। यदि इसमें (x – 3) का भाग दिया जाये तो शेषफल – 5 बचता है। a तथा b के मान ज्ञात कीजिए।
हल :
यहाँ f(x) = 3x³ + ax² + 4x + b का एक गुणनखण्ड (x + 2) है।
अत: x + 2 = 0
⇒ x = – 2 रखने पर
f(-2) = 0 होगा।
यहाँ f(x) = 3x³ + ax² + 4x + b
∴ f(-2) = 3(-2)³ + a(-2)² + 4(-2) + b
⇒ 0 = 3(-8) + a(4) – 8 + b
⇒ 0 = – 24 + 4a – 8 + b
⇒ 0 = 4a + b – 32
⇒ 4a + b = 32 ……..(i)
पुन: (x – 3) से भाग देने पर शेषफल – 5 बचता है।
अत: f(3) = – 5.
∵ f(x) = 3x³ + ax² + 4x + b
∴ f(3) = 3(3)³ + a(3)² + 4(3) + b
⇒ – 5 = 3 × 27 + a(9) + 12 + b
⇒ – 5 = 81 + 9a + 12 + b
⇒ – 5 = 93 + 9a + b
⇒ 9a + b = – 5 – 93
⇒ 9a + b = – 98 ….(ii)
समीकरण (ii) में से समीकरण (i) को घटाने पर,

∴ a = \(\frac{-130}{5}\) = – 26
a का मान समीकरण (i) में रखने पर,
⇒ 4(-26) + b = 32
⇒ – 104 + b = 32
∴ b = 32 + 104 = 136
अतः a = – 26 और b = 136 होगा।

प्रश्न 6.
3x³ + x² + 2x + 5 को 1 + 2x + x² से भाग दीजिए।
हल :
3x³ + x² + 2x + 5 ÷ 1 + 2x + x²
1 + 2x + x² को x² + 2x + 1 लिख सकते है

जाँच-
(3x – 5) (1 + 2x + x²) + 9x + 10
= 3x + 6x² + 3x² – 5 – 10x – 5x² + 9x + 10
= 3x³ + x² – 7x – 5 + 9x + 10
= 3x³ + x² + 2x + 5

प्रश्न 7.
x³ – 3x² + 3x – 5 को x – 1 – x² से भाग दीजिए और विभाजन एल्गोरिथ्म की सत्यता की जाँच कीजिए।
हल :
माना कि
f(x) = x³ – 3x² + 3x – 5
तथा g(x) = x – 1 – x² = – x² + x + 1
अब f(x) को g(x) से विभाजित करेंगे।

अत: भागफल q(x) = – x + 2
तथा शेषफल (x) = – 3
विभाजन एल्गोरिथ्म के प्रयोग से भाग्य = भाजक × भागफल + शेषफल
⇒ x³ – 3x² + 3x – 5 = (- x² + x – 1) (- x + 2) – 3
⇒ x³ – 3x² + 3x – 5 = (x³ – 2x² – x² + 2x + x – 2) – 3
⇒ x³ – 3x² + 3x – 5 = x³ – 3x² + 3x – 2 – 3
⇒ x³ – 3x² + 3x – 5 = x³ – 3x² + 3x – 5
चूँकि बायाँ पक्ष = दायाँ पक्ष
अतः विभाजन एल्गोरिथ्म सत्यापित होता है।

प्रश्न 8.
वह प्रतिबन्ध ज्ञात कीजिए कि बहुपद p(x) = ax² + bx + c के शून्यक एक दूसरे के व्युत्क्रम हैं।
हल :
माना कि α बहुपद p(x) = ax² + bx + c का प्रथम शून्यक है।
प्रश्नानुसार बहुपद p(x) का दूसरा शून्यक (β) = \(\frac{1}{α}\)
α × β = \(\frac{c}{a}\)
⇒ α × \(\frac{1}{α}\) = \(\frac{c}{a}\)
⇒ 1 = \(\frac{c}{a}\)
⇒ c = a
अतः प्रतिबन्ध c = a है ।

प्रश्न 9.
x³ – 6x² + 11x – 6 को x – 2 से भाग दीजिए और विभाजन एल्गोरिथ्म की सत्यता की जाँच कीजिए ।
हल :

अतः भागफल = x² – 4x + 3 तथा शेषफल = 0
विभाजन एल्गोरिथ्य की सत्यता
भागफल भाजक + शेषफल
= (x² – 4x + 3) × (x – 2) + 0
= x³ – 4x² + 3x – 2x² + 8x – 6
= x³ – 6x² + 11x – 6
= भाज्य
अतः भागफल × भाजक + शेपफल = भाज्य
इति सिद्धम्

प्रश्न 10.
यदि त्रिघातीय बहुपद x³ – 3x² – 10x + 24 का एक शून्यक 4 है, तो इसके अन्य दो शून्यक ज्ञात कीजिए।
हल :
माना p(x) = x³ – 3x² – 10x + 24
दिया है, 4, p(x) का एक शून्यक है।
∴ (x – 4), p(x) का एक गुणनखंड होगा।

भागफल = x² + x – 6 तथा शेषफल = 0
∴ x³ – 3x² – 10x + 24 = (x – 4)(x² + x – 6).
अब p(x) के अन्य शून्यक ज्ञात करने के लिए भागफल (x² + x – 6) = 0 रखकर गुणनखंड करने पर,
x² + x – 6 = 0
⇒ x² + 3x – 2x – 6 = 0
⇒ x(x + 3) – 2(x + 3) = 0
⇒ (x + 3) (x – 2) = 0
यदि x + 3 = 0, तो x = – 3
और यदि x – 2 = 0, तो x = 2
अत: – 3 और 2 बहुपद x³ – 3x² – 10x + 24 के अन्य दो गुणनखंड है।

प्रश्न 11.
एक अध्यापक ने अपने 10 विद्यार्थियों में से प्रत्येक को एक कागज पर एक चर वाला एक बहुपद लिखकर देने को कहा। विद्यार्थियों के उत्तर निम्न थे :
2x + 3, 3x² + 7x + 2, 4x² + 3x² + 2,
x² + \(\sqrt{3}\)x + 7, 5x³ – 7x + 2,
2x² + 3 – \(\frac{5}{x}\), 5x – \(\frac{1}{2}\),
ax³ + bx³ + cx + d, x + \(\frac{1}{x}\), x³ – 3
निम्न प्रश्नों के उत्तर दीजिए:
(i) उपरोक्त दस में कितने बहुपद नहीं हैं?
(ii) उपरोक्त दस में कितने द्विघात बहुपद हैं?
हल :
(i) तीन, x² + \(\sqrt{3x}\) + 7, 2x² + 3 – \(\frac{5}{x}\), x + \(\frac{1}{x}\) बहुपद नहीं है।
(ii) एक 3x² + 7x + 2 एक द्विघातीय बहुपद है।

प्रश्न 12.
यदि बहुपद f(x) = x² – 8x + k के शून्यकों के वर्गों का योग 40 है, तो का मान ज्ञात कीजिए।
हल :
दिया है, f(x) = x² – 8x + k
माना f(x) के शून्यक α और β हैं।
∴ शून्यकों का योग = – \(\frac{b}{a}\)
α + β = \(\frac{-(-8)}{1}\)
और शून्यकों का गुणन = \(\frac{c}{a}\)
αβ = \(\frac{k}{1}\)
प्रश्नानुसार, α² + β² = 40
हम जानते हैं कि
(α + β)² = α² + β² + 2αβ
⇒ (8)² = 40 + 2k
⇒ 64 – 40 = 2k
⇒ 24 = 2k
⇒ k = \(\frac{24}{2}\) = 12

प्रश्न 13.
बहुपद p(x) = 2x⁴ – x³ – 11x² + 5x + 5 के दो शून्यक \(\sqrt{5}\) और – \(\sqrt{5}\) है। इस बहुपद के अन्य दो शून्यक ज्ञात कीजिए।
हल :
\(\sqrt{5}\) और – \(\sqrt{5}\) दिए गए बहुपद के शून्यक है।
∴ (x – \(\sqrt{5}\))(x + \(\sqrt{5}\)) = x² – 5, दिए गए बहुपद का एक गुणनखंड होगा।

विभाजन एल्गोरिथ्य प्रमेय से
2x⁴ – x³ – 11x² + 5x + 5 = (2x² – x – 1)(x² – 5)
अब p(x) के अन्य दो शून्यक ज्ञात करने के लिए भागफल (2x² – x – 1) = 0 रखकर गुणनखंड करने पर
2x² – x – 1 = 0
⇒ 2x² – 2x + x – 1 = 0
⇒ 2x(x – 1) + 1 (x – 1) = 0
⇒ (x – 1) (2x + 1) = 0
यदि x – 1 = 0, तो x = 1
और यदि 2x + 1 = 0, तो x = – \(\frac{1}{2}\)
अतः 1 और – \(\frac{1}{2}\) व्यंजक p(x) के अन्य दो गुणनखंड है।

प्रश्न 14.
बहुपद 2x³ – 3x² + 6x + 7 में कम-से-कम क्या जोड़ा जाए कि प्राप्त बहुपद x² – 4x + 8 से पूर्णतया विभाजित हो जाए?
हल :
माना p(x) = 2x³ – 3x² + 6x + 7
और q(x) = x² – 4x + 8
अब p(x) को q(x) से भाग देने पर

यहाँ शेषफल 10x – 33 प्राप्त होता है। यदि 2x³ – 3x² + 6x +7 में 10x – 33 घटा दिया जाए तो जो बहुपद प्राप्त होगा वह q(x) से पूर्णतया विभाजित हो जाएगा।

प्रश्न 15.
यदि α तथा β बहुपद f(x) = 5x² – 7x + 1 के शून्यक है, तो (\(\frac{α}{β}=\frac{β}{α}\)) का मान ज्ञात कीजिए।
हल :
दिया, f (x)= 5x² – 7x + 1

प्रश्न 16.
k के किस मान के लिए, बहुपद
f(x) = 3x⁴ – 9x³ + x² + 15x + k,
माना, g(x) = 3x² – 5
g(x) से f(x) को भाग करने पर,

∵ f(x), g(x) से पूर्णतया विभाजित होता है, इसलिए शेषफल को शून्य के बराबर रखने पर,
k + 10 = 0
k = – 10

प्रश्न 17.
3x³ + 10x² – 9x – 4 के सभी शून्यक ज्ञात कीजिए यदि इसका एक शून्य 1 है।
हल :
दिया गया बहुपद है –
3x³ + 10x² – 9x – 4
माना, इस बहुपद के शून्यक α, β तथा γ है तथा α = 1 है।
दिए गए समीकरण को, ax³ + bx² + cx + d = 0 से तुलना करने पर प्राप्त होता है,
a = 3 b = 10, c = – 9 तथा d = – 4

⇒ 3β² + 4 = – 13β
⇒ 3β² + 13β + 4 = 0
⇒ 3β² + 12β + β + 4 = 0
⇒ 3β(β + 4) + 1(β + 4) = 0
⇒ (β + 4) (3β + 1) = 0
β = – 4 या β = \(\frac{-1}{3}\)
तब समीकरण (ii) से,
βγ = \(\frac{4}{3}\)
– 4 × γ = \(\frac{4}{3}\) या – \(\frac{1}{3}\) × γ = \(\frac{4}{3}\)
γ = –\(\frac{1}{3}\)
γ = – 4
अतः इसके शून्यक 1, – 4, – \(\frac{1}{3}\) या 1, – \(\frac{1}{3}\), – 4 होंगे।

प्रश्न 18.
p का यह मान ज्ञात कीजिए जिसके लिए बहुपद px² – 14x + 8 = 0 का एक शून्यक दूसरे का 6 गुना है।
हल :
दिया गया समीकरण है
px² – 14x + 8 = 0
माना, एक मूल = α
दूसरा मूल = 6α
मूलों का योग = – \(\frac{b}{a}\)
α + 6α = \(\frac{-(-14)}{p}\)
7α = \(\frac{14}{p}\)
α = \(\frac{14}{p \times 7}\)
α = \(\frac{2}{p}\) ……(i)
मूलों का गुणनफल = \(\frac{c}{a}\)
(α) (6α) = \(\frac{8}{p}\)
6α² = \(\frac{8}{p}\) ……(ii)
α का मान समीकरण (ii) में रखने पर,
6(\(\frac{2}{p}\))² = \(\frac{8}{p}\)
⇒ 6 × \(\frac{4}{p^2}\) = \(\frac{8}{p}\)
⇒ 24p = 8p²
⇒ 8p² – 24p = 0
⇒ 8p (p – 3) = 0
⇒ 8p = 0 ⇒ p = 0
या p – 3 = 0 ⇒ p = 3
p = 0 दिए द्विघात समीकरण px² – 14x + 8 = 0 की सन्तुष्ट नहीं करती है।
अत: p = 3

रिक्त स्थानों की पूर्ति कीजिए :

प्रश्न (क)

1. बहुपद में चर राशि की उच्चतम घात वाले पद के घातांक को बहुपद की …………… कहते हैं।
2. …………… बहुपद की कोई घात नहीं होती है।
3. यदि ग्राफ x-अक्ष के दो भिन्न बिन्दुओं पर काटता है, तो बहुपद के ……………. शून्यक होते हैं।
4. 1 घात के बहुपद के अधिकतम …………………. वास्तविक शून्यक हो सकते हैं।
5. ऐसे बहुपद जिसमें चर राशि की उच्चतम घात 1 हो, …………….. बहुपद कहलाते हैं।

हल:

1. घात,
2. शून्य,
3. दो,
4. 1,
5. रैखिक ।

निम्न में सत्य / असत्य बताइए :

प्रश्न (ख)

1. ऐसे बीजीय व्यजंक जिनमें चर राशियों की घात धनात्मक, ऋणात्मक अथवा शून्य हो, बहुपद कहलाते हैं।
2. बहुपद 5y³ + 2y² + 4y⁵ + y + 7 की घात 3 है।
3. बीजीय व्यंजक \(\frac{3}{4}\)x³ – \(\frac{5}{4}\)x² + 2x – 5 चर x का परिमेय संख्याओं पर बहुपद है।
4. ऐसे बहुपद को, जिसमें चर राशि की उच्चतम घात 2 हो विघातीय बहुपद कहलाता है।
5. यदि कोई व्यजंक f(x), x में बहुपद है, तब एक वास्तविक संख्या, बहुपद (x) का शून्यक कहलाती है, यदि और केवल यदि (k) = 0 हो ।

हल :

1. असत्य,
2. असत्य,
3. सत्य,
4. असत्य,
5. सत्य ।

(ग) बहुविकल्पीय प्रश्न :

प्रश्न 1.
यदि द्विघात बहुपद x² + 3x + k का एक शून्यक 2 है, तो k का मान है:
(A) 10
(B) – 10
(C) – 7
(D) – 2
हल :
माना p(x) = x² + 3x + k
∵ 2, p(x) का एक शून्यक है।
∴ p(2) = 0
⇒ (2)² + 3(2) + k = 0
⇒ 4 + 6 + k = 0
⇒ k = – 10
अत: सही विकल्प (B) है।

प्रश्न 2.
वह द्विघात बहुपद जिसके तथा गुणनफल 6 है, है :
(A) x² + 5x + 6
(B) x² – 5x + 6
(C) x² – 5x – 6
(D) – x² + 5x + 6
हल :
बहुपद = x² – (शून्यकों का योग)x + शून्यकों का गुणनफल
= x² – (- 5)x + 6
= x² + 5x + 6
अत: सही विकल्प (A) है।

प्रश्न 3.
निम्न आकृति में, बहुपद p(x) का आलेख दिया है। बहुपद के शून्यकों की संख्या है।

(A) 1
(B) 2
(C) 3
(D) 0
हल :
∵ बहुपद का आलेख x अक्ष को दो बिन्दुओं पर काटता है।
∴ बहुपद के दो शून्यक हैं।
अतः सही विकल्प (B) है।

प्रश्न 4.
बहुपद p(x) को x² – 4 से विभाजित करने पर भागफल तथा शेषफल क्रमशः x तथा 3 पाए गए। बहुपद p(x) है:
(A) 3x² + x – 12
(B) x³ – 4x + 3
(C) x² + 3x – 4
(D) x³ – 4x – 3
हल :
∵ भाज्य = भागफल × भाजक + शेषफल
p(x) = x × (x² – 4) + 3
= x³ – 4x + 3
अत: सही विकल्प (D) है।

प्रश्न 5.
ऐसे बहुपद जिनके शून्यक केवल 3 तथा 4 हैं, की घात है :
(A) 2
(B) 1
(C) 3 से अधिक
(D) 3
हल :
ऐसा बहुपद जिसके दो शून्यक होते हैं, द्विघातीय बहुपद होता है।
अत: सही विकल्प (A) है।

प्रश्न 6.
यदि α और β बहुपद x² + 2x + 1 के शून्यक हैं, तो \(\frac{1}{\alpha}+\frac{1}{\beta}\) बराबर है।
(A) – 2
(B) 2
(C) 0
(D) 1
हल :
दिया है, बहुपद = x² + 2x + 1
∴ α + β = \(\frac{-b}{a}=\frac{-2}{1}\) = – 2
और αβ = \(\frac{c}{a}=\frac{c}{a}=\frac{1}{1}\) = 1
अब \(\frac{1}{\alpha}+\frac{1}{\beta}\) = \(\frac{\beta+\alpha}{\alpha \beta}\) = \(\frac{-2}{1}\) = – 2
अत: सही विकल्प (A) है।

प्रश्न 7.
यदि बहुपद (3x² + 8x + k) का एक शून्यक दूसरे का व्युत्क्रम है, तो k का मान है :
(A) 3
(B) – 3
(C) \(\frac{1}{3}\)
(D) \(\frac{-1}{3}\)
हल :
दिया है,
बहुपद = 3x² + 8x + k
माना एक शून्यक α है, तब दूसरा शून्यक α होगा।
शून्यकों का गुणनफल = \(\frac{c}{a}\)
⇒ α × \(\frac{1}{α}\) = \(\frac{k}{3}\)
⇒ 1 = \(\frac{k}{3}\)
⇒ k = 3
अत: सही विकल्प (A) है।

प्रश्न 8.
एक तीन घात वाले बहुपद के शून्यकों की अधिकतम संख्या है :
(A) 1
(B) 4
(C) 2
(D) 3
हल :
किसी बहुपद के शून्यकों की अधिकतम संख्या उसके घात के बराबर होती है।
अत: सही विकल्प (D) है।

प्रश्न 9.
यदि बहुपद kx² + 2x + 3 के शून्यकों का योग उनके गुणनफल के बराबर है, तो k बराबर है:
(A) \(\frac{1}{3}\)
(B) \(\frac{-1}{3}\)
(C) \(\frac{2}{3}\)
(D) \(\frac{-2}{3}\)
हल :
शून्यकों का योग = \(\frac{-b}{a}=\frac{-2}{k}\)
शून्यकों का गुणनफल = \(\frac{c}{a}=\frac{3 k}{k}\) = 3
प्रश्नानुसार,
शून्यकों का योग = शून्यकों का गुणनफल
⇒ \(\frac{-2}{k}\) = 3
⇒ k = \(\frac{-2}{3}\)
अत: सही विकल्प (D) है।

प्रश्न 10.
द्विघात समीकरण x² + 99x + 127 के शून्यक होंगे:
(A) दोनों धनात्मक
(B) दोनों ऋणात्मक
(C) एक धनात्मक और एक ऋणात्मक
(D) दोनों समान।
हल :
∵ a > 0, b > 0 तथा c > 0 तब दोनों शून्यक ऋणात्मक होते हैं। अतः विकल्प (B) सही है।

प्रश्न 11.
चित्र में बहुपद f(x) = ax² + bx + c का आलेख दर्शाया गया हो तो :

(A) a > 0, b > 0 तथा c > 0
(B) a > 0 b < 0 तथा c < 0
(C) a > 0, b < 0 तथा c > 0
(D) a > 0, b > 0 तथा c < 0.
हल :
विकल्प (B) सही है।

प्रश्न 12.
चित्र में बहुपद f (x) = ax² + bx + c का आलेख दर्शाया गया हो तो :

(A) a < 0, b < 0 तथा c > 0
(B) a < 0, b < 0 तथा c < 0
(C) a < 0, b > 0 तथा c > 0
(D) a < 0, b > 0 तथा c < 0.
हल :
विकल्प (A) सही है।

प्रश्न 13.
त्रिघात बहुपद ax³ + bx² + cx+ d के दो शून्यक 0 दिए हैं। तीसरा शून्यक है:
(A) \(\frac{-b}{a}\)
(B) \(\frac{b}{a}\)
(C) \(\frac{c}{a}\)
(D) \(\frac{-d}{a}\)
हल :
बहुपद ax³ + bx² + cx + d के दो शून्यक 0 हैं। माना कि तीसरा शून्यक α है।
अतः शून्यकों का योग = α + 0 + 0 = \(\frac{-b}{a}\)
= α = \(\frac{-b}{a}\)
अंत: विकल्प (A) सही है।

प्रश्न 14.
यदि a – b, a तथा a + b बहुपद x³ – 3x² + x + 1 के शून्यक हैं तो (a + b) का मान होगा :
(A) 1 ± \(\sqrt{2}\)
(B) – 1 ± \(\sqrt{2}\)
(C) – 1 – \(\sqrt{2}\)
(D) 3
हल :
शून्यकों का योगफल = – \(\frac{b}{a}\)
⇒ a – b + a + a + b = \(\frac{-(-3)}{1}\)
⇒ 3a = 3
⇒ a = 1
तथा (a – b)a + a(a + b) + (a + b) (a – b) = \(\frac{c}{a}\)
⇒ (1 – b) × 1 + 1(1 + b) + (1 + b) (1 – b) = \(\frac{1}{1}\)
⇒ 1 – b + 1 + b + 1 – b² = 1
⇒ 3 – b² = 1
⇒ b² = 2
⇒ b = ± \(\sqrt{2}\)
अब a + b = 1 ± \(\sqrt{2}\)
अतः सही विकल्प (A) है।

प्रश्न 15.
यदि बहुपद f(x) = x² – 5x + k के शून्यक α तथा β इस प्रकार हों कि α – β = 1 तो k की मान होगा:
(A) 6
(B) 4
(C) 3
(D) शून्य।
हल :
बहुपद x² – 5x + k के शून्यक α, β हों तो
α + β = – (\(\frac{-5}{1}\)) = 5
αβ = \(\frac{k}{1}\) = k
अब α – β = 1
या (α – β)² = 1
या (α + β)² – 4αβ = 1
या 25 – 4k = 1
या 24 = 4k
∴ k = 6
अत: विकल्प (A) सही है।

Jharkhand Board JAC Class 10 Maths Solutions Chapter 1 Real Numbers Ex 1.1 Textbook Exercise Questions and Answers.

JAC Board Class 10 Maths Solutions Chapter 1 Real Numbers Exercise 1.1

Question 1.
Use Euclid’s division algorithm to find the of (1) 135 and 225 (2) 196 and 38220 (3) 867 and 255.
Solution:
1. 135 and 225
Here, 225 > 135
∴ 225 = 135 × 1 +90
Since remainder ≠ 0, we apply division lemma to 135 and 90.
∴ 135 = 90 × 1 + 45
Since remainder ≠ 0, we apply division lemma to 90 and 45.
∴ 90 = 45 × 2 + 0
Since remainder = 0, the divisor 45 is the HCF.
Hence, HCF (135, 225) = 45.

2. 196 and 38220
Here, 38220 > 196
∴ 38220 = 196 × 195 +0
Since remainder = 0, the divisor 196 is the HCF.
Hence, HCF (196, 38220) = 196.

3. 867 and 255
Here, 867 > 255
∴ 867 = 255 × 3 + 102
Since remainder ≠ 0, we apply division lemma to 255 and 102.
∴ 255 = 102 × 2 + 51
Since remainder ≠ 0. we apply division lemma to 102 and 51.
∴ 102 = 51 × 2 + 0
Since remainder = 0, the divisor 51 is the HCF.
Hence, HCF (867, 255) = 51

Question 2.
Show that any positive odd integer is of the form 6q + 1 or 6q + 3 or 6q + 5, where q is some integer.
Solution:
Let a be any positive integer and b = 6. Then. by Euclid’s division lemma, a = 6q + r, for some integer q ≥ 0 and r = 0, 1, 2, 3, 4 or 5. because 0 ≤ r < 6.
So, a = 6q or a = 6q + 1 or
a = 6q + 2 = 2(3q + 1) or a = 6q + 3 or
a = 6q + 4 = 2(3q + 2) or a = 6q + 5.
Since a is an odd integer, a cannot be 6q or 6q + 2 or 6q + 4 as they are all divisible by 2.
Therefore, any positive odd integer is of the form 6q + 1 or 6q + 3 or 6q + 5.

Question 3.
An army contingent of 616 members is to march behind an army band of 32 members in a parade. The two groups are to march in the same number of columns. What is the maximum number of columns in which they can march?
Solution:
To arrive at the answer, we have to find the HCF of 616 and 32.
Here, 616 > 32
∴ 616 = 32 × 19 + 8
∴ 32 = 8 × 4 + 0
Thus, HCF (616, 32)=8
Hence, the maximum number of columns in which they can march is 8 columns.

Question 4.
Use Euclid’s division lemma to show that the square of any positive integer is either of the form 3m or 3m + 1 for some integer m. [Hint: Let a be any positive integer then it is of the form 3q, 3q + 1 or 3q + 2. Now square each of these and show that they can be rewritten in the form 3m or 3m + 1.]
Solution:
Let a be any positive integer and b = 3. Then, by Euclid’s division lemma, a = 3q or a = 3q + 1 or a = 3q + 2; where q is a non- negative integer.
1. If a = 3q, then a² = (39)² = 9q² = 3(3q²) = 3m, where m = 3q² is some integer.
2. If a = 3q + 1, then a² = (3q + 1)² = 9q2 + 6q + 1 = 3(3q² + 2q) + 1 = 3m+ 1. where m = 3q² + 2q is some integer.
3. If a = 3q + 2, then a² = (3q + 2)² = 9q² + 12q + 4 = 9q² + 12q + 3 + 1 = 3 (3q² + 4q + 1) + 1 = 3m + 1, where m = 3q² + 4q + 1 is some integer.
Thus, in either case, the square of any positive integer is of the form 3m or 3m + 1.

Question 5.
Use Euclid’s division lemma to show that the cube of any positive integer is of the form 9m, 9m + 1 or 9m +8.
Solution:
Let a be any positive integer and b = 3. Then, by Euclid’s division lemma, a = 3q or a = 3q + 1 or a = 3q + 2; where q is negative integer.
1. If a = 3q, then
a³ = (39)³ = 27q³ = 9(3q³) = 9m,
where m = 3q³ is some integer.

2. If a = 3q + 1, then
a³ = (3q + 1)³
= 27q³ + 27q² + 9q + 1
= 9(3q³ + 3q² + q) + 1
= 9m + 1.
where m = 3q³ + 3q² + q is some integer.

3. If a = 3q + 2, then
a³ = (3q + 2)³
= 27q + 54q² + 36q + 8
= 9(3q³ + 6q² + 4q) + 8
= 9m + 8,
where m = 3q³ + 6q² + 4q is some integer.
Thus, in either case, the cube of any positive integer is of the form 9m or 9m + 1 or 9m + 8.

Jharkhand Board JAC Class 10 Science Solutions Chapter 13 Magnetic Effects of Electric Current Textbook Exercise Questions and Answers.

JAC Board Class 10 Science Solutions Chapter 13 Magnetic Effects of Electric Current

Jharkhand Board Class 10 Science Magnetic Effects of Electric Current Textbook Questions and Answers

Question 1.
Which of the following correctly describes the magnetic field near a long straight wire?
(a) The field consists of straight lines perpendicular to the wire.
(b) The field consists of straight lines parallel to the wire.
(c) The field consists of radial lines originating from the wire.
(d) The field consists of concentric circles centred on the wire.
Answer:
(d) The field consists of concentric circles centred on the wire.
Hint: This is an experimental fact. Which is only exhibited by the right-hand thumb rule.

Question 2.
The phenomenon of electromagnetic induction is…
(a) the process of charging a body.
(b) the process of generating magnetic field due to a current passing through a coll.
(c) producing induced current in a coil due to relative motion between a magnet and the coil.
(d) the process of rotating a coil of an electric motor.
Answer:
(c) producing induced current in a coil due to relative motion between a magnet and the coil.

Question 3.
The device used for producing electric current is called a…
(a) generator.
(b) galvanometer.
(c) ammeter.
(d) motor
Answer:
(a) generator.
Hint: A generator converts mechanical energy into electrical energy.

Question 4.
The essential difference between an AC generator and a DC generator is that…
(a) an AC generator has an electromagnet while a DC generator has permanent magnet.
(b) a DC generator will generate a higher voltage.
(c) an AC generator will generate a higher voltage.
(d) an AC generator has slip rings while the DC generator has a commutator.
Answer:
(d) an AC generator has slip rings while the DC generator has a commutator.
[Due to the slip rings, the direction of the current produced by an AC generator is reversed at regular interval of time, while the current produced by a DC generator always flows in one direction.]

Question 5.
At the time of short circuit, the current in the circuit…
(a) reduces substantially.
(b) does not change.
(c) increases heavily.
(d) varies continuously.
Answer:
(c) increases heavily.

Question 6.
State whether the following statements are true or false :
(a) An electric motor converts mechanical energy into electrical energy.
(b) An electric generator works on the principle of electromagnetic induction.
(c) The magnetic field lines at the centre of a long circular coil carrying current are parallel straight lines.
(d) The wire with green insulation is usually the live wire of an electric supply.
Answer:
(a) False
[An electric motor converts electrical energy into mechanical energy.]
(b) True
(c) True
(d) False
[The wire with green insulation is the earth wire, whereas the wire with red insulation is the live wire of an electric supply.]

Question 7.
List two methods of producing magnetic fields.
Answer:
Magnetic fields can be produced by passing an electric current through:

• a straight conductor
• a circular loop and
• a solenoid.

Question 8.
How does a solenoid behave like a magnet? Can you determine the north and south poles of a current-carrying solenoid with the help of a bar magnet? Explain.
Answer:

• A current-carrying solenoid behaves like a bar magnet and the polarities of its ends depend upon the direction of the current through it.
• Yes, we can use a bar magnet to determine the north and south poles of a current-carrying solenoid.
• In order to determine the magnetic poles of a current-carrying solenoid, place it in a brass hook and suspend it with a long thread so that it can moves freely in a horizontal plane.

Bring the north pole of a bar magnet near one of its ends. If that end of the solenoid moves towards the bar magnet, then it must be its south pole and the other end its north pole, On the contrary if that end of the solenoid moves away from the magnet, then it must be its north pole and the other end its south pole.

Question 9.
When is the force experienced by a current carrying conductor placed in a magnetic field largest?
Answer:
The force experienced by a current-carrying conductor placed in a magnetic field is the largest, when the current in the conductor is perpendicular to the magnetic field.

For reference see the following figure :

Question 10.
Imagine that you are sitting in a chamber with your back to one wall. An electron beam, moving horizontally from back wall towards the front wall, is deflected by a strong magnetic field to your right side. What is the direction of magnetic field?
Answer:

• For reference, see figure (a) and (b).
• The direction of the conventional current is opposite to the direction of motion of the electron.
• Applying Fleming’s left-hand rule, we find that when the middle finger points in the direction of the current and the thumb points in the direction of the force, the forefinger points downward giving the direction of the magnetic field.

[Note : IMg indicates a vector (arrow) going into the plane of figure, while IMG indicates a vector (arrow) coming out of the plane of the figure.]

Question 11.
Draw a labelled diagram of an electric motor. Explain its principle and working. What is the function of the split ring in an electric motor?
Answer:
Construction: The construction of an electric motor is as shown in figure 13.24.

• Electric motor consists of a rectangular coil ABCD of insulated copper wire.
• The coil is placed between the two poles of a permanent magnet such that the arms AB and CD are perpendicular to the direction of the magnetic field.
• The ends of the coil are connected to two halves P and Q of a split ring.
• The inner sides of these halves are insulated and attached to an axle such that they can rotate easily on it.
• The external conducting edges of P and Q touch two conducting stationary brushes (i.e., carbon strips) X and Y respectively.
• These brushes are connected to a plug key and battery as shown in figure 13.24.

Working:
(1) Current in coil ABCD enters from the source – battery through conducting brush X and flows back to the battery through brush Y.

(2) Current in arm AB of the coil flows from A to B while in arm CD it flows from C to D, i.e., opposite to the direction of the current through AB. Both the currents flowing in AB and CD are perpendicular to the magnetic field.

(3) On applying Fleming’s left hand rule for finding the direction of the force on a current carrying conductor in a magnetic field (see figure 13.24), we find that the force acting or arm AB pushes it downwards while the force acting on arm CD pushes it upwards. These forces are also equal in magnitude and perpendicular to the respective lengths of arms AB and CD.

(4) So, the coil and the axle, mounted free to turn about an axis, rotate anticlockwise.

(5) At half rotation, Q makes contact with brush X and P with brush Y. Therefore, the current in the coil gets reversed and flows along the path DCBA.

(6) A device that reverses the direction of flow of current through a circuit is called a commutator.
In an electric motor the split rings act as a commutator.

(7) Now, the reversal of current also reverses the direction of the force acting on the arms AB and CD. Thus arm AB that was earlier pushed down, is now pushed up and arm CD previously pushed up, is now pushed down.

(8) Therefore, the coil and the axle rotate half a turn more in the same direction.

(9) The reversing of the current is repeated at each half rotation, giving rise to a continuous rotation of the coil and the axle.

Important note : When currents through arms BC and DA are either parallel or antiparallel to the magnetic field, magnetic force does not act on them.

When a rectangular coil carrying a current is placed in a magnetic field, then forces, equal in magnitude and opposite in direction, act on its two parallel sides, perpendicular to their lengths, due to which the coil rotates continuously.

The function of the split ring : Due to the split ring commutator in an electric motor, the direction of the current through the coil is reversed after every half rotation of the coil. This reverses the direction of the force acting on the two arms of the coil. The arm that was earlier pushed downward is now pushed upward, while the other arm is now pushed downward. Therefore, the coil continues to rotate in the same direction.

Question 12.
Name some devices in which electric motors are used.
Answer:
Electric motors are used in electric fans, refrigerators, mixers and grinders, washing machines, water-pumps, coolers, etc.

Question 13.
A coil of insulated copper wire is connected to a galvanometer. What will happen if a bar magnet is (i) pushed into the coil, (ii) withdrawn from inside the coil, (iii) held stationary inside the coil?
Answer:
(i) The galvanometer will show a momentary deflection in one direction. It means a current is induced in the coil in one direction due to the relative motion between the coil and the magnet.

(ii) The galvanometer will show a momentary deflection in the opposite direction. It means a current is induced in the coil in the opposite direction due to the relative motion between the coil and the magnet.

(iii) There will be no deflection in the galvanometer. It means no current is induced in the coil, as there is no relative motion between the coil and the magnet.

[Note: The greater the speed of the magnet, the greater is the deflection of the pointer in the galvanometer.]

Question 14.
Two circular coils A and B are placed close to each other. If the current in coil A is changed, will some current be induced in coil B? Give reason.
Answer:
Yes. Current will be induced in coil B. Reason : When the current in coil A is changed, the magnetic field around it changes.

As the two circular coils (with their planes parallel to each other) are placed close to each other, the magnetic field lines linked with coil B also change. Therefore, some current is induced in coil B.

Question 15.
State the rule to determine the direction of a (i) magnetic field produced around a straight conductor-carrying current, (ii) force experienced by a current-carrying straight conductor placed in a magnetic field which is perpendicular to it, and (iii) current induced in a coil due to its rotation in a magnetic field.
Answer:
(i) Right-hand thumb rule : This rule is also called ‘Maxwell’s corkscrew rule.
Rule : Imagine that you are holding a current-carrying straight conductor in your right hand such that the thumb points towards the direction of current. Then your fingers of right hand wrap around the conductor in the direction of the field lines of the magnetic field.

This is known as the Right-hand thumb rule.

(ii) Fleming’s left-hand rule : In this case, Fleming’s left hand rule is used to find the direction of the force acting on the conductor.
Fleming left-hand rule:

Stretch the thumb, forefinger and middle finger of your left hand such that they are mutually perpendicular (figure 13.22). If the first (fore) finger points in the direction of magnetic field and second (middle) finger points in the direction of current, then the thumb will point in the direction of motion or the force acting on the conductor.

Note : Experimentally it is found that, when direction of current through the rod and direction of the magnetic field are perpendicular to each other, the force exerted on the rod is perpendicular to both of them.

(iii) Fleming’s right-hand rule : Direction of induced current in a conductor can be known with the help of Fleming’s right-hand rule.

Stretch the thumb, forefinger and middle finger of right hand so that they are perpendicular to each other. If forefinger indicates the direction of the magnetic field and thumb shows the direction of motion of conductor, then the middle finger will show the direction of induced current.

Question 16.
Explain the underlying principle and working of an electric generator by drawing a labelled diagram. What is the function of the brushes?
Answer:
Principle : When a coil is rotated properly in a magnetic field, electromotive force is induced in it. As a result, a current flows in the circuit containing the coil.
OR
When a coil is rotated in a magnetic field, electric current is induced in the circuit containing the coil.

Labelled diagram and working : An electric generator is a device that converts mechanical energy into electrical energy. Its working is based on electromagnetic induction.

Construction :

• Figure 13.30 (a) shows the construction of an AC generator. It consists of a rectangular coil ABCD placed between the two poles of a permanent magnet.
• Two ends of this coil are connected to the two metal (copper) rings R₁ and R₂. The inner side of these rings are insulated.
• The two conducting stationary brushes R₁ and R₂ are kept pressed separately on the rings R₁ and R₂ respectively.
• The two rings R₁ and R₂ are internally attached to an axle. The axle may be mechanically rotated from outside to rotate the coil inside the magnetic field.
  
• Outer ends of the two brushes are s connected to the galvanometer to show the flow of current in the given external circuit.

Working:
(1) Suppose, the axle attached to the two rings is rotated such that the arm AB moves up and the arm CD moves down in the magnetic field produced by the permanent magnet. Then the coil ABCD rotates clockwise in the arrangement shown in the figure. By applying Fleming’s right- hand rule, we find that the induced currents are set up in these arms along the directions AB and CD. This means that the current in external circuit flows from brush B₂ to B₁.

[If the coil is made of a larger number of turns, the current generated in each turn adds up to give a large current through the coil.]

(2) After half a rotation, arm CD starts moving up and AB moving down. As a result, the directions of the induced currents in both the arms change, giving rise to the net induced current in the direction DCBA. This means that the current in the external circuit now flows from brush B₁ to B₂.

(3) Thus, after every half rotation the direction of the current in the respective arms changes. Such a current, which changes direction after equal intervals of time, is called an alternating current (abbreviated as AC).

The function of the brushes in an electric generator : Brushes are used to transmit current induced externally from coil ABCD to the external circuit.

Question 17.
When does an electric short circuit occur?
Answer:
An electric short circuit takes place when the live wire and neutral wire of the electric supply line touch each other directly or indirectly via a conducting wire. This occurs when the plastic insulation of the wires gets torn or there is a fault in the electrical appliance.

Question 18.
What is the function of an earth wire? Why is it necessary to earth metallic appliances?
Answer:
Function of an earth wire : The function of an earth wire is to provide a low-resistance conducting path to the current leaking from an electrical appliance to the earth and thereby prevent electric shock to the user of the electrical appliance.

Therefore, it is necessary to earth metallic appliances such as an electric press, toaster, table fan, refrigerator, etc.

Sometimes accidently, the insulation of the wires connected to an electrical appliances may melt resulting in a current through the metallic casing of the appliance. A person touching such an appliance may get a severe electric shock which can be fatal. To avoid this, the current should be diverted to the earth.

Moreover, due to very low resistance (almost nil) offered by the earth wire, the current in the circuit rises to a very high value, thereby melting fuse in that circuit and cutting off its electric supply.

Thus, earthing the electrical appliances properly, saves us from possible electric shock.

Jharkhand Board Class 10 Science Magnetic Effects of Electric Current InText Questions and Answers

Question 1.
Why does a compass needle get deflected when brought near a bar magnet?
Answer:
A compass needle is a small bar magnet with one north pole and the other south pole. When a compass is brought near a bar magnet, compass needle gets deflected due to the forces acting on its pole due to the magnetic field of the bar magnet.

Note: When compass needle is brought near a bar magnet it is acted upon by the force due to the magnetic field of the earth as well as that due to the bar magnet. Therefore, it gets deflected and finally comes to rest in the direction of the net force.

Question 2.
Draw magnetic field lines around a bar magnet.
Answer:

Question 3.
List the properties of magnetic field lines.
Answer:
(1) The magnetic field lines emerge from north pole and merge at the south pole outside the magnet, while inside the magnet the direction of field lines is from its south pole to its north pole.
Thus, the magnetic field lines are closed and continuous curve.

(2) The magnetic field lines are crowded near the pole where the magnetic field is strong and are far apart near the middle of the magnet and far from the magnet where the magnetic field is weak.

(3) The magnetic field lines never intersect each other because if they do so, there would be two directions of magnetic field at that point which is absurd.

(4) In case the field lines are parallel and equidistant, these represent a uniform magnetic field.

Important note : The relative strength of the magnetic field is shown by the degree of closeness of the field lines.

Question 4.
Why don’t two magnetic field lines intersect each other?
Answer:
The direction of the magnetic field B at a point is obtained by drawing a tangent to the magnetic field line at that point.

If two magnetic field lines intersect each other, it would mean that there are two directions of the magnetic field at the point of intersection, which is not possible because magnetic field is a vector, at a given point in space, it can have only one direction, (or the resultant force on a pole (north / south) at any point can only be in one direction.)

Question 5.
Consider a circular loop of wire lying in the plane of the table. Let the current pass through the loop clockwise. Apply the Right-hand thumb rule to And out the direction of the magnetic field inside and outside the loop.
Answer:
Using the Right-hand thumb rule, the direction of the magnetic field inside and outside the circular loop of wire carrying an electric current can be found. This is shown in the following figure:

The dotted magnetic field lines are perpendicular to the plane of the paper.
The front face of the loop behaves as the south pole and the back face, i.e., the face touching the plane of the table behaves as the north pole.

Question 6.
The magnetic field in a given region is uniform. Draw a diagram to represent it.
Answer:
A uniform magnetic field in a given region can be represented by straight, parallel equally spaced field lines.

Question 7.
Choose the correct option :
The magnetic field inside a long straight solenoid carrying current…
(a) is zero.
(b) decreases as we move towards its end.
(c) increases as we move towards its end.
(d) is the same at all points.
Answer:
(d) is the same at all points.
Hint: The magnetic field inside a long straight current-carrying solenoid is uniform which is represented by straight parallel equally spaced lines. Thus, it is the same at all the points.

Question 8.
Which of the following property of a proton can change while it moves freely in a magnetic field? (There may be more than one correct answer.)
(a) mass
(b) speed
(c) velocity
(d) momentum
Answer:
(c) velocity and
(d) momentum
Hint: The proton is a charged particle. Whenever a charged particle moves in a magnetic field except in the direction of the magnetic field and opposite to the direction of the magnetic field, a magnetic force exerts on it. As a result its velocity and momentum (mass x velocity) both change.

Note: The magnetic force is perpendicular to the velocity of the charged particle (here, the proton). Therefore, only the direction of the velocity of the particle changes, i.e., there is no change in magnitude of the velocity (speed) of the particle. The mass does not change.

Question 9.
In Activity 13.7. how do we think the displacement of the rod AB will be affected if (i) the current in rod AB is increased; (ii) a stronger horse-shoe magnet is used; and (iii) the length of the rod AB is increased?
Answer:
Experiments have shown that, when a current-carrying conductor is placed in a magnetic field such that its length is perpendicular to the magnetic field, the force acting on the conductor and hence its displacement (as the conductor is initially at rest) is proportional to…

• the current in the conductor
• the strength of the magnetic field
• the length of the conductor

(i) When the current in the rod AB is increased, then more force will act on the rod and hence the displacement of the rod will also be more (in the same proportion).

(ii) If a stronger horse-shoe magnet is used, then the strength of magnetic field will increase leading to a greater force on the rod. Due to the greater force, the displacement of the rod will also be more (in the same proportion.).

(iii) If the length of the rod AB is increased, then more force will act on the rod and hence the displacement of the rod will also be more (in the same proportion).

Question 10.
A positively charged particle (alpha particle) projected towards west is deflected towards north by a magnetic field. The direction of magnetic field is…
(a) towards south
(b) towards east
(c) downward
(d) upward
Answer:
(d) upward
Hint:

• Here, the positively charged particle (alpha particle) is initially moving towards the west, so the direction of the corresponding conventional current (current opposite to electron-current) is towards the west.
• The deflection of alpha particle is towards north. This shows that the magnetic force acts on it towards the north (direction).
• Hence, here (a) direction of current I is towards west and (b) direction of force F is towards north
  
• Let us now hold the first (fore) finger, middle finger and thumb of your left hand at right angles to one another.
• Adjust the left hand in such a way that your middle finger points towards the west (in the direction of the current) and the thumb points towards the north (in the direction of the force.)

In this case, your first (fore) finger points upwards [shown by the symbol G], Since the direction of the forefinger gives the direction of the magnetic field, the magnetic field would be in the upward direction, [see figure 13.23 (a) or 13.23 (b)].

Question 11.
State Fleming’s left-hand rule.
Answer:
In this case, Fleming’s left hand rule is used to find the direction of the force acting on the conductor.

Fleming left-hand rule:

Stretch the thumb, forefinger and middle finger of your left hand such that they are mutually perpendicular (figure 13.22). If the first (fore) finger points in the direction of magnetic field and second (middle) finger points in the direction of current, then the thumb will point in the direction of motion or the force acting on the conductor.

Note : Experimentally it is found that, when direction of current through the rod and direction of the magnetic field are perpendicular to each other, the force exerted on the rod is perpendicular to both of them.

Question 12.
What is the principle of an electric motor?
Answer:
When a rectangular coil carrying a current is placed in a magnetic field, then forces, equal in magnitude and opposite in direction, act on its two parallel sides, perpendicular to their lengths, due to which the coil rotates continuously.

Question 13.
What is the role of the split rings s in an electric motor?
Answer:
The split rings act as a commutator and its function is to reverse the direction of the current flowing through the coil after every half rotation of the coil.

Due to reversing of the current, the direction of rotating couple (i.e., torque) remains unchanged and the coil continues to rotate in the same direction.

Question 14.
Explain different ways to induce a current in a coil.
Answer:

• A current can be induced in a coil by moving a magnet towards or away from it or by moving the coil towards or away from the magnet.
• A current can be induced in a coil by changing the current in the coil placed near it.
• A current can be induced in a coil by moving a coil properly in a non-uniform magnetic field or by changing a magnetic field around steady coil by some means.
• A current can be induced in a coil by rotating it properly in a magnetic field or by rotating a magnet properly placed near the coil.

[Note : The magnet used may be a bar magnet or a current-carrying conductor.]

Question 15.
State the principle of an electric generator.
Answer:
The working of an electric generator is based upon the principle of electromagnetic induction.
Principle : An electric current produced in a closed circuit or coil by a changing magnetic field is called an induced current. This phenomenon is called electromagnetic induction.

Question 16.
Name some sources of direct current.
Answer:
Electrochemical dry cells, batteries, DC generators, solar cells, etc. are sources of direct current (DC).

Question 17.
Which sources produce alternating current?
Answer:
AC generators (or powerhouse generators), car alternators, bicycle dynamos are sources of alternating current (AC).

Question 18.
Choose the correct option :
A rectangular coil of copper wires is rotated in a magnetic field. The direction of the induced current changes once in each…
(a) two rotations
(b) one rotation
(c) half rotation
(d) one-fourth rotation
Answer:
(c) half rotation
Hint: After each half rotation, the direction of motion of the two parallel sides of the coil (AB and CD) is reversed and so the direction of the induced current changes once in each half rotation.

Question 19.
Name two safety measures commonly used in electric circuits and appliances.
Answer:

1. A safety fuse of proper rating : It prevents damage to the appliances and circuit due to overloading.
2. An earth wire : It prevents possible electric shock when the live wire accidentally touches the metallic body of an appliance.

Question 20.
An electric oven of 2 kW power rating is operated in a domestic electric circuit (220 V) that has a current rating of 5 A. What result do you expect? Explain.
Answer:
The current drawn by this electric oven,
I = \(\frac { P }{ V }\)
= \(\frac { 2000 W }{ 220 V }\)
= 9.09 A
Since the current rating of the circuit is 5 A. the fuse used in this circuit is only of 5 A capacity.

Hence, when the oven is switch ON, the fuse wire (rated 5 A) will get heated too much, melt and break the circuit. This will save the electric oven from getting damaged.

[If fuse of current rating more than 9.09 A is used in the circuit or if no fuse has been put in the circuit, there may be a fire.]

Question 21.
What precaution should be taken to avoid the overloading of domestic electric circuits?
Answer:
To avoid overloading of domestic electric circuits, the following precautions should be taken :

• Wires used for carrying current should be of proper current rating.
• Two separate circuits should be used, one of 5 A current for lighting electric bulbs, tubes, TV, etc. and another of 15 A for higher current rating appliances such as heating appliances, AC, etc.
• Parallel circuits should be used and each circuit should have a fuse of proper rating.
• Too many higher power rating electrical appliances such as electric iron, geyser, air-conditioner, etc. should not be switched on at the same time.
• Too many electrical appliances should not be operated on a single socket simultaneously.
• Wires should be replaced by new wires of proper rating and good insulation after every 5-6 years.
• PVC of good quality should be used.

Activity 13.1 [T. B. Pg. 223]

To show that the magnetic field is produced s due to electric current.

Procedure:
1. Take a straight thick copper wire and place it between points X and Y in an electric circuit, as shown in figure 13.1. Wire XY is kept perpendicular to the plane of the paper.

2. Horizontally place a small compass near this copper wire.
See the position of its needle.

3. Pass the current through the circuit by inserting the key into the plug.

• Observe the change in the position of the compass needle.
• What does it indicate? (or What does it mean?)

Observation:
1. When no current flows in the straight thick copper wire (i.e., when plug key K is open), the compass needle (i.e., magnetic needle) remains stationary in the geographical north- south direction of the earth.

2. On passing the current through the copper wire XY (i.e., conducting wire), by inserting the key into the plug, compass needle is deflected.
[Any change in the direction of current through copper wire will show a variation in deflection.]

3. This indicates (shows) that the electric current through copper wire (the conductor) has produced a magnetic effect. It means that magnetic field is produced around the (copper) wire.

Conclusion :
Magnetic field is produced due to electric current. Thus, we can say that +electricity and ++magnetism are linked to each other.

Note: The ‘magnetic effect of electric current’ means that an electric current flowing in a conducting wire produces a magnetic field around it.

Activity 13.2 [T. B. Pg. 224]

To understand the magnetic field produced by a bar magnet.
OR
To obtain the magnetic field lines around a bar magnet.

Procedure :
1. Fix a sheet of white paper on a drawing board using some adhesive material.

2. Place a bar magnet in the centre of it.

3. Sprinkle some iron filings uniformly around a bar magnet as shown in figure 13.3. A salt- sprinkler may be used for this purpose.

4. Now tap the board gently.

• What do you observe?
• Why do the iron filings arrange in such a pattern?
• What does this pattern demonstrate?

Observation :
1. The iron filings arrange themselves in a pattern as shown in figure 13.3.

2. A bar magnet exerts its influence in the region surrounding it. Therefore, the iron filings experience a magnetic force. This force makes iron filings arrange in a specific (particular) pattern.

3. The region surrounding a magnet, in which the force of the magnet can be detected, is said to have a magnetic field.
The lines along which the iron filings align themselves represent magnetic field lines.

Conclusion:
A bar manget possesses a magnetic field which can be detected by sprinkling iron filings around it.

Activity 13.3 [T. B. Pg. 224]

To draw magnetic field lines of a bar magnet using a compass.

Procedure:
1. Take a small compass and a bar magnet.

2. Place the magnet on a sheet of white paper fixed on a drawing board, using some adhesive material.

3. Mark the boundary of the magnet.

4. Place a compass near the north pole of the magnet. How does it behave?

5. Mark the positions of two ends of the needle.

6. Now move the needle to a new position such that its south pole occupies the position previously occupied by its north pole.
In this way, proceed step by step till you reach the south pole of the magnet as shown in figure 13.4.

7. Join the points marked on the paper by a smooth curve. This curve represents a magnetic field line.

8. Repeat the above procedure and draw as many lines as you can. You will get a pattern shown in figure 13.5.
These lines represent the magnetic field around the magnet.
These are known as magnetic field lines.

• Observe the deflection in the compass needle as you move it along a field line. The deflection increases as the needle is moved towards the poles.

Observation:
1. The south pole of the needle points towards the north pole of the magnet. The north pole s of the compass needle is directed away from the north pole of the magnet.

2. Magnetic field is a quantity that has both direction and magnitude. The direction of the magnetic field is taken to be direction in which a north pole of the compass needle moves inside it.

Therefore, the field lines emerge from the north pole and merge at the south pole of a magnet. Inside the magnet, the direction of the field lines is from its south pole to its north pole. Thus, the magnetic field lines are closed curves.

Conclusion:

1. The region surrounding a magnet has a magnetic field, i.e., a bar magnet possesses magnetic field.
2. The direction of magnetic field is the direction in which the north pole of the compass needle move / deflect.
3. The field is stronger where the field lines are crowded and weaker where the field lines are farther away from each other.
4. The deflection of the needle increases as the needle is moved towards the poles.

Activity 13.4 [T. B. Pg. 226]

To show that the direction of the magnetic field due to a current depends on the direction of the current.

Procedure:
1. Take a long straight copper wire, two or three cells of 1.5 V each, and a plug key. Connect all of them in series as shown in figure 13.6 (a).

2. Place the straight wire parallel to and over a compass needle.

3. Plug the key in the circuit.
Observe the direction of deflection of the north pole of the needle.

4. Replace the cell connections in the circuit as shown in figure 13.6 (b). This would result in the change of the direction of current through the copper wire, that is, from south to north.
Observe the change in the direction of deflection of the needle.

Observation:
1. If the current flows from north to south as shown in figure 13.6 (a), the north pole of the compass needle moves towards the east.

2. Now, when the current flowing through the copper wire is reversed, the compass needle aligns / moves in the opposite direction i.e., towards the west as shown in figure 13.6 (b).

It means that the direction of the magnetic field due to a current is reversed, when the direction of the current is reversed.

Conclusion :
The direction of the magnetic field due to a current depends on the direction of the current.

Activity 13.5 [T. B. Pg. 226]

To study the pattern of the magnetic field (lines) due to a current through a straight conductor.

Procedure:
1. Take a battery (12 V), a variable resistance (or a rheostat), an ammeter (0-5 A), a plug key, connecting wires and a long straight thick copper wire.

2. Insert the thick wire through the centre, normal to the plane of a rectangular cardboard. Take care that the cardboard is fixed and does not slide up or down.

3. Connect the copper wire vertically between the points X and Y, as shown in figure 13.7 (a), in series with the battery and a plug key.

4. Sprinkle some iron filings uniformly on the cardboard. (You may use a salt-sprinkler for this purpose.)

5. Close the key so that a current flows through the wire. Ensure that the copper wire placed between the points X and Y remains vertically straight.

6. Keep the variable of the variable resistance (or rheostat) at a fixed position and note the current through the ammeter.

• Gently tap the cardboard a few times. Observe the pattern of the iron filings.
• What do these concentric circles represent?
• How can the direction of the magnetic field be found?
  (Place a compass at a point (say P) over a circle. Observe the direction of the needle.)
• Does the direction of the magnetic field lines get reversed if the direction of the current through the straight copper wire is reversed? Check it.
  
• What happens to the deflection of the compass needle placed at a given point if the current in the copper wire is changed?
• (To see this, vary the current in the wire using the variable resistance.)
• What happens to the deflection of the needle if the compass is moved away from the copper wire keeping the current through the wire remains tire same?
• (To see this, now place the compass needle at a farther point from the conducting wire [say at point Q (figure 13.7 (b)]).
• What change do you observe?

Observation:

• It is found that the iron filings align themselves forming a pattern of concentric circles around the copper wire (figure 13.7 (c)).
• These concentric circles represent the (pattern of) magnetic field lines.
• The direction shown by the north pole of the compass needle gives the direction of the magnetic field lines at point P. In figure 13.7 (a), it is shown by an arrow.
• Yes. The direction of the magnetic field lines get reversed if the direction of the current through the straight copper wire is reversed (see figure 13.7 (b)).
• We find that the deflection of the needle changes, when the current is changed.

If the current is increased, the deflection increases and if the current is decreased, the deflection decreases.

• When the compass is moved away from the wire, the deflection of the needle decreases.
• When the compass is moved away from the wire, it is observed that the concentric circles representing the magnetic field around a current-carrying straight wire become larger and larger.
• In other words, as we move away from the wire, the radius of a circle representing the magnetic field line increases.

Conclusion :
(1) Pattern of the magnetic field produced due to a straight conductor carrying current is concentric circles, which are nothing but the magnetic field lines of the produced magnetic field.

(2) Direction of magnetic field at a point is in the direction of tangent drawn at that point on particular circle, which is nothing but the direction of north pole of compass needle at that point.

(3) Direction of magnetic field lines get reversed if direction of current through the straight copper wire is reversed, i.e., it depends on the direction of the current.

(4) Magnitude of the magnetic field produced at a given point increases as the current through the wire increases.
i.e., Magnetic field B ∝ Current I.

(5) Magnitude of the magnetic field produced by a given current in the conductor decreases as the distance from wire increases.
i.e., Magnetic field B ∝ \(\frac{1}{\text { Distance from the wire }}\)

Activity 13.6 [T. B. Pg. 229]

To study the magnetic field produced near a circular coil carrying current.

Procedure:

• Take a rectangular cardboard having two holes. Insert a circular coil having a large number of turns through them, normal to the plane of the cardboard.
• Connect the ends of the coil in series with a battery, a plug key and a rheostat, as shown in figure 13.13.
• Sprinkle iron filings uniformly on the cardboard.
• Plug the key.
• Tap the cardboard gently a few times.
• Note the pattern of the iron filings that emerges on the cardboard.
  

Observation :
The iron filings arrange themselves in the pattern as shown in figure 13.13.

Conclusion:

• The magnetic field at the centre of the coil is nearly uniform.
• The magnetic field lines near the coil are circular and concentric.
• At the centre, as the field lines are crowded, the strength of the magnetic field is maximum.

Activity 13.7 [T. B. Pg. 230]

To understand the force experienced by a current-carrying small aluminium (conducting) rod placed in a magnetic field.

Procedure:
1. Take a small aluminium rod AB (of length about 5 cm.) Using two connecting wires suspend it horizontally from a stand, as shown in figure 13.20.

2. Place a strong horse-shoe magnet in such a way that the rod lies between the two poles with the magnetic field directed upwards. For this put the north pole of the magnet vertically below and the south pole vertically above the aluminium rod (see figure 13.20).

3. Connect the aluminium rod in series with a battery, a plug key and a rheostat.

4. Now pass a current through the aluminium rod from end B to end A.

• What do you observe?
• Reverse the direction of current flowing through the rod and observe the direction of its displacement.
  
• Why does the rod get displaced?
• Change the direction of magnetic field by interchanging the two poles of the magnet to vertically downwards and observe the direction of the force / displacement of the current-? carrying rod.

Observation :
1. It is observed that the rod is displaced to the left.

2. The direction of the displacement of the rod is reversed, i.e., now it is to the right, on reversing the direction of the current flowing through the rod.

It suggests that the direction of the force is also reversed when the direction of the current through the conductor (rod) is reversed.

3. The displacement of the rod is due to the force exerted on the current- carrying aluminium rod when it is placed in a magnetic field.

4. The direction of the force acting on the current-carrying rod and hence that of the displacement of the rod (as the rod is initially ; at rest) gets reversed on changing the direction of the magnetic field to vertically downwards.

Conclusion :

• A current-carrying conductor (here a rod) experiences a force when placed in a magnetic field.
• The direction of the force and hence that of the displacement of the conductor (rod) depends upon the direction of the current and the direction of the magnetic field.

Notes :

• Here, the displacement of the rod has the same direction as that of the force acting on the rod because the rod is initially at rest. Recall the kinematical equations.
• The magnetic force also depends upon the length of the rod in the magnetic field.

Activity 13.8 [T. B. Pg. 233]

To study the phenomenon of electromagnetic induction.

Procedure:
1. Take a coil of wire AB having a large number of turns.

2. Connect the ends of the coil to a galvanometer as shown in figure 13.25.

3. Take a strong bar magnet and move its north pole towards the end B of the coil.
Do you find any change in the galvanometer needle?

4. Now withdraw the north pole of the magnet away from the coil.
What do you observe?

5. Place the magnet stationary at a point near the coil, keeping its north pole towards the end B of the coil.

6. Move the coil to right and later to left.
What do you observe?

7. When the coil is kept stationary with respect to the magnet.
What do you observe?

8. Now, move the south pole of the magnet towards the end B of the coil.

• What do you observe?
• When the coil and the magnet both are stationary what do you observe?
• What do you conclude from this activity?
  

Observation :
1. There Is a momentary deflection in the needle of the galvanometer, say to the right.
This indicates the presence of a current in the coil AB. The deflection becomes zero the moment the motion of the magnet stops.

2. When the north pole of the magnet is moved away from the coil, the galvanometer needle is deflected to the left, showing that the current is now set up in the direction opposite to the first.

3. We see that the galvanometer needle deflects to the right when the coil is moved towards the north pole of the magnet. Similarly, the needle moves to the left when the coil is moved away.

4. When the coil is kept stationary with respect to the magnet, the deflection of the galvanometer needle drops to zero.

5. If you move the south pole of the magnet ‘c towards the end B of the coil, the deflections in the galvanometer would just be opposite > to those in the previous case.

6. When the coil and magnet both are stationary, there is no deflection in the galvanometer.

Conclusion :
Whenever there is a relative motion between s a magnet and coil, potential difference is produced between two ends of the coil, which sets up an electric current in the circuit.

This potential difference is called the induced potential difference and the corresponding current is called the induced current.

Activity 13.9 [T. B. Pg. 235]

To study the phenomenon of electromagnetic induction.

Procedure:
1. Take two different coils of copper wire having large number of turns (say 50 and loo turns respectively). Insert them over a non-conducting cylindrical roll, as shown in figure 13.27 (You may use a thick paper roll for this purpose.)

2. Connect the coil 1. having larger number of turns, in series with a battery and a plug key. Also connect the other coil 2 wIth a galvanometer as shown.

3. Plug in the key. Observe the galvanometer.
Is there a deflection in its needle?

4. Disconnect coil 1 from the battery.
What do you observe?

Observation :
1. When the key is plugged, the needle of the galvanometer is instantly deflected to one side and then quickly returns to zero, indicating a momentary current in coil 2.

2. When coil 1 is disconnected from the battery, the needle of the galvanometer momentarily moves, but to the opposite side, i.e., now the current flows in the opposite direction in coil 2.

In short in this activity, we observe that as soon as the current in coil 1 reaches either a steady value or becomes zero, the galvanometer connected to coil 2 shows no deflection.

Conclusion :
(a) A potential difference is induced in coil 2 whenever the electric current through the coil 1 changes with time.

(b) As the current in coil 1 (which is called Primary coil) changes, the magnetic field associated with it also changes. Then the magnetic field lines around coil 2 (which s is called Secondary coil) also change.

Thus, the change in magnetic field lines associated with the secondary coil is the cause of induced electric current in it. s

This process, by which a changing magnetic field in a conductor induces a current in another conductor, is called electromagnetic induction.

Students must go through these JAC Class 10 Science Notes Chapter 12 Electricity to get a clear insight into all the important concepts.

JAC Board Class 10 Science Notes Chapter 12 Electricity

→ Electric charge: An electric charge is an intrinsic property of the proton, electron and many other particles. There are two types of electric charges :

• Positive electric charge
• Negative electric charge.

→ Electric current: The electric current is the net amount of electric charge that passes through any cross-sectional area of the conductor in unit time (I = \(\frac { Q }{ t }\)).
Its SI unit is the coulomb/second (C/s) or the ampere (A).

The direction of the conventional current is taken as opposite to the direction of the flow of electrons.

ampere: If 1 coulomb charge flows through any cross-section of a conductor in 1 second, then the electric current flowing through the conductor is said to be 1 ampere.
1 mA = 10^-3 A, 1 μA = 10^-6A

→ Electric potential and Electric potential difference: The work done in bringing a unit positive charge from infinity to a particular point in the electric field against the electrostatic force due to the electric field is called the electric potential at that point.

(The charge is kept in equilibrium.)

The electric potential difference (p.d.) between any two points A and B in an electric field is the work done to move a unit positive charge ( + 1C) from one point A to the another point B against the electric force due to the electric field.
The SI unit of potential and potential difference is the joule /coulomb or volt (V).

→ volt: The potential difference between two points in an electric field is said to be 1 volt if 1 J of work is done to move a charge of 1 C from one point to another point.
1 V = \(\frac { 1 J }{ 1 C }\)

→ Ohm’s law: The current flowing through a conductor, such as a metallic wire, is directly proportional to the potential difference across its ends, provided its temperature and other physical conditions remains the same.

→ Resistance: Whenever a current flows through a conductor, the free electrons moving in one direction in the conductor collide with the ions, atoms and molecules of the conductor. Due to these collisions, the motion of electrons is opposed. The resulting opposition to the current is called resistance of the conductor.
Resistance R = \(\frac { V }{ I }\)
where, V = voltage applied between the two ends of the conductor
I = electric current flowing through the conductor
Unit: The SI unit of resistance is Ohm. It is denoted by Ω (omega).

Ohm : If the potential difference across the two ends of a conductor is 1V and the current through it is 1 A, then the resistance R of the conductor is 1Ω.
1 Ω = \(\frac { 1 V }{ 1 A }\)

→ Resistivity: The electrical resistivity of a material is the resistance of a conductor (of that material) having unit length and unit area of cross-section. Its SI unit is Ωm.

→ Series combination of resistors: When two (or more) resistances are connected end to end consecutively with one source such that the close path is formed, then they are said to be connected in series.
OR
When two or more than two resistors are connected between two points in a circuit in such a way that the current has only one way to flow and current flowing through every resistor in the circuit remains the same, such a combination of the resistors is called series combination of resistors.

The total resistance of the circuit increases in a series combination of resistors. This results in a reduction in the current.

→ Parallel combination of resistors: When two (or more) resistances and one source are connected between the same two points, they are said to be connected in parallel.
OR
When two or more than two resistors are connected between two points in a circuit in such a way that more than one paths are available for the current to flow and the voltage drop across two ends of each resistor remains the same, then the resistors are said to be connected in parallel between these two points and the combination of resistors is called parallel combination.

The total resistance in the circuit is reduced in a parallel combination of the resistors. This results in an increase in the electric current.

→ Heating effect of electric current: The production of heat in a metallic conductor by the electric current flowing through it is callled the heating effect of electric current.

The heat generated due to flow of electric current depends on the following factors:

• Electric current
• Resistance and
• Time taken to pass electric current.

The electrical energy consumed (or heat energy produced) when an electric current (7) flows in a conductor for time t is
H = I²Rt = \(\frac{V^2}{R}\) t = \(\frac { V }{ R }\) x Vt = IVt (joule)
The unit of electric energy is joule.

→ Electric power: Electric energy consumed (or heat energy produced) per unit time is termed as electric power.
OR
Electric power is the rate of consumption of electric energy with time. The SI unit of electric power is watt.

watt: If a device carries a current of 1 A when operated at a potential difference of 1 V, the power consumed is 1W.
1 watt = 1 volt x 1 ampere.

Students must go through these JAC Class 10 Science Notes Chapter 16 Management of Natural Resources to get a clear insight into all the important concepts.

JAC Board Class 10 Science Notes Chapter 16 Management of Natural Resources

→ Natural resources : The naturally occurring resources which are used by human beings for various purposes are called natural resources. Soil, water, air, forests, wildlife, coal, petroleum, etc. are the examples of natural resources.

→ In 1985, Ganga Action Plan was started for cleaning and maintaining the quality of water in the river Ganga.
The presence of faecal coliform bacteria in water indicates contamination by disease causing microorganisms.

→ There are some measurable factors which are used to quantify pollution or the quality of water.
The pH of water can be easily checked using universal indicator. Water with a pH level between 6.5-8.5 is safe for drinking.

→ Conservation is controlled utilization of natural resources for the benefit of all life forms.

→ The five R’s to save the environment are:

• Refuse : It means not to buy product that can harm the environment.
• Reduce : It means use less and save natural resources.
• Reuse : It means use things again and again instead of throwing away.
• Repurpose : It means a product can be used for other purpose, when it is no more useful for its primary and main purpose.

→ Recycle : It means the processing of things that are considered waste and turning them into useful products.

→ The concept of sustainable development encourages eco-friendly approach that meets current basic human needs. Economic development is linked to environmental conservation.

→ The natural resources need to be managed due to following reasons :

• They should last for future generation
• They should be equally distributed
• The resource are used in such a way that the environment should not be harmed.

→ Forests are important renewable natural resources. A wide range of different life forms are found there. They are biodiversity hot spots.

→ The different stakeholders in a forest are :

• People who live in or around forests
• The Forest Department of the Government
• The industrialists
• The wildlife and nature enthusiasts.

→ The Government of India has instituted an ‘Amrita Devi Bishnoi National Award for Wildlife Conservation’, in the memory of Amrita Devi Bishnoi. who in 1731 sacrificed her life along with 363 others for the protection of ‘Khejri’ trees.

→ Chipko Andolan (‘Hug the Trees Movement’) was started in early 1970 from Reni village, in Garhwal.
Chipko Andolan was the result of a grass root level effort to end the alienation of people from forests.

→ Sal forests in the Arabari forest range of Midnapore district of West Bengal were saved by the involvement of the local villagers.

→ Monoculture of pine, teak and eucalyptus in the forest area are an important source of revenue for the Forest Department, but such practise destroys biodiversity.

→ Water is a basic necessity for all terrestrial forms of life.

• Rain is a very important source of fresh water.
• Large dams can ensure the storage of adequate water mainly for irrigation and for generating electricity.
• Large dams cause social, economic and environmental problems.

→ Water harvesting means storing rainwater where it falls or storing run off water in a local areas. Dr Rajendra Singh won the Stockholm Water Prize in 2015. He is known as India’s ‘Waterman’.

→ Fossil fuels, i.e., coal and petroleum were formed from the degradation of biomass millions of years ago. Energy needs have been largely met by the reserves of coal and petroleum.

We would need to look for alternative sources of energy because coal and petroleum are exhaustible energy resources.

Students should go through these JAC Class 10 Maths Notes Chapter 10 वृत्त will seemingly help to get a clear insight into all the important concepts.

JAC Board Class 10 Maths Notes Chapter 10 वृत्त

भूमिका :
कक्षा IX में हम वृत्त से सम्बन्धित अवधारणाएँ जैसे-जीवा, वृत्तखण्ड, त्रिज्यखण्ड, चाप आदि के बारे में पढ़ चुके हैं। इस अध्याय में हम समतल में स्थित एक वृत्त तथा एक रेखा की विभिन्न स्थितियों पर विचार करेंगे।
→ अभिलम्ब (Normal): किसी वृत्त के किसी बिन्दु पर अभिलम्ब वह सरल रेखा है जो दिए गए बिन्दु से गुजरती है तथा उस बिन्दु पर स्पर्श रेखा के लम्बवत होती है।
→ सम्पूरक कोण (Supplementary angles) : यदि दो कोणों का योग 180° हो, तो वे सम्पूरक कोण कहलाते हैं।
→ अन्तः कोण (Co-interior angles) : ऐसे कोण जो तिर्यक रेखा के एक ही ओर स्थित होते हैं, अन्तः कोण कहलाते हैं।
→ समान्तर चतुर्भुज (Parallelogram) : वह चतुर्भुज जिसकी सम्मुख भुजाएँ समान्तर व बराबर होती हैं, समान्तर चतुर्भुज कहलाता है।
→ समचतुर्भुज (Rhombus ) : वह चतुर्भुज जिसकी सभी भुजाएँ बराबर होती हैं, समचतुर्भुज कहलाता है।
→ परिवृत्त (Circumscribed circle) : बहुभुज के सभी शीर्षों से होकर गुजरने वाला वृत्त परिवृत्त कहलाता है।
→ अन्तः वृत्त (Inscribed circle) : बहुभुज के अन्तर्गत खींचा जा सकने वाला वह बड़े से बड़ा वृत्त जो बहुभुज की प्रत्येक भुजा को एक बिन्दु पर छूता हो, अन्तः वृत्त कहलाता है।

छेदक रेखा और स्पर्श रेखा :
यदि एक समतल में एक वृत्त तथा कोई रेखा AB खींची जाए, तो वृत्त के सापेक्ष यह रेखा तीन स्थितियों में हो सकती है :
स्थिति I. अप्रतिच्छेदी रेखा (Non intersecting line) : रेखा AB वृत्त को स्पर्श नहीं करती है अर्थात् रेखा और वृत्त के मध्य कोई सम्बन्ध नहीं होता है। इस स्थिति में रेखा AB को वृत्त के सापेक्ष अप्रतिच्छेदी रेखा कहते हैं। जैसा कि संलग्न चित्र में दर्शाया गया है।

स्थिति II. छेदक रेखा (Secant) रेखा AB वृत्त को दो भिन्न-भिन्न बिन्दुओं पर प्रतिच्छेद करती है, यह रेखा वृत्त को अधिकतम दो बिन्दुओं पर ही प्रतिच्छेद कर सकती है, क्योंकि वृत्त तीन या तीन से अधिक सरेखीय बिन्दुओं से नहीं गुजर सकता है। अतः एक ऐसी सरल रेखा जो एक वृत्त को दो भिन्न-भिन्न बिन्दुओं पर प्रतिच्छेद करती है, छेदक रेखा (Secant) कहलाती है। जैसा कि संलग्न चित्र में दर्शाया गया है।

स्थिति III. स्पर्श रेखा (Tangent) : रेखा AB वृत्त को दो सम्पाती बिन्दुओं पर प्रतिच्छेद करती है, अर्थात् रेखा वृत्त को एक बिन्दु पर स्पर्श करती है। अतः एक सरल रेखा जो वृत्त को केवल एक बिन्दु पर स्पर्श करे, वृत्त की स्पर्श रेखा (Tangent) कहलाती है। वृत्त के समतल में स्पर्श रेखा और वृत्त में केवल एक बिन्दु उभयनिष्ठ होता है। यह उभयनिष्ठ बिन्दु स्पर्श बिन्दु (Point of contact) कहलाता है। जैसा कि संलग्न चित्र में दिखाया गया है।

छेदक रेखा के समान्तर स्पर्श रेखा (Tangent Parallel to Secant) : एक छेदक रेखा के समान्तर वृत्त की अधिकतम दो स्पर्श रेखाएँ खींची जा सकती हैं।

चित्र में l, m, n, o छेदक रेखाएँ हैं। AB तथा CD वृत्त पर स्पर्श रेखाएँ जो वृत्त को क्रमश: P और Q बिन्दु पर स्पर्श करती हैं।

Jharkhand Board JAC Class 10 Science Important Questions Chapter 15 हमारा पर्यावरण Important Questions and Answers.

JAC Board Class 10 Science Important Questions Chapter 15 हमारा पर्यावरण

अतिलघु उत्तरीय प्रश्न

प्रश्न 1.
जैवमण्डल किसे कहते हैं?
उत्तर:
संसार के समस्त बायोमों को एक साथ मिलाकर एक और भी बड़ी इकाई का निर्माण होता है जो एक विशाल स्वयं पोषी जैव तन्त्र है, इसे ही जैवमण्डल कहते हैं?

प्रश्न 2.
पारिस्थितिक तंत्र से क्या समझते हो?
उत्तर:
जीवमण्डल के जैविक एवं अजैविक घटकों में परस्पर निर्भरता जिसमें ये दोनों ही एक-दूसरे को प्रभावित करते हैं तथा एक-दूसरे पर निर्भर रहते हैं। पारिस्थितिक तंत्र कहलाता है।

प्रश्न 3.
पारिस्थितिक तंत्र के घटकों के नाम लिखिए।
उत्तर:
पारिस्थितिक तंत्र के मुख्यतः दो घटक होते हैं-

• जैव घटक
• अजैव घटक।

प्रश्न 4.
बायोम से क्या अभिप्राय है?
उत्तर:
किसी भी भौगोलिक क्षेत्र में समस्त पारिस्थितिक तंत्र एक साथ मिलकर एक और भी बड़ी इकाई का निर्माण करते हैं जिसको बायोम कहते हैं। उदाहरण के लिए मरुस्थली बायोम में कोई तालाब, झील, मैदान या वन भी दृष्टिगोचर हो सकते हैं। ‘सकते हैं।

प्रश्न 5.
उत्पादक से क्या समझते हो?
उत्तर:
उत्पादक के अन्तर्गत हरे पौधे आते हैं जो साधारण अकार्बनिक पदार्थों से सूर्य के प्रकाश की उपस्थिति प्रकाश संश्लेषण क्रिया द्वारा जटिल पदार्थों का निर्माण करते हैं। ये मूल उत्पादक भी कहलाते हैं।

प्रश्न 6.
नाइट्रोजन चक्र में भाग लेने वाले दो जीवाणुओं के नाम बताइये।
उत्तर:
नाइट्रोजन चक्र में भाग लेने वाले जीवाणु हैं-

• राइजोबियम (नाइट्रोजन स्थरीकरण में)
• नाइट्रोसोमोनास (अमोनीकरण में)।

प्रश्न 7.
जलीय चक्र किसे कहते हैं?
उत्तर:
सूर्य की गर्मी के कारण जलाशयों तथा जैव जीवों के शरीर की सतह से जल का लगातार वाष्पन, पौधों में वाष्पोत्सर्जन द्वारा वायु में जल वाष्प के रूप में उपस्थित रहना तथा इस जल वाष्प संघनन से पुनः जल वर्षा अथवा हिम के रूप में पृथ्वी पर वापस आ जाना, जल चक्र कहलाता है। अर्थात् जल का पर्यावरण में परिवहित होते रहना ही जलचक्र कहलाता है।

प्रश्न 8.
पौधे के किस भाग में सौर ऊर्जा अवशोषित करने की क्षमता होती है?
उत्तर:
पौधों की पत्तियों में सौर ऊर्जा अवशोषित करने की क्षमता होती है।

प्रश्न 9.
जीवमण्डल के अजैविक घटकों के नाम लिखिए।
अथवा
पर्यावरण के अजैविक घटकों को सूचीबद्ध कीजिए।
उत्तर:
जीवमण्डल के अजैविक घटक हैं- वायु, जल, मृदा तथा खनिज प्रकाश, ताप, नमी तथा वायु जैसे घटक जलवायु का निर्धारण करते हैं। स्थलमण्डल, जलमण्डल तथा वायुमण्डल भी इसके घटक हैं।

प्रश्न 10.
घास, मेढक, पक्षी तथा कीट पर आधारित खाद्य श्रृंखला को सही रूप में लिखिए।
उत्तर:
खाद्य शृंखला का सही रूप होगा-
घास → कीट → मेढक → पक्षी।

प्रश्न 11.
N₂ चक्र में अमोनिया को नाइट्रेट में परिवर्तित करने वाले जीवाणु का नाम लिखिए।
उत्तर:
N₂ चक्र में अमोनिया को नाइट्रेट में परिवर्तित करने वाले जीवाणु का नाम है- नाइट्रोसोमोनास।

प्रश्न 12.
नील हरित शैवालों की विशेषताएँ लिखिए।
उत्तर:
नील हरित शैवालों की विशेषताएँ निम्न हैं-

• ये नाइट्रोजन स्थरीकरण मं भाग लेते हैं।
• यह धान के खेतों तथा फलीदार पौधों की जड़ों की गाँठों में पाये जाते हैं।

प्रश्न 13.
खाद्य (आहार) श्रृंखला किसे कहते हैं? एक सरल आहार श्रृंखला का उदाहरण लिखिए।
उत्तर:
खाद्य श्रृंखला – जीवों की वह क्रमबद्ध श्रृंखला जो भोजन के आधार पर एक-दूसरे से सम्बन्धित रहती है, श्रृंखला कहलाती है। एक सरल खाद्य श्रृंखला नीचे दर्शायी गई है-
घास → हिरण → शेर

प्रश्न 14.
ऊर्जा के सन्दर्भ में कौन-सी आहार श्रृंखला लाभप्रद है?
उत्तर:
ऊर्जा के सन्दर्भ में कम से कम स्तर वाली आहार श्रृंखला अधिक लाभप्रद है।

प्रश्न 15.
ऊर्जा प्रवाह से क्या तात्पर्य है?
उत्तर:
किसी पारिस्थितिक तन्त्र ऊर्जा स्त्रोत से ग्रहण गई ऊर्जा का उत्पादकों से विभिन्न उपभोक्ताओं की तरफ क्रमशः स्थानान्तरित होने की क्रमबद्ध प्रणाली ही ऊर्जा प्रवाह है।

प्रश्न 16.
पारिस्थितिक तंत्र के जैव घटकों को सूचीबद्ध कीजिए।
उत्तर:
पारिस्थितिक तन्त्र के जैव घटक हैं-

• उत्पादक – हरे पांधे
• उपभोक्ता–समस्त जन्तु
• अपघटक – सूक्ष्मजीव

प्रश्न 17.
पृथ्वी पर जैव जीवों को पोषण करने वाले भागों के नाम लिखिए।
उत्तर:
पृथ्वी पर जैव जीवों को पोषण करने वाले भाग हैं-

• स्थलमण्डल
• जलमण्डल
• वायुमण्डल।

प्रश्न 18.
उपभोक्ता से क्या अर्थ है?
उत्तर:
उपभोक्ता का अर्थ उन जीवों से है जो खाद्य पदार्थ का उपभोग करते हैं। समस्त जन्तु उपभोक्ता होते हैं।

प्रश्न 19.
उत्पादक किसे कहते हैं?
उत्तर:
वे जीव जो अपना भोजन स्वयं बनाते हैं, उत्पादक कहलाते हैं। हरे पौधे प्रकाश संश्लेषण क्रिया द्वारा अपना भोजन स्वयं बनाते हैं अतः हरे पौधे उत्पादक होते हैं।

प्रश्न 20.
खाद्य जाल क्या है?
उत्तर:
जीवनण्डल में प्रतिपादित होने वाली आहार श्रृंखलाएँ आपस में अनेक प्रकार से जैसे आड़े-तिरछे जुड़कर एक जाल – सा बनाती है। खाद्य श्रृंखलाओं के इस जाल खाद्य जाल कहते हैं।

प्रश्न 21.
पारिस्थितिक पिरामिड किसे कहते हैं?
उत्तर:
आहार श्रृंखला की ऊर्जागतिकी को समझाने हेतु ऊर्जा स्थानान्तरण का पिरामिड के रूप में ग्राफीय निरूपण किया जा सकता है। इसे ही पारिस्थितिक पिरामिड कहते हैं।

प्रश्न 22.
नाइट्रोजन स्थिरीकरण का क्या अर्थ है?
उत्तर:
वायुमण्डल के स्वतन्त्र नाइट्रोजन को नाइट्रोजन के सरल घुलनशील लवणों में बदलने की प्रक्रिया नाइट्रोजन स्थिरीकरण कहलाती है।

प्रश्न 23.
नाइट्रोजन स्थिरीकरण में भाग लेने वाले जीवाणुओं के नाम लिखिए।
उत्तर:
नाइट्रोजन स्थिरीकरण में भाग लेने वाले जीवाणु हैं-

• राइजोबियम
• नील हरित शैवाल।

प्रश्न 24.
जनसंख्या किसे कहते हैं?
उत्तर:
किसी भी प्रजाति के एक ही स्थान पर पाए जाने वाले जीवों क की कुल संख्या को जनसंख्या कहते हैं।
उदाहरण – एक ही तालाब में पाये जाने वाले मेढकों की कुल संख्या इस तालाब में मेढकों की जनसंख्या है।

प्रश्न 25.
कोई चार पारिस्थितिक तंत्र का नाम लिखिए।
उत्तर:
चार पारिस्थितिक तंत्र हैं-

• तालाब
• झील
• प्राकृतिक जंगल
• खेत।

प्रश्न 26.
अपघटक क्या हैं? दो उदाहरण लिखिए।
उत्तर:
वे सूक्ष्मजीव जो मृत जीवों के जीवद्रव्यों को अपघटित कर अपना भोजन प्राप्त करते हैं, वे अपघटक कहलाते हैं। उदाहरण-बैक्टीरिया, कवक आदि।

प्रश्न 27.
पोषण स्तर से क्या समझते हैं?
उत्तर:
आहार श्रृंखला के विभिन्न स्तरों पर भोजन (ऊर्जा) का स्थानान्तरण एक निश्चित दिशा में होता है। इनमें से प्रत्येक स्तर पोषण स्तर कहलाता है।

प्रश्न 28.
नीचे दी गई आहार श्रृंखला में यदि मेढक को हटा दें तो पोषण स्तर पर क्या प्रभाव पड़ेगा?
घास → टिड्डा → मेढक → साँप → मोर
उत्तर:
दी गई आहार श्रृंखला से मेढक हटाने पर निम्नलिखित प्रभाव हो सकते हैं-

टिड्डों की संख्या में वृद्धि हो जायेगी।
घास में कमी हो जायेगी।”
टिड्रों का जीवित रहना सम्भव हो पायेगा।
साँप तथा मोर अपने भोजन के कारण अपना स्थान बदल सकते हैं।

प्रश्न 29.
जैवमण्डल में उत्पादक के दो कार्य लिखिए।
उत्तर:
जैवमण्डल में उत्पादक के कार्य-

• ये प्रकाश संश्लेषण क्रिया द्वारा उपयोगी ऑक्सीजन गैसें मुक्त करते हैं।
• ये उपभोक्ता वर्ग के जीवों के लिए भोज्य पदार्थों का निर्माण करते हैं।

प्रश्न 30.
नाइट्रीकरण किसे कहते हैं?
उत्तर:
वह प्रक्रिया जिसमें अमोनिया (NH₃) को नाइट्राइट (NO₂) तथा नाइट्रेट (NO₃) में परिवर्तित किया जाता है, नाइट्रीकरण कहलाती है।

प्रश्न 31.
विनाइट्रीकरण किसे कहते हैं?
उत्तर:
वह प्रक्रिया जिसके द्वारा मृदा में उपस्थित नाइट्रेट (NO₃) लवणों को मुक्त कर नाइट्रोजन गैस (N₂) में परिवर्तित किया जाता है, विनाइट्रीकरण कहलाती है।

प्रश्न 32.
अमोनीकरण किसे कहते हैं?
उत्तर:
जब जन्तु और पौधे मर जाते हैं तो उनके मृत शरीर में उपस्थित जन्तु प्रोटीनों एवं पादप प्रोटीनों को अपघटक जीवाणु अपघटित करके अमोनिया में बदल देते हैं जो अन्त में मृदा मिल जाती हैं। यह अमोनीकरण कहलाती है।

प्रश्न 33.
विनाइट्रीकरण करने वाले जीवाणु का नाम लिखिए।
उत्तर:
सूडोमोनास।

प्रश्न 34.
अमोनीकरण में भाग लेने वाले जीवाणु का नाम लिखिए।
उत्तर:
नाइट्रोसोमोनास।

प्रश्न 35.
जल चक्र में जीवों की क्या भूमिका है?
उत्तर:
जन्तुओं के शरीर से तथा पौधों से वाष्पोत्सर्जन की क्रिया द्वारा जल वाष्प वायुमण्डल में पहुँच जाता है जो वर्षा के रूप में पृथ्वी पर वापस आता है जिसे जीव-जन्तु तथा पौधे पुनः प्राप्त करते हैं। इस प्रकार जीवों द्वारा प्रकृति में जलचक्र सम्पन्न किया जाता है।

प्रश्न 36.
जीवाणु एवं कवक अपघटक की तरह कैसे कार्य करते हैं?
उत्तर:
जीवाणु एवं कवक मृत पौधों एवं जन्तु शरीरों को अमोनिया एवं अन्य सरल यौगिकों में अपघटित कर देते नाइट्रीकरण जीवाणु द्वारा अमोनिया नाइट्रेट में बदल दी जाती है। नाइट्रेट उर्वरक की तरह कार्य करते हैं और पौधों द्वारा अवशोषित कर लिये जाते हैं। इस प्रकार जीवाणु एवं कवक अपघटक रूप में कार्य करते हैं।

प्रश्न 37.
संघटन में जीवमण्डल को उच्चस्तरीय क्यों माना जाता है?
उत्तर:
जीवमण्डल की अपनी एक विशिष्ट संरचना होती है जो जैव एवं अजैव घटकों से बनी है। प्रत्येक घटक का एक विशिष्ट कार्य है। समस्त कार्यों का कुल योग जीवमण्डल को कार्यात्मक स्थिरता प्रदान करता है। यही कारण है कि जीवमण्डल को उच्चस्तरीय माना जाता है।

प्रश्न 38.
लेग्यूमिनस पौधों के नाम लिखिए।
उत्तर:
चना, मटर, अरहर आदि लेग्यूमिनस पौधों के उदाहरण हैं।

प्रश्न 39.
जीवोम (बायोम) किसे कहते हैं? इसका एक उदाहरण दीजिए।
उत्तर:
किसी भौगोलिक क्षेत्र के समस्त पारितन्त्रों के सामूहिक रूप को जीवोम कहते हैं। यह एक बड़ा भूमि क्षेत्र होता है जिसमें लगभग एक ही प्रकार के जन्तु और पौधे पाये जाते हैं और जिनमें सभी जगह भौतिक वातावरण भी एक समान ही होता है। उदाहरण के लिए किसी एक जीवोम में कई तालाब, झील, घास का मैदान या वन भी दृष्टिगोचर हो सकते हैं।

लघु उत्तरीय प्रश्न

प्रश्न 1.
पोषण स्तर क्या है? विभिन्न पोषण स्तर वाली कोई आहार श्रृंखला को समझाइए।
उत्तर:
आहार श्रृंखला में उत्पादक तथा उपभोक्ता का स्थान ग्रहण करने वाले जीव पारिस्थितिक तंत्र या जीवमण्डल द्वारा भी खाया जा को कोई निश्चित संरचना प्रदान करते हैं। इनको पोषण स्तर कहते हैं। कोई एक जीव एक से अधिक आहार शृंखलाओं में स्थान ग्रहण कर सकता है। उदाहण के लिए हिरण, सियार द्वारा खाया जा सकता है और सकता है।

चित्र में 4 पोषण आहार श्रृंखला दर्शायी गई हैं। ये आहार श्रृंखला न केवल जीवमण्डल में जैव घटकों संरचना प्रदर्शित करती हैं बल्कि दो महत्त्वपूर्ण प्रकार्यों भी प्रदर्शित करती हैं। ये प्रकार्य हैं, ऊर्जा एवं पदार्थों का की को स्थानान्तरण।। यही दो मूल कार्य जीवमण्डल को गतिशीलता प्रदान करते हैं।

प्रश्न 2.
जीवमण्डल के जैव घटक कौन-कौन से हैं? इनका पोषण पद्धति के आधार पर वर्गीकरण कीजिए।
उत्तर:
जीवमण्डल के जैव घटक पौधे एवं जन्तु हैं। इन जीवों को इनकी पोषण पद्धति के आधार पर उत्पादक, उपभोक्ता तथा अपघटक के रूप में वर्गीकृत किया जा सकता है।

उत्पादक – उत्पादक वे जीव होते हैं जो साधारण अकार्बनिक पदार्थों से सूर्य की उपस्थिति में प्रकाश संश्लेषण क्रिया द्वारा जटिल पदार्थों (कार्बोहाइट्रेट) का निर्माण करते हैं। इसके अन्तर्गत समस्त हरे पौधे आते हैं।

उपभोक्ता – ये वे जीव होते हैं जो वनस्पति या उनके द्वारा एकत्रित भोज्य पदार्थों का उपयोग करते हैं। इस वर्ग के जीव अपना भोजन स्वयं नहीं बनाते बल्कि दूसरे जीवों पर निर्भर रहते हैं। उपभोक्ता मुख्यत: तीन प्रकार के हो सकते हैं-

• शाकाहारी
• मांसाहारी
• सर्वभक्षी।

अपघटक – ये सूक्ष्मजीव होते जिनके अन्तर्गत जीवाणु, कवक तथा फंगस आते हैं। ये जीव अपना भोजन मृत पौधों एवं जन्तुओं के शरीर का अपघटन करके बनाते हैं। ये पर्यावरण में पदार्थों के चक्रण में प्रमुख भूमिका निभाते हैं।

प्रश्न 3.
पारिस्थितिक पिरामिड किसे कहते हैं? पिरामिड को उदाहरण सहित समझाइये।
उत्तर:
पारिस्थितिक पिरामिड-किसी पारिस्थितिक तंत्र में एक खाद्य शृंखला के विभिन्न पोषी स्तरों की संख्या, जीव भार तथा संचित ऊर्जा का आलेखी निरूपण पारिस्थितिक पिरामिड कहलाता है।

जीवसंख्या का पिरामिड-किसी पारितन्त्र के उत्पादकों की संख्या शाकाहारियों की संख्या से अधिक होती है तथा शाकाहारियों की संख्या मांसाहारियों से अधिक होती है। प्रत्येक आधार पर जीवों की संख्या बहुत अधिक होती है। परन्तु जैसे हम शीर्ष की ओर बढ़ते हैं, उनकी संख्या कम होती जाती है। इसे जीवसंख्या का पिरामिड कहते हैं।

उदाहरण – नीचे संख्या को आधार मानकर एक जीवसंख्या का पिरामिड दिखाया गया है जिसे उत्पादकों (घास) की संख्या सबसे ज्यादा तथा उसके बाद प्रथम श्रेणी के उपभोक्ता शाकाहारी (चूहे) की संख्या कम, उसके बाद द्वितीय श्रेणी के उपभोक्ता मांसाहारी (साँप ) व तृतीय श्रेणी के उपभोक्ता सर्वोच्च मांसाहारी (बाज ) की संख्या क्रमशः कम होती जाती है।

प्रश्न 4.
क्या आहार श्रृंखला में छह से अधिक स्तर हो सकते हैं? यदि नहीं तो क्यों?
उत्तर:
आहार श्रृंखला में छह से अधिक स्तर नहीं हो सकते हैं।

कारण – ऊर्जा स्थानान्तरण 10% नियम के अनुसार किसी आहार श्रृंखला में प्रत्येक स्तर पर ऊर्जा स्थानान्तरण के समय ऊर्जा का 90% भाग वातावरण में चला जाता है। और केवल 10% भाग ही अगले स्तर में जा पाता है। इस प्रकार छठवें स्तर पर पहुँचने पर ऊर्जा की मात्रा इतनी कम रह जाती है कि यदि आहार श्रृंखला में एक और स्तर बढ़ा दिया जाये तो अन्तिम उपभोक्ता को बहुत कम ऊर्जा प्राप्त होगी, जो उसे जीवित रहने के लिए पर्याप्त नहीं होगी।

प्रश्न 5.
पदार्थों के चक्रण में अपघटकों की भूमिका संक्षेप में समझाइये।
अथवा
जीवमण्डल के अपघटकों के महत्त्व लिखिए।
उत्तर:
अपघटक, जीवाणु, कवक तथा फंगस आदि सूक्ष्मजीवी होते हैं जो जीवमण्डल में मृत पेड़-पौधों (उत्पादकों) एवं मृत जन्तुओं (उपभोक्ताओं) का अपघटन करते हैं। ये उन जीवों में उपस्थित जटिल कार्बनिक पदार्थों को सरल अकार्बनिक पदार्थों में बदल देते हैं जिनका उपयोग पुन: उत्पादकों द्वारा कर लिया जाता है।

इस प्रकार तत्त्व पुनः परिवहन में आ जाते हैं, किन्तु अपघटक की अनुपस्थिति में उत्पादक द्वारा भोजन के रूप में ग्रहण किये गये तत्त्व (मुख्यत: C, N, O, S व P) पुनः जीवमण्डल में परिवहन में नहीं आ सकते हैं। फलस्वरूप पोषण भण्डार खाली रह जायेगा। एक बार अवशोषित किये जाने के बाद पदार्थ पुनः उपयोग करने योग्य रूप में उपलब्ध नहीं हो सकेगा और पारिस्थितिक तन्त्र में असन्तुलन की स्थिति हो सकती है।

प्रश्न 6.
जीवमण्डल में अपघटकों की अनुपस्थिति के क्या परिणाम हो सकते हैं?
उत्तर:
अपघटक सूक्ष्मजीव होते हैं जिसके अन्तर्गत जीवाणु तथा कवक या फंगस आते हैं। यदि जीवमण्डल में अपघटक अनुपस्थित रहे तो इसके निम्नलिखित परिणाम हो सकते हैं-

• अनेक प्रकार के प्राकृतिक चक्र जैसे- नाइट्रोजन चक्र और CO₂ चक्र बाधित हो जायेंगे।
• प्राकृतिक चक्रण के बाधित होने से प्रकृति का सन्तुलन बिगड़ सकता है।
• यदि अपघटक अनुपस्थित रहे तो धरातल में मृत अवशेष के ढेर लग जायेंगे, गन्दगी व दुर्गन्ध बढ़ जायेगी, क्योंकि अपघटकों का कार्य सभी मृत जीवों के अवशेष्षों को अपघटित कर सरल रूपों में तोड़ना है।

प्रश्न 7.
आहार श्रुंखला छोटी करने से क्या प्रभाव पड़ेगा?
उत्तर:
आहार श्रृंखला जीवमण्डल में दो महत्त्वपूर्ण प्रकार्य करती है। ये प्रकार्य हैं-ऊर्जा एवं पदार्थों का स्थानान्तरण जो जीवमण्डल को गतिशीलता प्रदान करती है। क्योंकि आहार शृंखला में ऊर्जा एक निश्चित दिशा में ही प्रवाहित होती है। इसकें विपरीत दिशा में प्रवाहित नहीं हो सकती अर्थात् आहार शृंखला में प्रत्येक स्तर का महत्त्व होता है। अब यदि एक सरल आहार श्रृंखला घास → हिरण → शेर को हटाकर, श्रृंखला को छोटी कर दिया जाय तो हिरणों की संख्या में अत्यधिक वृद्धि हो जापेगी तब इसका प्रभाव उत्पादन स्तर (घास) पर अधिक पड़ेगा और जीवमण्डल बुरी तरह प्रभावित हो जायेगा।

प्रश्न 8.
खाध्य भ्वृंखला एवं खाद्य जाल में अन्तर बताइये।
उत्तर:
खाद्य शृंखला एवं खाद्य जाल में अन्तर निम्नलिखित हैं-

खाद्य भृंखला	खाद्य जाल
1. जीवों की शृंखला जो भोजन के आधार पर एक-दूसरे से सम्बन्धित रहती है, खाद्य श्रृंखला कहलाती है।	1. खाद्ध जाल विभिन्न खाद्य शृंखलाएँ मिलकर खाद्य जाल बनाती हैं।
2. इसमें विभिन्न उपभोक्ता, अपघटनकर्ता तथा उत्पादक भाग लेते हैं।	2. इसमें अनेक जीवसमुदाय के उपभोक्ता, अपघटनकर्ता भाग लेते हैं।
3. इससे ऊर्जा का प्रवाह एक ही दिशा में होता है।	3. इसमें ऊर्जा का प्रवाह एक-ही दिशा में होते हुए कई पथों से होकर गुजरता है।

प्रश्न 9.
जीवमण्डल में ऊर्जा का प्रवाह कैसे होता है?
उत्तर:
जीवमण्डल में ऊर्जा का प्रवाह पोषण रीति द्वारा एक ही दिशा में होता है। हरे पौधे प्रथम पोषी स्तर हैं जहाँ सौर ऊर्जा अधिग्रहण करके रासायनिक ऊर्जा के रूप में संचित हो जाती है। खाद्य पदार्थों के रूप में भण्डारित यह रासायनिक ऊर्जा भोजन के साथ ही शाकाहारी जन्तुओं तक स्थानान्तरित हो जाती है यह द्वितीय पोषी स्तर है। शाकाहारियों का भक्षण मांसाहारियों द्वारा होता है, यह तृतीय पोषी स्तर है। इस प्रकार जीवमण्डल में ऊर्जा तीन स्तर पर प्रवाहित होती है।

प्रश्न 10.
प्रकृति में कार्बन डाइऑक्साइड चक्र को समझाइये।
उत्तर:
कार्बन डाइऑक्साइड चक्र-प्रकृति में कार्बन डाइऑक्साइड चक्र निम्न प्रकार से सम्पादित होता है-

• वायुमण्डल की CO₂ का कुछ भाग जल में घुल जाता है जिसका उपयोग जलीय पौधे एवं वायुमण्डल की हैं। इस प्रकार CO₂ के रूप में उपस्थित कार्बन भोजन के शेष CO₂ का उपयोग स्थलीय पौधे प्रकाश-संश्लेषण में करते रूप में एकत्रित हो जाता है।
• पौधों में यह कार्बन शाकाहारी जन्तुओं और फिर उनसे मांसाहारी जन्तुओं में भोजन के रूप में पहुँचता है।
• पौधों एवं जन्तुओं के श्वसन से तथा मृत जीवों के अपघटन से CO₂ बनती है, जो वायुमण्डल में वापस चली जाती है।
• पौधों से कोयला बनता है तथा पौधों एवं जन्तुओं पृथ्वी के गर्त में पेट्रोलियम बनता है जो हाइड्रोकार्बन होता है।
• कोयले के जलने तथा पेट्रोलियम के दहन से पुनः CO₂ बनती है, जो वायुमण्डल में चली जाती है। इस प्रकार प्रकृति में कार्बन डाइऑक्साइड चक्र पूर्ण होता है।
  

प्रश्न 11.
जीवमण्डल के जैविक घटक उदाहरण देकर समझाइये | घटक-जीवमण्डल के जैविक घटक
उत्तर:
जैविक के अन्तर्गत पौधे तथा जन्तु होते हैं। इन जैविक घटकों को उनकी पद्धति के आधार पर तीन समूहों में वर्गीकृत किया जाता है-
(a) उत्पादक- इसमें हरे पौधे आते हैं जो कि साधारण अकार्बनिक पदार्थों से सूर्य के प्रकाश की उपस्थिति में प्रकाश संश्लेषण क्रिया द्वारा जटिल पदार्थों का निर्माण करते हैं। ये प्राथमिक या मूल उत्पादक कहलाते हैं, क्योंकि पारिस्थितिक तंत्र में पाये जाने वाले सभी जीव इन्हीं अपना भोजन प्राप्त करते हैं।

(b) उपभोक्ता इसमें वे जीव आते हैं जो वनस्पति या उनके द्वारा एकत्रित भोज्य पदार्थों का उपयोग करते हैं। इसे तीन वर्गों में बाँटते हैं –

• प्राथमिक उपभोक्ता इसमें वे जीव आते हैं जो वनस्पतियों या उनके द्वारा प्राप्त अन्य किसी भाग को खाते हैं, जैसे- भेड़, बकरी, टिड्डे, घोड़ा आदि।
• द्वितीयक उपभोक्ता इसमें वे जीव आते हैं जो शाकाहारी जन्तुओं को अपने भोजन के रूप में ग्रहण करते हैं; जैसे – मोर, लोमड़ी, मेंढक आदि।
• तृतीयक उपभोक्ता – इसके अन्तर्गत वे जन्तु आते हैं जो द्वितीयक उपभोक्ताओं (मांसाहारी) को खा जाते हैं, इसे सर्वश्रेष्ठ उपभोक्ता कहते हैं।

(c) अपघटक – इस वर्ग में मुख्य रूप से जीवाणुओं तथा कवकों को रखा गया है जो मरे हुए उत्पादकों तथा उपभोक्ता को सड़ाकर साधारण भौतिक तत्त्वों में बदल देते हैं जो फिर से वातावरण में मिल जाते हैं जिन्हें पुनः हरे पौधे अवशोषित करके सूर्य प्रकाश में भोजन के रूप में परिवर्तित कर देते हैं। इस प्रकार प्रत्येक पारिस्थितिक तंत्र में यह चक्र चलता रहता है तथा तंत्र का प्रत्येक जीव एक-दूसरे पर निर्भर रहता है।

प्रश्न 12.
जीवमण्डल में नाइट्रोजन चक्र को एक आदर्श चक्र क्यों माना जाता है?
उत्तर:
वायुमण्डल में नाइट्रोजन सर्वाधिक (लगभग 78%) मात्रा में उपलब्ध है। इतनी अधिक मात्रा में उपलब्ध होने के बाद भी जीवधारी सीधे ही नाइट्रोजन का उपयोग नहीं कर पाते हैं। पौधे नाइट्रेट एवं नाइट्राइट्स के रूप में ग्रहण कर सकते हैं जो पौधों में दो प्रकार से पहुँचती है-
(अ) वायुमण्डल की स्वतन्त्र नाइट्रोजन बिजली कड़कने में ऑक्सीजन से मिलकर नाइट्रोजन के ऑक्साइड बनाते हैं। ये ऑक्साइड वर्षा के जल में घुलकर नाइट्रेट में परिवर्तित हो जाते हैं और मृदा में मिल जाते हैं।

(ब) सूक्ष्म जीवाणु नीले-हरे शैवाल द्वारा वायु की नाइट्रोजन का यौगिकीकरण करके नाइट्रेट बना लेते हैं। इसे पौधे अपनी जड़ों द्वारा घोल के रूप में लेते हैं। पौधे इसे अमीनो अम्ल में बदलकर प्रोटीन का निर्माण करते हैं। यह प्रोटीन पौधों से जन्तुओं में पहुँच जाती है। इस रूप में जन्तु वजन्तु मृत होने पर इनमें उपस्थित प्रोटीन मृतोपजीवी जीवाणु द्वारा अमोनिया नाइट्राइट व नाइट्रेट में परिवर्तन कर देते हैं।

इस प्रकार नाइट्रोंजन चक्र वायुमण्डल तथा जल भण्डारों में नाइट्रोजन की कुल मात्रा को अपरिवर्तित रखता है। इसलिए जीवमण्डल में नाइट्रोजन चक्र को एक आदर्श चक्र माना जाता है।

प्रश्न 13.
10% (दस प्रतिशत) नियम को स्पष्ट कीजिए।
उत्तर:
खाद्य- शृंखला के प्रत्येक चरण पर उपलब्ध ऊजा उससे पहले के चरण पर उपलब्ध ऊर्जा का लगभग 10 % होती है। इसे ऊर्जा का 10 % नियम कहते हैं।

उदाहरण-मान लो, यदि सूर्य से पृथ्वी पर 1000 J ऊर्जा किसी हरे पौंधे पर आपतित होती है तो हरा पौधा इसके केवल 1 % भाग को ही भोजन के रूप में परिवर्तित कर पाता है। अत: 1000 J में से 10 J भोजन के रूप में पौधे में जमा हो जाती है, शेष 990 J वातावरण में चली जाती है।

यदि कोई हिरण इस पौधे को खाता है तो उसके शरीर में 10 J ऊर्जा प्रवेश करेगी, परन्तु इसका केवल 10 % अर्थात् 1 J ही उसके शरीर में भोज्य पदार्थ के रूप में, जमा होगी। शेष 9 J पुन: पर्यावरण में मुक्त हो जायेगी। अत: यदि कोई शेर इस हिरण को खाता है तो उसे केवल 1 जूल ऊर्जा ही भोजन की प्राप्त होगी। ऊर्जा हानि का यह क्रम हर पोषण स्तर पर चलता रहता है। इसे निम्न चित्र से दर्शा सकते हैं-

दीर्घ उत्तरीय प्रश्न

प्रश्न 1.
प्रकृति में नाइट्रोजन चक्र को चित्र की सहायता से समझाइए।
उत्तर:
नाइट्रोजन चक्र – वायुमण्डल में नाइट्रोजन का समृद्ध भण्डार है। इसमें लगभग 78% नाइट्रोजन होती है। जो आण्विक रूप (N) में उपस्थित रहती है। जल भण्डारों में भी नाइट्रोजन होती है। यह जैव जीवों द्वारा इसके तत्त्व के रूप में उपयोग में नहीं लाई जा सकती है। इसका जीवमण्डल में प्रवेश कुछ विशेष जीवों द्वारा ही सम्भव हो पाता है।

वायुमण्डल से जीवमण्डल में नाइट्रोजन का प्रवेश- चना, मटर, मूँगफली आदि फलीदार पौधों की जड़ों में कुछ गाँठें होती हैं जिनमें राइजोबियम नामक जीवाणु रहते हैं जो वायुमण्डल। की नाइट्रोजन का स्थिरीकरण करके उसे नाइट्रेट्स में बदल देती हैं। कुछ नील हरित शैवाल भी की नाइट्रोजन का स्थिरीकरण करके उसे यौगिक में में बदल देते हैं जिसका उपयोग पौधे कर पाते हैं।

कुछ बिना फलीदार पौधे जैसे एनलस तथा ग्रीनकी भी वायुमण्डल की नाइट्रोजन का स्थिरीकरण करते हैं तथा उसे नाइट्रेटों में परिवर्तित करते हैं। वायुमण्डल में उपस्थित नाइट्रोजन का स्थिरीकरण वायुमण्डलीय एवं औद्योगिक प्रक्रियाओं द्वारा हो सकता है।

जीवमण्डल से नाइट्रोजन का वापस वायुमण्डल में पहुँचना – मृदा में उपस्थित नाइट्रेटों का सूडोमोनास नामक जीवाणु विनाइट्रीकरण प्रक्रिया द्वारा नाइट्रोजन गैस में परिवर्तित कर देते हैं जो वापस वायुमण्डल में पहुँच जाती है जहाँ से वह प्रारम्भ में आयी थी। जीवमण्डल में यह क्रिया बार-बार दोहराई जाती है जिससे वायुमण्डल में नाइट्रोजन का अनुपात स्थिर बना रहता है।

जीव मण्डल में नाइट्रोजन चक्र में निम्नलिखित मुख्य चरण होते हैं-

प्रश्न 2.
जीवमण्डल में ऊर्जा के प्रवाह को उदाहरण देकर स्पष्ट कीजिए।
उत्तर:
जीव जगत में ऊर्जा का प्रवाह आहार श्रृंखला के माध्यम से होता है। जीवमण्डल के जैव घटकों में सौर ऊर्जा हरे पौधों द्वारा की जाने वाली प्रकाश संश्लेषण प्रक्रिया के दौरान प्रवेश करती है। हरे पौधे सूर्य की ऊर्जा को अन्तर्ग्रहण: करके प्रकाश संश्लेषण द्वारा कार्बोहाइड्रेट के रूप में संचित करते करते हैं।

यह प्रारम्भिक चरण है जिसमें पर्यावरण से ऊर्जा जैव घटकों में प्रवेश करती है। केवल पौधों में ही के कारण सौर ऊर्जा का अन्तर्ग्रहण करने की क्लोरोफिल के क्षमता होती है। पौधे इस ऊर्जा का उपयोग अपनी उपापचयी प्रक्रियाओं को सम्पन्न करने में करते हैं। इस ऊर्जा का मुख्य अंश श्वसन, वृद्धि अथवा ऊतक निर्माण में उपयोग होता है। कुल ऊर्जा उपयोग नहीं होती है।

पौधों का शाकाहारियों द्वारा भक्षण कर लिया जाता है। इन पौधों में उपस्थित रासायनिक ऊर्जा भोजन के साथ शाकाहारी जन्तुओं में स्थानान्तरित हो जाती है। शाकाहारी इस ऊर्जा के कुछ हिस्से का उपयोग उपापचयी प्रक्रियाओं श्वसन तथा वृद्धि हेतु करते हैं। इस ऊर्जा का कुछ भाग उपयोग नहीं हो पाता है।

शाकाहारियों का भक्षण मांसाहारियों द्वारा होता है। इस प्रकार शाकाहारी जन्तु में मांस में भण्डारित रासायनिक ऊर्जा मांसाहारी जन्तु में स्थानान्तरित हो जाती है। मांसाहारी ऊर्जा के कुछ भाग का उपयोग अपनी उपापचयी प्रक्रियाओं, श्वसन एवं वृद्धि हेतु करते हैं कुछ ऊर्जा उपयोग नहीं हो पाती है।

इस प्रकार मांसाहारी जन्तुओं का भक्षण सर्वोच्च मांसाहारियों द्वारा किया जाता है। इस स्तर में भी ऊर्जा का उपयोग पूर्व के पोषण स्तर द्वारा किये गये प्रक्रियाओं के लिए ही किये जाते हैं। उपर्युक्त प्रक्रियाओं से स्पष्ट है, जीवमण्डल में आहार श्रृंखला में किसी पोषण रीति से अगली पोषण रीति तक ऊर्जा का प्रवाह (स्थानान्तरण) होता रहता है।

प्रश्न 3.
प्रकृति में जल चक्र को स्पष्ट कीजिए।
उत्तर:
जल चक्र – पर्यावरण में जल का परिवर्तित होते रहना जल चक्र कहलाता है।

जल में मुख्य तत्व हाइड्रोजन होता है जिसका चक्रण पानी के यौगिक के रूप में प्रवाहित होने के साथ होता है। जलाशयों जैसे सागर, समुद्र, झील, नदियों आदि तथा जैव जीवों के शरीर की सतह से जल का लगातार वाष्पन होता रहता है। पौधों में जल हानि मुख्यतः वाष्पोत्सर्जन द्वारा होता है। इस प्रकार वाष्पित जल वायु में जल वाष्प के रूप में उपस्थित रहता है। निम्न ताप पर ये वाष्प संघनित होकर जल की बूँद बनाते हैं। ये बूंदें वर्षा अथवा हिम रूप में बरसती हैं और इस प्रकार जल पुनः पृथ्वी पर वापस आ जाता है। यह प्रक्रिया लगातार चलती रहती है। यह जल चक्र है।

प्रश्न 4.
जीवमण्डल के घटकों को संक्षेप में बताइये।
उत्तर:
जीवमण्डल के मुख्यतः दो घटक होते हैं-

• अजैविक घटक
• जैविक घटक।

(1) अजैविक घटक – पारिस्थितिक तंत्र में पाये जाने वाले निर्जीव पदार्थों या घटकों को इस वर्ग में रखते हैं जो जैविक घटकों को नियंत्रित करता है। अजैविक घटकों को तीन भागों में बाँट सकते हैं-

• कार्बनिक पदार्थ – इसके अन्तर्गत कार्बोहाइड्रेट, प्रोटीन आदि आते हैं। ये पारिस्थितिक तंत्र को ऊर्जा देते हैं।
• अकार्बनिक पदार्थ ऑक्सीजन, नाइट्रोजन, जल, कैल्सियम, फॉस्फोरस, लोहा आदि अकार्बनिक पदार्थ निर्मित करते हैं। ये कभी खत्म नहीं होते बल्कि इनका नियमित चक्र चलता रहता है।
• जलवायु – प्रकाश, तापक्रम, वर्षा तथा पहाड़ इस वर्ग में आते हैं, जो किसी स्थान के वातावरण को निर्धारित करते हैं।

(2) जैविक घटक – इसके अन्तर्गत पौधे एवं जन्तु आते हैं। पोषण पद्धति के आधार पर इन्हें तीन समूहों में वर्गीकृत किया गया है-

• उत्पादक- इसमें हरे पौधे आते हैं जो कि साधारण अकार्बनिक पदार्थों से सूर्य प्रकाश का उपस्थिति में प्रकाश संश्लेषण द्वारा जटिल पदार्थों को निर्माण करते हैं। पारिस्थितिक तंत्र में पाये जाने वाले सभी जीव इन्हीं से अपना भोजन प्राप्त करते हैं।
• उपभोक्ता इसमें वे जीव आते हैं जो वनस्पति या उनके द्वारा एकत्रित भोज्य पदार्थों का उपयोग करते हैं।
• अपघटक जीवाणु एवं कवक इस वर्ग के अन्तर्गत आते हैं। ये मरे हुए उत्पादकों तथा उपभोक्ता को सड़ाकर साधारण भौतिक तत्त्वों में बदल देते हैं जो फिर से वातावरण में मिल जाते हैं जिन्हें पुनः हरे पौधे अवशोषित करके सूर्य प्रकाश में भोजन के रूप में परिवर्तित कर देते हैं।

प्रश्न 5.
कार्बन चक्र को चित्र की सहायता से स्पष्ट कीजिए।
उत्तर:
कार्बन चक्र – कार्बन का स्थानान्तरण आहार श्रृंखला के माध्यम से होता है। वायुमण्डल में यह कार्बन डाइऑक्साइड के रूप में प्राप्त होता है। इस कार्बन डाइऑक्साइड को हरे पौधे संश्लेषण प्रक्रिया द्वारा कार्बोहाइड्रेट के रूप में उपयोग करते हैं।

कार्बोहाइड्रेट खाद्य पदार्थ के रूप में एक पोषण रीति के जन्तुओं से दूसरे स्तर के जन्तुओं स्थानांतरित होता रहता है अपघटकों द्वारा यह फिर से वायुमण्डल एवं जल भण्डारों में वापस आ जाता है। वायुमण्डल एवं जल भण्डारों के मध्य CO₂ का विनिमय निरंतर चलता रहता है। ज्वालामुखी उद्गार से भी वायुमण्डल में CO₂ मुक्त होती है।

प्रश्न 6.
ऑक्सीजन चक्र को रेखाचित्र द्वारा समझाइये।
उत्तर:
वायुमण्डल के गैसीय घटकों का लगभग 21%

ऑक्सीजन है। जल भण्डारों में यह पानी में घुली हुई स्थिति में होती है। इनमें से वायुमण्डलीय ऑक्सीजन का उपयोग स्थलीय जन्तुओं द्वारा तथा जल में घुली हुई ऑक्सीजन का उपयोग जलीय जन्तुओं द्वारा किया जाता है। इस प्रकार ऑक्सीजन का प्रवेश जीवमण्डल में होता है। कोशिकीय श्वसन की प्रक्रिया कार्बन डाइऑक्साइड एवं जल बनता है। जल एवं कार्बन डाइऑक्साइड पेड़-पौधों द्वारा प्रकाश संश्लेषण प्रक्रिया के दौरान ग्रहण किये जाते हैं और ऑक्सीजन मुक्त की जाती है जो वायुमण्डल में चली जाती है।

इस प्रकार जीवमण्डल से ऑक्सीजन वापस वायुमण्डल में पहुँचती है। मृत जीवों के अपघटन से भी ऑक्सीजन मुक्त होकर वायुमण्डल में मिल जाती है। इस प्रकार ऑक्सीजन चक्र चलता रहता है और वायुमण्डल में ऑक्सीजन की मात्रा स्थिर बनी रहती है।

प्रश्न 7.
स्थलमण्डल और वायुमण्डल में कोई चार अन्तर लिखिए।
उत्तर:
स्थलमण्डल एवं वायुमण्डल में चार अन्तर निम्नलिखित हैं-

स्थलमण्डल	वायुमण्डल
1. भूमण्डल का वह भाग जिसके अन्तर्गत सभी ठोस स्रोत सम्मिलित किये जाते हैं, स्थलमण्डल कहलाता है।	1. इस मण्डल में वायु तथा समस्त गैसों का मिश्रण होता है। यह मण्डल अन्य दोनों मण्डलों को ढके रहता है।
2. यह मण्डल कठोर होता है।	2. यह वायु की उपस्थिति के कारण कठोर नहीं होता है।
3. स्थलमण्डल उपापचय क्रियाओं के लिए खनिज पदार्थों की पूर्ति करता है।	3. यह मुख्यतः वायु में उपस्थित पोषकों के चक्र को पूरा करता है।
4. इसमें खनिज तत्त्व प्रचुर मात्रा में पाये जाते हैं।	4. इसमें गैसीय तत्त्व प्रचुर मात्रा में पाये जाते हैं।

प्रश्न 8.
आहार श्रृंखला छोटी कैसे हो जाती है?
उत्तर:
आहार श्रृंखला में उत्पादक स्तर पर अधिक ऊर्जा उपलब्ध होती है। जैसे-जैसे हम उत्पादक स्तर से दूर होते हैं वैसे-वैसे ऊर्जा की मात्रा भी कम होती जाती है और उत्पादक स्तर के हम जितने करीब होंगे ऊर्जा की मात्रा उतनी ही अधिक प्राप्त होगी। मनुष्य विवेकशील प्राणी होने के कारण अपने हित की बात सोचता है और अधिक ऊर्जा प्राप्ति हेतु उत्पादक के करीब का उपभोक्ता बनना चाहता है, जिससे
प्राकृतिक तौर पर पायी जाने वाली आहार श्रृंखला टूटकर छोटी हो जाती है। अतः कहा जा सकता है कि मनुष्य के निहित स्वार्थ के कारण आहार श्रृंखला छोटी हो जाती है।

प्रश्न 9.
पारिस्थितिक तन्त्र किसे कहते हैं? उदाहरण देकर समझाइये।
उत्तर:
जैव संसार में जैव ऊर्जा एवं पदार्थ का निवेश आवश्यक है, जिनका जैव तथा अजैव घटकों के मध्य लगातार विनिमय होता रहता है। यह कार्यात्मक तन्त्र पारिस्थितिक तन्त्र कहलाता है। घास का मैदान, वन, नदी, समुद्र आदि पारिस्थितिक तन्त्र के उदाहरण हैं।

सभी पारिस्थितिक तन्त्र दो घटकों- जैविक घटक एवं अजैविक घटक से मिलकर बने हैं। उत्पादक जीव वायु, जल तथा मृदा आदि से CO₂ तथा जल को अवशोषित करते हैं तथा सौर ऊर्जा का उपयोग करके प्रकाश संश्लेषण द्वारा अपना भोजन बनाते हैं कुछ उत्पादक जीव उपभोक्ता जीवों द्वारा खा लिए जाते हैं।

फलस्वरूप उत्पादक की ऊर्जा उपभोक्ताओं को स्थानान्तरित हो जाती है। मृत पौधों एवं जन्तुओं को अपघटक जीव विघटन करके CO₂ तथा जल इत्यादि बना देते हैं जो वातावरण में वापस चले जाते हैं। यह प्रक्रिया बार-बार दोहराई जाती है जिससे पारिस्थितिक तन्त्र लगातार चलता रहता है।

प्रश्न 10.
खाद्य श्रृंखला किसे कहते हैं? घास पारितन्त्र में पायी जाने वाली खाद्य श्रृंखला को समझाइए।
उत्तर:
खाद्य श्रृंखला – किसी जीव द्वारा किसी अन्य जीव का उपभोग करने की क्रमबद्ध प्रक्रिया को खाद्य श्रृंखला कहते हैं। घास पारितन्त्र में पायी जाने वाली एक खाद्य श्रृंखला को नीचे बताए गए प्रारूप में प्रदर्शित किया जा सकता है-
घास → कीट → मेढक → पक्षी
उपर्युक्त खाद्य श्रृंखला चार चरणों वाली है जिसमें घास उत्पादक स्तर दर्शाती है। यह प्रकाश संश्लेषण द्वारा अपना भोजन कार्बोहाइड्रेट के रूप में बनाती है। इसका कीट द्वारा उपभोग किया जाता है। कीट का उपभोग मेढक तथा मेढक का उपभोग पक्षी करते हैं। इस श्रृंखला में कीट जो केवल घास खाते हैं, शाकाहारी कहलाते हैं। कीट को खाने वाले मेढक हैं, जो मांसाहारी कहलाते हैं। इस श्रृंखला में पक्षी पौधे तथा कीट दोनों का ही उपयोग करते हैं जो सर्वभक्षी कहलाते हैं।

प्रश्न 11.
जीवमण्डल क्या है? इसकी संरचना को संक्षेप में समझाइये।|
उत्तर:
जीवमण्डल – भू-मण्डल का वह भाग जहाँ जीवन की सम्भावनाएँ होती हैं अर्थात् पृथ्वी के स्थलमण्डल, जलमण्डल और वायुमण्डल तथा उनमें विद्यमान सभी जीवों को एक साथ जीवमण्डल कहते हैं।

जीवमण्डल की संरचना – जीवमण्डल पृथ्वी के एक मोटी सतह के रूप में पाया जाता है जिसकी मोटाई लगभग 10 किलोमीटर है। यह तीन भागों से मिलकर बना है-

• स्थलमण्डल
• जलमण्डल
• वायुमण्डल, जिसमें जीव उपस्थित रहते हैं।

(i) जलमण्डल – यह जीवमण्डल का वह भाग जिसमें जल पाया जाता है। इसके अन्तर्गत समुद्र, नदियाँ, तालाब एवं भूमि पर स्थित अन्य जल स्त्रोत आते हैं।

(ii) स्थलमण्डल – यह जीवमण्डल का वह भाग है जिसमें मृदा एवं चट्टानें पायी जाती है। यह पृथ्वी के स्थलीय सतह पर एवं जल अंदर भी उपलब्ध है।

(iii) वायुमण्डल- पृथ्वी की सतह से ऊपर का वह भाग जिसमें वायु (गैसीय मिश्रण) पायी जाती है, वायुमण्डल कहलाता है। यह जल तथा स्थल दोनों मण्डलों को घेरे रहता है।

उपरोक्त तीनों मण्डलों के साथ समस्त जैव जीव भी जीवमण्डल के अन्तर्गत सम्मिलित किये गये हैं।

प्रश्न 12.
खाद्य जाल को उदाहरण देकर सचित्र समझाइये।
उत्तर:
किसी भी पारिस्थितिक तंत्र या जीवमण्डल में प्रतिपादित होने वाली आहार श्रृंखलाएँ आपस में अनेक प्रकार से जैसे आड़े-तिरछे जुड़कर एक जाल सा बनाती हैं। आहार श्रृंखलाओं के इस जाल को खाद्य जाल कहते हैं।

उदाहरण – नीचे दिये गये खाद्य जाल में कई खाद्य श्रृंखलाएँ हैं, जो आपस में जुड़े हुए हैं-

अर्थात् चूहा उपर्युक्त दो खाद्य श्रृंखलाओं में शामिल है अतः ये शृंखलाएँ एक-दूसरे को चूहा पर जोड़ती हैं। इसी प्रकार अन्य दो श्रृंखलाएँ सर्प पर एक-दूसरे को जोड़ती हैं। इस प्रकार आड़ी-तिरछी जुड़ना ही खाद्य जाल है।

प्रश्न 13.
स्वपोषी व परपोषी से आप क्या समझते है?
उत्तर:
प्रकृति में खाद्य सामग्री के संश्लेषण के आधार पर जीव दो वर्गों में विभाजित किये जा सकते हैं- (i) स्वपोषी, (ii) परपोषी।

• स्वपोषी वे जीव जो अपना भोजन सरल पदार्थों से संश्लेषित कर लेते हैं, स्वपोषी कहलाते हैं। हरे पौधे स्वपोषी होते हैं और ये ऊर्जा के उत्पादक हैं।
• परपोषी जीव-वे जीव जो अपना भोजन स्वयं नहीं बना पाते हैं और भोजन के लिए अन्य जीवों पर निर्भर रहते हैं परपोषी कहलाते हैं। हरे पौधों और कुछ जीवाणुओं को छोड़कर अन्य सभी जीव परपोषी हैं।

प्रश्न 14.
हम क्यों कहते हैं कि जीवमण्डल में ऊर्जा का प्रवाह केवल एक ही दिशा में होता है?
उत्तर:
उत्पादक के रूप में हरे पौधे सूर्य से ऊर्जा ग्रहण करके प्रकाश संश्लेषण द्वारा भोजन का निर्माण करते हैं। शाकाहारी जन्तु भोजन के रूप में ऊर्जा को पौधों से ग्रहण करते हैं। शाकाहारी जन्तुओं से ऊर्जा भोजन के रूप में क्रमश: मांसाहारी और उच्च मांसाहारी जन्तुओं में जाती है। जब अपघटक इन जीवों के मृत शरीरों का अपघटन करते हैं तो यह ऊर्जा वातावरण में चली जाती है।

ऊर्जा का यह प्रवाह सदैव इसी क्रम में होता है। चूँकि जन्तु सूर्य से प्रत्यक्ष रूप से ऊर्जा प्राप्त नहीं कर सकता वह केवल पौधों में संचित भोजन के रूप में सौर ऊर्जा को ही ग्रहण कर सकता है। इसलिए हम कह सकते हैं कि जीवमण्डल में ऊर्जा का प्रवाह केवल एक ही दिशा में होता है।

प्रश्न 15.
पारितन्त्र एवं बायोम में अन्तर बताइए।
उत्तर:
पारितन्त्र एवं बायोम में प्रमुख अन्तर निम्नलिखित हैं-

पारितन्त्र	बायोम
1. सभी जीव अपने पर्यावरण में उपस्थित जैविक तथा अजैविक घटकों से क्रिया करते हैं। जीवों तथा उनके पर्यावरण में परस्पर सम्बन्धों के क्रियात्मक तन्त्र को पारितन्त्र (Ecosystem) कहते हैं। उदाहरण-एक जलाशय, झील या जंगल पारितन्त्र हैं।	1. एक बड़ा भौगोलिक क्षेत्र जिसमें कई पारितन्त्र होते हैं, एक बड़ी इकाई बनाता है, जिसे बायोम (Biome) कहते हैं अर्थात् बायोम एक विस्तृत क्षेत्र वाला पारितन्त्र है। उदाहरण-मरुस्थल, समुद्र।
2. पारितन्त्र को कृत्रिम रूप से भी विकसित किया जा सकता है। उदाहरण-एक्वेरियम व धान के खेत का पारितन्त्र।	2. बायोम पूर्ण रूप से प्राकृतिक होते हैं, इन्हें अलग से कृत्रिम रूप से विकसित नहीं किया जा सकता है।

प्रश्न 16.
“चील पर्यावरण का महत्त्वपूर्ण घटक है।” संक्षेप में समझाइये।
उत्तर:
चील पर्यावरण का एक महत्त्वपूर्ण घटक है। इसे निम्नलिखित आहार श्रृंखला द्वारा समझा जा सकता है-
हरे पौधे → चूहा → सौंप → चील
उपर्युक्त आहार श्रृंखला में चील सर्वोच्च मांसाहारी है जो साँप व चूहा का भक्षण करती है। यदि हम समस्त चीलों को हटा दें या मार डालें तब क्या होगा तब साँप की संख्या बढ़ जायेगी और उनको खाने वाला कोई नहीं रहेगा। साँप की संख्या इतनी बढ़ जायेगी कि वे समस्त चूहों को खा डालेगा तब एक ऐसी स्थिति आयेगी जब साँप अपने आप मरने लगेंगे क्योंकि उनको भोजन उपलब्ध नहीं होगा।

इस प्रकार चील किसी भी खाद्य श्रृंखला में हो उसे अथवा किसी भी एक जीव को हटाने पर पारिस्थितिक तन्त्र असन्तुलित हो जायेगा। इसी प्रकार चील मृत जीव-जन्तुओं को भी खाती है। इनकी अनुपस्थिति से पर्यावरण प्रदूषित हो सकता है। अत: चील पर्यावरण का महत्त्वपूर्ण घटक है।

प्रश्न 17.
निम्नलिखित में अन्तर स्पष्ट कीजिए-
(i) स्थलमण्डल तथा वायुमण्डल
(ii) पारिस्थितिकी तथा बायोम
(iii) आहार श्रृंखला तथा खाद्य जाल
(iv) मांसाहारी तथा सर्वभक्षी।
उत्तर:
(i) स्थलमण्डल तथा वायुमण्डल में अन्तर-स्थलमण्डल, भूमण्डल का वह भाग होता है जिसके अन्तर्गत सभी ठोस सम्मिलित किये जाते हैं। स्थलमण्डल उपापचयी क्रियाओं के लिए खनिज पदार्थों की पूर्ति करता है।

वायुमण्डल में वायु तथा समस्त गैसों का मिश्रण होता है। यह मण्डल अन्य दोनों मण्डलों (जलमण्डल एवं स्थलमण्डल) को ढके रहता है। यह मुख्यतः वायु में उपस्थित पोषकों के चक्र को पूरा करता है। इसमें गैसीय तत्त्व प्रचुर मात्रा में पाये जाते हैं।

(ii) पारिस्थितिकी अन्तर- पारिस्थितिकी में जीव-जन्तुओं का अध्ययन उनके पर्यावरण के साथ किया जाता है। किसी जीव की पारिस्थितिकी (Ecology) अध्ययन करने के लिए उसके पास के पर्यावरणीय कारकों भी अध्ययन करना आवश्यक होता है; जैसे- ताप, वर्षा, मृदा की रचना, वायु तीव्रता आदि। बायोम, किसी भौगोलिक क्षेत्र के समस्त पारितन्त्रों का सामूहक रूप है अर्थात् बायोम एक विस्तृत क्षेत्र वाला पारितन्त्र है। उदाहरण- मरुस्थल, समुद्र बायोम पूर्ण रूप से प्राकृतिक होते हैं, इन्हें कृत्रिम रूप से विकसित नहीं किया जा सकता।

(iii) आहार शृंखला तथा खाद्य जाल में अन्तर- किसी जीव द्वारा किसी अन्य जीव का उपभोग करने की क्रमबद्ध प्रक्रिया को खाद्य श्रृंखला या आहार श्रृंखला कहते हैं। इसमें जीव पोषण रीति के आधार पर किसी-न-किसी रूप में एक-दूसरे से जुड़े रहते हैं।

जीवमण्डल में अनेक आहार श्रृंखलाएँ आपस में अनेक प्रकार, आड़े-तिरछे जुड़कर एक जाल-सा बना लेती हैं, यह खाद्य जाल कहलाता है। खाद्य जाल का कोई एक जीव एक से अधिक खाद्य श्रृंखलाओं में स्थान ग्रहण कर सकता है।

(iv) मांसाहारी तथा सर्वभक्षी में अन्तर – जीवमण्डल में कुछ जन्तु भोजन के रूप में कुछ जन्तुओं को खा जाते हैं। ऐसे जन्तुओं को मांसाहारी कहा जाता है। ऐसे जन्तु मुख्यतः शाकाहारी जन्तुओं का भक्षण करते हैं; जैसे- शेर, चील, बाज आदि।

सर्वभक्षी वे प्राणी होते हैं जो मांसाहारी और शाकाहारी दोनों होते हैं। सर्वभक्षी प्राणी का सर्वोत्तम उदाहरण मानव है।

बहुविकल्पीय प्रश्न

1. प्रत्येक स्तर पर उपलब्ध ऊर्जा का कितने प्रतिशत उपभोक्ता के अगले स्तर एक पहुँचता है?
(a) 25%
(b) 10%
(c) 90%
(d) 15%
उत्तर:
(b) 10%

2. निम्नलिखित में से किस पोषी स्तर पर जीवों की संख्या अधिकतम होगी?
(a) प्राथमिक उपभोक्ता
(b) द्वितीयक उपभोक्ता
(c) उत्पादक
(d) शीर्षस्थ उपभोक्ता।
उत्तर:
(c) उत्पादक

9. पारितंत्र में ऊर्जा का प्रवाह होता है-
(a) एकदिशीय
(b) उत्क्रमणीय
(c) बहुमुखी
(d) कह नहीं सकते।
उत्तर:
(a) एकदिशीय

4. निम्नलिखित में से कौन-सी गैस सूर्य से आने वाली UV विकिरण से हमारी रक्षा करती है?
(a) O₂
(b) O₃
(c) CO₂
(d) CFCs
उत्तर:
(b) O₃

5. ओजोन परत को क्षति पहुँचाने वाले रसायन हैं-
(a) CO₂
(b) CO
(c) CFCs
(d) DDT
उत्तर:
(c) CFCs

6. पारितंत्र के शाखान्वित आहार सम्बन्ध क्या कहलाते हैं?
(a) आहार श्रृंखला
(b) आहार जाल
(c) जैव आवर्धन
(d) इनमें से कोई नहीं।
उत्तर:
(b) आहार जाल

7. निम्नलिखित में से किस कार्यक्रम के तहत CFC के उत्पादन को 1986 के स्तर तक सीमित करने पर सहमति हुई है?
(a) UNEP
(b) SBCO
(c) UNIEP
(d) WHO
उत्तर:
(a) UNEP

8. निम्नलिखित में से कौन-सा एक कृत्रिम पारितंत्र है?
(a) तालाब
(b) खेत
(c) झील
(d) वन
उत्तर:
(b) खेत

9. एक आहार श्रृंखला में, तीसरे पोषी स्तर पर हमेशा कौन होता है?
(a) मांसाहारी प्राणी
(b) शाकाहारी प्राणी
(c) अपघटक
(d) उत्पादक
उत्तर:
(a) मांसाहारी प्राणी

10. एक पारितंत्र में निम्नलिखित में से कौन शामिल होता है?
(a) सभी जीवधारी
(b) निर्जीव प्राणी
(c) जीवधारी और निर्जीव दोनों
(d) कभी जीवधारी और कभी निर्जीव वस्तुएँ
उत्तर:
(c) जीवधारी और निर्जीव दोनों

11. नीचे दी गई आहार श्रृंखला में, मान लीजिए कि चौथे पोषी स्तर पर ऊर्जा की मात्रा 5 kJ है, तो बताइए कि उत्पादक स्तर पर कितनी ऊर्जा उपलब्ध होगी?
घास → टिड्डा → मेंढक → साँप → बाज
(a) 5kJ
(b) 50 kJ
(c) 500kJ
(d) 5000kJ
उत्तर:
(d) 5000kJ

12. किसी आहार श्रृंखला में गैर-जैव निम्नीकरणीय पीड़कनाशियों का प्रत्येक उच्चतर पोषी स्तर पर बढ़ती हुई मात्रा में एकत्रित होते जाना क्या कहलाता है?
(a) सुपोषण
(b) प्रदूषण
(c) जैव आवर्धन
(d) एकत्रीकरण
उत्तर:
(c) जैव आवर्धन

13. ओजोन का अणुसूत्र है-
(a) O₃
(b) O₂
(c) O
(d) O₄
उत्तर:
(a) O₃

14. मानवों में UV किरणों से हो सकते हैं-
(a) प्रतिरक्षा तंत्र की क्षति
(b) फेफड़ों की क्षति
(c) त्वचा का कैंसर
(d) (a) तथा (c) दोनों
उत्तर:
(d) (a) तथा (c) दोनों

15. एक पारितंत्र में ऊर्जा का प्रवाह सदैव होता है-
(a) एकदिशिक
(b) द्विदिशिक
(c) बहुदिशिक
(d) किसी खास दिशा में नहीं
उत्तर:
(a) एकदिशिक

16. प्रकाश संश्लेषण की प्रक्रिया के लिए सभी हरे पौधों द्वारा अवशोषित सौर ऊर्जा की प्रतिशतता लगभग कितनी होती है?
(a) 1%
(b) 5%
(c) 8%
(d) 10%
उत्तर:
(a) 1%

17. यदि मेंढक टिड्डे को खा जाएँ, तो ऊर्जा स्थानांतरण किस दिशा में होगा?
(a) उत्पादक से अपघटक की दिशा में
(b) उत्पादक से प्राथमिक उपभोक्ता की दिशा में
(c) प्राथमिक उपभोक्ता से द्वितीयक उपभोक्ता की दिशा में
(d) द्वितीयक उपभोक्ता से प्राथमिक उपभोक्ता की दिशा में।
उत्तर:
(c) प्राथमिक उपभोक्ता से द्वितीयक उपभोक्ता की दिशा में

18. भोजन खाने के पश्चात् प्लास्टिक की जिन प्लेटों को फेंक दिया जाता है उन्हें दोबारा से उपयोग नहीं करना चाहिए, क्योंकि-
(a) ये हल्के पदार्थ की बनी होती हैं।
(b) ये आविषी पदार्थ (Toxic Materials) की बनी होती हैं।
(c) ये जैवनिम्नीकरणीय पदार्थों की बनी होती हैं।
(d) ये गैर-जैवनिम्नीकरणीय पदार्थों की बनी होती हैं।
उत्तर:
(d) ये गैर-जैवनिम्नीकरणीय पदार्थों की बनी होती हैं।

19. निम्नलिखित कथनों में से कौन-सा कथन सही नहीं है?
(a) सभी हरे पौधे और नीले-हरे शैवाल उत्पादक होते हैं।
(b) हरे पौधे अपना भोजन कार्बनिक यौगिकों से प्राप्त करते हैं।
(c) उत्पादक स्वयं अपना भोजन अकार्बनिक यौगिकों से तैयार करते हैं।
(d) पौधे सौर ऊर्जा को रासायनिक ऊर्जा में बदल देते हैं।
उत्तर:
(b) हरे पौधे अपना भोजन कार्बनिक यौगिकों से प्राप्त करते हैं।

20. निम्नलिखित में से कौन अजैव निम्नीकरणीय है?
(a) कागज
(b) लकड़ी
(c) कपड़ा
(d) प्लास्टिक।
उत्तर:
(d) प्लास्टिक।

21. अजैव निम्नीकरणीय कृषि रसायनों को आहार श्रृंखला में प्रवेश व उत्तरोत्तर संग्रहण क्या कहलाता है?
(a) जैविक आवर्धन
(b) सुपोषण
(c) अंगीकरण
(d) संश्लेषण।
उत्तर:
(a) जैविक आवर्धन

रिक्त स्थानों की पूर्ति कीजिए-

1. जब हम एक पोषी स्तर से दूसरे पोषी स्तर पर जाते हैं तो ऊर्जा का …………………… होता है, यह आहार श्रृंखला में पोषी स्तरों को सीमित कर देता है।
2. मानव की गतिविधियों का पर्यावरण पर …………………… होता है।
3. …………………… जैसे रसायनों ने ओजोन परत को नुकसान पहुँचाया है क्योंकि ओजोन परत सूर्य से आने वाली …………………… विकिरण से सुरक्षा प्रदान करती है अतः इसकी क्षति से पर्यावरण को नुकसान पहुँच सकता है।
4. हमारे द्वारा उत्पादित कचरा …………………… निम्नीकरणीय अथवा …………………… निम्नीकरणीय हो सकता है।
5. DDT एक …………………… है, जो हमारी …………………… में शामिल होकर …………………… होता रहता है।

उत्तर:

1. ह्यस
2. समाघात
3. CFCs, पराबैंगनी
4. जैव, अजैव
5. कीटनाशक, आहार श्रृंखला, जैव आवर्धित

Jharkhand Board JAC Class 10 Maths Important Questions Chapter 9 त्रिकोणमिति का अनुप्रयोग Important Questions and Answers.

JAC Board Class 10th Maths Important Questions Chapter 9 त्रिकोणमिति का अनुप्रयोग

लघुत्तरात्मक / निबन्धात्मक प्रश्न :

प्रश्न 1.
क्षैतिज तल पर किसी निश्चित बिन्दु से एक मीनार के शिखर का उन्नयन कोण 30° है। यदि प्रेक्षक 20 मीटर मीनार की ओर चलता है, तो मीनार के शिखर का उन्नयन कोण 15° बढ़ जाता है। मीनार की ऊँचाई ज्ञात करो ।
हल:
माना AB एक मीनार है जिसकी ऊँचाई h मीटर है। बिन्दु C से मीनार के शिखर का उन्नयन कोण 30° है। बिन्दु C से 20 मीटर मीनार की तरफ चलने पर मीनार के शिखर का उन्नयन कोण 15° बढ़ जाता है।
अर्थात् ∠ACB = 30° तथा ∠ADB = 45°

समकोण ΔABD में,
tan 45° = \(\frac{A B}{B D}\)
⇒ 1 = \(\frac{h}{B D}\)
⇒ BD = h मी. …..(1)
समकोण ΔABC में tan 30° = \(\frac{A B}{B C}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{h}{20+B D}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{h}{20+h}\)
[ समी. (1) से BD = h]
20 + h = \(\sqrt{3}\)h
20 = \(\sqrt{3}\)h – h
20 = h(\(\sqrt{3}\) – 1)
h = \(\frac{20}{\sqrt{3}-1}\)
h = \(\frac{20(\sqrt{3}+1)}{(\sqrt{3}-1)(\sqrt{3}+1)}\)
h = \(\frac{20(\sqrt{3}+1)}{3-1}\)
h = 10(\(\sqrt{3}\) + 1) मीटर
अतः मीनार की ऊँचाई = 10 (\(\sqrt{3}\) + 1) मीटर ।

प्रश्न 2.
एक झील में पानी के तल से 20 मीटर ऊंचे बिन्दु A से, एक बादल का उन्नयन कोण 30° है। झील में बादल के प्रतिबिम्ब का A से अवनमन कोण 60° हैं। A से बादल की दूरी ज्ञात कीजिए।
हल:
माना कि BD पानी का तल है। B से 20 मीटर ऊँचे बिन्दु A से एक बादल (P) का उन्नयन कोण 30° है। माना झील में बादल के प्रतिबिम्ब की स्थिति C है तथा प्रतिबिम्ब इस प्रकार है कि झील में बादल के प्रतिबिम्ब का अवनमन कोण 60° है। माना कि PQ = h मी है।

अतः ∠PAQ = 30° तथा ∠QAC = 60°
QD = AB = 20 मी
CD = PD = (20 + h) मी
QC = 20 + h + 20
= (40 + h) मी
BD = AQ
समकोण ΔPAQ में
tan 30° = \(\frac{P Q}{A Q}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{h}{A Q}\)
⇒ AQ = h\(\sqrt{3}\) मीटर …..(1)
समकोण ΔAQC में
tan 60° = \(\frac{Q C}{A Q}\)
⇒ \(\sqrt{3}=\frac{40+h}{h \sqrt{3}}\)
[समीकरण (1) का प्रयोग करने पर]
⇒ 3h = 40 + h
⇒ 3h – h=40
⇒ 2h = 40
⇒ h = \(\frac{40}{2}\) = 20 मी
पुन: समकोण ΔPAQ में,
sin 30° = \(\frac{P Q}{A P}\)
⇒ \(\frac{1}{2}=\frac{h}{A Q}\)
⇒ \(\frac{1}{2}=\frac{20}{A Q}\) मीटर
AQ = 20 × 2
अतः A से बादल की दूरी = 40 मीटर ।

प्रश्न 3.
4000 मीटर की ऊँचाई पर उड़ते हुए वायुयान के ठीक नीचे जिस क्षण दूसरा वायुयान आता है, उसी क्षण क्षैतिज तल पर किसी बिन्दु से इन वायुयानों के उन्नयन कोण क्रमश: 60° और 45° हैं। उस क्षण पर दोनों वायुयानों के बीच की ऊर्ध्वाधर दूरी ज्ञात कीजिए।
हल:
माना दो वायुयान A और B हैं जिनके बीच की ऊर्ध्वाधर दूरी AB = h मीटर
क्षैतिज तल पर स्थित बिन्दु D से इन वायुयानों A और B के उन्नयन कोण क्रमश: 60° और 450 हैं।
अर्थात् ∠ADC = 60° तथा ∠BDC = 45°
AC = 4000 मीटर
BC = AC – AB = (4000 – h) मीटर
समकोण ΔBCD में,
tan 45° = \(\frac{B C}{C D}\)

1 = \(\frac{4000-h}{x}\)
x = 4000 – h …..(i)
पुन: समकोण ΔACD में,
tan 60° = \(\frac{A C}{C D}\)
\(\sqrt{3}\) = \(\frac{4000}{x}\)
⇒ x\(\sqrt{3}\) = 4000
⇒ x = \(\frac{4000}{\sqrt{3}}\) …..(ii)
समीकरण (i) से x का मान रखने पर,
4000 – h = \(\frac{4000}{\sqrt{3}}\)
⇒ h = 4000 – \(\frac{4000}{\sqrt{3}}\)
⇒ h = 4000\(\left(1-\frac{1}{\sqrt{3}}\right)\)
⇒ h = 4000\(\left(\frac{\sqrt{3}-1}{\sqrt{3}}\right)\)
⇒ h = 4000\(\frac{4000 \times 0.732}{1.732}\)
⇒ h = \(\frac{2928}{1.732}\) = 1690.53 मीटर
अतः दोनों वायुयानों के बीच ऊर्ध्वाधर दूरी 1690.53 मीटर होगी।

प्रश्न 4.
धरातल के एक बिन्दु से एक हवाई जहाज का उन्नयन कोण 60° है। 15 सेकण्ड की उड़ान के पश्चात्, उन्नयन कोण 30° का हो जाता है। यदि हवाई जहाज एक निश्चित ऊँचाई 1500\(\sqrt{3}\) मीटर पर उड़ रहा हो, तो हवाई-जहाज की गति किमी / घंटा में ज्ञात कीजिए ।
हल:
माना P और Q हवाई जहाज की दो स्थितियाँ है। माना ABC एक क्षैतिज रेखा है जो A से जाती है।
दिया है कि स्थिति P और Q से, A बिन्दु से हवाई जहाज द्वारा बने उन्नयन कोण 30° तथा 60° है।
∴ ∠PAB = 60° और ∠QAC = 30°
इसी तरह, PB = QC = 1500\(\sqrt{3}\) मीटर

ΔABP में,
tan 60° = \(\frac{B P}{A B}\)
\(\sqrt{3}\) = \(\frac{1500 \sqrt{3}}{A B}\)
AB = 1500 मीटर
ΔACQ में,
tan 30° = \(\frac{Q C}{A C}\)
\(\frac{1}{\sqrt{3}}=\frac{1500 \sqrt{3}}{A C}\)
⇒ AC = 1500 × 3 = 4500 मीटर
∴ BC = AC – AB = 4500 – 1500
= 3000 मीटर
इस प्रकार हवाई जहाज 15 सेकण्ड में 3000 मीटर की दूरी तय करता है।
∴ हवाई जहाज की चाल = \(\frac{3000}{15}\) = 200 मी/से
= \(\frac{200 \times 60 \times 60}{1000}\)
= 720 किमी/ घण्टा
अतः हवाई जहाज की चाल 720 किमी/घण्टा है।

प्रश्न 5.
10 मीटर ऊँचे भवन के शिखर से एक टॉवर के शिखर का उन्नयन कोण 60° है और इसके पाद का अवनमन कोण 45° है। टावर की ऊँचाई ज्ञात कीजिए।
हल:
माना AB एक टॉवर है उसी धरातल में एक भवन CD है जिसकी ऊँचाई 10 मीटर है।
टॉवर के शिखर का उन्नयन कोण और पाद का अवनमन कोण क्रमश: 60° और 45° है।
अर्थात् ∠ACE = 60°
और ∠ECB = 45°
BD || CE, CD || BE
CD = BE = 10 मी.
अब समकोण त्रिभुज CBD में,
tan 45° = \(\frac{C D}{D B}\)
\(1=\frac{10}{D B}\)
DB = 10 मी.
CE = DB = 10 मी.
पुनः समकोण त्रिभुज AEC में,

tan 60° = \(\frac{A E}{C E}=\frac{A E}{10}\)
AE = 10\(\sqrt{3}\) मी
अतः टॉवर AB की ऊँचाई
= AE + EB = 10\(\sqrt{3}\) + 10
= 10 (\(\sqrt{3}\) + 1) मीटर
अतः टॉवर AB की ऊँचाई = 10(\(\sqrt{3}\) + 1) मीटर

प्रश्न 6.
एक नदी के पुल के एक बिन्दु से नदी के सम्मुख किनारों के अवनमन कोण क्रमश: 30° और 45° है। यदि पुल किनारों से 4 मी की ऊँचाई पर है तो नदी की चौड़ाई ज्ञात कीजिए।
हल:
दिया है, नदी से पुल की ऊँचाई
AC = 4 मी
BC = x, CD = y

∴ समकोण ΔABC में,
\(\frac{A C}{B C}\) = tan 45°
⇒ \(\frac{4}{x}\) = 1 ⇒ x = 4 मी
पुन: समकोण ΔACD में,
\(\frac{A C}{C D}\) = tan 30°
⇒ \(\frac{4}{y}=\frac{1}{\sqrt{3}}\)
y = 4\(\sqrt{3}\) मी …..(ii)
समी. (i) व (ii) से,
∴ नदी की चौड़ाई (x + y)
= 4\(\sqrt{3}\) + 4
= 4(\(\sqrt{3}\) + 1)
= 10.92 मी ।

प्रश्न 7.
एक मीनार के पाद से एक भवन के शिखर का उन्नयन कोण 30° है और भवन के पाद से मीनार के शिखर का उन्नयन कोण 60° है। यदि मीनार के ऊँचाई 48 मी है तो भवन की ऊंचाई ज्ञात कीजिए।
हल:
माना AB एक मीनार है जिसके ऊँचाई 48 मी है तथा CD एक भवन है जिसकी ऊँचाई h मी है। दिया है, मीनार के पाद से भवन के शिखर का उन्नयन कोण 30° तथा भवन के पाद से मीनार के शिखर का उन्नयन कोण 60° है।
अर्थात् ∠CBD = 30°
तथा ∠ADB = 60°

समकोण ΔCDB में,
tan 30° = \(\frac{C D}{B D}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{h}{B D}\)
⇒ BD = h\(\sqrt{3}\) मी
पुन: समकोण ΔABD में,
tan 60° = \(\frac{A B}{B D}\)
\(\sqrt{3}\) = \(\frac{48}{h \sqrt{3}}\)
h = \(\frac{48}{\sqrt{3} \times \sqrt{3}}=\frac{48}{3}\)
= 16 मी
अतः भवन की ऊँचाई 16 मी है।

प्रश्न 8.
आँधी आने पर एक पेड़ टूट जाता है और टूटा हुआ भाग इस तरह मुड़ जाता है कि पेड़ का शिखर जमीन को छूने लगता है और इसके साथ 60° का कोण बनाता है। पेड़ के पाद- बिंदु की दूरी जहाँ पेड़ का शिखर जमीन को छूता है, 3 मी है। पेड़ की ऊंचाई ज्ञात कीजिए।
हल:
माना आँधी से पहले पेड़ की लम्बाई BD है। आँधी के बाद पेड़ A स्थान से टूटकर पेड़ का शिखर जमीन पर C बिन्दु पर पड़ता है। टूटा हुआ भाग जमीन से 30° का कोण बनाता है।

∴ ∠ACB = 30°
समकोण ΔABC में,
tan 30° = \(\frac{A B}{B C}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{A B}{3}\)
⇒ AB = \(\frac{3}{\sqrt{3}}\) मी
पुन: समकोण ΔABC में,
cos 30° = \(\frac{B C}{A C}\)
⇒ \(\frac{\sqrt{3}}{2}=\frac{3}{A C}\)
⇒ AC = \(\frac{6}{\sqrt{3}}\) मी
पेड़ की कुल ऊँचाई = BD
= AB + AD
= AB + AC [∵ AD = AC]
= \(\left(\frac{3}{\sqrt{3}}+\frac{6}{\sqrt{3}}\right)\) मी
= \(\frac{9}{\sqrt{3}}\) मी = \(\frac{9 \sqrt{3}}{\sqrt{3} \times \sqrt{3}}\)
= \(\frac{9 \sqrt{3}}{3}\) मी
= 3\(\sqrt{3}\) मी

प्रश्न 9.
अमित जो कि समतल जमीन पर खड़ा है, अपने से 200 मी दूर उड़ते हुए पक्षी का उन्नयन कोण 30° पाता है। दीपक जो कि 50 मी ऊँचे भवन की छत पर खड़ा है, उसी पक्षी का उन्नयन कोण 45° पाता है। अमित और दीपक पक्षी के विपरीत दिशा में हैं। दीपक से पक्षी की दूरी ज्ञात कीजिए।
हल:
माना अमित बिन्दु C पर खड़ा है तथा AB चिड़िया की धरातल पर स्थित बिन्दु B से ऊँचाई है तथा माना दीपक बिन्दु D पर स्थित है, जहाँ DE भवन की ऊँचाई है।
दिया है, ∠ACB = 30°, ∠ADF = 45° तथा DE = 50 मी

अब, समकोण ΔABC में,
sin 30° = लम्ब / कर्ण
= \(\frac{A B}{A C}\)
\(\frac{1}{2}=\frac{A B}{200}\)
AB = 100 मी
समकोण ΔAFD में,
sin 45° = लम्ब / कर्ण
= \(\frac{A F}{A D}\)
(AB = AF + BF
100 = AF + 50
AF = 50 मी)
= \(\frac{1}{\sqrt{2}}=\frac{50}{A D}\)
AD = 50\(\sqrt{2}\) मी
अतः दीपक की चिड़िया से दूरी 50\(\sqrt{2}\) मी है।

प्रश्न 10.
एक ऊर्ध्वार मीनार क्षैतिज तल पर खड़ी है तथा उसके ऊपर एक 6 मी ऊँचा झंडा लगा है। तल के किसी बिन्दु से झंडे के पाद तथा शिखर के उन्नयन कोण क्रमश: 30° तथा 45° है। मीनार की ऊँचाई ज्ञात कीजिए। (\(\sqrt{3}\) = 1.732 लीजिए)
हल:
माना AB एक मीनार है तथा BC झंडा है। अब माना कि P भूमि पर एक ऐसा बिन्दु है, जिसका मीनार के शिखर का उन्नयन कोण ∠APB = 30° तथा मीनार पर स्थित झंडा का उन्नयन कोण ∠APC = 45° है तथा BC = 6 मी अब माना AB = h मी तथा PA = x मी

समकोण ΔPAB से,
cos 30° = \(\frac{P A}{P B}=\frac{x}{h}\)
\(\sqrt{3}=\frac{x}{h}\) (∵ cot 30° = \(\sqrt{3}\))
x = \(\sqrt{3}\)h …..(i)
समकोण ΔPAC से,
cot 45° = \(\frac{x}{h+6}=\frac{P A}{A C}\) (∵ cot 45° = 1)
x = h + 6 ….. (ii)
समीकरण (i) व (ii) से,
\(\sqrt{3}\)h = h + 6
\(\sqrt{3}\)h – h = 6
= \(\frac{6}{\sqrt{3}-1}\)
= \(\frac{6}{1.732-1}\)
= \(\frac{6}{0.732}\)
= \(\frac{6000}{732}\)
= 8.19 मी
अतः मीनार की ऊँचाई 8.19 मी है।

प्रश्न 11.
100 मी ऊंचे एक लाइट हाउस से दूर एक नाव को ले जाता हुआ व्यक्ति 2 मिनट में लाइट हाउस में शिखर का उन्नयन कोण को 60° से 30° बढ़लता हुआ पाता है। मीटर प्रति मिनट में नाव की चाल ज्ञात कीजिए। (\(\sqrt{3}\) = 1.732 लीजिए)
हल:
माना AB एक 100 मी ऊँचाई का लाइट हाउस है प्रारम्भ में व्यक्ति C बिन्दु पर था तथा 2 मिनट बाद D बिन्दु पर आता है।

यहाँ, ∠ACB = 60° तथा ∠ADB = 30° है। माना BC = x मी तथा BD = y मी है।
समकोण ΔABC में,
tan 60° = \(\frac{A B}{B C}\)
\(\sqrt{3}=\frac{100}{x}\)
x = \(\frac{100}{\sqrt{3}}\) ……..(i)
पुन: समकोण ΔABD में,
tan 30° = \(\frac{A B}{B D}\)
\(\frac{1}{\sqrt{3}}=\frac{100}{B D}\)
BD = 100\(\sqrt{3}\)
BC + CD = 100\(\sqrt{3}\)
x + y = 100\(\sqrt{3}\) …..(ii)
समीकरण (i) से, x = \(\frac{100}{\sqrt{3}}\) समीकरण (ii) में रखने पर,
\(\frac{100}{\sqrt{3}}\) + y = 100\(\sqrt{3}\)
y = \(100 \sqrt{3}-\frac{100}{\sqrt{3}}\)
= \(\frac{300-100}{\sqrt{3}}\)
y = \(\frac{200}{\sqrt{3}}\) मी
बिन्दु C से D तक जाने मैं नाव द्वारा लगा समय 2 मिनट है।
तथा CD = \(\frac{200}{\sqrt{3}}\)
अतः नाव को चाल = समय / दूरी
\(\frac{C D}{2}\) ⇒ \(\frac{y}{2}\)
= \(=\frac{200}{\sqrt{3} \times 2}\) (∵ y = \(\frac{200}{\sqrt{3}}\) मी)
= \(\frac{100}{\sqrt{3}}=\frac{100}{1.732}\)
= 57.73 मीटर / मिनट

प्रश्न 12.
एक मीनार के पाद से गुजरने वाली सीधी रेखा पर पाद से क्रमशः 4 मी तथा 16 मी की दूरियों पर दो बिंदु C व D स्थित हैं। यदि C व D से मीनार के शिखर के उन्नयन कोण एक-दूसरे के पूरक हों, तो मीनार की ऊँचाई ज्ञात कीजिए।
हल:
माना मीनार की ऊँचाई = h मीटर
ΔABC में, \(\frac{A B}{B C}\) = tan(90° – θ)
\(\frac{h}{4}\) = cot θ …..(i)

ΔABD में,
\(\frac{A B}{B D}\) = tan θ
\(\frac{h}{16}\) = tan θ …..(ii)
समीकरण (i) और (ii) का गुणा करने पर,
\(\frac{h}{4} \times \frac{h}{16}\) = cot θ × tan θ
\(\frac{h^2}{64}=1\)
[∵ cot θ × tan θ = \(\frac{1}{\tan \theta}\) × tan θ = 1]
⇒ h² = 64
⇒ h = 8 मी
अतः मीनार की ऊँचाई 8 मीटर है।

प्रश्न 13.
एक हवाई जहाज भूतल से ऊपर 300 मी की ऊँचाई पर उड़ रहा है। इस ऊँचाई पर उड़ते हुए हवाई जहाज से एक नदी के दोनों किनारों पर परस्पर विपरीत दिशाओं में स्थित दो बिंदुओं के अवनमन कोण क्रमशः 45° तथा 60° हैं। नदी की चौड़ाई ज्ञात कीजिए। [\(\sqrt{3}\) = 1.732 प्रयोग कीजिए]
हल:
माना हवाई जहाज A बिंदु पर नदी से 300 मीटर ऊँचाई पर है। C व D नहीं के विपरीत किनारों पर है।

समकोण ΔABC में,
\(\frac{B C}{A B}\) = cot 60°
⇒ \(\frac{x}{300}=\frac{1}{\sqrt{3}}\)
⇒ x = \(\frac{300}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}}\)
= 100\(\sqrt{3}\) मी
= 100 × 1.732 = 173.2 मी
समकोण ΔABD में,
⇒ \(\frac{B D}{A B}\) = cot 45°
⇒ \(\frac{y}{300}\) = 1
⇒ y = 300
नदी को चौड़ाई = x + y
= 173.2 + 300
= 473.2 मी

प्रश्न 14.
समुद्र तल से 75 मी. ऊँचे लाइट हाउस के शिखर से देखने पर दो समुद्री जहाजों के अवनमन कोण 30° तथा 45° है। यदि दोनों जहाज लाइट हाउस की विपरीत दिशाओं में हो, तो दोनों जहाजों के बीच की दूरी ज्ञात कीजिए।
हल:
माना AB लाइट हाउस है।
जहाज क्रमश: बिन्दु C व D पर है।

समकोण ΔABC में,
⇒ \(\frac{A B}{B C}\) = tan 30°
⇒ \(\frac{75}{x}=\frac{1}{\sqrt{3}}\)
⇒ x = 75\(\sqrt{3}\) मी
समकोण ΔABD में,
⇒ \(\frac{A B}{B D}\) = tan 30°
⇒ \(\frac{75}{y}\) = 1
y = 75 मी
जहाजों के बीच की दूरी = x + y
= (75\(\sqrt{3}\) + 75) मी
= 75(\(\sqrt{3}\) + 1) मी

प्रश्न 15.
एक झील के पानी की सतह में 60 मी ऊँचाई पर स्थित एक बिन्दु से बादल का उन्नयन कोण 30° है, तथा झील के पानी में बादल का परछाई का अवनमन कोण 60° है। बादल की झील के पानी की सतह से ऊँचाई प्राप्त कीजिए।
हल:
ΔCMP में,
tan 30° = \(\frac{C M}{P M}\)
\(\frac{1}{\sqrt{3}}=\frac{h}{P M}\) या PM = \(\sqrt{3}\)h …….(i)

ΔPMC में,
tan 60° = \(\frac{C M}{P M}\)
= \(\frac{h+60+60}{P M}=\sqrt{3}\)
या PM = \(\frac{h+120}{\sqrt{3}}\) …..(ii)
समीकरण (i) और (ii) से,
\(\sqrt{3}\)h = \(\frac{h-120}{\sqrt{3}}\)
3h = h + 120
2h = 120 ⇒ h = 60 मी
पानी के तल से बादल की ऊँचाई = h + 60
= 60 + 60 = 120 मी

प्रश्न 16.
एक मीनार के एक ही ओर तथा इसके आध र से एक ही सरल रेखा में दो बिंदु A तथा B हैं। मीनार के शिखर से इन बिंदुओं अवनमन कोण क्रमश: 60° व 45° हैं। यदि मीनार की ऊँचाई 15 मी हो, तो इन बिंदुओं के बीच की दूरी ज्ञात कीजिए।
हल:
माना PT एक मीनार है।

समकोण ΔPTA में,
tan 60° = \(\frac{P T}{T A}\)
⇒ \(\sqrt{3}=\frac{15}{T A}\)
⇒ TA = \(\frac{15}{\sqrt{3}}\)
पुन: समकोण ΔPTB में,
tan 45° = \(\frac{P T}{T B}\)
⇒ 1 = \(\frac{15}{T B}\)
⇒ TB = 15 मी
बिन्दुओं A व B के बीच की दूरी
AB = TB – TA
= 15 – \(\frac{15}{\sqrt{3}}\) = 15\(\left(\frac{\sqrt{3}-1}{\sqrt{3}}\right)\) मी

प्रश्न 17.
एक नदी के एक किनारें पर खड़ा एक व्यक्ति, नदी के दूसरे किनारे पर खड़े एक वृक्ष के शिखर का उन्नयन कोण 60° पाता है जब वह किनारे से 30 मी दूर जाता है, तो वह उन्नयन कोण 30° पाता है। वृक्ष की ऊँचाई तथा नदी की चौड़ाई ज्ञात कीजिए। [\(\sqrt{3}\) = 1.732 प्रयोग कीजिए]
हल:
माना नदी के एक किनारे पर एक वृक्ष AB है तथा नदी के दूसरे किनारे पर व्यक्ति P बिंदु पर है। यहाँ AP नदी की चौड़ाई है।
जब व्यक्ति P से बिंदु M पर जाता है, तो उन्नयन कोण 60° से 30° हो जाता है।

समकोण ΔPAB में,
⇒ tan 60° = \(\frac{A B}{P A}\)
⇒ \(\sqrt{3}\) = \(\frac{A B}{P A}\)
⇒ AB = \(\sqrt{3}\)PA …..(i)
पुन: समकोण ΔMAB में,
tan 30° = \(\frac{A B}{M A}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{A B}{30+A P}\)
⇒ 30 + AP = \(\sqrt{3}\)AB
⇒ 30 + AP = \(\sqrt{3}\)(\(\sqrt{3}\)AP) [समी (i) से]
⇒ 30 + AP = 3AP
⇒ 2AP = 30
⇒ AP = 15 मी.
समीकरण (i) से,
AB = \(\sqrt{3}\) × 15 = 15\(\sqrt{3}\) मी
अतः नदी की चौड़ाई = 15 मी
तथा पेड़ की ऊँचाई = 15\(\sqrt{3}\) मी

प्रश्न 18.
भूमि पर स्थित बिंदु A से एक हवाई जहाज का उन्नयन कोण 60° है। 10 सैकंड की उड़ान के बाद उसी ऊँचाई पर उड़ते हुए हवाई जहाज का उन्नयन कोण बिंदु A से 30° हो जाता है। यदि हवाई जाहज की औसत चाल 720 किमी / घंटा हो, तो हवाई जाहज की धरती से स्थिर ऊँचाई ज्ञात कीजिए।
हल:
माना P और Q हवाई जहाज की दो स्थितियाँ है। माना ABC एक क्षैतिज रेखा है जो A से जाती है।
∵ हवाई जाहज द्वारा 1 घंटे में तय दूरी,
PQ = 720 किमी

∵ हवाई जहाज द्वारा 1 सकण्ड में तय दूरी
= \(\frac{720 \times 1000}{60 \times 60}\) मी
= 200 मी
और 10 सेकंड में तय दूरी = 10 × 200 मी = 2000 मी
∴ PQ = 2000 मी
समकोण ΔABP में
tan 60° = \(\frac{P B}{A B}\)
⇒ \(\sqrt{3}=\frac{P B}{A B}\)
⇒ PB = \(\sqrt{3}\)AB …..(i)
⇒ tan 30° = \(\frac{Q C}{A C}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{Q C}{A C}\)
⇒ AC = \(\sqrt{3}\)QC
⇒ AB + BC = \(\sqrt{3}\)PB [∵ QC = PB]
⇒ AB + 200 = \(\sqrt{3}\) × \(\sqrt{3}\)AB
[∵ PQ = 2000
[तथा PB = \(\sqrt{3}\)AB]
⇒ AB + 2000 = 3AB
⇒ 2AB = 2000
⇒ AB = 1000 मी
समीकरण (i) से
PB = \(\sqrt{3}\) × 1000
= 1000\(\sqrt{3}\) मी
अतः हवाई जहाज की धरती से स्थिर ऊँचाई 1000\(\sqrt{3}\) मी है।

वस्तुनिष्ठ प्रश्न :

रिक्त स्थानों की पूर्ति कीजिए :

प्रश्न (क).

1. यदि कोई प्रेक्षक किसी वस्तु को देख रहा है, तो प्रेक्षक की आँख को उस वस्तु से मिलाने वाली क्षैतिज रेखा को ……………. रेखा कहते हैं।
2. वह रेखा, जो प्रेक्षक की आँख से सीधी भूमि के समांतर जाती है, ……………… रेखा कहलाती है।
3. जब प्रेक्षक किसी वस्तु को देखने के लिए अपने सिर को ऊपर उठाता है, तो वस्तु प्रेक्षक की आँख पर ………………. कोण बनाती है।
4. जब प्रेक्षक किसी वस्तु को देखने के लिए अपना सिर नीचे झुकता है, तो वस्तु की आँख पर कोण ……………… बनाती है।
5. उन्नयन कोण एवं अवनमन कोण सदैव बराबर और ……………… कोण होते हैं।

उत्तर:

1. दृष्टि,
2. क्षैतिज,
3. उन्नयन,
4. अवनमन,
5. न्यून ।

निम्न में सत्य / असत्य ज्ञात कीजिए :

प्रश्न (ख).

1. अवनमन कोण को अवनति कोण भी कहते हैं।
2. उन्नयन कोण एवं अवनमन कोण एकांतर कोण होते हैं।
3. उन्नयन कोण सदैव अधिक कोण होता है।
4. अवनमन कोण सदैव समकोण होता है।
5. त्रिकोणमिति की सहायता से दूरियों तथा ऊँचाइयों की गणना सरलता से की जा सकती है।

उत्तर:

1. सत्य,
2. सत्य,
3. असत्य,
4. असत्य,
5. सत्य ।

(ग) बहुविकल्पीय प्रश्न :

प्रश्न 1.
एक सीधी खड़ी छड़ की लंबाई तथा उसकी परछाई में 1 : \(\sqrt{3}\) का अनुपात है। उस समय सूर्य का उन्नयन कोण ज्ञात कीजिए:
(A) 30°
(B) 60°
(C) 45°
(D) 90°
हल:
माना छड़ की लंबाई AB तथ उसकी परछाई BC है।
माना उन्नयन कोण θ है।
दिया है, BA : BC = 1 : \(\sqrt{3}\)
⇒ \(\frac{B A}{B C}=\frac{1}{\sqrt{3}}\)

समकोण ΔCAB में
sin θ = \(\frac{A B}{B C}\)
⇒ sin θ = \(\frac{1}{\sqrt{3}}\)
⇒ sin θ = sin 60°
⇒ θ = 60°
अत: सही विकल्प (B) है।

प्रश्न 2.
निम्न आकृति में वस्तु 1 को बिंदुओं O₁ तथा O₂ से देखने पर बने अवनमन कोण क्रमश: हैं:
(A) 45°, 75°
(B) 60°, 90°
(C) 30°, 60°
(D) 45°, 30°
हल:
एक रेखा PO₁ इस प्रकार खींची कि PO₁ || AC
यहाँ ∠PO₁A + ∠AO₁C = 90°
⇒ ∠PO₁A + 60° = 90°

⇒ ∠PO₁A = 90° – 60° = 30°
अब ∠PO₂A = ∠O₂AB = 45° (एकान्तर कोण)
O₁ से अवनमन कोण = 30°
O₂ से अवनमन कोण = 45°
अत: सही विकल्प (D) है।

प्रश्न 3.
निम्न आकृति में अच्छी तरह से तनी हुई एक 20 मी लम्बी रस्सी भूमि पर सीधे लगे खंभे के शिखर से बंधी है। यदि भूमि स्तर के साथ रस्सी द्वारा बनाया गया कोण 30° का है, तो खंभे की ऊँचाई ज्ञात कीजिए।
(A) 10 मी
(B) 20 मी
(C) 40 मी
(D) 50 मी
हल:
समकोण ΔBAC में.

sin 30° = \(\frac{A B}{B C}\)
⇒ \(\frac{1}{2}=\frac{A B}{20}\)
⇒ \(\frac{20}{2}\) मी = 10 मी
अत: सही विकल्प (A) है।

प्रश्न 4.
निम्न आकृति में, भूमि के एक बिन्दु C से, जो मीनार के पाद बिन्दु से 60 मी की दूरी पर है, मीनार AB के शिखर का उन्नयन कोण 30° है। मीनार की ऊँचाई है :
(A) 60\(\sqrt{3}\) मी.
(B) 60 मी
(C) 20\(\sqrt{3}\) मी
(D) 20 मी
हल:
समकोण ΔABC में,

tan 30° = \(\frac{A B}{B C}\)
\(\frac{1}{\sqrt{3}}=\frac{A B}{60}\)
AB = \(\frac{60}{\sqrt{3}}=\frac{60 \sqrt{3}}{\sqrt{3} \times \sqrt{3}}\)
\(\frac{60 \sqrt{3}}{3}\) = 20\(\sqrt{3}\) मी
अत: सही विकल्प (C) है।

प्रश्न 5.
एक मीनार के आधार से 100 मीटर की दूरी पर स्थित बिन्दु से उसके शिखर का उन्नयन कोण 45° है। मीनार की ऊँचाई है:
(A) 50 मीटर
(B) 100 मीटर
(C) \(\frac{100}{\sqrt{2}}\) मीटर
(D) \(\frac{100 \times \sqrt{3}}{2}\) मीटर
हल:
माना मीनार की ऊँचाई (BC)h मीटर है।
मीनार के आधार से 100 मीटर दूरी पर स्थित बिन्दु उसके शिखर का उन्नयन कोण 45° है। अर्थात् AB = 100 मी. तथा ∠CAB = 45°
समकोण ΔABC में,

tan 45° = \(\frac{B C}{A B}\)
⇒ 1 = \(\frac{h}{100}\)
∴ h = 100 मीटर
अतः मीनार की ऊँचाई = 100 मी.
सही विकल्प (B) है।

प्रश्न 6.
15 मी लम्बी एक सीढ़ी एक ऊर्ध्वाधर दीवार के शिखर तक पहुँचती है। यदि यह सीढ़ी दीवार के साथ 60° का कोण बनाती है, तो दीवार की ऊँचाई है :
(A) 15\(\sqrt{3}\) मी.
(B) \(\frac{15 \sqrt{3}}{2}\) मी.
(C) \(\frac{15}{2}\) मी.
(D) 15 मी.
हल:
माना कि AB एक ऊर्ध्वाधर दीवार है जिसकी ऊँचाई 1⁄2 मी. है। माना कि AC एक सीढ़ी है जो दीवार के शिखर तक पहुँचती है तथा सीढ़ी की लम्बाई 15 मी. है। सीढ़ी दीवार के साथ 60° का कोण बनाती है, तब
∠ACB = 60° तथा AC = 15 मी.
समकोण ΔABC में,

sin 60° = \(\frac{A B}{A C}\)
\(\frac{\sqrt{3}}{2}=\frac{h}{15}\)
h = \(\frac{15 \sqrt{3}}{2}\) मी.
अत: विकल्प (B) सही है।

प्रश्न 7.
6 मीटर ऊँचे एक खम्भे की छाया 2\(\sqrt{3}\) मीटर लम्बी हो तो सूर्य का उन्नतांश कोण है:
(A) 60°
(B) 45°
(C) 30°
(D) 90°
हल:
माना कि AB एक खम्भा है जिसकी ऊँचाई 6 मीटर है।
खम्भे की छाया की लम्बाई (BC) = 2\(\sqrt{3}\) मीटर
माना कि सूर्य का उन्नतांश कोण (∠ACB) = θ
अत: समकोण ΔABC में

tan θ = \(\frac{A B}{B C}\)
⇒ tan θ = \(\frac{6}{2 \sqrt{3}}\)
⇒ tan θ = \(\sqrt{3}\)
⇒ tan θ = tan 60°
⇒ θ = 60°
अत: सही विकल्प (A) है।

प्रश्न 8.
किसी मीनार की छाया उसकी ऊँचाई के बराबर हो तो सूर्य का उन्नयन कोण है:
(A) 90°
(B) 60°
(C) 45°
(D) 30°
हल:
माना BC कोई मीनार है, जिसकी ऊँचाई / मीटर है।

इसकी छाया AB, h मीटर होगी।
पुनः माना सूर्य का उन्नयन कोण ∠CAB = θ
समकोण ΔABC में,
tan θ = \(\frac{B C}{A B}=\frac{h}{h}\)
⇒ tan θ = 1
⇒ tan θ = tan 45°
∴ θ = 45°
अतः सही विकल्प (C) है।

प्रश्न 9.
10 मीटर ऊँची एक मीनार के शिखर से पृथ्वी पर एक बिन्दु का अवनमन कोण 30° है। बिन्दु की मीनार के आधार से दूरी है:
(A) 10 \(\sqrt{3}\) मीटर
(B) \(\frac{10}{\sqrt{3}}\) मीटर
(C) 10 मीटर
(D) 5\(\sqrt{3}\) मीटर
हल:
माना AC कोई मीनार है जिसकी ऊँचाई 10 मीटर है।

माना मीनार के आधार से बिन्दु की दूरी (BC) = x मीटर
मीनार के शिखर से पृथ्वी पर एक बिन्दु का अवनमन कोण 30° है।
∴ ∠XAB = 30°
∠XAB = ∠ABC = 30° (एकान्तर कोण)
समकोण ΔACB में,
tan 30° = \(\frac{A C}{B C}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{10}{x}\)
∴ x = 10\(\sqrt{3}\) मीटर
अतः बिन्दु की मीनार के आधार से दूरी 10\(\sqrt{3}\) मीटर होगी सही विकल्प (A) है।

प्रश्न 10.
एक पतंग भूमि से 30 मी की ऊँचाई पर 60 मी लंबी डोरी की सहायता से उड़ रही है। यह मानते हुए कि डोरी में कोई ढील नहीं है, पतंग का भूमि पर उन्नयन कोण है:
(A) 45°
(B) 30°
(C) 60°
(D) 90°
हल:
माना कि भूमि से 30 मीटर की ऊँचाई पर पतंग की स्थिति है जोकि 60 मीटर लंबी डोरी (AC) की सहायता से उड़ रही है। माना कि पतंग की डोरी का क्षैतिज के साथ कोण θ है।
अर्थात् ∠ACB = θ
समकोण ΔABC में,

sin θ = \(\frac{A B}{A C}\)
⇒ sin θ = \(\frac{30}{60}\)
⇒ sin θ = \(\frac{1}{2}\)
⇒ sin θ = sin 30°
⇒ θ = 30°
अतः विकल्प (B) सही है।

प्रश्न 11.
एक नदी के ऊपर एक पुल नदी के तट के साथ 45° का कोण बनाता है। यदि नदी के ऊपर पुल की लम्बाई 150 मीटर है, तो नदी की चौड़ाई क्या होगी:
(A) 75\(\sqrt{2}\) मीटर
(B) 50\(\sqrt{2}\) मीटर
(C) 75 मीटर
(D) 150 मीटर
हल:
माना AC पुल है जिसकी लम्बाई 150 मीटर है
तथा BC नदी की चौड़ाई है। पुल नदी के साथ 45° का कोण बनाता है।
अर्थात् ∠CAB = 45°
समकोण ΔABC में,
sin 45° = \(\frac{B C}{A C}\)

\(\frac{1}{\sqrt{2}}=\frac{B C}{150}\)
BC = \(\frac{150}{\sqrt{2}}\)
BC = \(\frac{150 \sqrt{2}}{\sqrt{2} \times \sqrt{2}}\)
BC = 75\(\sqrt{2}\)
अतः नदी की चौड़ाई 75\(\sqrt{2}\) मीटर होगी।
सही विकल्प (A) है।

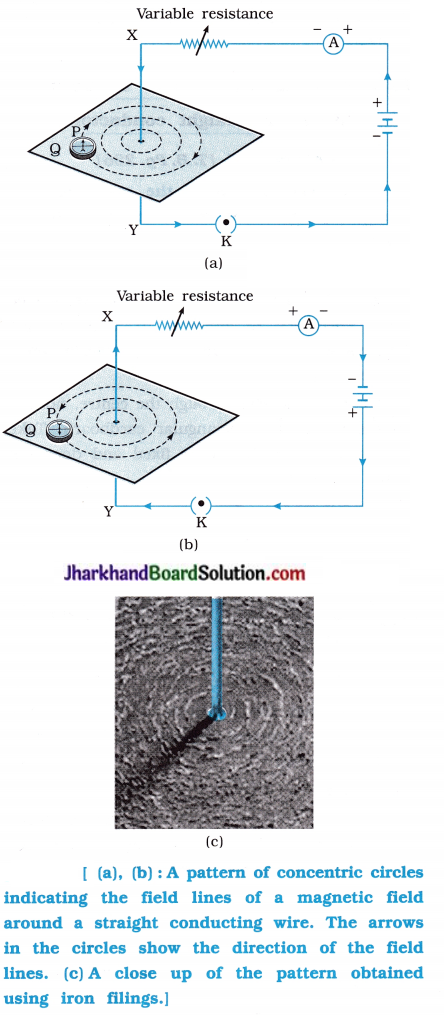
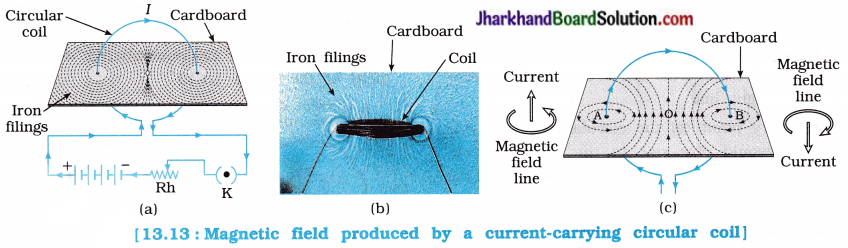
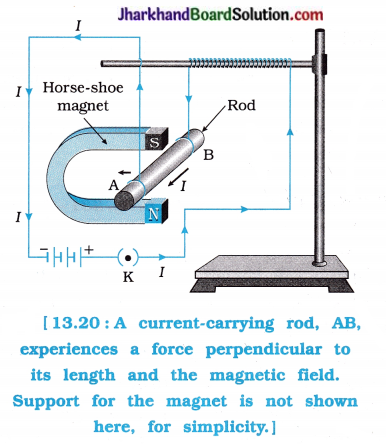
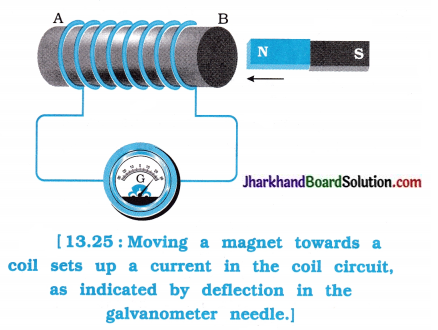
Jharkhand Board JAC Class 10 Science Important Questions Chapter 12 Electricity Important Questions and Answers.

JAC Board Class 10 Science Important Questions Chapter 12 Electricity

Additional Questions and Answers

Question 1.
Solve the following examples :
1. 240 joules of work Is done in moving 20 coulomb electric charge from one pole to the other pole of a battery. Calculate the voltage of the battery.
Answer:
12 V

2. 5 V electric potential difference is applied between two ends of a conducting wire. If 600 C of electric charge passes through it in 10minutes, calculate the resistance of the wire.
Answer:
5 Ω

3. TWo copper wires A and B have the same mass. The resistance of wire A is 0.5 Ω and the length of wire B is double that of wire
A. Find the resistance of wire B.
Answer:
2 Ω

4. If the length of a given conducting wire is kept constant and its diameter is doubled, what will be the resistance of the new wire?
Answer:
\(\frac { R }{ 4 }\)

5. Two resistors of 1 kΩ and 200Ω are connected in series with a 12 V battery. Calculate the current flowing in the circuit and the voltage developed across the 200 Ω resistor.
Answer:
I = 0.01 A, V = 2 V

6. For the circuit shown in figure given below, calculate
(a) the total resistance of the circuit.
(b) the total current flowing in the circuit.
(c) the voltage developed across the two ends of R₁.

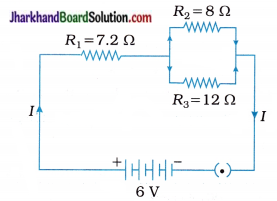
Answer:
(a) 12 Ω
(b) 0.5 A
(c) 3.6 V

7. Two 60W bulbs are used for 4hours every day and five 100W bulbs are used for 5 hours everyday. How many units of electricity will be consumed in 30 days?
Answer:
89.4 units

8. 24 W power is consumed by a bulb when it is connected with 12 V battery. How much power will be consumed if it is connected with 6V battery?
Answer:
6 W

9. Find the equivalent resistance of the circuit shown in the following figure :

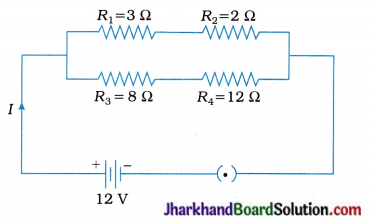
Also find the total current flowing in this circuit.
Answer:
R = 4Ω, I = 3 A

10. In the following circuit A, B and C are three ammeters. 0.5A current is shown by ammeter B.

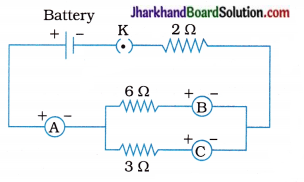
(a) Find the currents passing through ammeters A and C.
(b) Find the total resistance of the circuit.
Answer:
(a) current passing through ammeter C = 1 A, current passing through ammeter A= 1.5 A
(b) 4Q

11. If an electric bulb gives light for lhour while carrying 0.5A current, how much electric charge passes through it and how many electrons pass through it during this time? (e = 1.6 x 10^-19C)
Answer:
Q = 1800 C,
n = 1.125 x 10²²

12. An electric current of 64mA flows through a bulb for 10minutes. How many electrons pass through the bulb during this time?
(e = 1.6 x 10^-19C)
Answer:
n = 2.4 x 10²⁰

13. In order to get a current of 0.5A in a circuit by connecting a bulb of resistance 20 Ω with 12 V battery, what should be the resistance to be connected in series? What will be the voltage drop across the bulb?
Answer:
R = 4Ω, V = 10 V

14. Three resistors are connected in parallel with a 30V battery. A current of 7.5A flows through the circuit. If two out of the three resistors are of 10Ω and 12Ω, determine the resistance of the third resistor.
Answer:
15 Ω

15. For the circuit shown in the following figure, determine the equivalent resistance between points A and B. Also find the current I flowing through the circuit.

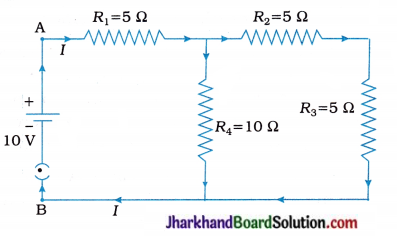
Answer:
10 Ω, 1 A

16. Determine the equivalent resistance between point A and B in the following circuit:

Answer:
2 Ω

17. In a house, if three bulbs of 100W, 60W and 40 W respectively are used 2 hours per day, how many units of electrical energy will be consumed in 30 days?
Answer:
12 units

18. Find the electric current in the following circuit:

Answer:
0.1 A

19. Determine the equivalent resistance between points X and Y in the following circuit:

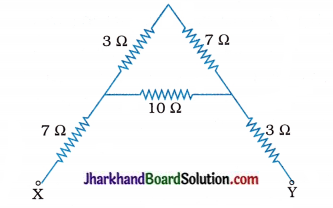
Answer:
15 Ω

20. An electric heater consumes 4.4 kW power when connected with a 220V line voltage.
(1) Calculate the current flowing in the heater.
(2) Calculate the resistance of the heater.
(3) Calculate the energy consumed in 2 hours.
Answer:
(1) 20 A
(2) 11 Ω
(3) 3.168 x 10⁷ J

Question 2.
State at least three points of difference between the following terms / quantities:
(1) Resistance and Resistivity
Answer:

Resistance	Resistivity
1. Resistance is the ratio of the voltage applied between the two ends of a conductor to the electric current flowing through the conductor. (R = \(\frac { V }{ I }\))	1. Resistivity of a substance is the resistance offered by a conducting wire of unit length and unit cross-sectional area. (ρ = R. \(\frac { A }{ l }\))
2. It depends on the type of substance, physical conditions such as temperature and pressure and dimensions of the conductor (length and area of cross-section).	2. It depends on the type of substance, temperature of the conductor and pressure exerted on the conductor.
3. Its SI unit is Ω.	3. Its SI unit is Ω m.

(2) Series combination of resistors and Parallel combination of resistors
Answer:

Series combination of resistors	Parallel combination of resistors
1. In a series combination of resistors, the resistors are connected across two points in the circuit such that the current flowing through each resistor is the same and only one path is available for the current to flow.	1. In a parallel combination of resistors, the resistors are connected across two points in the circuit such that the voltage drop across two ends of each resistor remains the same and more than one path are available for the current to flow.
2. In this case, the total voltage drop is equal to the sum of the voltage drops across each resistor.	2. In this case, the total current flowing through the circuit is equal to the sum of the currents flowing through each resistor.
3. The equivalent resistance of a series combination of resistors is equal to the sum of the resistances of the individual resistors.	3. The reciprocal of the equivalent resistance of a parallel combination of resistors is equal to the sum of the reciprocal of the resistances of the  individual resistors.
4. In this case, the equivalent resistance is greater than the largest of all the individual resistances.	4. In this case, the equivalent resistance is smaller than the smallest of all the individual resistances.
5. This combination is used in order to increase the resistance of the circuit.	5. This combination is used in order to decrease the resistance of the circuit.
6. In this case, the total current in the circuit decreases.	6. In this case, the total current in the circuit increases.

Question 3.
Give scientific reasons for the following statements:
(1) It is not advisable to connect an electric bulb and electric heater in series.
Answer:
An electric bulb and electric heater have different resistances and the requirement of current for their proper functioning is different.
Moreover, sometimes the devices may get damaged. If one of the devices stops and the circuit breaks, the other device cannot be used.

(2) For domestic purposes, different electrical devices are connected in parallel instead of connecting them in series.
Answer:
Each device gets the full and same voltage as that of the electric supply.

Also each device gets proper current depending on its resistance.
If one of the devices is switched OFF / ON, other electrical devices remain unaffected.

(3) Fairy decorative lights are always connected in parallel.
Answer:
If the fairy lights are connected in series, the equivalent resistance offered will be greater and the brightness of the bulbs will be affected. But in parallel connection all the bulbs will glow with same intensity and if any one bulb gets fused the other bulbs will continue to glow.

(4) In a tungsten electric-bulb, a coil of wire, rather than a straight wire, is used.
Answer:
A coil of wire has much greater surface area than a straight wire if enclosed in the same bulb. This results in more emission of light.
Hence, in a tungsten electric-bulb, a coil of wire, rather than a straight wire, is used.

Objective Questions and Answers

Question 1.
Answer the following questions in one word / sentence:
(1) Name the scientist in whose honour the SI unit of electric current is named.
Answer:
Andre Ampere

(2) Which type of electric force acts between the proton and the electron?
Answer:
Attractive electric force

(3) Write the relation between the joule, coulomb and the volt.
Answer:
1 volt = \(\frac { 1 joule }{ 1 coulomb }\)

(4) How is the device measuring electric current connected in the circuit?
Answer:
In series

(5) You are given aluminium wire and copper wire having the same dimensions. Which wire will carry more electric current if the applied potential difference is the same for the two wires?
Answer:
Copper wire

(6) Name one electric appliance working on the principle of heating effect of electric current.
Answer:
Electric iron

(7) If a resistive wire is uniformly stretched, what will be a change in its resistance?
Answer:
Its resistance will increase

(8) Name a physical quantity which can be expressed in Ws.
Answer:
Electric energy

(9) Name the particle responsible for flow of electric current in a metallic conductor.
Answer:
The electron

(10) Which instrument is used to measure electric potential difference ?
Answer:
Voltmeter

(11) Draw a symbol for variable resistor.
Answer:

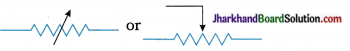
(12) How much energy in joule is consumed when 100 units electricity are used?
Answer:
3.6 x 10⁸ J

(13) Write the Ohm’s law in the form of a formula.
Answer:
V = IR
Where
V = Potential difference across the ends of metallic wire of resistance R at constant temperature
I = Current flowing through the metallic wire

Question 2.
Fill in the blanks:

1. Electric charge on …………………… is taken negative.
2. In a substance, the direction of conventional current is opposite to the direction of …………………… current.
3. Electric component, …………………… has symbol
   
4. The SI unit of electric potential is ……………………
5. In a circuit, a voltmeter is always connected …………………… to the electrical component.
6. When a battery is connected in a circuit, in the external circuit, electric current flows from the …………………… terminal to the ………………….. terminal of the battery.
7. The SI unit of electric power is ……………………
8. According to Joule’s law, heat energy produced in a resistor is directly proportional to the square of ……………………
9. 1 unit (commercial unit of electric energy) = …………………… J
10. The equivalent resistance between points X and Y in the circuit shown in the following figure is ……………………
    
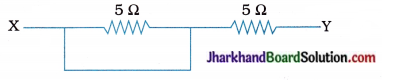
11. The rate of consumption of electric energy with time is called ……………………
12. 1 A = …………………… µA.

Answer:

1. the electron
2. electron
3. resistor
4. the volt or the joule per coulomb
5. in parallel
6. positive, negative
7. the watt
8. the electric current flowing through the resistor
9. 3.6 x 10⁶
10. 5 Ω
11. electric power
12. 10⁶

Question 3.
State whether the following statements are true or false:

1. The SI unit of electric current is the coulomb.
2. To measure electric current, an ammeter is connected in series with a resistor.
3. W = VQ.
4. The current flowing through each resistor is the same when resistors having different! resistances are connected in parallel.
5. The equivalent resistance (R_s) of a series combination of resistors is given by \(\frac{1}{R_{\mathrm{s}}}=\frac{1}{R_1}+\frac{1}{R_2}\) + ……………
6. Nichrome wire is used to make resistors.
7. When the temperature of a metallic substance increases up to a certain limit, its resistance decreases.
8. For two wires of the same material and having the same length, the resistance of the thicker wire is less than that of the other wire.
9. Electric charge is measured by using an ammeter.
10. Work can be expressed in coulomb-volt.
11. Two bulbs of 60 W, 220 V when connected in series with a supply voltage of 220 V, light up with maximum intensity.

Answer:

1. False
2. True
3. True
4. False
5. False
6. True
7. False
8. True
9. False
10. True
11. False

Question 4.
Match the following :
(1)

Column I	Column II	Column III
1. Electric current	a. VI	p. The volt / ampere
2. Electric potential difference	b. Q/t	q. The joule
3. Resistance	c. W/Q	r. The coulomb / second
4. Electric energy	d. V/I	s. The joule / second
5. Electric power	e. V I t	t. The volt

Answer:
(1 – b – r), (2 – c – t), (3 – d – p), (4 – e – q), (5 – a – s).

(2)

Column I	Column II	Column III
1. Copper	a. Insulator	p. It is used to make resistance.
2. Nichrome	b. Semi-conductor	q. It stops electric current.
3. Wood	c. Conductor	r. It is used to make conduc-ting wire.
4. Silicon	d. Resistor	s. It is used to make elec-tronic components.

Answer:
(1 – c – r), (2 – d – p), (3 – a – q ), (4 – b – s).

(3)

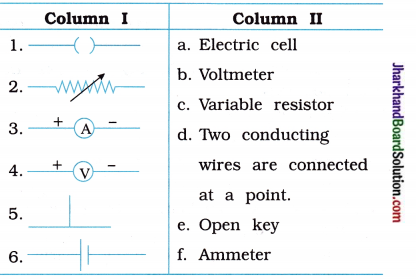
Answer:
(1 – e), (2 – c), (3 – f), (4 – b), (5 – d), (6 – a).

Question 5.
Choose the correct option from those given below each question:
1. The SI unit of electric charge is the ……………….
A. ampere
B. volt
C. watt
D. coulomb
Answer:
D. coulomb

2. How many electrons will be there in 1.6 C charge?
A. 10¹⁷
B. 10¹⁸
C. 10¹⁹
D. 10²⁰
Answer:
C. 10¹⁹

3. 1 µA = …………………. mA
A. 10^-16
B. 10^-3
C. 10¹⁹
D. 10²⁰
Hint IµA = 10^-3 x 10^-3A = 10^-3 mA
Answer:
B. 10^-3

4. Which of the following materials has more number of free electrons in a given volume?
A. Copper
B. Glass
C. Rubber
D. Iron
Answer:
A. Copper

5. According to Ohm’s law ………………….
A. the resistance increases with an increase in current.
B. the resistance increases with an increase in voltage.
C. the current increases with an increase in voltage.
D. both the resistance and current increase with an increase in voltage.
Answer:
C. the current increases with an increase in voltage.

6. The formula for electric current is
A. I = Qt
B. I = Y
C. I = \(\frac { Q }{ t }\)
D. I = W.t
Answer:
C. I = \(\frac { Q }{ t }\)

7. 2 A electric current is passed for 1 minute through a conducting wire. How much electric charge will pass through this wire?
A. 2C
B. 30 C
C. 60 C
D. 120 C
Answer:
D. 120 C
Hint : I = \(\frac { Q }{ t }\)
∴ Q = I x t = (2 A)(1 x 60s)
= 120 C

8. If 4.8 A current is passed through an electrical appliance, the number of electrons passing through it in 1 second will be ………………..
A. 0.33 x 10¹⁹
B. 3.3 x 10¹⁹
C. 3 x 10¹⁹
D. 4.8 x 10¹⁹
Answer:
C. 3 x 10¹⁹
Hint: I = \(\frac { Q }{ t }\) = \(\frac { ne }{ t }\)
∴ n = \(\frac { I × t }{ e }\)
= \(\frac{4.8 \times 1}{1.6 \times 10^{-19}}\)
= 3 x 10¹⁹

9. Which of the following formulae represents voltage?
A. \(\frac{\text { Work }}{\text { current } \times \text { time }}\)
B. \(\frac{\text { Work } \times \text { time }}{\text{ Current} }\)
C. Work x electric charge
D. Work x electric charge x time
Answer:
A. \(\frac{\text { Work }}{\text { current } \times \text { time }}\)
Hint: V = \(\frac { W }{ Q }\) = \(\frac { W }{ I.t }\)

10. The unit of electric potential difference is ………………..
A. J
B. J/C
C. JC
D. C/J
Answer:
B. J/C

11. If the work done to take 3C electric charge from one point to another point is 15 J, what will be the potential difference between these two points?
A. 3V
B. 15 V
C. 5V
D. 45 V
Answer:
C. 5V
Hint: Potential difference V = \(\frac { W }{ Q }\)
= \(\frac { W }{ Q }\)

12. The resistance of a conducting wire is 10 Ω. If a battery of 1.5 V is connected to it, the electric current flowing through it will be
A. 0.15 mA
B. 1.5 mA
C. 15 mA
D. 150 mA
Answer:
D. 150 mA
Hint:
R = \(\frac { V }{ I }\) = \(\frac { 1.5 }{ 10 }\) = 0.15
= 150 x 10^-3
= 150 mA

13. On which factors does the resistivity of a conducting wire depend?
A. The length of the wire
B. The area of cross-section of the wire
C. The volume of the wire
D. The material of the wire
Answer:
D. The material of the wire

14. If five equal pieces of a resistance wire having 5 Ω resistance are connected in parallel, their equivalent resistance will be ……………………….
A. \(\frac { 1 }{ 5 }\) Ω
B. 1 Ω
C. 5 Ω
D. 25 Ω
Answer:
A. \(\frac { 1 }{ 5 }\) Ω
Hint: When a resistance wire having 5 Ω resistance is cut into five equal pieces, each piece will have resistance 1 Ω, i.e., R₁ = R₂ = R₃ = R₄ = R₅ = 1 Ω.
The equivalent resistance (R_p) of their parallel combination is given by,
\(\frac{1}{R_{\mathrm{p}}}=\frac{1}{R_1}+\frac{1}{R_2}+\frac{1}{R_3}+\frac{1}{R_4}+\frac{1}{R_5}\)
∴ \(\frac{1}{R_{\mathrm{p}}}=\frac{1}{1}+\frac{1}{1}+\frac{1}{1}+\frac{1}{1}+\frac{1}{1}\)
∴ \(\frac{1}{R_{\mathrm{p}}}=\frac{5}{1}\)
∴ R_p = \(\frac { 1 }{ 5 }\) Ω

15. The SI unit of resistivity is …………………
A. Ω
B. Ω m
C. \(\frac { Ω }{ m }\)
D. \(\frac { m }{ Ω }\)
Answer:
B. Ω m

16. What will be the equivalent resistance between points A and B of the following electric circuit?

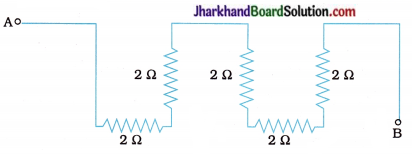
A. 1 Ω
B. 2 Ω
C. 5 Ω
D. 10 Ω
Answer:
D. 10 Ω
Hint: The given electric circuit can be drawn as follows:

As all resistors are connected in series, their equivalent resistance,
R_s = 2 + 2 + 2 + 2 + 2 = 10 Ω

17. The equivalent resistance between points A and B of the following electric circuit will be ………………..

A. 4 Ω
B. 8 Ω
C. 2 Ω
D. 16 Ω
Answer:
A. 4 Ω
Hint: The given electric circuit can be drawn as follows:

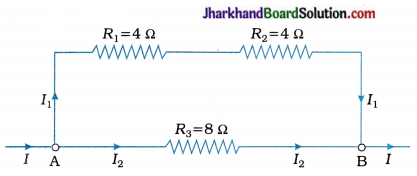
It is clear from the circuit that resistors R₁ and R₂ are connected in series.
∴ R’ = R₁ + R₂ = 4 + 4 = 8 Ω
Now, R’ is connected with R₃ in parallel combination.
∴\(\frac{1}{R}=\frac{1}{R^{\prime}}+\frac{1}{R_3}\)
= \(\frac { 1 }{ 8 }\) + \(\frac { 1 }{ 8 }\)
= \(\frac { 2 }{ 8 }\) = \(\frac { 1 }{ 4 }\)
∴ R = 4 Ω

Question 18.
The equivalent resistance between points A and B in the following electric circuit is ……………..

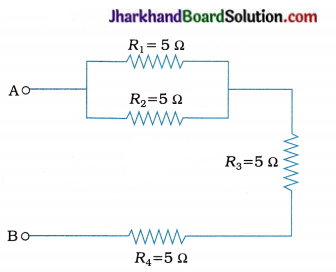
A. 2.5 Ω
B. 5 Ω
C. 12.5 Ω
D. 20 Ω
Answer:
C. 12.5 Ω
Hint: Resistors R₁ and R₂ are connected in parallel.
∴ R’ = \(\frac{R_1 R_2}{R_1+R_2}=\frac{5 \times 5}{5+5}\) = 2.5 Ω
Now, R’, R₃ and R₅ are connected in series
∴R = R’ + R₃ + R₅
= 2.5 + 5 + 5 = 12.5 Ω

19. Which physical quantity is expressed in kWh?
A. Work
B. Electric power
C. Electric Current
D. Electric potential
Answer:
A. Work

20. 1 kWh = ………………. J
A. 3.6 x 10⁶
B. 3.6 x 10³
C. 3.6 x 10^-6
D. 3.6 x 10^-3
Answer:
A. 3.6 x 10⁶
Hint : 1 kWh = (1000 W) (3600 s)
= 3.6 x 10⁶ J

21. An electric heater consumes 1.1 kW power when 220 V voltage is applied to it. The current flowing through it must be ……………….
A. 1.1 A
B. 2.2 A
C. 4 A
D. 5 A
Answer:
D. 5 A
Hint: P = VI
∴ I = \(\frac{P}{V}=\frac{1.1 \times 10^3}{220}=\frac{1100}{220}\)

22. 1 A = ……………. mA
A. 100
B. 10³
C. 10^-3
D. 10^-6
Answer:
B. 10³

23. In an electric field, electric potential at a point is 10 V How much work has to be done to bring 0.5 C electric charge from infinite distance to that point?
A. 0.5 J
B. 2 J
C. 5 J
D. 10 J
Answer:
C. 5 J
Hint: W = VQ = (10) (0.5) = 5 J

24. ……………… is the work done in moving a unit positive charge from point A to B in an electric field.
A. Electric potential at point A.
B. Electric potential at point B.
C. Electric potential difference between points A and B.
D. Electric current from point A to point B.
Answer:
C. Electric potential difference between points A and B.

25. In an electric field, electric potential at point A is 40 V and that at point B is 90 V The work to be done in taking 2 C charge from point A to point B is ………………
A. 25 J
B. 50 J
C. 90J
D. 100 J
Answer:
D. 100 J
Hint: V_B – V_A = \(\frac { W }{ Q }\)
∴ W = Q (V_B – V_A)
= (2) (90 – 40) = 100 J

26. Sonika is working with the circuit shown in the following figure :

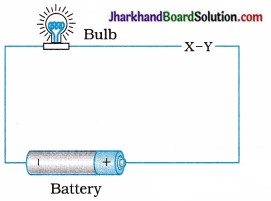
The circuit has two gaps : X and Y. She has wires of five different materials – I, II, III, IV and V She knows that the bulb will light up only when both gaps are filled with conducting materials.
She records her observations in a table. After completing the experiment, ink fell on the paper and she lost entries in row 3.

No.	Material in X	Material in Y	Bulb (On/Off)
1	I	II	Off
2	I	IV	On
3			Off
4	III	V	On

Based on the rest of the information in the table, out of the following what could be the materials in row 3?
A. II and III
B. III and IV
C. IV and V
D. I and III
Answer:
A. II and III
Hint: The entries in rows 2 and 4 show that
I : conducting material, IV : conducting material, III : conducting material, V : conducting material,
This information, combined with the entries in row 1, shows that
II : Non-conducting material Row 3 must include II.

27. ‘Ω m’ is the SI unit of ……………..
A. resistance
B. resistivity
C. conductivity
D. resistance per unit length
Answer:
B. resistivity

28. I – V graph for a resistance wire is shown in the figure given below:

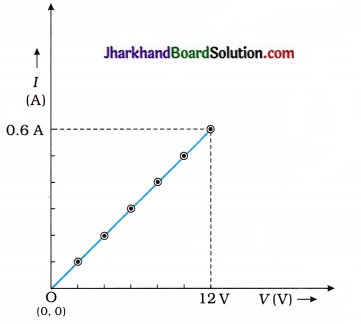
What will be resistance of this wire?
A. 50 Ω
B. 20 Ω
C. 7.2 Ω
D. \(\frac { 1 }{ 120 }\)Ω
Answer:
B. 20 Ω
Hint: The slope of I-V graph represents the reciprocal of resistance.
∴ Slope = \(\frac { 1 }{ R }\) = \(\frac { ∆I }{ ∆V }\)
∴R = \(\frac{\Delta V}{\Delta I}=\frac{12-0}{0.6-0}=\frac{12}{0.6}\) = 2.0 Ω

29. What will be the change in the resistivity of a resistance wire, when its length is doubled by stretching it uniformly?
A. Will be doubled
B. Will be half
C. Will be one-fourth
D. Will not change
Answer:
D. Will not change

30. Observe the circuit in the following figure and select the correct statement:

A. Resistors with resistances R₁ and R₂ are connected in series.
B. Resistors with resistances R₁ and R₂ are connected in parallel.
C. Resistors with resistances R₂ and R₃ are connected in series.
D. Resistors with resistances R₂ and R₃ are connected in parallel.
Answer:
D. Resistors with resistances R₂ and R₃ are connected in parallel.

31. Resistors with resistances 5 Ω, 10 Ω and 15 Ω are connected in parallel to each other. What will be the equivalent resistance of the circuit? A. Less than 5 Ω
B. More than 15 Ω
C. More than 30 Ω
D. Equal to 30 Ω
Answer:
A. Less than 5 Ω

32. The resistance of a wire is R. When this wire is stretched so that its length becomes double, its cross-sectional area becomes half. What will be the resistance of this stretched wire?
A. \(\frac { R }{ 2 }\)
B. R
C. 2R
D. 4R
Answer:
D. 4R
Hint: Original resistance of the wire R = ρ.\(\frac { 1 }{ A }\)
Resistance of the stretched wire R’ = ρ.\(\frac { l’ }{ A’ }\)
But l’ = 2l and A’ = \(\frac { A }{ 2 }\)
∴ R’ = ρ\(\frac{2 l}{\frac{A}{2}}\) = (4)ρ\(\frac { 1 }{ A }\) = 4R

33. When three resistors, each of resistance R, are connected in parallel, the equivalent resistance is found out to be 10 Ω. R must be …………..
A. 10 Ω
B. 20 Ω
C. 30 Ω
D. 5 Ω
Answer:
C. 30 Ω
Hint :
\(\frac{1}{R^{\prime}}=\frac{1}{R_1}+\frac{1}{R_2}+\frac{1}{R_3}\)
Now, R₁ = R₂ = R₃ = R
∴ \(\frac{1}{R^{\prime}}=\frac{1}{R}+\frac{1}{R}+\frac{1}{R}=\frac{3}{R}\)
∴ R = 3R’ = 3 (10) = 30 Ω

34. In the following figure, what will be the equivalent resistance between points A and B?

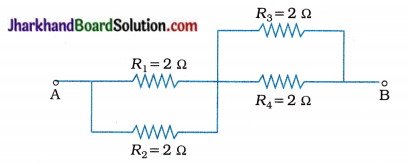
A. 1 Ω
B. 2 Ω
C. 4 Ω
D. 8 Ω
Answer:
B. 2 Ω
Hint: Here, resistors with resistances R₁ and R₂ are in parallel.
∴ R’ = \(\frac{R_1 R_2}{R_1+R_2}=\frac{2 \times 2}{2+2}\) = 1 Ω
Also, resistors with resistances R₃ and R₄ are in parallel.
∴ R” = \(\frac{R_3 R_4}{R_3+R_4}=\frac{2 \times 2}{2+2}\) = 1 Ω
The equivalent resistance of the resulting series combination will be
R = R’ + R”
= 1 + 1 = 2 Ω

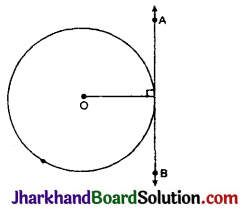
35. A wire having resistance 20 Ω is bent in the form of a circle as shown in the following figure :

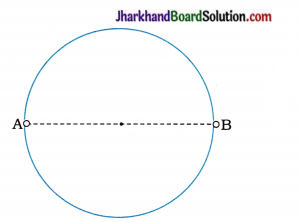
What will be the resistance between two end points A and B located on the diameter?
A. 5 Ω
B. 10 Ω
C. 20 Ω
D. 40 Ω
Answer:
A. 5 Ω
Hint: The resistance of the wire is R = 20 Ω. Hence, the resistance of the upper part of the circle = \(\frac { R }{ 2 }\) = 10 Ω
Similarly, the resistance of the lower part of the circle = \(\frac { R }{ 2 }\) = 10 Ω.

As shown in the figure above, resistors with resistances 10 Ω and 10 Ω are in parallel. So, the equivalent resistance of the combination will be R’ = \(\frac { (10)(10) }{ 10+10 }\) = 5 Ω

36. Which of the following options is not the formula for heat energy (in joule) produced when a current is passed through a conductor?
A. H = I²Rt
B. H = \(\frac { V²t }{ R }\)t
C. H = IVt
D. H = \(\frac { V² }{ I² }\)t
Answer:
D. H = \(\frac { V² }{ I² }\)t

37. Which option is correct for the following statements A and B?
Statement A : The current passing through each resistor is the same in a series combination of resistors.
Statement B : The voltage between two ends of each resistor is the same in a parallel combination of resistors.
A. Statement A is true but statement B is false.
B. Statement A is false but statement B is true.
C. Statement A and statement B both are true.
D. Statement A and statement B both are false.
Answer:
C. Statement A and statement B both are true.

38. In which of the following circuits the voltmeter and ammeter are connected properly to verify the Ohm’s law?

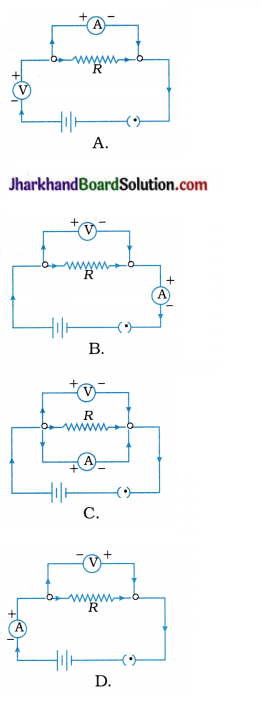
Answer:

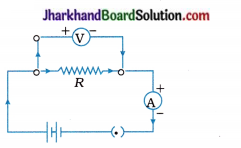
Hint: An ammeter should be connected in series to measure electric current and a voltmeter should be connected in parallel to the resistor to measure potential difference. While joining these two instruments their positive and negative terminals should be taken into consideration.

39. Maximum 1A current can pass through a bulb having resistance 100 Q. What will be the power of this bulb?
A. 10W
B. 100 W
C. 1000 W
D. 0.01 W
Answer:
B. 100 W
Hint: P = I²R = (1)²(100) = 100 W

40. Which of the following is not a unit of electric energy?
A. The watt-second
B. The kilowatt hour
C. The joule
D. The watt
Answer:
D. The watt

41. Of which physical quantity is the unit, ‘VA’?
A. Electric energy
B. Electric power
C. Heat energy
D. Electric potential
Answer:
B. Electric power

42. Which of the following circuit symbols is used to represent an electric cell?

Answer:

43. The resistance of 300 m long wire with cross-sectional area 10^-6m² and resistivity 10^-7 Ω m is
A. 30 Ω
B. 3 Ω
C. 0.3 Ω
D. 300 Ω
Answer:
A. 30 Ω
Hint: R = ρ\(\frac { l }{ A }\)
= 10^-7 x \(\frac{300}{10^{-6}}\)
= 30 Ω

44. What will be the equivalent resistance of the following circuit?

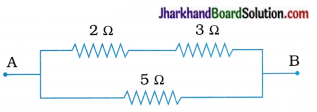
A. 10 Ω
B. 5 Ω
C. 2.5 Ω
D. 1 Ω
Answer:
C. 2.5 Ω
Hint: The resistance of the upper arm of the circuit is 2 + 3 = 5Ω.
Now, the upper arm and lower arm are connected in parallel to each other. So, the equivalent resistance of the circuit
R = \(\frac { 5×5 }{ 5+5 }\) = 2.5 Ω

45. Three resistors of resistances 2 Ω, 3 Ω and 5 Ω are connected in series with a 10 V battery.
The voltage across 2 Ω resistor will be
A. 10 V
B. 5V
C. 3V
D. 2V
Answer:
D. 2V
Hint: The equivalent resistance of the circuit R = 2 + 3 + 5= 10 Ω.
The current flowing through the circuit
I = \(\frac { V }{ R }\) = \(\frac { 10 }{ 10 }\) = 1 A
The voltage across 2 Ω resistor
= 2 x I = 2 Ω x 1 A = 2 V

46. An electric iron of power 2 kW is used for 3 hours. At ₹ 5 per unit, the electricity bill will be
A. ₹ 45
B. ₹ 30
C. ₹ 15
D. ₹ 10
Answer:
B. ₹ 30
Hint: Electric energy consumed in 3 hours W = P x t
= 2 kW x 3 h = 6 kWh = 6 unit
Cost of electric power consumed (electricity bill)
= 5 x 6 = ₹ 30

47. The working of electric fuse is based on the ……………
A. chemical effect of electric current.
B. heating effect of electric current.
C. voltage regulation in the circuit.
D. current regulation in the circuit.
Answer:
B. heating effect of electric current.

48. Resistivity of a material depends on …………………. of the material.
A. the length of the wire
B. the area of cross-section of the wire
C. the temperature
D. the volume of the wire
Answer:
C. the temperature

49. When resistors of resistances R₁ and R₂ (R₂ < R₁) are connected in parallel and the currents flowing through them are I₁ and I₂ respectively, then
A. I₁ = I₂
B. I₁ > I₂
C. I₁ < I₂
D. nothing can be said about the currents
Answer:
C. I₁ < I₂

50. When resistors of resistances R₁ and R₂ (R₂ > R₁) are connected in series and the currents flowing through them are I₁ and I₂ respectively, then
A. I₁ = I₂
B. I₁ > I₂
C. I₁ < I₂
D. nothing can be said about the currents
Answer:
A. I₁ = I₂

51. In the following circuit, which electric component is connected in a wrong manner?

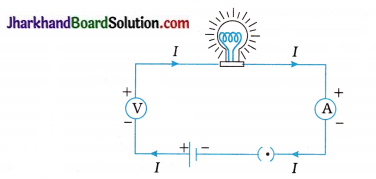
A. Voltmeter
B. Ammeter
C. Plug key
D. Cell
Answer:
A. Voltmeter

52. 1 C = ……………. µC
A. 10^-6
B. 10^-3
C. 1
D. 10⁶
Answer:
D. 10⁶

53. Connection of two cells of 1.5 V is shown in the following figure :

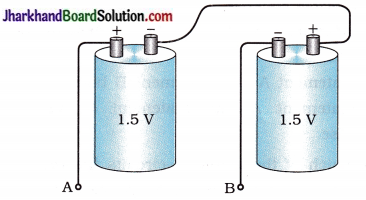
What is the voltage between the points A and B?
A. 1.5 V
B. 3 V
C. 0.75 V
D. 0 V
Answer:
B. 3 V

54. The following figure shows the V-I graph for four different resistors :

Which resistor has the maximum resistance?
A. A
B. B
C. C
D. D
Answer:
D. D

55. A potential difference of 100 V is applied across an electric bulb marked 40 W, 200 V. The power consumed in the bulb is …………………
A. 100 W
B. 40 W
C. 20 W
D. 10W
Answer:
D. 10W
Hint: P = \(\frac{V^2}{R}\)
∴ Resistance of the filament of the bulb,
R = \(\frac{V^2}{P}=\frac{(200)^2}{40}\) = 1000 Ω
Now, power consumed in the bulb,
P = \(\frac{V^2}{R}=\frac{(100)^2}{1000}\) = 10 W

Question 6.
Answer the following questions as directed (Miscellaneous):
(1) The voltage – current (V – I) graphs for a metallic conductor at two different temperatures T₁ and T₂ are shown below:

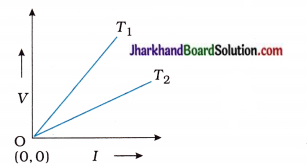
At which temperature is the resistance higher?
Answer:
At temperature T₁.
Because, the slope \(\left(\frac{\Delta V}{\Delta I}\right)\) of the graph i.e., its resistance is greater at T₁.

(2) A wire of given length is doubled on itself and this process is repeated once again. By what factor does the resistance of the wire change?
Answer:
As the length of the wire become y- of the original length its area of cross-section become 4 times.
So, new resistance
R’ = ρ\(\frac { l’}{ A’}\) = ρ\(\frac{(1 / 4)}{4 A}=\frac{1}{4 \times 4}\left(\rho \frac{l}{A}\right)\) = \(\frac { R}{ 16}\) (∵ R = ρ\(\frac { l}{ A’}\))
Thus, the new resistance is \(\frac { R}{ 16}\) of the original resistance.

(3) Through which of the following two wires, does the electric current flow more easily?
(a) A thick wire
(b) a thin wire of the same material and of the same length when connected to the same source. State the reason.
Answer:
The thick wire because its resistance would be less compared to that of the thin wire.

(4) 400 J of heat is produced in 4 s in a 4 Q resistor. Find the potential difference across the resistor.
Answer:
Here, H = 400 J
∴ \(\frac { V²t }{ R }\) = 400
∴ V² = 400 x \(\frac { R }{ t }\) = 400 x \(\frac { 4 }{ 4 }\) = 400
∴ V = 20 volts

(5) What is the commercial unit of electrical energy?
Answer:
The kilowatt hour, i.e., kWh

(6) On what principle is an electric bulb based?
Answer:
Heating effect of current

(7) In a circuit, two resistors of resistances 5 Ω and 10 Ω are connected in series. Compare the current passing through the two resistors.
Answer:
In a series combination of resistors, the same current passes through all the resistors and it is same as the total current of the circuit.
Hence, here current will be the same in two resistors.
So, the ratio of the current will be 1:1.

(8) Write the relation between resistance R of the filament of a bulb, its power and a constant S voltage V applied across it.
Answer:
R = \(\frac { V² }{ P }\)

(9) Which of the following bulbs has more resistance?
(a) A 220 V, 100 W bulb
(b) a 220 V 60 W bulb.
Answer:
60 W bulb
Here, R \(\frac { 1 }{ p }\) (∵ as both the bulbs have the j same voltage rating.)

(10) Find the minimum resistance that can be made using five resistors, each of 5 Ω.
Answer:
By connecting given resistors in parallel, resistance 1 Ω is obtained which is the minimum.
R_p = \(\frac { R }{ n }\) = \(\frac { 5 Ω }{ 5 }\) = 1 Ω

(11) How does the resistance (R) of a wire depend upon its radius (r)?
Answer:
As R ∝ \(\frac{1}{A} \propto \frac{1}{\pi r^2}\) (∵A = πr²)
∴ R ∝ \(\frac { 1 }{ r² }\) (∵π = constant)

(12) An ammeter has a range (0 – 3A) and there are 30 divisions on its scale. Calculate the least count of the ammeter.
Answer:
Here, range of ammeter = 3 ampere
(i.e., The maximum current that can be measured by the given ammeter is 3A.)
No. of divisions on scale of ammeter = 30
The least count of the ammeter
= \(\frac{\text { Range of the ammeter }}{\text { Total no. of divisions }}\)
= \(\frac { 3 A }{ 30 }\)
= 0.1 A
(i.e., Minimum current that can be accurately measured by a given ammeter is 0.1 A.)

(13) In a voltmeter there are 20 divisions between 0 mark and 0.5 V mark. Calculate the least count of the voltmeter.
Answer:
Range of voltmeter = 0.5 V
No. of divisions = 20
∴The least count of the voltmeter
= \(\frac{\text { Range }}{\text { Total no. of divisions }}\)
= \(\frac { 0.5 V }{ 20 }\)
= 0.025 V

(14) To verify the Ohm’s law a circuit diagram was drawn by a student as shown below:

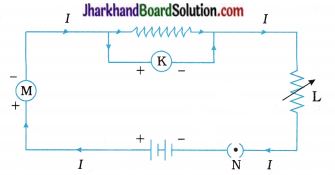
What do K, L, M, N stand for?
Answer:
K = voltmeter, L = rheostat, M = ammeter and N = plug key

(15) Four students connect 4 cells of 1.5 V each to get a battery of voltage 6 V. State the incorrect connection / connections. Justify your answer.

Answer:
A, B and C are incorrect connections (D is correct).
Because for a proper series connection of four cells, each of 1.5 V to obtain a battery of 6 V, the negative terminal of each cell should be connected to the positive terminal of the next cell or the positive terminal of each cell should be connected to the negative terminal of the next cell (connection D).

(16) Which of the following statements is correct?
(a) one volt is one joule per ampere.
(b) one volt is one joule per coulomb.
Answer:
(b) one volt is one joule per coulomb.

(17) Keeping the resistance constant, the potential difference applied across the ends of a component is halved. How does the current change?
Answer:
The current becomes half the initial current.

(18) Keeping the potential difference constant, the resistance of a circuit is halved. How does the current change?
Answer:
The current becomes double the initial S current.

(19) A potential difference of 10 V is needed i to make a current of 0.02 A flow through a wire. What potential difference is needed to make a current of 250 mA to flow through the same wire?
Answer:
According to the Ohm’s law,
R = \(\frac { V }{ I }\)
So, R = \(\frac { 10 }{ 0.02 }\)
= 500 Ω
Now, to make a current of
250 mA = 250 x 10^-3A to flow through the ; same wire, the required potential difference is
V = IR = 250 x 10^-3 x 500 = 125 V

(20) A current of 250 mA flows through a 4 kΩ resistor. What is the potential difference across the resistor?
Answer:
The potential difference across the resistor
V = IR
= 200 x 10^-3 x 4 x 10³ = 800 V

(21) The electrical resistivities of four materials ; A, B, C and D are given below:
A : 110 x 10^-8 Ω m
B : 1.0 x 10¹⁰ Ω m
C : 10.0 x 10^-8 Ω m
D : 2.3 x 10³ Ω m
Which material is a (a) good conductor (b) resistor (c) insulator (d) semi-conductor?
Answer:
(a) C
(b) A
(c) B
(d) D

(22) The equivalent resistance of a series combination of two resistors is 9 Ω and the equivalent resistance of a parallel combination of the same two resistors is 2Ω. Find the resistances of the resistors.
Answer:
Suppose two unknown resistances are x and y.
When the resistors are connected in parallel \(\frac { xy }{ x+y }\) = 2Ω and when they are connected in series x + y = 9 Ω.
So, \(\frac { xy }{ 9 }\) = 2 ∴ xy = 18 Ω
Now,
From x + y = 9, y = 9 – x
∴ x(9 – x) = 18
∴ 9x – x² = 18
∴ x² – 9x + 18 = 0
∴ x² – 6x – 3x + 18 = 0
∴ x (x – 6) – 3 (x – 6) = 0
∴ (x – 3) (x – 6) = 0
∴ x = 3 Ω or x = 6 Ω
Hence y = 9 – x = 9 – 3 = 6 Ω or y = 9 – 6 = 3 Ω
Thus, x = 3 Ω and y = 6 Ω or x = 6Ω and y = 3 Ω.

(23) An electric lamp is labelled 12 V, 36 W. This indicate that it should be used with a 12 V supply. What other information does the label provide?
Answer:
The electric lamp consumes electric energy at the rate of 36 J/s.

(24) Name two devices whose working is based on the heating effect of electric current.
Answer:
An electric iron, electric toaster

(25) Name the gases which are filled into the filament-type electric light bulbs.
Answer:
Argon, nitrogen

(26) Why are the filament-type electric light bulbs not power efficient?
Answer:
When electric power is supplied to the filament-type electric light bulb, most of the electric power consumed by the filament appears as heat (due to which the bulb becomes hot), only a small amount of electric power is converted into light. So, the filament-type electric light bulbs are not power efficient.
[Note : Tube-lights are much more power efficient because they have no filaments.]

(27) Under what conditions is the Ohm’s law applicable?
Answer:
The Ohm’s law is applicable when the physical conditions like temperature, pressure, etc. of a conductor do not change when a potential difference is applied across it.

(28) Why is Nichrome used as a heating element?
Answer:
Nichrome (alloy) has high resistivity and high melting point. Moreover, it does not react with air when it is red-hot (800 °C).

(29) Why are Constantan and Manganin used for making standard resistors?
Answer:
The resistivity of Constantan and Manganin is moderate and almost independent of temperature.
Hence, these materials are used for making standard resistors.

(30) Why is lead-tin alloy used for making a fuse?
Answer:
Lead-tin alloy has low melting point. Hence it is used for making a fuse.

(31) What is the difference between a resistor and resistance?
Answer:
A resistor is a component whereas resistance is its property due to which it opposes the flow of electrons through it.

(32) What do you mean by the term load in an electric circuit?
Answer:
Electric lamps, heaters, air conditioners, motors and other devices which work on electricity are called loads because they consume electric energy.

(33) How does the current divide itself in a parallel combination of resistors?
Answer:
In a parallel combination of resistors, the current divides in the inverse ratio of the resistances.

(34) How does the potential difference divide itself in a series combination of resistors?
Answer:
In a series combination of resistors, the potential difference across any of the resistors is directly proportional to its resistance.

(35) How is heat produced in a conductor by an electric current?
Answer:
Heat produced in a conductor by an electric current is due to collisions of free electrons with the ions and atoms in the conductor.

(36) If a number of bulbs of different wattages? are joined in parallel with a voltage source, which s bulb will glow with maximum brightness?
Answer:
The bulb with highest wattage (here, s lowest resistance) will glow with maximum brightness as P = \(\frac { V² }{ R }\) and here P ∝ \(\frac { 1 }{ R }\).

(37) Which bulb will glow with maximum brightness in case bulbs of different wattages are : joined in series with a voltage source?
Answer:
The bulb with highest wattage (here S highest resistance) will glow with maximum brightness as P = I²R and here P ∝ R.

(38) What can you say about the resistance s of an ammeter?
Answer:
The resistance of an ammeter should s be very low, ideally zero.

(39) What can you say about the resistance ; of a voltmeter?
Answer:
The resistance of a voltmeter should be very high, ideally infinite.

(40) How does the resistance of a metallic wire depend on its temperature?
Answer:
The resistance of a metallic wire increases with temperature.

(41) Two wires have the same length, same s radius but one of them is made of copper and l the other is made of iron. Which will have more resistance?
Answer:
As resistance R = ρ\(\frac { 1 }{ A }\) and resistivity ρ for iron is more than that for copper, the iron wire will have more resistance.

(42) Name a substance whose resistance decreases with temperature.
Answer:
Germanium (a semi-conductor)

(43) Name a substance whose resistance almost? remains unchanged with a change in temperature.
Answer:
Manganin (an alloy of Cu, Mn and Ni)

(44) Distinguish between the kilowatt and the kilowatt hour.
Answer:
The kilowatt (kW) is a unit of power and the kilowatt hour (kWh) is a unit of electric energy. .
1 kWh = 1 kW x 1 h

(45) Name two characteristics of a heater coil.
Answer:
A heater coil should have (i) high resistivity and (ii) high melting point.

(46) The power-voltage rating of an electric appliance is 100W-250V What does it signify?
Answer:
The electric appliance consumes a power of 100 W (i.e., an electric energy of 100 J per second) when connected to a 250 V electric supply.

(47) Why is much less heat generated in long electric cables than in the filament of an electric bulb?
Answer:
Electric cables are made of thick copper i.e., their area of cross-section is large, hence they have much less resistance as compared to the thin tungsten filament of an electric bulb.

(48) How much energy is consumed by 2 kW AC when used for 2 hour?
Answer:
Electric energy E = P x t
= 2 kW x 2 hour = 4 kWh
Where, 1 kWh = 3.6 x 10⁶ J

(49) A wire of resistivity p is pulled to double its length. What will be its new resistivity?
Answer:
Resistivity of wire remains the same as resistivity p depends on the nature of material and temperature.

(50) How does the resistance of an ohmic < conductor depend on the applied voltage?
Answer:
Resistance R is a constant, so it does not depend upon the applied voltage.

(51) What causes the potential difference between the two terminals of a cell?
Answer:
Chemical reactions taking place within a cell result in excess of electrons at the one terminal and deficiency of electrons at the other terminal.

(52) Name and define the smallest commercial unit of electricity.
Answer:
The smallest commercial unit of electricity is the watt-hour (Wh).
It is defined as the amount of electric energy consumed by an electrical device of power rating 1 W in one hour.

(53) If the current I through a resistor is increased by 100 % (assume that the temperature of the resistor remains unchanged), find the percentage increase in power dissipated.
Answer:
Power dissipated P = I²R
Current after increased by 100% = I’
= I + \(\frac { 100 I }{ 100 }\)
= 2 I
So, new power P’ = I’²R = (2I)²R = 4I²R
∴ Percentage increase in power dissipation
= \(\frac{P^{\prime}-P}{P}\) x 100 %
= \(\frac{4 I^2 R-I^2 R}{I^2 R}\) x 100 %
= \(\frac{I^2 R(4-1)}{I^2 R}\) x 100 %
= 3 x 100%
= 300 %

Value Based Questions With Answers

Question 1.
Medha observed that the tubelights in the corridor of her school were always switched on the whole day. She brought the matter to the notice of her class teacher who talked to the Principal about it. The Principal took immediate action.

1. Medha helped this way to reduce air pollution. Explain how.
2. What values do you learn from Medha in this episode?
3. What steps can the school take to get electricity consumption reduced?

Answer:

1. Power / Electricity production involves burning of fossil fuel. Electricity saved is fuel saved, less fuel burnt means less air pollution.
2. Courage, appreciation and protection of environment.
3. (i) Students should switch off the lights while going out of their class, (ii) they should not waste water as it also involves the use of electricity many a times.

Question 2.
Shalini is a student of class X. Her mother was preparing tea in an old electric kettle having metal case. When she switched on the electric kettle, she got a severe electric shock. Shalini put off the main switch quickly and found that the connecting cord was torn, where her mother touched the metal case of the kettle. She also found that the red and black wires of the connecting cord were firmly connected to the two lower terminals of the power plug but S the green wire of the cord was not connected to the upper terminal of the plug.

Shalini replaced the torn connecting cord and also connected the three wires of the cord firmly to the power plug terminals.

On the basis of the above passage, answer the following questions :

1. Why did Shalini switch off the main switch quickly?
2. Which wire red, black or green, touched the metal case of electric kettle when Shalini’s s mother got electric shock?
3. What values are displayed by Shalini in this incident?

Answer:

1. Shalini switched off the main switch to cut-off the electricity supply to the faulty s’ kettle in order to save her mother.
2. Red wire which is at high potential of 220 V was touching the metal case of electric kettle.
3. The values displayed by Shalini are :
   • • Presence of mind.
     • Concern for her mother.
     • Knowledge of household wiring and daily life activities.

Question 3.
Bharat was doing an experiment by using an ammeter. Suddenly, it fell from his hand and broke. He was afraid, that he might be scolded and threaten by his teacher. His classmate advised him not to tell the teacher, but he refused and told his teacher. On listening to him patiently, the teacher did not scold him and threaten as it was just an accident and used the opportunity to show the internal structure of the ammeter to the whole class.

On the basis of above passage, answer the following questions :

1. What values do you learn from Bharat?
2. What is the use of ammeter? How is it connected in the circuit?
3. State the aim of any one experiment, where Bharat could have used the ammeter.

Answer:

1. The values learnt from Bharat are honesty, confidence and courage.
2. An ammeter is used to measure the electric current flowing in a circuit. It is always connected in series in a circuit.
3. Bharat could have used the ammeter to verify the Ohm’s law.

Question 4.
Mitali’s mother was cooking in the kitchen for guests. Mitali saw her mother had plugged in microwave, hot plate and food processor on the same plug point. She immediately switched off the plug and removed all the plugs and re-plugged them in separate individual plugs.

1. What happens when we use too many electrical devices plugged in one power point?
2. What is the power of a device?
3. What value did Mitali display in the above act?

Answer:

1. Plugging too many devices in one power point causes overloading which can lead to electrical fire.
2. The power of an electrical device is the amount of electric energy used by it per unit time.
3. Mitali showed the values of awareness and responsibility.

Question 5.
Vishva noticed in a dhaba around 50 bulbs, each of 100 W all were glowing. She calculated the cost of electricity consumed in one hour and told the dhaba owner to reduce the expenses and at the same time save electricity by using CFL bulbs instead of ordinary filament-type bulbs.
(1) What would be the cost of electric energy if 50 bulbs, each of 100 W, are used for one hour? 1 unit costs ₹ 5.
(2) What is a CFL?
(3) What value did Vishva display in the above case?
Answer:
(1) The cost of electricity consumed when 50 bulbs are used for 1 hour
= (number of units consumed) x ₹ 5 per unit
= (50 x \(\frac { 100 }{ 1000 }\) kWh x 1 h) x \(\frac { ₹ 5 }{ 1 unit }\)
= (5 k Wh) x \(\frac { ₹ 5 }{ 1 unit }\)
= ₹ 25

(2) A CFL is a compact fluorescent lamp.

(3) Vishva displayed responsible behaviour, awareness as a citizen, critical thinking and analysis.

Practical Skill Based Questions With Answers

Question 1.
The scales of an ammeter and a voltmeter are shown below:

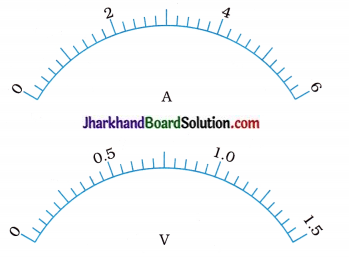
(1) What is the range of the ammeter and the range of the voltmeter?
(2) Find the least count of the ammeter and the voltmeter.
Answer:
(1) The range of the ammeter is 6 A and the range of the voltmeter is 1.5 V.

(2) The least count of the ammeter

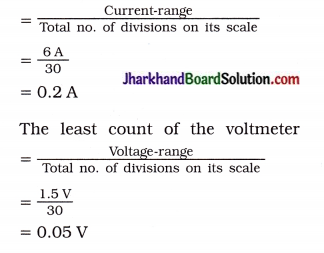
Question 2.
See the figure given below and find the readings of ideal ammeter and ideal voltmeter. (There is no zero error.)

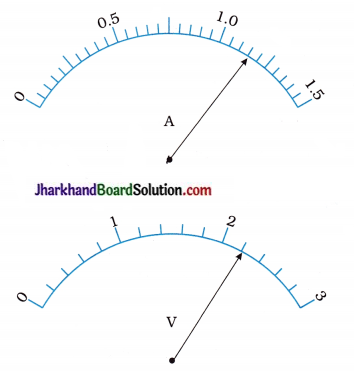
Answer:
The reading of the ammeter = 1.15 A. The value of 1 division on the scale of the ammeter (the least count) is \(\frac { 1.5 }{ 30 }\) = 0.05 A and its pointer shows 23 divisions on its scale. Hence, the ammeter reading = 23 x 0.05 A = 1.15 A.
The reading of the voltmeter = 2.2 V
The value of 1 division on the scale of the voltmeter (the least count) is \(\frac { 3 }{ 15 }\) = 0.2 V and its pointer shows 11 divisions on its scale. Hence the voltmeter reading 11 x 0.2 = 2.2V

Question 3.
In a given ammeter, a student observes that the needle indicates 17 divisions in the ammeter when performing an experiment to verify Ohm’s law. If the ammeter has 10 divisions between 0 and 0.5 A, then what is the value of 17 divisions?
Answer:
Since the ammeter has 10 divisions between 0 and 0.5 A, its least count or Vale of 1 division = \(\frac { 0.5A}{ 10 }\) = 0.5 A
Therefore, the value of 17 divisions
= 17 (0.05 A) = 0.85 A

Question 4.
Four resistors, each of 10 Ω, are connected to form a square as shown in the given figure. Find the equivalent resistance between the opposite corner A and C and between two points on any one side (AB).

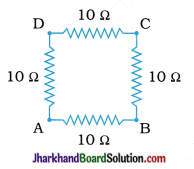
Answer:
(i) Resistance in the path
ADC = 10 Ω + 10 Ω = 20 Ω
Resistance in the path
ABC = 10 Ω + 10 Ω = 20 Ω
Now, \(\frac{1}{R_{\mathrm{AC}}}=\frac{1}{20}+\frac{1}{20}=\frac{1}{10}\)
∴Equivalent resistance,
R_AC = 10 Ω

(ii) Resistance in the path ADCB
= 10 Ω + 10 Ω + 10 Ω = 30 Ω.
The resistance of 30 Ω is in parallel with AB (= 10 Ω).
Now, \(\frac{1}{R_{\mathrm{AB}}}=\frac{1}{30}+\frac{1}{10}\)
= \(\frac{1+3}{30}=\frac{4}{30}\)
∴ Equivalent resistance,
R_AB = \(\frac { 30 }{ 4 }\) = 7.5 Ω

Question 5.
In the circuit shown in figure given below and find the current recorded by the ammeter (A).

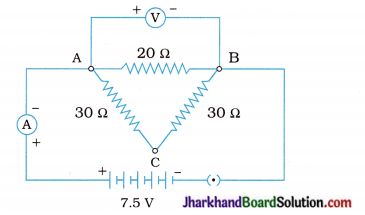
Answer:
Since resistance, AC = 30 Ω and resistances CB = 30 Ω. resistance in the path ACB = 30 Ω + 30 Ω = 60 Ω.
Further, 60 Ω (in the path ACB) and 20 Ω (in the path AB) are in parallel.
Hence, effective resistance (R) between A and B is given by
\(\frac{1}{R}=\frac{1}{60}+\frac{1}{20}=\frac{1+3}{60}=\frac{4}{60}=\frac{1}{15}\)
∴ R = 15 Ω
∴Current I = \(\frac { 7.5V }{ 15Ω }\) = 0.5 A
This is the current recorded by the ammeter.

Question 6.
Read the following information:
(1) The resistivity of copper is lower than that of aluminium which in turn is lower than that of constantan.
(2) Six wires labelled as A, B, C, D, E and F have been designed as per the following parameters :

Wire	Length	Diameter	Material	Resistance
A	l	2d	Aluminium	RA
B	2l	d/2	Constantan	RB
C	3l	d/2	Constantan	Rc
D	l/2	3d	Copper	RD
E	2l	2d	Aluminium	RE
F	l/2	4 d	Copper	RF

Answer the following questions using the above data :
(1) Which of the wires has maximum resistance and why?
(2) Which of the wires has minimum resistance and why?
(3) Arrange R_A, R_B and R_C in ascending order of their values. Justify your answer.
Answer:
(1) The wire C has maximum resistance because it has maximum length, least thickness and highest resistivity.

(2) The wire F has the minimum resistance, since it has least length, maximum thickness and least resistivity. (Using, R = ρ\(\frac { l }{ A }\))

(3) R_C > R_E > R_A
(Using relation, R = ρ\(\frac { l }{ A }\) and comparision)

Question 7.
n resistors, each of resistance R are first connected in series and then in parallel. What is the ratio of the total effective resistance of the circuit in the series to the parallel combination?
Answer:
In the series combination, R_s = nR
In the parallel combination, R_p = \(\frac { R }{ n }\)
∴ \(\frac{R_{\mathrm{s}}}{R_{\mathrm{p}}}=\frac{n R}{R / n}\) = n²

Question 8.
Find out the readings of ammeter and voltmeter in the circuit given below:

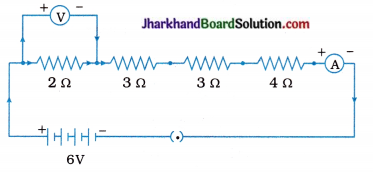
Answer:
Equivalent resistance R = (2 + 3 + 3 + 4)Ω
= 12 Ω
Total current drawn = Ammeter reading
= \(\frac { V }{ R }\)
= \(\frac { 6 }{ 12 }\) = 0.5 A
Voltmeter reading = IR₁ = 0.5 x 2 = 1 V

Question 9.
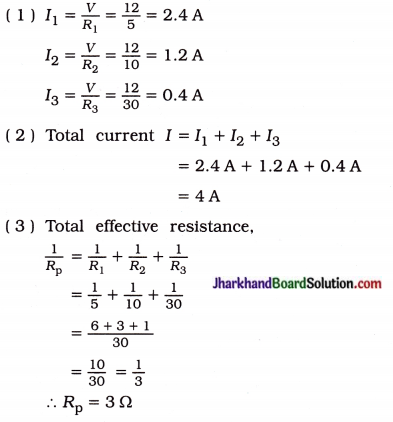
In the given circuit diagram, calculate :
(1) the value of current through each resistor
(2) the total current in the circuit
(3) the total effective resistance of the circuit.

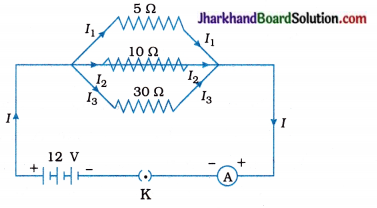
Answer:

Question 10.
Show four different ways in which three resistors of Y ohm, each may be connected in a circuit. In which case is the equivalent resistance of the combination :
(1) Maximum?
(2) Minimum?
Answer:

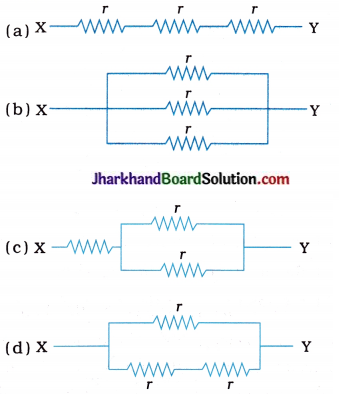
(1) Circuit (a) has maximum resistance

(2) Circuit (b) has minimum resistance

Question 11.
Find the current drawn from the battery by the network of four resistors shown in the figure.

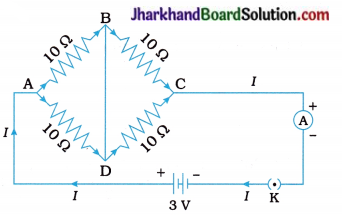
Answer:
Two combinations of 3V two parallel resistors of 10 Ω each are connected in series.

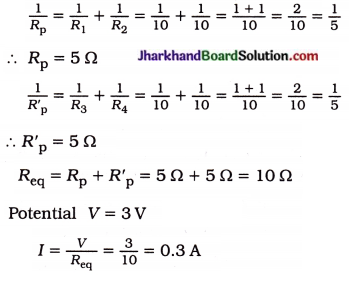
Question 12.
Find the equivalent resistance across the ends A and B of this circuit.
Answer:

\(\frac{1}{R_{1,2}}=\frac{1}{R_1}+\frac{1}{R_2}=\frac{1}{2}+\frac{1}{2}=\frac{2}{2}\) = 1
∴ R_1,2 = 1 Ω
Similarly R_3,4 = 1 Ω
R_5,6 = 1 Ω
R_7,8 = 1 Ω
Now R_1,2 and R_3,4 are connected in series
R_1,2,3,4 = R_1,2 + R_3,4 = 1 + 1 = 2Ω
Also, R_5,6 and R_7,8 are connected in series
R_5,6,7,8 = R_5,6 + R_7,8 = 1 + 1 = 2Ω
Now, equivalent resistance
\(\frac{1}{R_{\mathrm{eq}}}=\frac{1}{R_{1,2,3,4}}+\frac{1}{R_{5,6,7,8}}\)
= \(\frac{1}{2}+\frac{1}{2}=\frac{2}{2}\) = 1
∴ R_eq = 1 Ω

Question 13.
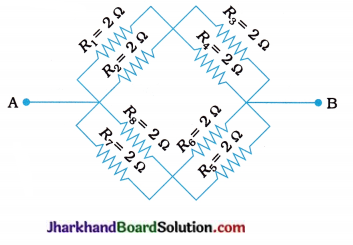
Five resistors are connected in a circuit as shown. Find the ammeter reading when the circuit is closed.

Answer:
Let the resistance between the points B and D be x.
∴ x = \(\frac{3 \Omega \times 6 \Omega}{3 \Omega+6 \Omega}\)
Now, the resistance of the entire circuit is
R = 0.5 Ω + x + 0.5 Ω
= 0.5 Ω + 2.0 Ω + 0.5 Ω
= 3.0 Ω
∴ Ammeter reading = \(\frac { 3 V }{ 3 Ω }\) = 1.0 A.

Question 14.
Draw a diagram of an electric circuit containing a cell, a key, an ammeter, a resistor of 2 Ω in series with a combination of two resistors (4 Ω each) in parallel and a voltmeter across the parallel combination.
Will the potential difference across the 2 Ω resistor be the same as that across the parallel combination of 4 Ω resistors? Give the reason.
Answer:
If R_p is the effective resistance of two resistors of 4 Ω each in parallel, then
\(\frac{1}{R_{\mathrm{p}}}=\frac{1}{4}+\frac{1}{4}=\frac{2}{4}=\frac{1}{2}\)
∴ R_p = 2 Ω

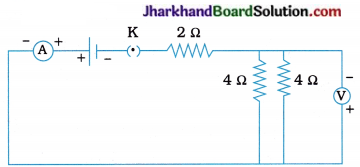
Since, the combined parallel resistance is also 2 Ω, the potential difference across 2 Ω resistor will be the same as that across the parallel combination of two 4 Ω resistors.

Question 15.
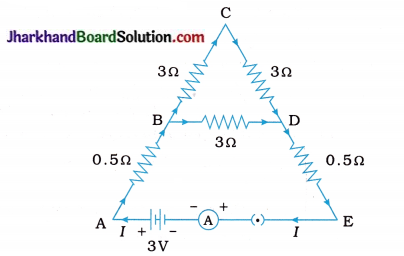
A current of 1A flows in a series circuit containing an electric lamp and a conductor of 5 Ω connected to a 10 V battery. Calculate the resistance of the electric lamp.

Now if a resistance 10 Ω is connected in parallel with this series combination, what change (if any) in current flowing through 5 Ω conductor and potential difference across the lamp will take place Give the reason.
Answer:

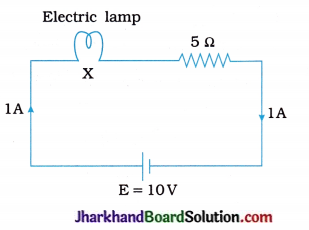
As, I = \(\frac{E}{R_1+R_2}\)
1 = \(\frac { 10 }{ x+5 }\)
or
∴ x = 5 Ω is the resistance of the lamp.
When a resistor of resistance 10 Ω is connected in parallel with x + 5 = 10 Ω, the total resistance R_p of the arrangement is given by,
\(\frac { 10 }{ x+5 }\)
= \(\frac { 1 }{ 5 }\)
∴ R_p = 5 Ω
∴ The current in circuit = \(\frac{E}{R_{\mathrm{p}}}\)
= \(\frac { 10 }{ 5 }\)
= 2 A
This current (2 A) divides eΩually between 10 Ω and (resistance of lamp + 5 Ω).

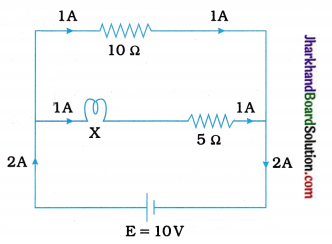
∴ The current through the lamp will be again 1 A, i.e., it remains the same.
The potential difference across the lamp will be 1 × x = 1 x 5 = 5V.
Therefore, the potential difference across the lamp will remain the same.

Memory Map:

Students must go through these JAC Class 10 Science Notes Chapter 11 Human Eye and Colourful World to get a clear insight into all the important concepts.

JAC Board Class 10 Science Notes Chapter 11 Human Eye and Colourful World

→ Human eye (eyeball) : The human eye is one of the most valuable and sensitive sense organs in our body. It enables us to see the wonderful world and colours around us. The human eye is like a camera. The eyeball is approximately spherical in shape with a diameter of about 2.3 cm.

The main parts of the human eye are as follows:

• Cornea
• Iris
• Pupil
• Crystalline lens
• Ciliary muscles
• Retina
• Optic nerves
• Aqueous humour and
• Vitreous humour

→ The ability of the eye lens to adjust its focal length, so that image of a nearby as well as distant objects can be focussed on the retina and hence seen comfortably and distinctly is called the power of accommodation of the eye.

→ The minimum distance at which an object can be seen most distinctly without strain is called the least distance of distinct vision or the near point of the human eye. For a young adult with normal vision, the near point is about 25 cm.

→ The farthest point up to which the eye can see an object clearly is called the far point of the eye. It is infinity for a normal eye.

→ A person with normal vision can see objects clearly that are between 25 cm and infinity from the eye.
Ciliary muscles hold the eye lens in position and help in changing (modifying) the curvature of the eye lens and hence the focal length of the eye lens.

→ Defects of vision and their correction:
(i) Myopia or near-sightedness: A person with myopia can see nearby objects clearly but cannot see distant objects distinctly. This defect may arise due to (1) excessive curvature of the eye lens or (2) elongation of the eyeball. This defect is corrected by using a concare lens of suitable power.

(ii) Hypermetropia or far-sightedness : A person with hypermetropia can see distant objects clearly but cannot see nearby objects distinctly. This defect arises due to (1) the focal length of the eye lens is increased due to least curvature of the eye lens, or (2) the length of the eyeball is shortened (becomes shorter than normal). This defect is corrected by using a convex lens of suitable power.

(iii) Presbyopia (also called old age hyper-metropia) : Presbyopia is the defect of vision in which aged people find it difficult to see the nearby objects comfortably and distinctly without spectacles.

It arises due to the gradual weakening of the ciliary muscles and diminishing flexibility of the eye lens. This defect can be corrected by using a convex lens of suitable power. Sometimes, a person may suffer from both myopia and hypermetropia. Such persons have to use bifocal lenses.

(4) Cataract: The crystalline lens of people at old age becomes milky and cloudy. This condition is called cataract. It is possible to restore vision through a cataract surgery.

→ Refraction of light through a prism: When light is refracted through a triangular glass prism, the emergent ray makes an angle with the incident ray. This angle is called the angle of deviation. It depends on the angle of incidence, the refractive index of the glass and the angle of the prism.

→ Dispersion of light: The splitting of light into its component colours is called dispersion. The phenomenon of a splitting of white light into its seven constituent colours is called dispersion of white light.

Prism disperses white light into its constituent colours. The sequence of colour from bottom to top is violet, indigo, blue, green, yellow, orange and red. (Remember: vibgyor)

The band of the coloured components of a light beam is called its spectrum. The band of seven colours obtained from the splitting of white light is called spectrum of white light.

→ Reason for dispersion of light: Actually white light is composed of seven colours as VIBGYOR. Now different colours of white light have different wavelengths. They travel with the same speed in vacuum and air but in any other medium they travel with different speeds.

The absolute refractive index of a medium is n_m = \(\frac { c }{ v }\) As \(v_{\text {violet }}<v_{\text {red }}, n_{\text {violet }}>n_{\text {red }}\). Hence, the violet light bends the most while the red light bends the least.

As the refractive index n of glass of prism for different colours is different, they deviate through different angles with respect to incident ray on passing through prism.

→ Rainbow: A rainbow is a natural spectrum appearing in the sky after a rain shower. Rainbow is caused by dispersion of sunlight on refraction by tiny water droplets, present in the atmosphere. It is always formed in the direction opposite to that of the Sun. In this case there is dispersion, internal reflection and refraction of light.

→ Atmospheric refraction : Atmospheric refraction is the phenomenon of bending of light passing through the earth’s atmosphere.

Some phenomena based on atmospheric refraction are :

• Twinkling of stars
• Advanced sunrise
• Delayed sunset
• The stars seem higher than they actually are (i.e., the displacement of stars).
• The Sun appears oval (or flattened) at sunrise and sunset, but appears circular at noon, (i.e., the apparent change in the shape of the sun)

→ Scattering of light: The deflection of light by minute particles and molecules / atoms in all directions is known as scattering of light. The amount of scattering (i.e., extent of deflection) of light depends on the frequency (colour) of light and the size of the particles responsible for scattering.

Very fine particles scatter mainly blue light. Bigger particles scatter light of longer wavelength. If the scattering particles are very large, the scattered light appears white as light of all wavelengths in the visible region is scattered.

Some examples of scattering of light are:

• Tyndall effect
• Blue colour of clear sky
• The signal lights for danger are red in colour.
• Reddish colour of the Sun at sunrise and sunset.
• Reddish appearance of the full moon at the time of rising and setting.