Prasanna

Students must go through these JAC Class 10 Science Notes Chapter 10 Light Reflection and Refraction to get a clear insight into all the important concepts.

JAC Board Class 10 Science Notes Chapter 10 Light Reflection and Refraction

→ Light: Light is an electromagnetic radiation, which produces the sensation of sight in our eyes.

• Light is a form of energy that travels in the form of waves.
• These waves do not require a material medium for their propagation. They are non-mechanical waves.
• These waves travel at a speed of 3 x 10⁸ m s^-1 in vacuum.
• The wavelength of visible light ranges from 4 x 10^-7m to 8 x 10^-7m.

→ Image: When a number of rays starting from a point on an object, after reflection or refraction, meet at another point or appear to meet (i.e., give illusion of meeting at another point), then the second point is called the image of the first point. A group of such rays of light is called a beam of light.

Ray of light: A straight-line path joining one point to another in the direction of propagation of light is known as a ray of light.

• The image formed is called a real image, if the rays actually meet after reflection or refraction. It can be obtained on a screen.
• If the rays do not meet actually at a point, but appear to meet, after reflection or refraction, then the image is called a virtual image. It cannot be obtained on a screen.
• When a parallel beam of light is incident on a shining plane or smooth surface, the beam remains parallel after reflection in a specific direction. Such a reflection is called regular reflection.
• Example: the reflection of light by a plane mirror.
• In case of irregular reflection, the reflected beam of light does not remain parallel in specific direction but spreads over a wide region.
• Example : reflection from a book, a table, a chair, etc.

→ Laws of reflection:

• The angle of incidence is equal to the angle of reflection, i.e., i = r.
• The incident ray, the normal to the mirror at a point of incidence and the reflected ray, all lie in the same plane.

→ Image formation by a plane mirror:

• The image formed by a plane mirror is always virtual and erect.
• The image formed is as far behind the mirror, as the object is in front of it.
• The size of the image is equal to that of the object.
• The image formed is laterally inverted, i.e., the left side of the object seems to be the right side of the image and vice versa.

(The phenomenon, by which the left side of the object becomes the right side of the image and right side of the object becomes the left side of the image, is called lateral inversion.)

→ Spherical mirrors: A mirror whose reflecting surface is a part of a hollow sphere is called a spherical mirror. The reflecting surface of a spherical mirror is curved inwards or outwards.
The curved mirrors are of two types:

•  Concave mirror and
•  Convex mirror.

→ Concave mirror: A spherical mirror with reflecting surface curved inwards is called a concave mirror. Concave mirror forms either a real or virtual image of the object depending on the object distance.

→ Convex mirror: A spherical mirror with reflecting surface curved outwards is called a convex mirror. Convex mirror forms a virtual image of the object for all object distances.

→ Terminology used in respect of spherical mirrors:
(1) Pole (P): The centre of the reflecting surface of a sperical miror is a point called the pole (P) of the mirror.

(2) Centre of curvature (C) : The centre of curvature of a spherical mirror is the centre of the hollow sphere, of which the reflecting surface of the spherical mirror forms a part.

(3) Radius of curvature (R): The radius of curvature of a spherical mirror is the radius of the hollow sphere, of which the reflecting surface of the spherical mirror forms a part.

(4) Aperture : The diameter of the edge of the reflecting surface of a spherical mirror is called the aperture of the mirror.

(5) Principal axis : The imaginary straight¬line passing through the pole (P) and the centre of curvature (C) of a spherical mirror is called the principal axis of the mirror.

(6) Principal focus (F): The point on the principal axis of a concave mirror, at which rays of light incident on the mirror in the direction parallel to the principal axis (actually) meet / intersect after reflection from the mirror is called the principal focus (F) of the concave mirror. When rays parallel to the principal axis are incident on a convex mirror, the reflected rays appear to come from a point on the principal axis. This point is called the principal focus of the convex mirror.

(7) Focal length (f): The distance between the pole (P) and the principal focus (F) of a spherical mirror is called the focal length (f).

→ Selection of rays for locating the image formed by a spherical mirror:

• A ray parallel to the principal axis, after reflection, will pass through the principal focus (F) in case of a concave mirror or appear to diverge from the principal focus (F) in case of a convex mirror.
• A ray passing through the principal focus (F) of a concave mirror or a ray which is directed towards the principal focus of a convex mirror, after reflection, will emerge parallel to the principal axis.
• A ray passing through the centre of curvature of a concave mirror or directed towards the centre of curvature of a convex mirror, after reflection, is reflected back along the same path.
• A ray incident obliquely to the principal axis, towards a point P (pole of the mirror), on the concave mirror or a convex mirror is reflected obliquely, following the laws of reflection.

Out of these four types of rays, only two types of rays are necessary to locate the position of an image of a point object.

→ Image formation by a Concave mirror:

Position of the object	Position of the image	Size of the image	Nature of the image
At infinity	At the focus F	Highly diminished, point-sized	Real and inverted
Beyond C	Between F and C	Diminished	Real and inverted
At C	At C	Same size	Real and inverted
Between C and F	Beyond C	Enlarged	Real and inverted
At F	At infinity	Highly enlarged	Real and inverted
Between P and F	Behind the mirror	Enlarged	Virtual and erect

→ Image formation by a Convex mirror:

Position of the object	Position of the image	Size of the image	Nature of the image
At infinity	At the focus F, behind the mirror	Highly diminished, point-sized	Virtual and erect
Between infinity and the pole P of the mirror	Between P and F, behind the mirror	Diminished	Virtual and erect

→ New Cartesian sign convention for reflection by spherical mirrors:

• The object is always placed to the left of the mirror. This implies that the light from the object falls on the mirror from the left-hand side.
• All distances parallel to the principal axis are measured from the pole of the mirror.
• All the distances measured to the right of the origin (along + X-axis) are taken as positive while those measured to the left of the origin (along-X-axis) are taken as negative.
• The distances measured perpendicular to and above the principal axis of the mirror (along + Y-axis), are taken as positive.
• The distances measured perpendicular to and below the principal axis (along -Y-axis), are taken as negative.

→ Magnification prpduced by a spherical mirror: The ratio of the height of the image to the height of the object is called the magnification (m). It is the relative extent to which the image of the object is magnified with respect to the size of the object.

→ Refraction: When a ray of light travels obliquely from one transparent medium to another, its speed changes. Therefore, at the boundary separating the two media, there occurs a change in its direction of propagation. This phenomenon is called refraction of light.

→ Laws of refraction:
(1) The incident ray, the refracted ray and the normal to the interface of two transparent media at the point of incidence, all lie in the same plane.

(2) The ratio of the sine of angle of incidence to the sine of angle of refraction is a constant, for the light of a given colour and for the given pair of media. This law is known as Snell’s law of refraction. (This is true for angle 0° < i° < 90°)
If i is the angle of incidence and r is the angle of refraction,
\(\frac { sin i }{ sin r }\) = constant = n₂₁
where, n₂₁ is known as the refractive index of medium 2 with respect to medium 1.
The above equation is the mathematical representation of the Snell’s law.

→ Absolute Refractive Index: The ratio of the speed of light in vacuum (c) to the speed of light in medium (v), is called the absolute
refractive index of the medium.
n_m = \(\frac { c }{ v }\)
Absolute refractive index of any medium is always more than 1.

→ Relative Refractive Index: The ratio of the speed of light v1 in medium 1, to the speed of light v₁ in medium 2, is called the relative refractive index of medium 2 with respect to medium 1 and is represented by the symbol n₂₁. v₁ and v₂ correspond to the same frequency of light.
n₂₁ = \(\frac{v_1}{v_2}\)
Also, n₂₁ = \(\frac{n_2}{n_1}\) where, n₂ = \(\frac{c}{v_2}\) and n₁ = \(\frac{c}{v_1}\)
Here, v₁ is the speed of light in medium 1, v₂ is the speed of light in medium 2, n₁ is the absolute refractive index of medium 1 and n₂ is the absolute refractive index of medium 2, for the given frequency (given colour) of light.

→ Lateral shift: When a ray of light is refracted at two parallel refracting surfaces, the ray is shifted sideward. This sideward displacement of a ray of light is called lateral shift.

The amount of lateral shift depends upon the perpendicular distance between two parallel refracting surfaces as well as upon the angle of incidence and the refractive index of the second medium with respect to the first medium.

→ Terminology used in respect of lens:
(1) Centre of curvature (C): The centre of a transparent (glass) sphere, of which the curved surface of a lens forms a part is called the centre of curvature C of the respective spherical surface.
Lens has two centres of curvatures C₁ and C₂.

(2) Principal axis : The imaginary straight-line passing through the two centres of curvature C₁ and C₂ of a lens, is called the principal axis of the lens.

(3) Radius of curvature (R): The radius of a transparent (glass) sphere of which the curved surface of a lens forms a part is called the radius of curvature R of the respective spherical surface of the lens. Lens has two radii of curvature R₁ and R₂.

(4) Optical centre (O): The central point of a lens on the principal axis of the lens is called an optical centre O of the lens.

(5) Principal focus (F): When the rays parallel to the principal axis of a convex lens are refracted through the lens, they converge at a point on the principal axis. This point is called the principal focus F of the convex lens.

When the rays parallel to the principal axis of a concave lens are refracted through the lens, they appear to diverge from a point on the principal axis. This point is called the principal focus of the concave lens. A lens has two principal foci F₁ and F₂ on either side of the lens.

(6) Focal length (f): The distance of the principal focus from the optical centre of a lens is called the focal length f of the lens.

(7) Aperture : The effective diameter of the circular outline of a spherical lens is called the aperture of the lens.

→ Selection of rays for image formation by lens:

• A ray of light from the object, parallel to the principal axis, after refraction through a convex lens, passes through the principal focus on the other side of the lens.
• A ray of light from the object, parallel to the principal axis, after refraction through a concave lens, appears to diverge from the principal focus located on the same side of the lens.
• A ray of light passing through the principal focus, after refraction through a convex lens, will emerge parallel to the principal axis. A ray of light directed towards the principal focus on the other side of a concave lens, after refraction, will emerge parallel to the principal axis.
• A ray of light passing through the optical centre of a lens will emerge without any deviation. (This is true for a convex lens as well as a concave lens.)

From the above three types of rays any two types of rays will locate the position of the image.

→ Image formation by a Convex Lens:

Position of the object	Position of the image	Relative size of the image	Nature of the image
At infinity	At focus F2	Highly diminished, point-sized	Real and inverted
Beyond 2F1	Between F2 and 2F2	Diminished	Real and inverted
At 2F1	At 2F2	Same size	Real and inverted
Between F1 and 2F1	Beyond 2F2	Enlarged	Real and inverted
At focus F1	At infinity	Infinitely large or Highly enlarged	Real and inverted
Between focus F1 and optical centre O	On the same side of the lens as the object	Enlarged	Virtual and erect

→ Image formation by a Concave Lens:

Position of the object	Position of the image	Relative size of the image	Nature of the image
At infinity	At focus F1	Highly diminished, point-sized	Virtual and erect
Between infinity and optical centre O of the lens	Between focus F1 and optical centre O	Diminished	Virtual and erect

→ Sign convention for spherical lens : It is similar to that followed for spherical mirrors, but the optical centre of a lens is chosen as the origin of the co-ordinate system.

→ Magnification produced by a lens: The magnification (m) produced by a lens is defined as the ratio of the height of the image to the height of the object.

→ Power of a lens : The reciprocal of the focal length of a lens is called the power of the lens (P).
P = \(\frac { 1 }{ f }\)
SI unit: The dioptre (D)
1 D = 1 m^-1
The power of a convex lens is positive and that of a concave lens is negative.

Jharkhand Board JAC Class 10 Science Important Questions Chapter 14 Sources of Energy Important Questions and Answers.

JAC Board Class 10 Science Important Questions Chapter 14 Sources of Energy

Additional Questions and Answers

Question 1.
Distinguish between :
(1) Solar Cooker and Solar Cell Panel
Answer:

Solar Cooker	Solar Cell Panel
1. It converts solar energy into heat.	1. It converts solar energy into electricity.
2. It is mainly designed for cooking purpose, i.e., for domestic use	2. It is designed for delivering enough electricity for commercial use.
3. It consists of mirror, glass sheet, black wooden box.	3. It consists of large number of solar cells and silicon is used in solar cells.
4. It is useful for cooking only at certain times of the day.	4. The limitation of using solar energy is overcome by using it.

(2) Fossil fuels and Bio fuels
Answer:

Fossil Fuels	Bio Fuels
1. It is a conventional source of energy mainly used in industries and vehicles.	1. It is a conventional source of energy mainly for domestic use.
2. Coal, petroleum and natural gas are fossil fuels.	2. Wood, cow-dung cakes, biogas, etc. are bio fuels.
3. The fossil fuels are non-renewable and exhaustible source of energy.	3. Bio fuels are renewable source of energy in the form of biomass.
4. Fossil fuels are the major fuels used all over the world.	4. Bio fuels are mainly used in rural area.

Question 2.
Give scientific reasons for the following statements:
(1) From environmental point of view, we should reduce the use of fossil fuels.
Answer:
The fossil fuels are non-renewable source of energy and in limited reserves.

• The growing demand for energy at global level are largely meet by fossil fuels.
• Excessive burning of fossil fuels – coal and petroleum increases the level of air pollution. example:
• Carbon dioxide, sulphur dioxide and oxides of nitrogen are released in excess in atmosphere causing such air pollution.
• Carbon dioxide is a main greenhouse gas that causes global warming.
• Sulphur dioxide and oxides of nitrogen lead to acid rain which affects water and soil resources.

So, to reduce the adverse effects on environment, we should reduce the use of fossil fuels.

(2) Though hydropower is renewable source of energy, it creates certain problems.
Answer:
Hydropower is renewable source of energy because water gets refilled in reservoir during raining.

• High-rise dams are constructed to generate hydroelectricity.
• Such dams are constructed in hilly areas causing environmental changes.
• Large eco-systems are submerged under water and destroyed.
• The submerged vegetation decaying anaerobically releases large amount of greenhouse gas, i.e., methane.
• Large areas of agricultural land is also submerged. Many natives are thus displaced.
• Rural-tribal human races may lose their habitat.

So, Hydropower plant construction creates certain problems.

(3) The use of dung as raw material for biogas production is profitable than using it only as fertiliser or fuel.
Answer:
Animal dung is used as raw material c for production of biogas. It provides a safe and i efficient method of waste disposal.

• Besides it, by supplying biogas as a fuel gas, cooking, lighting, etc. we can save natural gas.
• The residual slurry can be used as excellent manure that is rich in nitrogen and phosphorous.

This natural fertiliser maintains soil fertility and agriculture practice becomes more economical.

(4) Inspite of the high cost, solar cells are widely used.
Answer:
A special grade silicon is used for making solar cells and silver is used for interconnection of cells in panel.

• This makes the solar cells expensive.
• But it converts solar energy into electricity and about 0.7 W of electricity is produced when exposed to the Sunlight.
• Solar cells and panels are widely used in artificial satellite, space probes like Mars orbiters, wireless transmission systems, radio or TV relay stations, traffic signals, calculators and in many toys.
• At domestic level, maximum use of solar energy can be achieved by using solar roof top thus saving electricity.
• So, solar cells are widely used.

(5) Generation of nuclear energy is hazardous.
Answer:
Nuclear fission and nuclear fusion are two processes used to generate nuclear energy.

• Radioactive heavy atoms such as uranium, plutonium or thorium are used in a nuclear fission process whereas hydrogen isotopes are used in a nuclear fusion.
• There is a risk of accidental leakage of nuclear radiation.
• There is a possibility of high risk of environmental contamination i.e., radioactive pollution.
• Radioactive waste is hazardous to human health and environment. Radiations which are emitted from the radioactive waste can cause mutation (Change in DNA) in living organisms.
• It creates various disorders and cancer in human beings.

So, generation of nuclear energy is hazardous.

Question 3.
Carefully observe the given diagram / chart and answer the questions based on it.
(1) Observe the following pie-chart showing the major sources of energy.

Questions:
(1) Identify ‘a’ and state where is it used as a fuel to generate electric energy through conversion of heat energy.
Answer:
Coal. It is used as a fuel in thermal power plant.

(2) Label ‘b’ and give the name of processes to generate it.
Answer:
Nuclear energy. The processes to generate nuclear energy are: nuclear fission and nuclear fusion.

(3) Label ‘c’ and state the name of any two place where such power plants are established.
Answer:
Hydropower. Hydropower plants are established at Tehri Dam on the Ganga river and Sardar Sarovar Project on the Narmada river.

(4) Refer to the pie-chart above and arrange the sources of energy in an ascending order.
Answer:
Nuclear < hydro < petroleum and natural gas < coal

Questions:

(1) Label a, b and c in given diagram.
Answer:
a – Digester, b – Slurry, c – Gas outlet

(2) How is biogas obtained from slurry?
Answer:
Slurry consists of bio-wastes / biomass and biogas can be obtained through decomposition by anaerobic microorganisms.

(3) Why is biogas considered as an excellent fuel?
Answer:
Biogas is considered as an excellent fuel because it burns without smoke, leaves no ash residue and has high heating capacity.

(4) Which are the gases present in biogas?
OR
What are components of biogas?
Answer:
Biogas mainly consists gases like methane, carbon dioxide, hydrogen and hydrogen sulphide.

(5) Which are abundant nutrients in the slurry left behind in a biogas plant?
Answer:
Nitrogen and phosphorous are abundant nutrients in the slurry left behind in a biogas plant.

Questions :
(1) Which metal is used in the panel for the interconnection of cells?
Answer:
Silver

(2) Which energy conversion occurs by using solar cells?
Answer:
Solar energy is converted into electricity.

(3) Write any two uses of solar cells for space science.
Answer:
Solar cells are used as a main energy source in an artificial satellites and space probes like Mars orbiters.

(4) If 12 solar cells are interconnected in the panel. How many watt of electricity can be produced from it when exposed to Sun?
Answer:
About 8.4 W

(5) Why is silicon used in a solar cells?
Answer:
Silicon is a natural semiconductor, easily obtained and easily modified to convert solar energy into electricity.

Objective Questions and Answers

Question 1.
Answer the following questions in short:
(1) Name the form of energy obtained from sea.
Answer:
The forms of energy obtained from sea are tidal energy, wave energy and ocean thermal energy.

(2) Which fuel is considered as a cleaner source for vehicles?
Answer:
CNG (Compressed Natural Gas)

(3) State the name of fuels that we are using.
Answer:
We are using wood, coal, petrol, diesel, CNG, kerosene, LPG, etc. as fuels.

(4) What is a source of energy?
Answer:
A source of energy is one which is capable of providing useful energy in sufficient amount.

(5) Which energy conversion occurs in a hydropower plant?
Answer:
In a hydropower plant, potential energy of stored water is converted into electrical energy.

(6) Which is a main constituent of biogas? What is its content?
Answer:
Methane gas is a main constituent of biogas and it is about 75 %.

(7) What are the conventional sources of energy?
Answer:
Fossil fuels, i.e., coal and petroleum are the conventional source of energy, which are used on a large scale.

(8) Name two elements that are used in manufacturing solar cell panels.
Answer:
Silicon, Silver

(9) Name the devices to harness solar energy.
Answer:
Solar cell, solar cooker, solar water heater.

(10) Where are the fossil fuels used directly?
Answer:
In gas stoves, vehicles and thermal power plants, fossil fuels are used directly.

(11) Why is charcoal used as a fuel?
Answer:
Charcoal is used as a fuel because it burns without flames is comparatively smokeless s and has a higher heat generation efficiency.

(12) Why is the term thermal power plant used?
Answer:
The term thermal power plant is used because in such power plants fuel is burnt to produce heat energy which is converted into electrical energy.

(13) State the name of any two greenhouse gases.
Answer:
CO₂ and CH₄

(14) Which fuels leave residue like ash on burning?
Answer:
Wood, charcoal and coal leave residue like ash on burning.

(15) How anaerobic microorganisms help in formation of fuel?
Answer:
Anaerobic microorganisms (Methanogenic bacteria) produce biogas by decomposition of complex compounds that present in cow-dung slurry.

(16) State two advantages of wind energy.
Answer:
Advantages of wind energy :

• It is an environment-friendly energy
• It is efficient source of renewable energy.

(17) What is the age of the sun? What will be its expected lifespan?
Answer:
The sun is 5 billion years old and another 5 billion years will its expected lifespan.

(18) Why is our demand for energy increasing day by day?
Answer:
Our demand for energy is increasing day by day because –

• We use machines to do more and more of our tasks, which needs more energy.
• Industrialisation improves our living standards. The energy requirement therefore constantly rises.

(19) Where is solar cell panels are mounted for domestic use? What is its advantages?
Answer:
The solar cell panels are mounted on specially designed inclined rooftops. More solar rays are incident over it, producing more electric
energy.

(20) Name the two oxides that causes acid rain.
Answer:
Oxides of nitrogen and sulphur cause acid rain.

(21) What is the minimum speed of wind required by a windmill to maintain the necessary speed of turbine in electric generator?
Answer:
More than 15 km/h

(22) Which are the renewable sources of energy?
Answer:
Hydropower, biomass, solar energy, energy from the sea, wind energy, etc. are renewable sources of energy.

Question 2.
Define: OR Explain the terms :
(1) Nuclear fission
Answer:
The process of splitting a heavy nucleus into smaller nuclei along with the release of large amount of energy is called nuclear fission.

(2) Nuclear fusion
Answer:
The process of joining lighter nuclei to make a heavier nucleus along with release of tremendous amount of energy is called nuclear fusion.

(3) Solar constant
Answer:
The solar energy reaching unit area on outer surface of the earth’s atmosphere which is exposed perpendicularly to the rays of sun at the average distance between the sun and earth is called solar constant.

(4) Geothermal energy
Answer:
The heat energy obtained in the form of steam from hot spots region is called geothermal energy.

(5) Biogas
Answer:
A fuel gas that is produced anaerobically from biomass is called biogas.

(6) Conventional sources of energy
Answer:
Those sources of energy which meet our major energy requirement are known as conventional sources of energy.

(7) Non-conventional sources at energy
Answer:
Those sources of energy which meet only a limited energy requirement are known as non-conventional sources of energy.

(8) Wind energy farm
Answer:
A number of windmills erected over a large area for the commercial use of wind energy is known as wind energy farm.

(9) Fossil fuels
Answer:
The fuels such as coal, petroleum and natural gas, that were formed millions of years ago from dead and fossiliged animals or plants in the ground are called fossil fuels.

Question 3.
Fill in the blanks:

1. Biogas obtained from decomposition of biomass by the activity of ……………… microorganisms.
2. Petroleum products are ……………… fuel.
3. Hydropower plants convert the ……………… of falling water into electricity.
4. When wood is burnt in a limited supply of oxygen, ……………… is left behind as a residue.
5. Manure obtained from biogas plant is rich in ……………… and ………………
6. ……………… plant is an efficient method of bio waste disposal.
7. ……………… is the best process to capture solar energy and convert it into biomass.
8. Use of ……………… mirror would be best for in solar cooker.
9. ……………… is abundant in nature but availability of its special grade is limited.
10. The hydrogen bomb is based on ……………… reaction.
11. India is ranked ……………… in harnessing wind-energy for the production of electricity.
12. The total ……………… of the universe remains constant because it can neither be created nor destroyed.

Answer:

1. anaerobic
2. fossil
3. potential energy
4. charcoal
5. nitrogen, phosphorous
6. Biogas
7. Photosynthesis
8. concave
9. Silicon
10. thermonuclear fusion
11. fifth
12. energy

Question 4.
State whether the following sentences are true or false:

1. The air pollution is caused by burning of fossil fuels.
2. The potential energy of flowing water gets, transformed into kinetic energy by collecting the water in dam.
3. In solar cookers, a plain mirror is used to converge the sunlight.
4. Fossil fuels are the major fuels used for generating electricity.
5. Submerged vegetation rots under anaerobic condition producing large amounts of methane.
6. Biogas is derived totally from animal biomass.
7. The level of water in the sea rises and falls due to the gravitational pull of the moon on the spinning earth.
8. Nuclear energy is the best alternative of fossil fuel because of its environment friendly nature.
9. The nuclear bomb is embedded in a deuterium and lithium containing substance.
10. The assembly of the solar cell may be pollution free but the actual operation of it may cause some environmental harm.
11. The energy sources that can be regenerated are called renewable energy sources.
12. Nuclear fusion reactions are the source of energy in the sun and other stars.

Answer:

1. True
2. False
3. False
4. True
5. True
6. False
7. True
8. False
9. True
10. False
11. True
12. True

Question 5.
Graph / diagram based question :
Which of the following graph is correct for Burning of fossil fuel → level of air pollution?

Answer:

Question 6.
Match the following:
(1)

Column I	Column II
1. Silicon	a. nuclear reactor
2. Hot-springs	b. leaves residue like ash
3. Fission of uranium atom	c. used in solar cells
4. Charcoal	d. geothermal energy

Answer:
(1 – c), (2 – d), (3 – a), (4 – b).

(2)

Column I	Column II
1. Fossil fuel	a. Plutonium, thorium
2. Biomass	b. coal, petroleum
3. Nuclear fuel	c. CNG
4. Clean fuel	d. wood, cow-dung cake

Answer:
(1 – b), (2 – d), (3 – a), (4 – c).

(3)

Column I	Column II
1. Geothermal energy plant	a. Traffic signals
2. Solar cell panels	b. New Zealand
3. Wind energy farm	c. Tehri dam
4. Hydropower plant	d. Kanyakumari

Answer:
(1 – b), (2 – a), (3 – d), (4 – c).

Question 7.
Select the correct alternative from those given below each questions:
1. Which of the following is a main component of biogas?
A. Methane
B. Hydrogen
C. Hydrogen sulphide
D. Oxygen
Answer:
A. Methane

2. Which fuel is used in thermal power plant?
A. Biomass
B. Fossil fuel
C. Wood
D. Charcoal
Answer:
B. Fossil fuel

3. Which of the following is the adverse effect caused by burning of fossil fuels?
A. Acid rain
B. Greenhouse effect
C. Both A and B
D. Agricultural land submerged
Answer:
C. Both A and B

4. Find greenhouse gases.
A. Hydrogen and hydrogen sulphide
B. Sulphure dioxide and nitrogen
C. Nitrogen and hydrogen
D. Methane and carbon dioxide
Answer:
D. Methane and carbon dioxide

5. Which of the following fuel does not leave ash-like residue while burning?
A. Wood
B. Charcoal
C. Biogas
D. Coal
Answer:
C. Biogas

6. Which one of the following is a non-renewable source of energy?
A. Bio fuel
B. Fossil fuel
C. Wind power
D. Tidal energy
Answer:
B. Fossil fuel

7. Which of the following exploits energy due to temperature difference at surface water and water at depth?
A. Tidal energy
B. Wave energy
C. Ocean thermal energy
D. All of the given
Answer:
C. Ocean thermal energy

8. Statement A: We need to look for more and more source of energy.
Reason R: There are only limited reserves of fossil fuels.
Which option is correct for statement A and reason R?
A. Both A and R correct, R is explanation of A.
B. Both A and R correct, R is not explanation of A.
C. A is correct, R is incorrect.
D. A is incorrect, R is correct.
Answer:
A. Both A and R correct, R is explanation of A.

9. Charcoal is considered as a better fuel compared to wood because …
A. it burns without flames.
B. it is comparatively smokeless.
C. it has a higher heat generation efficiency.
D. all of the given.
Answer:
D. all of the given.

10. From which of following, electricity can be generated without the use of turbine?
A. Solar energy
B. Tidal energy
C. Geothermal energy
D. Wave energy
Answer:
A. Solar energy

11. Which is the fundamental reaction in nuclear weapon for destructive purposes?
A. Fusion chain
B. Fission chain
C. Radiation chain
D. Thermal chain
Answer:
B. Fission chain

12. By which of the following greenhouse effect can be achieved in a solar cooker?
A. Black surface
B. Concave mirror
C. Convex mirror
D. Covered glass plate
Answer:
D. Covered glass plate

13. Which one of following is used as a main source of energy in artificial satellite?
A. Fossil fuel
B. Uranium
C. Solar cells
D. Gravitation pull
Answer:
C. Solar cells

14. Statement X: Ocean thermal energy conversion plant can operate if the temperature difference is 20°C or more between the water at surface and water at depth. Statement Y: Wind energy farm can generate electricity only if the wind speed should be higher than 15 km/h.
Which is correct option for statement X and Y?
A. X is correct, Y is incorrect.
B. Both X and Y are correct.
C. X is incorrect, Y is correct.
D. Both X and Y are incorrect.
Answer:
B. Both X and Y are correct.

15. Which pf the following is the ultimate source of energy on the earth?
A. Solar
B. Wind
C. Ocean
D. Fossil fuel
Answer:
A. Solar

16. Which of the following is an environmental friendly source of energy?
A. Wind energy
B. Fossil fuel
C. Nuclear energy
D. Solar energy
Answer:
A. Wind energy, Solar energy

17. Statement A: Many of the sources ultimately derive energy from the sun.
Reason R: Nuclear fusion reactions are the source of energy in the sun.
Which option is correct for statement A and reason R?
A. Both A and R correct, R is explanation of A.
B. Both A and R correct, R is not explanation of A.
C. A is correct, R is incorrect.
D. A is incorrect, R is correct.
Answer:
B. Both A and R correct, R is not explanation of A.

Question 8.
Answer as directed (Miscellaneous):
(1) Write full form of CNG, OTEC; MeV
Answer:
CNG – Compressed Natural Gas
OTEC-Ocean Thermal Energy Conversion
MeV – Mega-electron Volts

(2) State the units of Solar constant, Electricity, Energy.
Answer:
Solar constant – kW / m²
Electricity – MW
Energy – eV or Joules

(3) Find mismatched pair :
I. Fossil fuel – Air pollution
II. Wind energy farm – Large area of land
III. Biogas – Aerobic microorganisms
IV. Geothermal energy – Hot springs
Answer:
III. Biogas – Aerobic microorganisms

(4) Find correct sequence for following events :
I. Winds to blow
II. Kinetic energy of huge waves near the sea-shore
III. Solar radiation
IV. Electricity generated
V. Waves generated
Answer:
III → IV → II → IV

(5) Find mismatched pair :
I. Bio fuel-wood, cow-dung cake
II. Solar cell panel – Silicon, copper
III. Windmill – Large fan, turbine of generator
IV. Nuclear energy – Fission and Fusion reaction
Answer:
II. Solar cell panel – Silicon, copper

(6) Sequentially arrange the events that occur in biogas plant.
p. activity of anaerobic microbes
q. The slurry left behind is used as manure
r. cow-dung and water is mixed and this slurry is fed into the digester
s. Methane gas is generated
t. Breakdown of complex compounds of the cow-dung slurry.
Answer:
r → p → t → s → q

Value Based Questions With Answers

Question 1.
Your father decided to fit CNG kit in his petrol car. Your mother is a working woman. Your father has managed their working time in such a way that he took your mother with him.

Your school is just 3 km away from your home. Your father also insists you to use bicycle instead of fuel using vehicle for going to school.
Questions:

1. Why CNG is prefered as a fuel?
2. Why we need to conserve fossil fuels?
3. What value is reflected in the above family?
4. State any two disadvantages of petrol as a fuel.

Answer:

1. CNG is a cleaner fuel than petrol and it is economical. It is less polluting.
2. The fossil fuels are non-renewable and exhaustible sources of energy. So we need to conserve them.
3. This family shows the values of responsibility to environment and they contribute their efforts to save fuels.
4. Disadvantages of petrol as a fuel: (i) It is expensive, (ii) It causes air pollution.

Question 2.
A group of students visited to a village. They noticed that village people uses dung cakes, agricultural wastes, etc. as a fuel. Students found smoke and ash particles in the environment. They requested the sarpanch to install a biogas plant and insist the people to collect the waste to produce biogas.

Questions:

1. What is the use of slurry left behind?
2. State the two uses of biogas.
3. What are the advantages of biogas plant?

Answer:

1. Slurry is used as nitrogen and phosphorous rich manure.
2. Two uses of biogas : Cooking, lighting
3. Biogas plant is a safe and efficient method for waste-disposal besides supplying energy and manure.

Question 3.
In high rised residential flats, maintainance expense per month per flat is high. Some members suggested to install solar panel and solar water heater. They explained that this would provide some electricity to light the building and may reduce electric bill amount.
Questions:

1. Which energy conversion is achieved by installation of solar panel and solar water heater?
2. What is the disadvantage of appliances which run on solar energy?
3. How much electric power is generated by a solar cell?

Answer:

1. Solar energy is converted to electricity by solar panel and in solar water heater, solar energy is converted to heat.
2. Solar appliances do not work during cloudy and rainy days.
3. 0.7 W

Practical Skill Based Questions With Answers

Question 1.
Two cars are parked In open parking area. One car is of black colour with black film on window glass. Other car is of white colour and without black film on window glass.
You just open the door and sit for 1-2 minute in each car.
Questions:

1. Which car is more hot inside?
2. Do both the cars have warm internal environment? Yes or no, why?
3. Why is black car more hotter than a white s car?
4. What are your suggestions to keep top floor cool in summer?

Answer:

1. Black car
2. Due to absorbing heat the cars are heated, making the inside very warm.
3. A black surface absorbs more heat as compared to a white surface.
4. Suggestions to keep top floor cool in summer:

• • • Paint white colour on the outer I side wall.
    • Mount china mosaic on the s terrace.
    • Prepare terrace garden.

Question 2.

A picture to demonstrate windmill and its function.
Questions :

1. Which energy conversion is shown in the picture?
2. What function does windmill show in the picture?
3. Which is the structure similar to windmill?

Answer:

1. Kinetic energy into mechanical energy.
2. To lift water from a well.
3. The structure of windmill is simillar to electric fan.

Memory Map:

Jharkhand Board JAC Class 10 Maths Solutions Chapter 6 Triangles Ex 6.4 Textbook Exercise Questions and Answers.

JAC Board Class 10 Maths Solutions Chapter 6 Triangles Exercise 6.4

Question 1.
Let ΔABC ~ ΔDEF and their areas be, respectively, 64 cm² and 121 cm². If EF = 15.4 cm, find BC.
Solution :
ΔABC ~ ΔDEF

Question 2.
Diagonals of a trapezium ABCD with AB || DC intersect each other at the point O. If AB = 2 CD, find the ratio of the areas of triangles AOB and COD.
Solution :

In trapezium ABCD, AB || CD and diagonals AC and BD intersect at O.
Then, in ΔAOB and ΔCOD.
∠OAB = ∠OCD (Alternate angles)
∠OBA = ∠ODC (Alternate angles)
∠AOB = ∠COD (Vertically opposite angles)
∴ By AAA criterion, ΔAOB ~ ΔCOD.

Question 3.
In the given figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that ar (ABC) / ar (DBC) = \(\frac{AO}{DO}\)
Solution :

Draw AM ⊥ BC and DN ⊥ BC.
Then, ar (ABC) = \(\frac{1}{2}\) × BC × AM and
ar (DBC) = \(\frac{1}{2}\) × BC × DN

In ΔAMO and ΔDNO
∠AMO = ∠DNO (Right angles)
∠AOM = ∠DON (Vertically opposite angles)
∴ By AA criterion, ΔAMO ~ ΔDNO.
∴ \(\frac{AM}{DN}=\frac{AO}{DO}\) ……………….(2)
From (1) and (2), ar (ABC) / ar (DBC) = \(\frac{AO}{DO}\)

Question 4.
If the areas of two similar triangles are equal, prove that they are congruent.
Solution :
Given: ΔABC ~ ΔPQR and ar (ABC) = ar (PQR)
To prove : ΔABC ≅ ΔPQR

Proof: ΔABC ~ ΔPQR

∴ AB = PQ, BC = QR and CA = RP
∴ By SSS criterion for congruence of triangles, ΔABC = ΔPOR.

Question 5.
D, E and F are respectively the mid-points of sides AB, BC and CA of ΔABC. Find the ratio of the areas of ΔDEF and ΔABC.
Solution :

In ΔABC, D, E and F are the mid-points of sides AB, BC and CA respectively.
Then, EF || AB and DE || AC
∴ EF || AD and DE || AF
∴ ADEF is a parallelogram.
∴ ∠A = ΔDEF
(Opposite angles of parallelogram)
Similarly, we can prove that ∠B = ∠EFD and ∠C = ∠EDF
Now, in ΔABC and ΔEFD,
∠A = ∠E, ∠B = ∠F and ∠C = ∠D
∴ By AAA criterion, ΔABC ~ ΔEFD.
∴ ar (DEF) / ar(ABC) = (\(\frac{EF}{AB}\))² ………………(1)
In ΔABC, E and F are the mid-points of BC and CA respectively.
∴ EF = \(\frac{1}{2}\)AB ………………(2)
(Alternately: ADEF is a parallelogram.
∴ EF = AD = \(\frac{1}{2}\)AB)
From (1) and (2),

Question 6.
Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
Solution :
Given: ΔABC ~ ΔPQR, AD and PM are medians of triangles ABC and PQR respectively.

Proof :
In ΔABC, AD is a median ∴ BD = \(\frac{1}{2}\)BC
In ΔPQR, PM is a median ∴ QM = \(\frac{1}{2}\)QR.
ΔABC ~ ΔPQR
∴ ∠B = ∠Q and \(\frac{AB}{PQ}=\frac{BC}{QR}\)
∴ ∠ABD = ∠PQM and \(\frac{AB}{PQ}=\frac{BD}{QM}\)
So, by SAS criterion ΔABD ~ ΔPQM.
∴ \(\frac{AB}{PQ}=\frac{AD}{PM}\) ……………(1)
Now, ΔABC ~ ΔPQR
∴ ar (ABC) / ar (PQR) = (\(\frac{AB}{PQ}\))²
∴ ar (ABC) / ar (PQR) = (\(\frac{AD}{PM}\))² [By (1)]

Question 7.
Prove that the area of an equilateral triangle described on one side of a square is equal to half the area of an equilateral triangle described on one of its diagonals.
Solution :

ABCD is a square. PAB, is an equilateral triangle described on side AB and QAC is an equilateral triangle described on diagonal AC.
In ΔABC, ∠B = 90° and AB = BC (Properties of a square)
Then, AC² = AB² + BC² (Pythagoras theorem)
∴ AC² = AB² + AB²
∴ AC² = 2AB²
∴ \(\frac{\mathrm{AB}^2}{\mathrm{AC}^2}\) = \(\frac{1}{2}\)
∴ (\(\frac{18}{36}\))
ΔPAB is an equilateral triangle.
∴ ∠P = ∠A = ∠B = ∠60°
ΔQAC is an equilateral triangle.
∴ ∠Q = ∠A = ∠C = 60°
Thus, in ΔPAB and ΔQAC,
∠P = ∠Q and ∠A = ∠A and ∠B = ∠C
∴ By AAA criterion, ΔPAB ~ ΔQAC.
∴ ar (PAB) / ar(QAC) = (\(\frac{AB}{AC}\))²
∴ ar (PAB) / ar(QAC) = \(\frac{1}{2}\) [By (1)]
∴ ar (PAB) = \(\frac{1}{2}\)ar (QAC)
Thus, the area of an equilateral triangle described on a side of a square is half the area of an equilateral triangle described on one of its diagonals.

Tick the correct answer and justify:

Question 8.
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Ratio of the areas of triangles ABC and BDE is
(A) 2 : 1
(B) 1 : 2
(C) 4 : 1
(D) 1 : 4
Solution :
The correct answer is (C) 4 : 1.
ΔABC and ΔBDE are equilateral triangles.
Hence, any of their correspondences is a similarity.

Question 9.
Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio
(A) 2 : 3
(B) 4 : 9
(C) 81 : 16
(D) 16 : 81
Solution :
The correct answer is (D) 16 : 81.
By theorem 6.6.
Ratio of areas of two similar triangles
= (Ratio of their corresponding sides)²
= (4 : 9)²
= (\(\frac{4}{9}\))²
= 16 : 81

Jharkhand Board JAC Class 10 Science Solutions Chapter 10 Light Reflection and Refraction Textbook Exercise Questions and Answers.

JAC Board Class 10 Science Solutions Chapter 10 Light Reflection and Refraction

Jharkhand Board Class 10 Science Light Reflection and Refraction Textbook Questions and Answers

Question 1.
Which one of the following materials cannot be used to make a lens?
(a) Water
(b) Glass
(c) Plastic
(d) Clay
Answer:
(d) Clay
[Hint: The material of a lens must be transparent but clay is opaque.]

Question 2.
The image formed by a concave mirror is observed to be virtual, erect and larger than the object. Where should be the position of the object?
(a) Between the principal focus and the centre of curvature
(b) At the centre of curvature
(c) Beyond the centre of curvature
(d) Between the pole of the mirror and its principal focus
Answer:
(d) Between the pole of the mirror and its principal focus.

Question 3.
Where should an object be placed in front of a convex lens to get a real image of the size of the object?
(a) At the principal focus of the lens
(b) At twice the focal length of the lens
(c) At infinity
(d) Between the optical centre of the lens and its principal focus
Answer:
(b) At twice the focal length of the lens

Question 4.
A spherical mirror and a thin spherical lens have each a focal length of – 15 cm. The mirror and the lens are likely to be …
(a) both concave.
(b) both convex.
(c) the mirror is concave and the lens is convex.
(d) the mirror is convex, but the lens is concave.
Answer:
(a) both concave.

Question 5.
No matter how far you stand from a mirror, your image appears erect. The mirror is likely to be _______.
(a) only plane.
(b) only concave.
(c) only convex.
(d) either plane or convex.
Answer:
(d) either plane or convex.
[Hint: Both give erect images. The size of image in plane mirror is same as the object but it is diminished in convex mirror.]

Question 6.
Which of the following lenses would you prefer to use while reading small letters found in a dictionary?
(a) A convex lens of focal length 50 cm
(b) A concave lens of focal length 50 cm
(c) A convex lens of focal length 5 cm
(d) A concave lens of focal length 5 cm
Answer:
(c) A convex lens of focal length 5 cm [Hint: Since a convex lens gives a magnified image of the object and the smaller the focal length, the more the magnifying power.]

Question 7.
We wish to obtain an erect image of an object, using a concave mirror of focal length 15cm. What should be the range of distance of the object from the mirror? What is the nature of the image? Is the image larger or smaller than the object? Draw a ray diagram to show the image formation in this case.
Solution:
When the object is placed between the pole and the principal focus of a concave mirror, an erect, virtual and enlarged image is formed. This image can be viewed in the mirror itself, not on the screen. In short, image is formed behind the mirror as shown in the figure 10.57.

Therefore, the range of distance of the object from the mirror must be greater than zero and less than 15 cm (focal length of the concave mirror).

Question 8.
Name the type of mirror used in the following situations:
(a) Headlights of a car
(b) Side / rear-view mirror of a vehicle
(c) Solar furnace
Support your answer with reason.
Answer:
(a) For headlights of a car, a concave mirror is used.
The light source is kept at the focus s of the mirror. On reflection, a strong? parallel beam of light emerges out.

(b) For side / rear-view mirror of a vehicle, s convex mirror is used.
This is because its field of view is very large and it forms a virtual, erect and diminished image of the object behind the vehicle, which enables a driver to see most of the traffic behind him/her.

(c) For a solar furnace, a concave mirror is used.
Light from the Sun, on reflection from t the mirror, is concentrated at the focus of s the mirror, producing heat and temperature increases sharply up to 180°C-200°C

Question 9.
One-half of a convex lens is covered with a black paper. Will this lens produce a complete image of the object? Verify your answer experimentally. Explain your observations.
Answer:
Yes.
Even when one-half of a convex lens is covered with a black paper, the lens produces a complete (or full) image of the object.

Here, the intensity (brightness) of image will become one-forth as that with complete lens exposed, because less number of light rays can be passed / refracted through the lens.

The nature, size and location of the image will be the same since light from all parts of the object reach the exposed part of the lens.

It can be understood by the following two cases (Experimental verification) :
(1) When the upper half of the lens is covered :
In this case, the ray of light coming from the object will be refracted by the lower half of the lens.

These rays meet at the other side of the lens to form the image of the given object.

(2) When the lower half of the lens is covered:
In this case, the ray of light coming from the object will be refracted by the upper half of the lens.

These rays meet at the other side of the lens to form the image of the given object.

[Note: Covering one-half of the lens reduces the brightness of the image.]

Question 10.
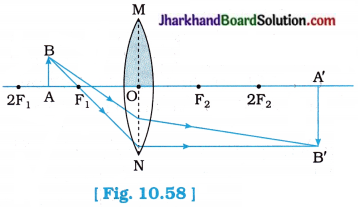
An object 5 cm in length is held 25 cm away from a converging lens of focal length 10 cm. Draw the ray diagram and find the position, size and the nature of the image formed.
Solution:
Here, Object size h = + 5 cm
Object distance u = – 25 cm
Focal length of lens f = + 10 cm
(∵ Converging, i.e., Convex lens)
Image distance v = ?
Image height h’ = ?
As, \(\frac { 1 }{ f }\) = \(\frac { 1 }{ v }\) – \(\frac { 1 }{ u }\)
\(\frac { 1 }{ v }\) = \(\frac { 1 }{ f }\) + \(\frac { 1 }{ u }\)
= \(\frac{1}{(+10)}+\frac{1}{(-25)}=\frac{5-2}{50}=\frac{3}{50}\)
= 16.67 cm
Image distance v is positive. This shows that the image formed is real and on the other side of the lens, at 16.67 cm from the lens as shown in the figure 10.60:

Now, Magnification m = \(\frac { h’ }{ h }\) = \(\frac { v }{ u }\)
∴ h’ = h\(\frac { v }{ u }\)
= \(\frac { 1 }{ f }\)
= – \(\frac { 10 }{ 3 }\) cm
= – 3.3 cm
The negative (minus) sign shows that the image is inverted, real, diminished (3.3 cm).
Figure 10.60 show the position, size and nature of the image formed.

[Note: The ray diagram can be drawn without calculating v and h’. Once, f, u and h are fixed, v and h’ have definite values.

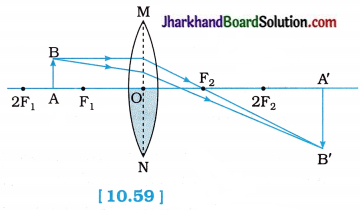
Question 11.
A concave lens of focal length 15 cm forms an image at 10 cm from the lens. How far is the object placed from the lens? Draw the ray diagram.
Solution:
Here, Focal length of the lens f = – 15cm (∵ Concave lens)
Image distance v = – 10 cm
(∵ In case of a concave lens the image is formed on the same side of the object)
Object distance u = ?

The negative (minus) sign shows that the object is placed on the left side of the lens.

Question 12.
An object is placed at a distance of 10 cm from a convex mirror of focal length 15 cm. Find the position and nature of the image.
Solution:
Here, Object distance u = – 10 cm
Here, Object distance u = – 10 cm
Focal length of the mirror f = + 15 cm (∵ Convex mirror)
Image distance v = ?
Using the mirror formula \(\frac { 1 }{ f }\) = \(\frac { 1 }{ u }\) + \(\frac { 1 }{ v }\)
We have
\(\frac { 1 }{ v }\) = \(\frac { 1 }{ f }\) – \(\frac { 1 }{ u }\)
= \(\frac{1}{(+15)}-\frac{1}{(-10)}\)
= \(\frac{1}{15}+\frac{1}{10}\)
= \(\frac { 2+3 }{ 3 }\)
= \(\frac { 5 }{ 30 }\)
= \(\frac { 1 }{ 6 }\)
∴ v = 6 cm
The positive (plus) sign of v indicates that the image is formed behind the mirror.
Now, magnification m = – \(\frac { v }{ u }\) = – \(\frac { 6 }{ – 10 }\) = + 0.6
Positive value of magnification indicates that the image is virtual and erect.
The magnitude of magnification is 0.6, which is less than 1. This shows that the S image is diminished.

Question 13.
The magnification produced by a plane mirror is + 1. What does this mean?
Solution:
Here, m = + 1
As, m = \(\frac { h’ }{ h }\)
\(\frac { h’ }{ h }\) = + 1
∴ h’ = h
So, the size of the image is equal to the size of object.
Further, the positive (plus) sign of m indicates that the image is erect and hence virtual.
Again, m = – \(\frac { v }{ u }\) and m = + 1
∴ – \(\frac { v }{ u }\) = 1
∴ v = – u
This shows that the image is formed behind the mirror and the distance of the image from the mirror equals that of the object from the mirror.

Question 14.
An object 5.0 cm in length is placed at a distance of 20 cm in front of a convex mirror of radius of curvature 30 cm. Find the position of the image, its nature and size.
Solution:
Here, Size of object h = + 5.0 cm
Object distance u = – 20 cm
Radius of curvature R = + 30 cm (∵ Convex mirror)
Focal length f = \(\frac { + 30 cm }{ 2 }\) = + 15 cm
Image distance v = ?
Image size h’ = ?
Mirror formula: \(\frac { 1 }{ v }\) + \(\frac { 1 }{ u }\) = \(\frac { 1 }{ f }\)

The positive (plus) sign of u indicates that the image is behind the mirror or on the right-hand side of the mirror.

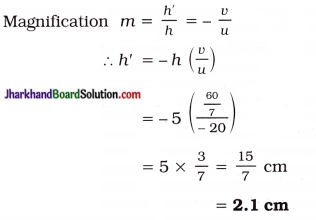
Positive value of image height indicated that the image is virtual and erect.
Moreover, h’ < h
So, the image is smaller in size than the object.

Question 15.
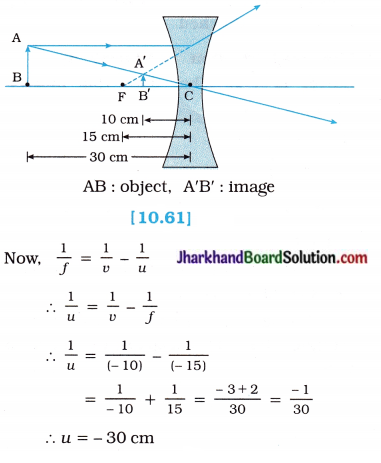
An object of size 7.0 cm is placed at 27 cm in front of a concave mirror of focal length 18 cm. At what distance from the mirror should a screen be placed, so that a sharp focussed image can be obtained? Find the size and the nature of the image.
Solution:
Here, Object size h = 7.0 cm
Object distance u = – 27 cm
Focal length f = – 18 cm
(∵ Concave mirror)
Image distance u = ?
Image size h’ = ?
From mirror formula :
\(\frac { 1 }{ v }\) + \(\frac { 1 }{ u }\) = \(\frac { 1 }{ f }\)

The negative (minus) sign of v indicates that ’ the image is formed on the same side of the object. So, screen should be held in front of the mirror at a distance of 54 cm from the mirror. The image can be obtained on the screen and hence, it is real.
Now, magnification m = \(\frac { h’ }{ h }\) = – \(\frac { v }{ u }\)
∴ h’ = – h(\(\frac { v }{ u }\))
= – 7.0 (\(\frac { -54 }{ -27 }\))
= – 14 cm
The negative (minus) sign of h’ shows that the image is inverted and hence would be real.

Question 16.
Find the focal length of a lens of power -2.0D. What type of lens is this?
Solution :
Here, Focal length of the lens f = ?
Power P = – 2.0 D = – 2.0 m^-1
Now,
As, P = \(\frac { 1 }{ f }\)
f = \(\frac { 1 }{ p }\)
= \(\frac { 1 }{ -2D }\)
= – 0.5 cm
As the power of the lens is negative (given), the lens must be concave.

Question 17.
A doctor has prescribed a corrective lens of power + 1.5 D. Find the focal length of the lens. Is the prescribed lens diverging or converging?
Solution:
Here, Power P = + 1.5 D = + 1.5 m^-1
Focal length f = ?
From, f = \(\frac { 1 }{ p }\)
f = \(\frac { 1 }{ 1.5 D }\)
= 0.67 m
= 67 cm
As the power of the lens is positive (given), it is a converging lens, i.e., a convex lens.

Jharkhand Board Class 10 Science Light Reflection and Refraction InText Questions and Answers

Question 1.
Define the principal focus of a concave mirror.
Answer:
The point on the principal axis of a concave mirror, at which rays of light incident on the mirror in a direction parallel to the principal axis (actually) meet / intersect after reflection from the mirror is called the principal focus (F) of the concave mirror.

[Note : In general, the point on the principal axis where rays of light incident parallel to the principal axis converge to or appear to diverge from after reflection is called the principal focus of the spherical mirror.]

Question 2.
The radius of curvature of a spherical mirror is 20 cm. What is its focal length?
Solution:
Here, R = 20 cm; f =?
As f = \(\frac { R }{ 2 }\), f = \(\frac { 20 }{ 2 }\)
= 10 cm
[Note: According to the New Cartesian sign convention, if the given spherical mirror is a convex mirror, then f = + 10cm and if the given spherical mirror is a concave mirror, then f = – 10 cm.]

Question 3.
Name a mirror that can give (form) an erect and enlarged image of an object.
Answer:
Concave mirror:
A concave mirror produces an erect and enlarged image of an object, when an object is placed between the pole and principal focus of the concave mirror.

Question 4.
Why do we prefer a convex mirror as a rear-view mirror in vehicles?
Answer:
This is because a convex mirror (always) forms an erect, virtual and diminished image of an object, wherever the object may be located.

Also, a convex mirror has a wider field of view, relative to a plane mirror, as it is curved outwards. Thus, a convex mirror fitted on the side of a vehicle enables the driver to view much larger area than that would be possible with a plane mirror, enabling the driver to see traffic behind him/her to facilitate safe driving.

Question 5.
Find the focal length of a convex mirror whose radius of curvature is 32 cm.
Solution:
Here, the radius of curvature R = 32 cm
We know that, the focal length f = \(\frac { R }{ 2 }\)
∴ f = \(\frac { 32 }{ 2 }\) = 16 cm

Question 6.
A cancave mirror produces three times magnified (enlarged) real image of an object placed at 10 cm in front of it. Where 5 is the image located?
Solution:
Here, the linear magnification m = – 3
(negative sign for a real image, which is inverted)
The object distance u = – 10 cm
(object distance (real) is always negative)
The image distance v =?
As m = – \(\frac { v }{ u }\)
v = – mu
∴ v = – (-3) (- 10)
= – 30 cm
Thus, the real image is located at 30 cm in front of the mirror (on the same side as the object).

Question 7.
A ray of light travelling in air enters obliquely into water. Does the light ray bend towards the normal or away from the normal? Why?
Answer:
For oblique incidence, the light ray bends towards the normal, on entering water. It happens because water is optically denser than air.

The speed of light is higher in an optically rarer medium than in an optically denser medium. So, a ray of light travelling from air to water slows down and bends towards the normal.
OR

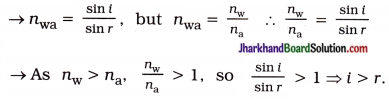
Thus, the angle of refraction is less than the angle of incidence. It implies that the light ray bends towards the normal.

Question 8.
Light enters from air to glass having refractive index 1.50. What is the speed of light in the glass? The speed of light in vacuum is 3 x 10⁸ m s^-1.
Solution:
Speed of light in vaccum c = 3 x 10⁸ m s^-1.
Refractive index of glass n_g = 1.50
Speed of light in glass v = ?
Now, absolute refractive index of glass,
n_g = \(\frac { c }{ v }\)
∴ The speed of light in the glass,
v = \(\frac{c}{n_{\mathrm{g}}}=\frac{3 \times 10^8}{1.50}\)
= 2 x 10⁸ m s^-1.

Question 9.
Find out from Table 2 (Given with Q. 42), the medium having highest optical density. Also, find the medium with lowest optical density.
Answer:
The higher the refractive index, the higher is the optical density. Diamond has the highest optical density as it has the highest refractive index, 2.42 and air has the lowest optical density as it has the lowest refractive index, 1.0003.

Question 10.
You are given kerosene, turpentine and water. In which of these does the light travel fastest? Use the information given in Table 2.
Answer:
From Table 2,
refractive index of kerosene = 1.44
refractive index of turpentine = 1.47 and
refractive index of water = 1.33
Here, from given liquids, turpentine has the highest refractive index and water has the lowest refractive index.
Now, the lower the refractive index of a medium, the higher is the speed of light in the medium.
Hence, out of the given liquids the light travels fastest in water.

Question 11.
The refractive index of diamond is 2.42. What is the meaning of this statement?
Answer:
Refractive index of diamond
= \(\frac{\text { Speed of light in vacuum / air }}{\text { Speed of light in diamond }}\)
∴ Speed of light in diamond
= \(\frac{\text { Speed of light in vacuum / air }}{\text { Refractive index of diamond }}\)
This expression states that the speed of light in diamond is \(\frac { 1 }{ 2.42 }\) times the speed of light in vacuum / air.
Speed of light in diamond = \(\frac{3 \times 10^8}{2.42}\)
= 1.24 x 10⁸ms^-1
In other words, it can also be said that, the ratio of the speed of light in vacuum/air to the speed of light in diamond is equal to 2.42.

Question 12.
Define 1 dioptre of power of a lens.
OR
Define the dioptre.
Answer:
1 dioptre is the power of a lens of focal length 1 metre.

Question 13.
A convex lens forms a real and inverted image of a needle at a distance of 50 cm from it. Where is the needle placed in front of the convex lens if the image is equal to the size of the object? Also, find the power of the lens.
Solution:
A convex lens forms a real, inverted image of the same size as that of the object, if the object is placed at 2F₁.
In this case v = + 50 cm and m = – 1

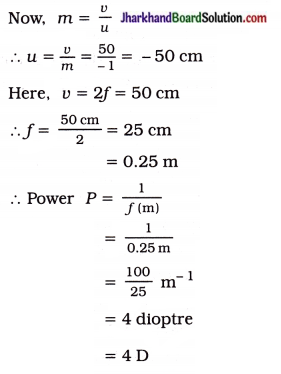
Thus, the needle is placed at 50 cm from the convex lens of power 4 D.

Question 14.
Find the power of a concave lens of focal length 2 m.
Solution :
Here, focal length f = – 2 m (∵ Concave lens)
∴ Power P = \(\frac { 1 }{ f(m) }\)
= \(\frac { 1 }{ -2m }\)
= – 0.5 m^-1
= – 0.5 dioptre
= – 0.5 D

Activity 10.1 [T. B. Pg. 161]

To determine the nature of the inner and outer curved surface of a spoon.

Procedure :
1. Take a large shining spoon. Try to view your face in its inner curved surface.

• Do you get the image?
• Is it smaller or larger?

2. Move the spoon slowly away from your face. Observe the image.

• How does it change?

3. Reverse the spoon and repeat the activity.

• How does the image look like now?

4. Compare the characteristics of the image on the two surfaces.

Observation :

• Yes.
• The image formed is enlarged and erect when the spoon is closer to the face.
• As the spoon is moved slowly away from the face, the image becomes large and inverted. When the face is very far off, highly diminished and inverted image of our face is seen.
• When the spoon is reversed, the image formed by the outer surface of the spoon is virtual and diminished in size.
• Now, if we move the spoon away from our face, the image moves away, however the image continues to be virtual and diminished in size.
• Finally, at last the image is diminished to almost point-size.

Conclusion:
The inner surface of the spoon acts as a concave mirror, whereas the outer surface acts as a convex mirror.

Activity 10.2 [T. B. Pg. 162]

To show the converging nature of a concave mirror and find its focal length.

Procedure:

• Hold a concave mirror in your hand and direct its reflecting surface towards the Sun.
• Direct the light reflected by the mirror on to a sheet of paper held close to the mirror.
• Move the sheet of paper back and forth gradually until you find on the paper sheet a bright, sharp spot of light.
• Hold the mirror and the paper in the same position for a few minutes.
• What do you observe? Why?
  

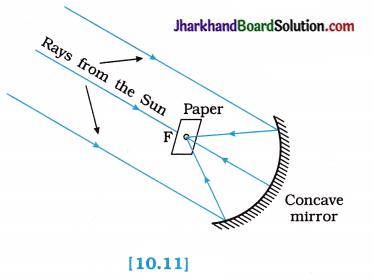
Observation:
The paper initially turns blackish, then burns producing smoke. Eventually it catches fire. The light from the Sun is converged at a point, as a sharp bright spot by the mirror. In fact, this spot of light is the image of the Sun on the sheet of paper.

This point is the principal focus of the concave mirror. The heat produced due to concentration of sunlight ignites the paper.

Conclusion:

• The distance of this image of the Sun from the pole of the mirror gives the approximate value of the focal length of the concave mirror.
• It is also concluded that when a parallel beam of light from a far off object falls on a concave mirror, a real, inverted and point-ized image is formed at the focus of the concave mirror.

Activity 10.3 [T. B. Pg. 163]

To locate the image formed by a concave mirror for different positions of the object.

Procedure :
1. Take a concave mirror.

2. Find out its approximate focal length in the way described in Activity 10.2.

3. Note down the value of the focal length.

4. Mark a straight line on a table with a chalk. Place the concave mirror on a stand. Place the stand over the line such that the pole of the mirror lies over the line.

5. Draw with a chalk two more straight lines parallel to the previous line such that the distance between any two successive lines is equal to the focal length of the mirror.

These lines will now correspond to the positions of the points P F and C respectively.
(Remember : For a spherical mirror of small aperture, the principal focus F lies mid-way between the pole P and centre of curvature C.)

6. Keep a bright object, say a burning candle, at a position far beyond C.
Place a paper screen and move it in front of the mirror till you obtain a sharp bright image of the candle flame on it.

7. Observe the image carefully. Note down its nature, position and relative size with respect to the object size.

8. Repeat the activity by placing the candle –

• just beyond C
• at C
• between F and C
• at F
• between P and F

In one of the cases, you may not get the image on the screen. Identify the position of the object in such a case. Where would you look to observe the image of that object?

9. Note down and tabulate your observations.

Observation:
1. Image formation by a concave mirror for different positions of the object.

Position of the object	Position of the image	Size of the image	Nature of the image
At infinity	At the focus F	Highly diminished, point-sized	Real and inverted
Just beyond C	Between F and C	Diminished	Real and inverted
At C	At C	Same size	Real and inverted
Between C and F	Beyond C	Enlarged	Real and inverted
At F	At infinity	Highly enlarged	Real and inverted
Between P and F	Behind the mirror	Enlarged	Virtual and erect

2. When an object (the burning candle in the present case) is placed between P and F, its image cannot be obtained on the screen.

3. Hence, to observe its image, in this case one has to look in the mirror itself because the image is virtual.

Conclusion:
The nature, position and size of the image formed by a concave mirror depends on the position of the object in relation to the points E F and C.

Activity 10.4 [T. B. Pg. 166]

To locate the image formed by a concave mirror for different positions of an object using ray diagrams.

Procedure:

• Draw a neat ray diagram for each position of the object as discussed in the Activity 10.3. You may take any two of the rays (mentioned in the previous point 10.2.2), for locating the image.
• Observe the nature, position and relative size of the image formed in each case.
• Tabulate the results in a convenient format.

Observation:
The following figures illustrate the ray diagrams for the formation of image by a concave mirror for various positions of the object:

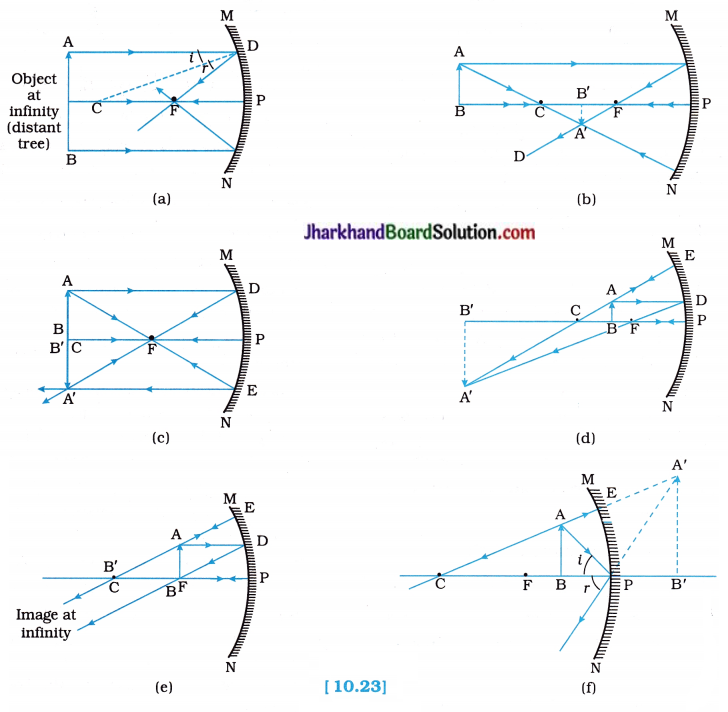
Conclusion:
Image formation by the concave mirror for different positions of an object using ray diagrams is tabulated as follows :

Position of the object	Position of the image	Size of the image	Nature of the image
At infinity	At the focus F	Highly diminished, point-sized	Real and inverted
Just beyond C	Between F and C	Diminished	Real and inverted
At C	At C	Same size	Real and inverted
Between C and F	Beyond C	Enlarged	Real and inverted
At F	At infinity	Highly enlarged	Real and inverted
Between P and F	Behind the mirror	Enlarged	Virtual and erect

Activity 10.5 [T. B. Pg. 167]

To locate the image formed by a convex mirror for different positions of an object.

Procedure:

• Take a convex mirror. Hold it in one hand.
• Hold a pencil in the upright position in the other hand.
• Observe the image of the pencil in the mirror.
• Is the image erect or inverted? Is it diminished or enlarged?
• Move the pencil away from the mirror slowly.
• Does the image become smaller or larger?
• Repeat this activity carefully.
• State whether the image will move closer to or farther away from the focus as the object is moved away from the mirror.

Observation:
1. When we hold a pencil in the upright position in front of a convex mirror, the image of the pencil is observed on the back side of the mirror, i.e., in the mirror itself.
The image is erect and virtual.
The image is diminished in size relative to the object.

2. When the pencil is moved away from the mirror slowly, the image becomes smaller and smaller, moving away from the mirror.

3. On repeating this activity, we find that as the object is moved away from the mirror, the image moves closer to the focus of the mirror.

Conclusion:
This activity confirms all the characteristics of the images formed by a convex mirror for different positions of the object and it is tabulated as follows :

Position of the object	Position of the image	Size of the image	Nature of the image
At infinity	At the focus F, behind the mirror	Highly diminished, point-sized	Virtual and erect
Between infinity and the pole P of the mirror	Between P and F, behind the mirror	Diminished	Virtual and erect

Activity 10.6 [T. B. Pg. 167]

To demonstrate the wide field of view nature of a convex mirror.

Procedure:

• Observe the image of a distant object, say a distant tree in a plane mirror.
• Could you see a full-length image?
• Try with plane mirrors of different sizes.
• Did you see the entire object in the image?
• Repeat this activity with a concave mirror.
• Did the concave mirror show full-length image of the object?
• Now, try using a convex mirror.
• Did you succeed?
• Explain your observation with reason.

Observation :
No, we can not see the full-length image of an object in plane mirror.

When we try with plane mirrors of different sizes, we find that the entire object in the image is seen when the size of the plane mirror is at least half of the size of the object.

No, concave mirror did not show full-length S image of the object (here a distant tree).

When we use a small convex mirror, we succeed in seeing the full-length image of an object, wherever the object may be located.

The reasons for these observations are as follows :
(1) In a plane mirror, size of image is S always equal to the size of the object.

(2) In case of a concave mirror, when object is between P and F, then only its enlarged, virtual and erect image is seen behind the concave mirror.
(i.e., full-length image of an object can be seen).
But here our object is far away from the concave mirror, e.g., distant tree; so its full- length image can not be seen in the concave mirror itself (i.e., behind the concave mirror).

(3) While, in a convex mirror, the image is always virtual, erect and smaller than the object, wherever the object may be located.

Conclusion:
Out of plane mirror, concave mirror and convex mirror, only a convex mirror can show a full-length image of a tall object in all its positions.
OR
A convex mirror has a wide field of view and the image formed by it is always virtual, erect and shorter than the object, wherever the object may be located.

Activity 10.7 [T. B. Pg. 172]

To demonstrate refraction of light.
OR
To show that the apparent depth of the bucket filled with water is less than the real depth of the bucket.

Procedure:

• Place a coin at the bottom of a bucket filled with water.
• With your eye to a side above water, try to pick up the coin in one go.
• Did you succeed in picking up the coin?
• Repeat the Activity.
• Why did you not succeed in doing it in one go?
• Ask your friends to do this.
• Compare your experience with theirs.

Observation:
No.
When you try picking up the coin with your eye to a side above water, you do not succeed in one go.

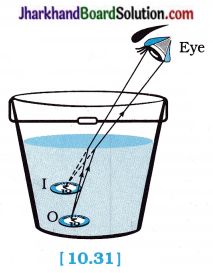
The reason for this can be explained as follows :
As shown in the figure 10.31, a coin is at point ‘O’ at the bottom of a bucket filled with water.

When we view this coin with our eye to a side above water, we observe the image I of the coin, which is above ‘O’. When we try to pick up this coin in one go, we do not succeed in picking up the coin. This is because we move our hand up to I, where the coin is being observed, but actually the coin lies below at O, the bottom of the bucket.

When our friends try the same way, they also fail to pick up the coin.

Conclusion:
In going from water to air, rays of light bend away from the normal and the bottom of the bucket appears to be raised, i.e., the apparent depth of the bucket is less than the real depth of the bucket.

Activity 10.8 [T. B. Pg. 172]

To show that the object in water appears to be raised due to refraction.
OR
Apparent depth of an object in the large shallow bowl filled with water increases as its real depth increases.

Procedure:

• Place a large shallow bowl filled with water on a table and put a coin in it.
• Move away slowly from the bowl. Stop when the coin just disappears from your sight.
• Ask a friend to pour water gently into the bowl without disturbing the coin.
• Keep looking for the coin from your position.
• Does the coin becomes visible again from your position?
• How could this happen?

Observation:

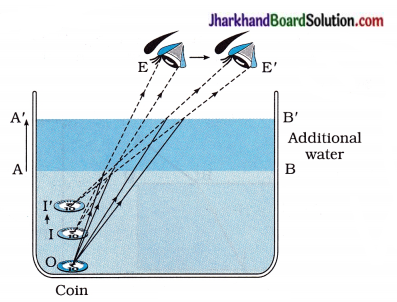
O = Real position of the coin
I = Initial apparent position of the coin
I’ = New apparent position of the coin

As shown in the figure 10.32, in a large shallow bowl filled with water, the real position of the coin is ‘O’.
On account of refraction of light the coin appears raised from O to I. Image I of the coin appears to be raised which is original apparent position of a coin.

When we move our eye slowly away from the bowl as shown by an arrow in the figure, the coin disappears from our sight. This happens because rays starting from the coin O fail to enter our eye, after refraction at water surface AB.

When a friend pours water gently into the bowl without disturbing the coin, the coin becomes visible again as it appears raised further to position I’.

This happens because on adding water, the real- depth of the coin increases. As the apparent depth of a coin is equal to the real depth divided by the refractive index (n) of water, the apparent depth of the coin also increases. Hence, the coin appears raised from I to I’. Therefore, the coin becomes visible from the displaced position (new position) of our eye.

Conclusion:
The coin becomes visible again and slightly raised above its actual position on pouring water into the bowl. This is because of refraction of light.

In other words, apparent depth of a coin in the large shallow bowl filled with water increases as its real depth increases.

Activity 10.9 [T. B. Pg. 172]

To show that refraction does not occur for normal incidence.

Procedure:

1. Draw a thick straight line in ink, over a sheet of white paper placed on a table.

2. Place a glass slab over the line in such a way that one of its edges makes an angle with the line.

3. Look at the portion of the line under the slab from the sides.

• What do you observe?
• Does the line under the glass slab appear to be bent at the edges?

4. Next, place the glass slab such that it is normal to the line.

• What do you observe now?
• Does the part of the line under the glass slab appear bent?

5. Look at the line from the top of the glass slab.

• Does the part of the line, beneath the slab, appear to be raised?
• Why does this happen?

Observation :

• When we look at the portion of the line under the slab from sides, careful observation shows that line AB is bent at edge B and line BC at edge C. [figure 10.33 (a)]
• Yes. The line under the glass slab appears bent at edges B and C.
• On placing the glass slab normal to the line, we observe that the part of the line under the glass slab does not appear bent, [figure 10.33 (c)]
• No. The line under the glass slab does not appear bent at edges B and C.
• Yes. The part of the line, beneath the slab, appears raised while looking at the line from the top of the glass slab.
• This is due to refraction of light.

Conclusion:
The object appears raised due to refraction of light and for normal incidence, refraction of light does not occur.

Activity 10.10 [T. B. Pg. 173]

To show the refraction of light and lateral displacement through a rectangular glass slab.

Procedure:

• Fix a sheet of white paper on a drawing board using drawing pins.
• Place a rectangular glass slab over the sheet in the middle.
• Draw the outline of the slab with a pencil and name the outline as ABCD.
• Take four identical pins.
• Fix two pins, say E and F, vertically such that the line joining the pins is inclined to the edge AB.
• Look for the images of the pins E and F through the opposite edge.
• Fix two other pins, say G and H, such that these pins and the images of E and F lie on a straight-line.
• Remove the pins and the slab.
• Join the positions of tip of the pins E and F and produce the line up to AB. Let EF meet AB at O. Similarly,
• join the positions of tip of the pins G and H and produce it up to the edge CD. Let HG meet CD at O’.
• Join O and O’. Also, produce EF up to P as shown by a dotted line in the following figure.
• At O, draw NN’ perpendicular to AB. At O’, draw MM’ perpendicular to CD. Also, draw O’L perpendicular to OP.
  

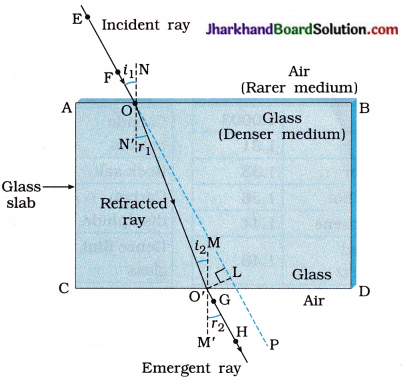
[Note : (1) In figure 10.34, face AB is air-glass interface and face CD is glass-air interface. ( 2 ) Reflection of light is not shown in the figure.]

Observation :

• At O, light ray along EF enters from air into glass. It bends towards the normal NN’. This is the first refraction.
• At O’, the light ray enters from glass into air. It bends away from the normal MM’ and travels 5 along GH. This is second refraction.
• Here, angle of emergence r₂ is equal to angle of incidence i₁, i.e., the emergent ray is parallel to the original direction of the incident ray. This is because there is identical medium, air in this case, adjacent to both edges AB and CD.
• However, the light ray is shifted sideways slightly. This is lateral displacement and is represented by O’L.

Conclusion:

• The ray of light travelling from a rarer medium (air in this case) to a denser medium (glass in this case) bends towards the normal.
• The ray of light travelling from a denser medium (glass in this case) to a rarer medium (air in this case) bends away from the normal.
• The emergent ray is parallel to the incident ray but is slightly displaced sideways.

Activity 10.11 [T. B. Pg. 177]

To show the converging nature of a convex? lens and find its focal length.

Procedure :
1. Hold a convex lens in your hand. Direct it towards the Sun.

2. Focus the light from the Sun (through lens) on a sheet of paper. Obtain a sharp bright image? of the Sun.

3. Hold the paper and the lens in the same position for a while.
Keep observing the paper.

• What happened?
• Why?
  

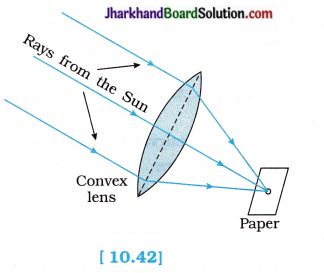
Observation :

• When the paper and the lens were held in the same position for sometime, the paper began to burn producing smoke. After sometime, it caught fire.
• Because, the light from the Sun constitutes parallel rays of light. These rays were converged by the lens at the sharp bright spot formed on the paper and so heat is produced by these Sun rays as they concentrate on the spot. This caused the paper to burn.

Conclusion:

• When a parallel beam of light from a far-off object like the Sun falls on a convex lens, a real, inverted, point size image of the Sun is formed at the focus of the lens.
• The distance between the position of the lens and the position of the image of the Sun gives the approximate focal length of the lens.

[Note : The distance between the lens and the image of the Sun on the paper is the approximate focal length of the lens.]

Activity 10.12 [T. B. Pg. 178]

To locate the position and examine the nature of the image formed by a convex lens for different positions of the object.

Procedure:

• Take a convex lens. Find its approximate focal length in a way described in Activity 10.11.
• Draw five parallel lines, using chalk, on a long table such that the distance between the successive lines is equal to the focal length of the lens.
• Place the lens on a lens stand. Place it on the central line such that the optical centre of the lens lies just over the line.
• The two lines on either side of the lens correspond to F and 2F of the lens respectively. Mark them with appropriate letters such as 2F₁, F₁, F₂ and 2F₂ respectively.
• Place a burnig candle, far beyond 2F₁ to the left. Obtain a clear sharp image on a screen on the opposite side of the lens.
• Note down the nature, position and relative size of the image.
• Repeat this Activity by placing the object just beyond 2F₁, at 2F₁, between F₁ and 2F₁, at F₁, between F₁ and O.
• Note down and tabulate your observations.

Observation:
Position, relative size and the nature of the image formed by a convex lens for various positions of the object.

Position of the object	Position of the image	Relative size of the image	Nature of the image
At infinity	At focus F2	Highly diminished, point-sized	Real and inverted
Beyond 2F1	Between F2and 2 F2	Diminished	Real and inverted
At F1	At 2F2	Same size	Real and inverted
Between F1and 2F1	Beyond 2 F2	Enlarged	Real and inverted
At focus F1	At infinity	Infinitely large or Highly enlarged	Real and inverted
Between focus F1 and optical centre O*	On the same side of the lens as the object	Enlarged	Virtual and erect

Conclusion:
The nature, position and relative size of the image formed by a convex lens for various positions of the object depends on the position of the object in front of the lens.

Activity 10.13 [T. B. Pg. 179]

To locate the position and examine the nature of the image formed by a concave lens for different positions of the object.

Procedure:

• Take a concave lens. Place it on a lens stand.
• Place a burning candle on one side of the lens.
• Look through the lens from the other side and observe the image. Try to get the image on a screen, if possible.
• If not, observe the image directly through the lens.
• Note down the nature, relative size and approximate position of the the image.
• Move the candle away from the lens. Note the change in the size of the image.
• What happens to the size of the image when the candle is placed too far away from the lens?
• What conclusion can you draw from this Activity?

Observation:
The image formed by a concave lens, when a burning candle is kept too far away from the lens is highly diminished, i.e., point-sized.
Position, relative size and the nature of the image formed by a concave lens for various position of an object

Position of the object	Position of the image	Relative size of the image	Nature of the image
At infinity	At focus F1	Highly diminished, point-sized	Virtual and erect
Between infinity and optical centre O of the lens	Between focus F1 and optical centre O	Diminished	Virtual and erect

Conclusion:
The image formed by a concave lens is always virtual, erect and diminished irrespective of the position of the object.

Jharkhand Board JAC Class 10 Science Solutions Chapter 11 Human Eye and Colourful Worlds Textbook Exercise Questions and Answers.

JAC Board Class 10 Science Solutions Chapter 11 Human Eye and Colourful World

Jharkhand Board Class 10 Science Human Eye and Colourful World Textbook Questions and Answers

Question 1.
The human eye can focus on objects at different distances by adjusting the focal length of the eye lens. This is due to ……………
(a) presbyopia.
(b) accommodation.
(c) near-sightedness.
(d) far-sightedness.
Answer:
(b) accommodation.
[Hint : Accommodation is the ability of the eye lens to focus both nearby and distant objects by adjusting its focal length.]

Question 2.
The human eye forms the image of an object at its ……………..
(a) cornea.
(b) iris.
(c) pupil.
(d) retina.
Answer:
(d) retina.
The retina is the light sensitive surface of the eye on which the image is formed.

Question 3.
The least distance of distinct vision for a young adult with normal vision is about ……………..
(a) 25 m
(b) 2.5 m
(c) 25 m
(d) 2.5 m
Answer:
(c) 25 cm.
The minimum distance at which an object can be seen most distinctly without any strain (i.e., comfortably) is 25 cm.

Question 4.
The change in focal length of an eye lens is caused by the action of the…
(a) pupil.
(b) retina.
(c) ciliary muscles.
(d) iris.
Answer:
(c) ciliary muscles.
The ciliary muscles contract and expand, in order to change the curvature of the eye lens for focussing the image of an object at varying distances at the retina.

Question 5.
A person needs a lens of power – 5.5 dioptres for correcting his distant vision. For correcting his near vision he needs a lens of power +1.5 dioptres. What is the focal length of the lens required for correcting (1) distant vision and (2) near vision?
Solution :
(1) For distant vision, f = ?
P = – 5.5, D = – 5.5 m^-1
Now, f = \(\frac { 1 }{ p }\)
∴ f = \(\frac{1}{-5.5 m^{-1}}\)
= – 0.182m
= – 18.2 cm (concave lens)

(2) For near vision, f = ?
P = + 1.5, D = + 1.5 m^-1
Now, f = \(\frac { 1 }{ p }\)
∴ f = \(\frac{1}{+1.5 m^{-1}}\)
= 0.667 m
= 66.7 cm (Convex lens)

Question 6.
The far point of a myopic person is 80 cm in front of the eye. What is the nature and power of the lens required to correct the problem?
Solution:
1. The defect of an eye called myopia (short-sightedness or near-sightedness) is corrected by using spectacles containing concave lens of appropriate focal length.

2. Here the far point of myopic person is 80 cm. (while far point of normal person is infinity oo)

3. This means that this person can see the distant object (kept at infinity) clearly if the image of this distant object is formed at his own far point (which is 80 cm here).
So, in this case:
Object distance u = – ∞ (Normal far point)
Image distance v = – 80 cm (Far point of this defective eye in front of lens)
Focal length f = ?
Now,
By the lens formula, \(\frac{1}{f}=\frac{1}{v}-\frac{1}{u}\)
∴ \(\frac{1}{f}=\frac{1}{-80}-\frac{1}{-\infty}\)
∴ \(\frac{1}{f}=-\frac{1}{80}\)
∴ f = – 80 cm
= – 0.8 m (Concave lens)
Now,
Power of the lens,
P = \(\frac { 1 }{ f }\)
∴ P = \(\frac { 1 }{ -0.8m }\)
= – \(\frac { 10 }{ 8 }\)m^-1
= – 1.25 D
A concave lens of power -1.25D is required to correct the problem.

Question 7.
Make a diagram to show how hypermetropia is corrected. The near point of a hypermetropic eye is 1 m. What is the power of the lens required to correct this defect? Assume that the near point of the normal eye is 25 cm.
Solution:
1. The defect of an eye called hypermetropia (long-sightedness or far-sightedness) is corrected by using spectacles containing convex lenses of appropriate focal length.

[N = Near point of a hypermetropic eye and
N’ = Near point of a normal eye]
[11.16 : (a) Hypermetropic eye (b) corrected eye]

2. Here the near point of the hypermetropic eye is 1 m = 100 cm
(while near point of normal eye is 25 cm)

3. This means that this person can see the nearby object (kept at 25 cm) clearly if the image of this nearby object is formed at his own near point (which is lm = 100 cm here).
So, in this case :
Object distance u = – 25 cm (Normal near point)
Image distance u = – 1 m = – 100 cm
(Near point of this defective eye in front of lens)
Focal length f = ?
Now,

Now,
Power of the lens,
P = \(\frac { 1 }{ f }\)
= \(\frac{1}{\left(\frac{1}{3} \mathrm{~m}\right)}\)
= 3D

Question 8.
Why is a normal eye not able to see clearly the objects placed closer than 25 cm?
Answer:
For seeing nearby objects, the ciliary muscles contract to make the eye lens thicker near the middle, so as to reduce the focal length of the eye lens.

But ciliary muscles cannot be contracted beyond a certain limit and hence we cannot see clearly the objects closer than 25 cm from the eye.

In other words, a normal eye is not able to see clearly the objects placed closer than 25 cm because all its power of accommodation has already been exhausted.

Question 9.
What happens to the image distance in the eye when we increase the distance of an object from the eye ?
Answer:
For a normal eye, the image distance (u) in the eye is fixed = distance of the retina from the eye lens ≈ 2.3 cm
When we increase the distance of the object (u) from the eye, the focal length of eye lens is changed on account of the accommodating power of the eye so as to keep the image distance (u) constant according to the relation \(\frac{1}{f}=\frac{1}{v}-\frac{1}{u}\).

Question 10.
Why do stars twinkle?
Answer:
Twinkling of stars is due to atmospheric refraction of starlight.

• Starlight, on entering the earth’s atmosphere, undergoes refraction continuously before it reaches the earth.
• As the optical density of air increases towards surface of the earth, light from the star travels from rarer to denser layers, bending every time towards the normal.
• On producing the final refracted ray backwards as shown in the following figure, we find that the apparent position (B) of a star is higher than the actual position (A) of the star as shown in figure 11.11.
  
• The star appears somewhat higher (above) than its actual position when viewed near the horizon.
  Further, this apparent position of the star is not stationary, but keeps on changing slightly, since physical conditions of the earth’s atmosphere are not stationary.
• Since the stars are very distant, they appear as point-sized sources of light.
• Due to a continuous change in the direction of propagation of light, the apparent position of the star fluctuates all the time and the amount of starlight entering the eye flickers, i.e., the brightness of the star changes continuously (the star sometimes appears brighter and at some other time fainter). This is called the twinkling s of a star.

Question 11.
Explain why the planets do not twinkle.
Answer:
Planets are much closer to the earth relative to stars. Therefore, planets appear bigger > than stars. Stars are far away from the earth. Therefore they appear smaller.

• So, stars can be considered as point-sized sources and planets can be considered as a ? collection of a large number of point-sized sources of light, i.e., extended sources of light.
• As planet is a collection of a large number of point-sized sources of light, the total variation in amount of light entering our eye from all the individual point-sized sources averages out to zero, thereby nullifying the twinkling effect. That is why planets do not twinkle.

Question 12.
Why does the Sun appear reddish early in the morning?
Answer:

• In figure 11.14, the situation at the sunrise is shown.
• Here, the white light coming from the Sun near the horizon, passes through thick layers of air and covers larger distance in the earth’s atmosphere before reaching the observer. During this, more scattering of blue light and shorter wavelengths take place. Hence, the reddish light reaches the observer and the Sun appears reddish.
• The same thing occurs at the sunset.

[Note : Rising and setting of the full moon from the horizon appears reddish due to this reason.]

Question 13.
Why does the sky appear dark instead of blue to an astronaut?
Answer:
The sky appears dark to the astronaut because in outer space, there is no atmosphere to scatter the sunlight. Since there is no scattering of the blue component of the white sunlight which can reach the eye of an astronaut in outer space, the sky appears dark to the astrounaut, instead of blue.

Jharkhand Board Class 10 Science Human Eye and Colourful World InText Questions and Answers

Question 1.
What is meant by power of accommodation of the eye?
Answer:
The ability of the eye lens to adjust its focal length, so that nearby as well as distant objects can be focussed on the retina and hence seen comfortably and distinctly is called the power of accommodation of the eye.

Question 2.
A person with a myopic eye cannot see objects beyond 1.2 m distinctly. What should be the type of the corrective lens used to restore proper vision?
Answer:
A person with a myopic eye should use a concave lens of suitable focal length or power to restore proper vision.
[Here, the person with defect of myopia has the far point nearer than infinity at a distance of 1.2 m from the eye.
So, v = – 1.2m; u = – ∞; f = ?
So, from the lens formula
\(\frac{1}{f}=\frac{1}{-u}-\frac{1}{v}\)
∴ \(\frac{1}{f}=\frac{1}{-(-\infty)}+\frac{1}{-1.2}\)
∴ f = – 1.2 m
∴ P = \(\frac { 1 }{ – 1.2 }\) = – 0.83 D
∴ A concave lens of focal length 1.2 m should be used to restore proper vision.]

Question 3.
What is the far point and near point of the human eye with normal vision?
Answer:
For the human eye with normal vision, f the far point is at infinity and the near point is at 25 cm from the eye.

Question 4.
A student has difficulty in reading the blackboard while sitting in the last row. What could be the defect the child is suffering from? How can it be corrected?
Answer:
As the child cannot see distant objects 5 clearly it means that he is suffering from myopia or near-sightedness. In this case the child can S see nearby objects clearly but cannot see far off objects distinctiy as their images are formed s before the retina.

To correct this defect, the child has to use spectacles with concave lens of suitable focal length.

Activity 11.1 [T. B. Pg. 192]

To study the refraction of light through a s triangular glass prism.

Procedure:
1. Fix a sheet of white paper on a drawing board ; using drawing pins.

2. Place a glass prism on it in such a way that ; it rests on its triangular base. Trace the outline of the prism using a pencil.

3. Fix two pins, say at points P and Q, on the line PE as shown in the following figure :

Refraction of light through a triangular glass prism
PE – Incident ray
EE – Refracted ray
FS – Emergent ray
∠A – Angle of the prism
∠i – Angle of incidence
∠r – Angle of refraction
∠e – Angle of emergence
∠D – Angle of deviation

4. Look for the images of the pins, fixed at P and Q, through the other face AC.

5. Fix two more pins, at points R and S, such that the pins at R and S and the images of the pins at P and Q lie on the same straight line.

6. Remove the pins and the glass prism.

7. Join the points P and Q and extend the line till it meets the nearest boundary of the prism. Similarly, join and produce the points R and S. Let these lines meet the boundary of the prism at E and F respectively. Join E and E

8. Extend PE and RS such that they meet at point G. Mark the angle of deviation (∠D) as shown in figure.

9. Draw perpendiculars to the refracting surfaces AB and AC of the prism at points E and F respectively.

10. Mark the angle of incidence (∠i), the angle of refraction (∠r) and the angle of emergence (∠e) as shown in the figure.

Compare the angle of incidence and the angle of refraction at each refracting surface of the prism.
Bending of rays PE, EF and FS are similar to the kind of bending that occurs in a glass slab.

Observation:
1. A ray of light suffers two refractions while passing through a prism.

2. The first refraction occurs at E on the surface AB. The incident ray PE, enters from air into glass E. It is refracted along EF bending towards normal NN’ on face AB at E.

3. The second refraction occurs at F on the surface AC. The initial refracted ray EF travelling in glass emerges in air at F It emerges along FS, bending away from normal MM’ on face AC at F.

4. At the first refracting surface AB, angle of refraction (r) is smaller than the angle of incidence (i).

But at the second refracting surface AC, angle of refraction (e) is larger than angle of incidence (∠EFM’).

5. Bending of rays PE, EF and FS is of the same kind of bending that occurs in a glass slab. The net deviation in a rectangular glass slab is zero and there is lateral shift. However, due to the peculiar shape of the prism, net diviation in passing through a prism is not zero and the prism makes the emergent ray bend at an angle to the direction of the incident ray. This angle is called the angle of deviation, i.e., D = ∠HGS, It is the angle through which the incident ray is deviated as it passes through the prism.

6. The angle of deviation depends on the angle of incidence, the angle of the prism and the nature of the material of the prism as it depends on the refractive index of the material.

Conclusion :
While passing through a prism, a ray of light undergoes two refractions as it does in case of rectangular glass slab.

However due to peculiar shape of the prism, net deviation through prism is never zero, though the net deviation is zero in case of rectangular glass slab.

In short, deviation suffered by a ray of light on passing through a prism is non-zero.

Activity 11.2 [T. B. Pg. 193]

To show that white light is made up of seven colours and different colours suffer different deviations on passing through a prism.

Procedure:
1. Take a thick sheet of cardboard and make a small hole or narrow slit in its middle.

2. Allow sunlight to fall on the narrow slit. This gives a narrow beam of white light.

3. Now, take a glass prism and allow the light from the slit to fall on one of its faces as shown in the following figure:

4. Turn the prism slowly until the light that comes out of it appears on a nearby screen.

• What do you observe?
• Why does this happen? OR How could white light of the Sun give us various colours of the rainbow?
• What is the sequence of colours that you observe on the screen?

Observations:

• We observe beautiful band of seven colours (VIBGYOR) on the screen. The deviation suffered by the violet light is maximum and that by the red light is minimum. Hence, the violet colour is at the lower end and the red colour is at the upper end of the screen.
• This happens because prism itself splits the incident white light into a band of colours.
• The sequence of colours seen from the lower end of the screen is violet (V), indigo (I), blue (B), green (G), yellow (Y), orange (O) and red (R).

Conclusion :
White light is made up of seven colours and different colours suffer different deviations.

Activity 11.3 [T. B. Pg. 196]

To observe scattering of light by colloidal particles.

Procedure:
1. Place a strong source (S) of white light at the focus of a convex (converging) lens (L₁) as shown in the figure 11.15 to produce a parallel beam of light.

2. Allow the light beam to pass through a transparent glass tank vessel (T) containing clean water.

3. Now, allow the beam of light to pass through the circular hole (C) made in a cardboard and obtain a sharp image of the circular hole on screen (MN) using a second convex (converging) lens (L₂) as shown in figure.

4. Dissolve 200g of sodium thiosulphate (hypo) – (Na₂S₂O₃) in about 2 L of clean water taken in the tank. Add 1 to 2 mL of concentrated sulphuric acid (H₂SO₄) in the water.
What do you observe?

Observation:
In the arrangement shown in the figure, we find that fine microscopic sulphur particles precipitate in water (from solidum thiosulphate) in a couple of minutes.

Blue colour is seen from three sides of the tank due to scattering of short wavelengths by minute sulphur particles. We see transmitted light from the fourth side of the tank facing the hole (C) of the cardboard.

We first observe orange-red colour and then bright crimson red colour on the screen (MN) because the transmitted light contains mainly longer wavelengths only.

Conclusion:
Very fine particles of liquid, mainly scatter blue light of smaller wavelength and the red light containing longer wavelength passes straight (without scattering) from the vessel.

Jharkhand Board JAC Class 10 Science Solutions Chapter 12 Electricity Textbook Exercise Questions and Answers.

JAC Board Class 10 Science Solutions Chapter 12 Electricity

Jharkhand Board Class 10 Science Electricity Textbook Questions and Answers

Question 1.
A piece of wire of resistance R is cut into five equal parts. These parts are then connected in parallel. If the equivalent resistance of this combination is R’, then the ratio \(\frac { R }{ R }\) is
(a) \(\frac { 1 }{ 25 }\)
(b) \(\frac { 1 }{ 5 }\)
(c) 5
(d) 25
Answer:
(d) 25
Hint Here, the resistance of a piece of wire is R. This piece of wire is cut into five equal parts, so the resistance of each part would be \(\frac { R }{ 5 }\).
Now, these five parts each having resistance of \(\frac { R }{ 5 }\) are connected in parallel.
The equivalent resistance R’ of this combination is given by
\(\frac{1}{R^{\prime}}=\frac{1}{\left(\frac{R}{5}\right)}+\frac{1}{\left(\frac{R}{5}\right)}+\frac{1}{\left(\frac{R}{5}\right)}+\frac{1}{\left(\frac{R}{5}\right)}+\frac{1}{\left(\frac{R}{5}\right)}\)
∴ \(\frac{1}{R^{\prime}}=\frac{5}{R}+\frac{5}{R}+\frac{5}{R}+\frac{5}{R}+\frac{5}{R}=\frac{25}{R}\)
∴ \(\frac { R }{ R’ }\) = 25

Question 2.
Which of the following terms does not represent electrical power in a circuit?
(a) I²R
(b) IR²
(c) VI
(d) \(\frac{V^2}{R}\)
Answer:
(b) IR²
Hint: Electric power P = VI = (IR) I = I²R
Thus, the three expressions given in (a), (c) and (d) represent power while expression given in (b) does not.

Question 3.
An electric bulb is rated 220 V and 100 W. When it is operated on 110 V, the power consumed will be ……………..
(a) 100 W
(b) 75 W
(c) 50 W
(d) 25 W
Answer:
(d) 25 W
Hint: Here, the power rating of the electric bulb is P = 100 W and the voltage rating is V = 220 V
So, the resistance of the filament of the bulb is
R = \(\frac{V^2}{P}\)
= \(\frac{(220)^2}{100}\)
= 484 Ω

Now, the power consumed by the bulb when it is operated at 110 V is given by
P = \(\frac{V^{\prime 2}}{R}\)
= \(\frac{(110)^2}{484}=\frac{110 \times 110}{484}\)
= 25 W

Question 4.
Two conducting wires of the same material and of equal lengths and equal diameters are first connected in series and then parallel in a circuit across the same potential difference. The ratio of heat produced in series and parallel combinations would be-
(a) 1 : 2
(b) 2 : 1
(c) 1 : 4
(d) 4 : 1
Answer:
(c) 1 : 4
Hint: As both the wires are made of the same material and are of equal lengths and equal diameters, they have the same resistance R.
1. When they are connected in series their equivalent resistance Rs would be –
R_s = R + R = 2R
and when they are connected in parallel their equivalent resistance R_p is given by
\(\frac{1}{R_{\mathrm{p}}}=\frac{1}{R}+\frac{1}{R}=\frac{2}{R}\)
∴ R_p = \(\frac { R }{ 2 }\)

2. Now, heat produced in time t is H = \(\frac{V^2 t}{R}\). As here source voltage V is same,
When wires are connected in series,
H_s = \(\frac{V^2 t}{R_{\mathrm{s}}}=\frac{V^2 t}{2 R}\)
When wires are connected in parallel
∴ H_p = \(\frac{V^2 t}{R_{\mathrm{p}}}=\frac{V^2 t}{\left(\frac{R}{2}\right)}=\frac{2 V^2 t}{R}\)
The ratio of heat produced,
\(\frac{H_{\mathrm{s}}}{H_{\mathrm{p}}}=\frac{V^2 t}{2 R} \times \frac{R}{2 V^2 t}\) = \(\frac { 1 }{ 4 }\)
∴ H_s : H_p = 1 : 4

Question 5.
How is a voltmeter connected in the circuit to measure the potential difference between two points?
Answer:
A voltmeter is (always) connected in parallel across the points in the circuit between which the potential difference is to be measured.

Question 6.
A copper wire has diameter 0.5 mm and resistivity of 1.6 x 10^-8 Ωm. What will be the length of this wire to make its resistance 10 Ω? How much does the resistance change if the diameter is doubled?
Solution:
We are given,
the diameter of the wire d = 0.5 mm = 0.5 x 10^-3 m = 5 x 10^-4 m
Resistivity of copper ρ = 1.6 x 10^-8 Ω m
Required resistance R= 10 Ω
Length l = ?

R ∝ \(\frac { 1 }{ d² }\) (If there is no change in p and L)
So, when diameter d is doubled, then resistance R becomes one-fourth of its original value.

Question 7.
The values of current I flowing in a given resistor for the corresponding values of potential difference V across the resistor are given below:

I (amperes)	0.5	1.0	2.0	3.0	4.0
V (volts)	1.6	3.4	6.7	10.2	13.2

Plot a graph between V and I and calculate the resistance of that resistor.
Answer:
The graph between V and I plotted by using the given data is shown below:

Calculation of the resistance of given resistor :

Question 8.
When a 12 V battery is connected across an unknown resistor, there is a current of 2.5 mA in the circuit. Find the value of the resistance of the resistor.
Solution:
Here, V = 12 V; I = 2.5 mA = 2.5 m A = 2.5 x 10^-3 A; R = ?
The resistance of the resistor R = \(\frac { V }{ I }\)
= \(\frac { 1 }{ 2 }\)
= 4800 Ω
= 4.8 k Ω

Question 9.
A battery of 9 V is connected in series with resistors of 0.2 Ω, 0.3 Ω, 0.4 Ω, 0.5 Ω and 12 Ω respectively. How much current would flow through the 12 Ω resistor?
Solution:
Since all the given resistors are connected in series, their equivalent resistance R_s = 0.2 + 0.3 + 0.4 + 0.5 + 12 = 13.4 Ω The current through the circuit,
I = \(\frac{V}{R_{\mathrm{s}}}=\frac{9}{13.4}\) = 0.67 A
In a series combination, the same current I flows through all the resistors, so the current flowing through 12 Ω resistor = 0.67 A.

Question 10.
How many 176 Ω resistors (in parallel) are required to carry 5A on a 220V line?
Solution :
Here, I = 5 A; V = 220 V
So, the total resistance of the given circuit is
R_total = \(\frac { V }{ I }\) = \(\frac { 220 }{ 5 }\) = 44 Ω
i.e., when 44 Ω resistance is connected with 220 V line, 5 A current would flow through the given circuit.
Now, suppose ‘n’ resistors, each of resistance R, are required to be connected in parallel, so that the total resistance R_total becomes 44 Ω.
Hence, \(\frac{1}{R_{\text {total }}}=\frac{1}{R}+\frac{1}{R}\) + … n times
= \(\frac{1+1+\ldots n \text { times }}{R}=\frac{n}{R}\)
∴ R_total = \(\frac { R }{ n }\)
Now, R_total = 44 Ω and R = 176 Ω
So, 44 = \(\frac { 176 }{ 44 }\)
∴ n = \(\frac { 176 }{ 44 }\) = 4
Thus, 4 resistors each of 176 Ω connected in parallel will result in total resistance of 44 Ω causing a current of 5 A to flow when connected to 220 V line.

Question 11.
Show how you would connect three resistors, each of resistance 6 Ω, so that the combination has a resistance of (i) 9 Ω, (ii) 4 Ω.
Solution:
(i) In order to get a resistance of 9 Ω from three resistors, each of resistance 6 Ω, we connect two 6 Ω resistors in parallel and this parallel combination is connected in series with the third 6 Ω resistor as shown in the following figure:

(ii) In order to get a resistance of 4 Ω from three resistors, each of resistance 6 Ω, we connect two 6 Ω resistors in series and this series combination is connected in parallel to the third 6 Ω resistor as shown in the following figure:

Question 12.
Several electric bulbs designed to be used on a 220 V electric supply line are rated 10W. How many bulbs can be connected in parallel with each other across the two wires of 220 V line if the maximum allowable current is 5 A?
Solution:
Here, the voltage rating of each bulb is 220 V and the power rating of each bulb is 10W.
So, the resistance of each bulb
R = \(\frac{V^2}{\rho}=\frac{220^2}{10}=\frac{220 \times 220}{10}\) = 4840 Ω
Now, here, V = 220 V and I = 5 A given.
So, the total resistance of the given circuit is
R_total = \(\frac { V }{ I }\) = \(\frac { 220 }{ 5 }\) = 44 Ω
i.e., When 44 Ω resistance is connected with 220 V line, 5 A current would flow through the given circuit.
Now, when ‘n’ bulbs each of resistance R, are connected in parallel, their equivalent
resistance P_total = \(\frac { R }{ n }\)
Hence, 44 = \(\frac { 4840 }{ 44 }\)
∴ n = \(\frac { 4840 }{ 44 }\) = 110
Thus, 110 bulbs each of resistance 4840 Ω connected in parallel will result in total resistance of 44 Ω causing a current of 5 A to flow, when connected to the 220 V line.

Question 13.
A hot plate of an electric oven connected to a 220 V line has two resistance coils A and B, each of 24 Ω resistance, which may be used separately, in series, or in parallel. What are the currents in the three cases?
Solution:
Here, the potential difference V = 220 V
The resistance of each coil R_A = R_s = 24 Ω
(1) When each of the coils A or B is connected separately, the current through each coil is
I = \(\frac{V}{R_{\mathrm{A}}}\) or \(\frac{V}{R_{\mathrm{B}}}\)
= \(\frac { 220 }{ 24 }\)
= 9.166 A

(2) When the coils A and B are connected in series, the equivalent resistance of the circuit R_s = R_A + R_B = 24 + 24 = 48 Ω
So, the current through the series combination
I_s = \(\frac{V}{R_{\mathrm{s}}}\)
= \(\frac { 220 }{ 48 }\)
= 4.58 A ≈ 4.6 A

(3) When the coils A and B are connected in parallel, the equivalent resistance R_p of the circuit is given by
\(\frac{1}{R_{\mathrm{p}}}=\frac{1}{R_{\mathrm{A}}}+\frac{1}{R_{\mathrm{B}}}=\frac{1}{24}+\frac{1}{24}=\frac{2}{24}\)
∴ R_p = 12 Ω
So, the current through the parallel combination
I_p = \(\frac{V}{R_{\mathrm{p}}}=\frac{220}{12}\) = 18.33 A

Question 14.
Compare the power used in the 2 Ω resistor in each of the following circuits:
(i) a 6 V battery in series with 1 Ω and 2 Ω resistors
(ii) a 4 V battery in parallel with 12 Ω and 2 Ω resistors.
Solution:
(i) As 1 Ω resistor and 2 Ω resistor are connected in series, the equivalent resistance R_s = 1 + 2 = 3 Ω
Now, the voltage of the battery V = 6 V
So, the current flowing through the circuit,
I_s = \(\frac{V}{R_{\mathrm{s}}}=\frac{6}{3}\) = 2 A
In a series combination the same current 2 A flows through each resistor. Hence the current flowing through 2 Ω resistor is also 2 A.
∴ Power used in 2Ω resistor,
P₁ = I²_sR
= (2)² x 2 = 8 W

(ii) As 12 Ω resistor and 2 Ω resistor are connected in parallel and 4 V battery is connected in parallel with this parallel combination of resistors,
The p.d. across 2 Ω resistor will also be 4 V.
∴ Power used in 2 Ω resistor,
P₂ = \(\frac{V^2}{R}=\frac{4^2}{2}=\frac{16}{2}\) = 8 W
In order to compare the power used in 2 Ω resistor in two different circuits, find the ratio of P₁ and P₂.
So, \(\frac{P_1}{P_2}=\frac{8}{8}\) = 1
∴ P₁ = P₂
Hence, 2 Ω resistor uses equal power, i.e., 8 W in both the circuits.

Question 15.
Two lamps, one rated 100 W at 220 V, and the other 60 W at 220 V are connected in parallel to electric mains supply. What current is drawn from the line if the supply voltage is 220 V?
Solution :
The resistance of 100 W lamp,
R₁ = \(\frac{V^2}{P_1}=\frac{220^2}{100}=\frac{220 \times 220}{100}=\frac{4840}{10}\)Ω = 484 Ω
The resistance of 60 W lamp,
R₂ = \(\frac{V^2}{P_2}=\frac{220^2}{60}=\frac{220 \times 220}{60}=\frac{4840}{6}\)Ω = 806.7 Ω
When, these lamps are connected in parallel, their equivalent resistance R_p is given by
\(\frac{1}{R_p}=\frac{1}{R_1}+\frac{1}{R_2}=\frac{R_2+R_1}{R_1 R_2}\)
∴ R_p = \(\frac{R_1 R_2}{R_1+R_2}\)
= \(\frac{484 \times 806.7}{484+806.7}=\frac{390442.8}{1290.7}\) = 302. 6 Ω
The current drawn from the line,
I = \(\frac{V}{R_{\mathrm{p}}}=\frac{220}{302.6}\) = 0.7270 ≈ 0.73 A

Question 16.
Which uses more energy, a 250 W TV set in 1 h, or a 1200 W toaster in 10 minutes?
Solution:
For TV set:
Power P = 250 W = 250 \(\frac { J }{ s }\)
Time t = 1 h = 3600 s
Electric energy (used by TV set)
= P x t
= 250 \(\frac { J }{ 2 }\) x 3600 s
= 900000
= 900 kJ
For Toaster:
Power P = 1200 W = 1200\(\frac { J }{ s }\)
Time t = 10 minute = 10 x 60 = 600 s
Electric energy (used by toaster)
= P x t
= 1200 \(\frac { J }{ s }\) x 600 s = 720000 = 720 kJ s
From above calculations, it is clear that a 250 W TV set in 1 h consumes more electric energy than a 1200 W toaster in 10 minute.

Question 17.
An electric heater of resistance 8 Ω draws 15 A from the service mains for 2 hours. Calculate the rate at which heat is developed in the heater.
Solution :
Here, I = 15 A; R = 8 Ω; t = 2 h
The rate at which heat is developed in the
heater means its electric power,
P = I² R
= (15)² x 8 = 225 x 8 = 1800 W = 1800 \(\frac { J }{ s }\)

Question 18.
Explain the following:
(a) Why is the tungsten used almost exclusively for filament of electric lamps?
(b) Why are the conductors of electric heating devices, such as bread-toasters and electric irons, made of an alloy rather than a pure metal?
(c) Why is the series arrangement not used for domestic circuits?
(d) How does the resistance of a wire vary with its area of cross-section?
(e) Why are copper and aluminium wires usually employed for electricity transmission?
Answer:
(a) Because tungsten has a high melting point (3380 °C), It does not melt at high temperature. It retains as much of heat generated, so that it becomes very hot and emits light without melting away.

Moreover, tungsten has high flexibility and low rate of evaporation at high temperature. That is the reason why tungsten is used as filament of electric lamps.

(b) The coils of electrical heating devices such as electric toasters and electric irons are made of an alloy, e.g., Nichrome rather than a pure metal because :

• the resistivity of an alloy e.g., Nichrome is much higher than that of its constituent metals, c
• alloys do not oxidise (i.e., burn) readily at high temperature (i.e., when it is red hot at 800 °C)
  alloy has a high melting point.

(c) (1) In a series circuit the current is constant throughout the electric circuit. So it s is obviously impracticable to connect an electric
bulb and an electric heater in series because they need currents of widely different values to operate properly.

(2) In a series circuit when one component (or electrical appliance) fails due to some defect, the circuit is broken and none of the components (or electrical appliances) works.

(3) In a series circuit all the electrical appliances have only one switch due to which they cannot be turned ON or OFF separately.

(4) In a series circuit electrical appliances of different power ratings do not get the same voltage (220V) as that of the power supply line because the voltage is shared by all the appliances. The appliances get less voltage and hence do not work properly.

(d) The resistance of a wire is inversly proportional to its area of cross-section
i.e., R ∝ \(\frac { 1 }{ A }\)
Thus, if the wire is thick (large area of cross-section), then its resistance is less. If the wire is thin (less area of cross-section), then its resistance is large.

(e) Because:

• Copper and aluminium have low electric resistivity, so they conduct electric current without heavy heat losses (due to which they are known as very good conductors of electricity).
• Copper and aluminium are much more easily and cheaply available as compared to metal like silver.
  They can be easily made into wires due to high malleability.

Therefore, copper and aluminium wires are usually employed for electricity transmission.

Jharkhand Board Class 10 Science Electricity InText Questions and Answers

Question 1.
What does an electric circuit mean?
Answer:
An electric circuit is a continuous and closed path of an electric current.
OR
A continuous and closed path consisting of conducting wires and other electrical components along which an electric current flows is called an electric circuit.

Question 2.
Define the unit of current.
Answer:
The SI unit of current is called an ampere (A).
If 1 coulomb charge flows through any cross-section of a conductor in 1 second, then the electric current flowing through the conductor is
said to be 1 ampere.
i.e., 1 A = \(\frac { 1C }{ 1s }\) = 1C s^-1

Question 3.
Calculate the number of electrons constituting one coulomb of charge.
Solution :
We know that one electron possesses a negative charge of 1.6 x 10^-19 C.
i.e., charge on 1 electron = – 1.6 x 10^-19 C

∴ No. of electron constituting – 1 C of charge
= \(\frac{-1 C \times 1}{-1.6 \times 10^{-19} \mathrm{C}}\)
= \(\frac { 10 }{ 1.6 }\) x 10¹⁸ = 6.25 x 10¹⁸
Thus, 6.25 x 10¹⁸ electrons taken together constitute 1 coulomb of charge.
In other words, the SI unit of electric charge coulomb (C) is equivalent to the charge contained in 6.25 x 10¹⁸ electrons.

Question 4.
Name a device that helps to maintain a potential difference across a conductor.
Answer:
An electric cell or battery is a device that helps to maintain a potential difference across a conductor.

Question 5.
What is meant by saying that the potential difference between two points is 1 V?
Answer:
The potential difference between two points (in a electric field) is said to be 1 volt if 1 J of work is done to move a charge of 1 C from one point to another point.
1 V = \(\frac { 1 J}{ 1 C }\)

Question 6.
How much energy is given to each coulomb of charge passing through a 6 V battery?
Solution:
Here the term, ‘each coulomb’ means ‘every 1 coulomb’. So Q = 1 coulomb, potential difference V = 6 volt, Energy = work done, W = ?
Now, W = VQ
= 6V x 1C
= 6 J
Since the work done on each coulomb of charge is 6 J, 6 J energy is given to each coulomb of charge passing through a 6V battery.

Question 7.
On what factors does the resistance of a conductor depend?
Answer:
The resistance R of a conductor depends on :

• its length l as R ∝ l
• its area of cross-section (i.e., thickness of the conductor) as R ∝ \(\frac { 1 }{ A }\)
• the nature of the material of the conductor (i.e., resistivity of material of the conductor)
• its temperature

Question 8.
Will current can flow more easily through a thick wire or a thin wire of the same material, when connected to the same source? Why?
Answer:
The current will flow more easily through a thick wire than through a thin wire of the s same material and having the same length when connected to the same source.

• Because, the resistance of a wire is inversly proportional to its area of cross-section, A thick wire has more area of cross-section and hence less resistance compared to a thin wire provided the two wires have the same length.
• Hence, a current can flow more easily through a thick wire compared to a thin wire when connected to the same source.

Question 9.
Let the resistance of an electrical component remain constant while the protential difference across the two ends of the component decreases to half of its former value. What change will occur in the current through it?
Answer:
According to Ohm’s law, I = \(\frac { V }{ R }\)
Here, as R = constant, I ∝ V.
So, when the potential difference across the two ends of the electrical component decreases to half of its former value, the current through it will also decreases to half of its former value.

Question 10.
Why are coils of electric toasters and electric irons made of an alloy rather than a pure metal?
Answer:
The coils of electrical heating devices such as electric toasters and electric irons are made of an alloy, e.g., Nichrome rather than a pure metal because :

• the resistivity of an alloy e.g., Nichrome is much higher than that of its constituent metals.
• alloys do not oxidise (i.e., burn) readily at high temperature (i.e., when it is red hot at 800 °C)
• alloy has a high melting point.

Question 11.
Use the data in Table 2 to answer the following:
(a) Which is a better conductor, iron or mercury?
(b) Which material is the best conductor?
Answer:
(a) The electrical resistivity of iron is 10.0 x 10^-8 Ω m whereas that of mercury is 94.0 x 10″8fim. As the resistivity of iron is less than that of mercury, iron (Fe) is a better conductor than mercury (Hg).

(b) Silver metal has the lowest electrical resistivity of 1.60 x 10^-8 Ω m, therefore silver metal is the best conductor of electricity.

Question 12.
Draw a schematic diagram of a circuit consisting of a battery of three cells of 2 V each, a 5Ω resistor, an 8 Ω resistor, and a 12 Ω resistor, and a plug key, all connected in series.
Answer:
A schematic diagram of a circuit consisting of a battery of three cells of 2 V each, a 5 Ω resistor, an 8 Ω resistor, and a 12 Ω resistor, and a plug key, all connected in series is shown in figure 12.12

Question 13.
Redraw the circuit of putting in an ammeter to measure the current through the resistors and a voltmeter to measure the potential difference across the 12 Ω resistor. What would he the readings in the ammeter and the voltmeter?
Answer:
The circuit diagram of above with an ammeter A and a voltmeter V across 12 Ω resistor is shown in figure 12.13.

Calculation of current flowing in the circuit:
Equivalent resistance of the circuit,
R_s = 5 Ω + 8 Ω + 12 Ω = 25 Ω
Potential difference V = 6 volt
In a series combination, the current flowing through each resistor is the same and equal to the total current flowing through the circuit.
∴ Current through the resistors,
I = \(\frac { V }{ R }\)
= \(\frac { 6 }{ 25 }\)
= 0.24 A
Since the current in the circuit and the current through each resistor is the same, the ammeter will show a reading of 0.24 A.
Calculation of potential difference across 12 Ω resistor:
As total current 0.24 A flows in the circuit, the same current 0.24 A would also flow through the 12 Ω resistor which is connected in series.
∴ The potential difference across the 12 Ω resistor,
V = IR
= 0.24 x 12 = 2.88 V
Thus, the p.d. across 12 Ω resistor is 2.88 volt.
So, the voltmeter will show a reading of 2.88 V.

Question 14.
Judge the eΩuivalent resistance when the following are connected in parallel:
(a) 1 Ω and 10⁶ Ω
(b) 1 Ω, 10³ Ω and 10⁶Ω
Answer:
(a) Less than 1 Ω (but approximately 1 Ω)
(b) less than 1 Ω (but approximately 1 Ω)
Explanation: When resistors are connected in parallel, the equivalent resistance is less than the least resistance (here in both the cases it is 1 Ω) connected in the combination.
or
(a) Here, R₁ = 1 Ω and R₂ = 10⁶ Ω
The equivalent resistance R_p of the parallel combination is given by,
\(\frac{1}{R_{\mathrm{p}}}=\frac{1}{R_1}+\frac{1}{R_2}\)
∴ R_p = \(\frac{R_1 \times R_2}{R_1+R_2}\)
= \(\frac{1 \times 10^6}{1+10^6}\)
= \(\frac { 1000000 }{ 1000001 }\) ≈ 1 Ω

(b) Here, R₁ = 1 Ω, R₂ = 10³ Ω and R₃ = 10⁶
The equivalent resistance Rp of the parallel combination is given by,

Question 15.
An electric lamp of 100 Ω, a toaster of resistance 50 Cl, and a water filter of resistance 500 Ω are connected in parallel to a 220 V source. What is the resistance of an electric iron connected to the same source that takes as much current as all three appliances and what is the current through it?
Solution :
Here, Resistance of an electric lamp R₁ = 100 Ω
Resistance of a toaster R₂ = 50 Ω
Resistance of a water filter R₃ = 500 Ω.
Equivalent resistance R_p of three resistors R₁, R₂ and R₃ connected in parallel is given by,

Total current through the circuit (i.e., through all the three appliances)
I = \(\frac{V}{R_p}\)
= \(\frac { 220 }{ 31.25 }\)
= 7.04 A
Since the electric iron connected to the same source (i.e., 220 V) takes as much current as taken by all the three appliances (i.e., f = 7.04A), its resistance must be equal to R_p.
So, the resistance of the electric iron = 31.25 Ω
and the current through the electric iron = 7.04 A

Question 16.
What are the advantages of connecting electrical devices in parallel with the battery instead of connecting them in series?
Answer:
The advantages of connecting electrical devices in parallel with the battery instead of connecting them in series are as follows :
(1) A parallel combination / circuit is helpful when each device has different resistance and requires different current for its operation, as in this case the total resistance of the circuit is reduced due to which the current from the battery is high and hence each electrical device can draw the required amount of current.

This is not so in a series combination / circuit because total resistance increases too much in series circuit due to which the current from the battery is low and the same low current flows through all the devices, irrespective of their resistances and hence devices cannot work properly.

(2) In a parallel combination / circuit each electrical device gets the same p.d. (potential difference) across it as that of the battery due to which all the devices work properly.

While in case of a series combination / circuit the devices do not get the same p.d. as that of the battery because the p.d. is shared by all the devices connected in series.

(3) In a parallel combination / circuit if one electrical device stops working due to some defect, then other devices are not affected. They continue to work without any problem.

On the other hand, in a series combination/ circuit if one electrical device stops working due to some defect, then all other devices also stop working as the whole circuit is broken.

(4) In a parallel combination / circuit each electrical device has its own switch due to which it can be turned ‘ON’ or turned ‘OFF’ independently without affecting other devices.

But in a series combination / circuit all the electrical devices have only one switch due to which they cannot be turned ‘OFF’ or turned ‘ON’ independently.

Question 17.
How can three resistors of resistances 2 Ω, 3 Ω and G Ω be connected to give a total resistance of (a) 4 Ω, (b) 1 Ω?
Answer:
(a) In order to obtain a total resistance of 4 Ω from three resistors of 2 Ω, 3Ω and 6Ω…
1. First connect the two resistors of 3 Ω and 6 Ω in parallel to get a total resistance of 2 Ω. This is because in parallel combination,

2. Now, above parallel combination of 3 Ω and 6 Ω resistors is connected in series with the remaining 2 O resistor to get total resistance of 4 Cl. This is because in series combination.
R_s = R_p + R₃
= 2 + 2
= 4 Ω
Hence, the arrangement of three resistors 2 Ω, 3 Ω and 6 Ω which gives total resistance 4 Ω can be represented as follows :

(b) In order to obtain a total resistance of 1 Ω from three resistors of 2 Ω, 3 Ω and 6 Ω, all the three resistors should be connected in parallel. This is because in a parallel combination,

Hence, the arrangement of three resistors 2 Ω, 3 Ω and 6 Ω which gives total resistance 1 Ω can be represented as follows :

Question 18.
What is (a) the highest, (b) the lowest total resistance that can be secured by combinations of four coils of resistances 4 Ω,
8 Ω, 12 Ω, 24 Ω?
Solution:
(a) The highest resistance can be secured (or obtained) by connecting all the four coils in series. In this case,
R_s = R₁+R₂ + R₃ + R₄
= 4 + 8 + 12 + 24 = 48 Ω
Thus, the highest resistance which can be secured is 48 Ω.

(b) The lowest resistance can be secured by connecting all the four coils in parallel. In this cae,

Thus, the lowest resistance which can be secured is 2 Ω.

Question 19.
Why does the cord of an electric heater not glow while the heating element does?
Answer:
The heating element of an electric heater is made of an alloy (such as Nichrome) which has high resistance (partly due to high resistivity) whereas the cord is made of copper metal which has very low resistance (partly due to low resistivity).

• Now, the heating element of an electric heater made of Nichrome glows because it becomes red-hot due to the large amount of heat (according to H = I²Rt) is produced on passing current.
• On the other hand, the connecting cord of the electric heater made of copper does not glow because relatively very less (negligible) heat (according to H = I²Rt) is produced in it by passing the same current.

Question 20.
Compute the heat generated while transferring 96000 coulomb of charge in one hour through a potential difference of 50 V.
Solution:
Here, charge, Q = 96000 C,
time t = 1 h = 60 x 60 = 3600 s,
potential difference V = 50 V
Now,
Heat generated H = VIt
= V(\(\frac { Q }{ t }\))t (∵ I = \(\frac { Q }{ t }\))
= 50 x 96000
= 4800000 J
= 4.8 x 10⁶ J
or
= 4.8 x 10³ x 10³ J
= 4.8 x 10³ kJ
= 4800 kJ

Question 21.
An electric iron of resistance 20 Ω takes a current of 5 A. Calculate the heat devloped in 30 s.
Solution :
Here, current I = 5 A,
resistance R = 20 Ω, time t = 30 s
Now,
Heat produced H = I²Rt
= (5)² x 20 x 30
= 25 x 20 x 30
= 15000 J
= 15 kJ

Question 22.
What determines the rate at which energy is delivered by a current?
Answer:
The electric power of the source determines the rate at which energy is delivered by the current to the load / appliance.
[Whereas the electric power of an appliance determines the rate at which energy delivered by a current Is consumed by appliance.]

Question 23.
An electric motor takes 5 A from a 220 V line. Determine the power of the motor and the energy consumed in 2h.
Solution:
Here, I = 5A, V = 220V,
t = 2h = (2 x 60 x 60)s = 7200s
Power P = VI = 220 x 5 = 1100W = 1100J/s
Now,
Energy consumed W = Pt = 1100J/s x 7200s
= 7920000
= 7.92 x 106J
OR
Energy consumed W = Pt = 1100 w x 2 h
= 2200 Wh
= 2.2 x 10³ Wh
= 2.2 k Wh

Activity 12.1 [T. B. Pg. 203]

To verify Ohm’s law.
OR
To find the relationship between potential difference (V) and current (I).

Procedure:
1. Set up a circuit as shown in figure 12.3, consisting of a +Nichrome wire XY of length say 0.5 m, an ammeter, a voltmeter and four cells of 1.5 V each.

2. First use only one cell as the source in the circuit.
Note the reading of ammeter I, for the current and reading of the voltmeter V for the potential difference across the Nichrome wire XY in the circuit.
Tabulate them in the Table given.

3. Next connect two cells in the circuit and note the respective readings of the ammeter and voltmeter for the values of current through the Nichrome wire and potential difference across the Nichrome wire.

4. Repeat the above steps using three cells and then four cells in the circuit separately.
Calculate the ratio V to I for each pair of potential difference V and current I.

Observation table :

Sr. No.	Number of cells used in the circuit	Potential difference across the Nichrome wire V (volt)	Current through the Nichrome wire I (ampere)	\(\frac { V }{ I }\) (volt / ampere)
1.	1	1.5	0.1	15
2.	2	3	0.2	15
3.	3	4.5	0.3	15
4.	4	6	0.4	15

Plot a graph between V and I and observe the nature of the graph.
(Take the voltage (V) on X-axis and the current (J) on Y-axis. Draw the graph of I-V taking proper scale.)

Observation:
When V increases I also increases linearly, i.e., I ∝ V. The ratio V/I is found to be (approximately) the same, i.e., 15V/A.
The graph between V and 1 is a straight line passing through the origin O.

Conclusion:
The electric current flowing through a metallic wire is directly proportional to the potential difference across its ends (J ∝ V) and V/I is a constant ratio in particular case.

Activity 12.2 [T. B. Pg. 205]

To show that the strength of an electric current in a circuit depends on resistance used in it.

Procedure:
1. Take a Nichrome wire, a torch bulb, a low bulb (10W) and an ammeter (0-5A range), a plug key and some connecting wires.

2. Set up the circuit by connecting four dry cells of 1.5 V each in series with the ammeter leaving a gap XY in the circuit, as shown in figure 12.5.

3. Complete the circuit by connecting the Nichrome wire in the gap XY. Plug the key.
Note down the ammeter reading. Take out the key from the plug after measuring the current through the circuit.
[Note: Always take out the key from the plug after measuring the current through the circuit.]

4. Replace the Nichrome wire with the torch bulb in the circuit and find the current through it by measuring the reading of the ammeter.

5. Now repeat the above step with the 10 W bulb in the gap XY.

• Are the ammeter readings different for different s components connected in the gap XY?
• What do the above observations indicate?

6. You may repeat this Activity by keeping any material component in the gap.
Observe the ammeter readings in each case. Analyse the observations.

Observation :

• Yes, the ammeter readings are different for different components (Nichrome wire, a torch bulb and a low bulb) when connected in the gap XY.
• If we take any other material component in the gap XY, the reading of the ammeter may be different.
• This is because certain components offer an easy path for the flow of electric current while the others resist the flow.
• i.e., the current through an electric component depends on its resistance.

Conclusion :
The strength of an electric current in a circuit depends on the resistance used in it.

• A component of a given size that offers low resistance is called a good conductor.
• A component of the same size which offers appreciable resistance is called a resistor.
• A component of the same size that offers a higher resistance is called a poor conductor or a bad conductor.
• A component of the same size that offers very high resistance is called an insulator.

Activity 12.3 [T. B. Pg. 206]

To study the factors on which resistance of conducting wire depends.

Procedure:

• Complete an electric circuit consisting of a cell, an ammeter, a Nichrome wire of length l [say, marked (1)] and a plug key, as shown in figure 12.6.
  
• Now, plug the key. Note the current in the ammeter.
• Replace the Nichrome wire by another Nichrome wire of same thickness but twice the length, that is 21 [marked (2) in the figure],
• Note the ammeter reading.
• Now replace the wire by a thicker Nichrome wire, of the same length l [marked (3)]. A thicker wire has a larger cross-sectional area. Again note down the current through the circuit.
• Instead of taking a Nichrome wire, connect a copper wire [marked (4) in the figure] in the circuit.
• Let the wire be of the same length and same area of cross-section as that of the first Nichrome wire [marked (1)]. Note the value of the current.
• Notice the difference in the current in all cases,
• Does the current depend on the length of the conductor ?
• Does the current depend on the area of s cross-section of the wire used ?

Observations :

• Ammeter shows the current I flows in the Nichrome wire marked (1).
• When the length of Nichrome wire marked (2) is doubled keeping its same thickness (i.e., when the Nichrome wire marked (1) is replaced by the Nichrome wire marked (2) the ammeter reading decreases to half, i.e. current becomes 1/2.
• When the thickness of Nichrome wire marked (3) is increased keeping its length same i.e., when the Nichrome wire marked (3) is S connected in the circuit), the ammeter reading increases, i.e., current I increases.
• When Nichrome wire is replaced by a copper s wire marked (4) of same length and same cross-sectional area as marked (1), the ammeter reading increases, i.e., current I increases.

Conclusion :

• Resistance of the conducter depends on its s length. Here resistance of wire R is directly proportional to length l, i.e., R ∝ l.
• Resistance of the conductor depends on its area of cross-section. Here resistance of wire R is inversely proportional to area of cross-section A, i.e., R ∝ \(\frac { 1 }{ 2 }\)
• Copper is a better conductor than Nichrome. Resistance of wire depends on the nature of S its material.

Activity 12.4 [T. B. Pg. 210]

To study the series combination of resistors.

Procedure :

• Join three resistors of different values in series. Connect them with a battery, an ammeter and a plug key, as shown in figure 12.9.
• You may use the resistors of values like 1 Ω, 2 Ω, 3 Ω, etc. and a battery of 6 V for performing this Activity.
• Plug the key. Note the ammeter reading. [part (1)]
• Change the position of the ammeter to anywhere inbetween the resistors. Note the ammeter reading each time, [part (2)]
• Do you find any change in the value of current through the ammeter?
  

Observation :

• The ammeter reading is nearly 1 A.
• When the position of the ammeter is changed to anywhere inbetween the resistors, its reading remains the same, i.e., the current flowing through it and through each part of the circuit, i.e., through each resistor is the same.
• No, current flowing through the ammeter does not change.

Conclusion :

• The value of the current in the ammeter is the same, independent of its position in the electric circuit.
• In a series combination of resistors the current is the same in every part of the circuit, i.e., the same current flows through each resistor. This is due to the reason that, the current has only one path to flow.

Important note:
(1) In discussion of Activity 12.4, ammeter and voltmeter are considered as ideal meters. (2) Ideally, an ammeter should have zero resistance and a voltmeter should have infinite resistance. But in practice, these conditions cannot be realized and hence practically the voltmeter draws some current from the main branch and so the ammeter reading in part (2) is slightly less than that in part (1).

Activity 12.5 [T. B. Pg. 211]

To show that in a series combination of resistors, the total potential difference across the combination divides itself across the individual resistors.

Procedure:

• In Activity 12.4, insert a voltmeter across the ends X and Y of the series combination of three resistors, as shown in figure 12.7.
• Plug the key in the circuit and note the voltmeter reading. It gives the potential difference across the series combination of resistors. Let it be V.
• Now measure the potential difference across the two terminals of the battery. Compare the two values.
• Take out the plug key and disconnect the voltmeter. Now insert the voltmeter across the ends X and P of the first resistor, as shown
  
• Plug the key and measure the potential difference across the first resistor. Let it be V₁
• Similarly, measure the potential difference across the other two resistors, separately. Let these values be V₂ and V₃, respectively.
• Deduce a relationship between V, V₁, V₂ and V₃.

Observation:

• The p.d. across the series combination is equal to the p.d. across the two terminals of the battery.
• p.d. across the first resistor R₁ is found out to be V₁ using voltmeter.
• Similarly with the help of voltmeter, p.d. across resistors R₂ and R₃ separately are found out to be V₂ and V₃ respectively.
• It is found out that V = V₁ + V₂ + V₃.

Conclusion:

• In a series combination of different resistors, the potential difference across different resistors is different, i.e., If R₁ ≠ R₂ ≠ R₃, V₁ ≠ V₂ ≠ V₃.
• The total p.d. across the combination is equal to the sum of p.d. across each of the resistors, i.e., total p.d. across the combination divides itself across the individual resistors, i.e., V is equal to V₁ + V₂ + V₃.

Important note:
In Activity 12.5 it is assumed that, R (ammeter) = zero and R (voltmeter) = ∞, so V₁ + V₂ + V₃, In practice, these conditions cannot be realized, hence practically V is (very nearly) equal to V₁ + V₂ + V₃.

Activity 12.6 [T. B. Pg. 213]

To study the parallel combination of resistors.
OR
To study the relationship between potential difference and respective resistance in parallel circuit as well as the relationship between s current and respective resistance in parallel circuit.

Procedure:
1. Make a parallel combination XY of three resistors having resistances R₁, R₂ and R₃, respectively. Connect it with a battery, a plug key and an ammeter, as shown in figure 12.14. Also connect a voltmeter in parallel with the combination of resistors.

2. Plug the key and note the ammeter reading. (Let the current be I).

3. Also take the voltmeter reading. (It gives the potential difference V, across the combination).

4. Connect a voltmeter across the resistor with resistance R₁ and note the voltmeter reading V₁ (see figure 12.14).

5. Similarly connect a voltmeter across the resistors with resistances R₂ and R₃ separately and note s the corresponding voltmeter readings V₂ and V₃.

6. Take out the plug from the key. Remove the ammeter and voltmeter from the circuit.

7. Insert the ammeter in series with the resistor s with resistance R₁, as shown in figure 12.15. Note the ammeter reading, I₁.

8. Similarly, measure the current through the resistors with resistances R₂ and R₃. Let these be I₂ and I₃, respectively.
What is the relationship between I, I₁, I₂ and I₃?

Observations :

• Ammeter showed the reading I when connected as shown in figure 12.14.
• Voltmeter showed reading V when connected as shown in figure 12.14.
• It is found that the p.d. across each resistor is the same (i.e., V), as across the combination of resistors, i.e., V₁ = V₂ = V₃ = V
• Ammeter showed reading I₁, when connected with the resistor R₁ as shown in figure 12.15.
• Similarly ammeter showed reading I₂ and I₃ measured separately through R₂ and R₃ respectively.
• The relationship between I, I₁, I₂ and I₃ is:
  I = I₁ + I₂ + I₃
  Where, I = Total current through the combination of resistors in parallel
  I₁, I₂, I₃ = Currents through R₁, R₂ and R₃ respectively.

Conclusion:

• In parallel combination of resistors, the potential difference across different resistances will be same.
• Total current I in the given parallel circuit is divided amongst different resistors.

Jharkhand Board JAC Class 10 Science Solutions Chapter 14 Sources of Energy Textbook Exercise Questions and Answers.

JAC Board Class 10 Science Solutions Chapter 14 Sources of Energy

Jharkhand Board Class 10 Science Sources of Energy Textbook Questions and Answers

Question 1.
A solar water heater cannot be used to get hot water on ………………
A. a sunny day
B. a cloudy day
C. a hot day
D. a windy day
Answer:
a cloudy day

Question 2.
Which of the following is not an example of a biomass energy source?
A. wood
B. gobar-gas
C. nuclear energy
D. coal
Answer:
nuclear energy

Question 3.
Most of the sources of energy we use represent stored solar energy. Which of the following is not ultimately derived from the Sun’s energy?
A. geothermal energy
B. wind energy
C. nuclear energy
D. biomass
Answer:
nuclear energy

Question 4.
Compare and contrast fossil fuels and the sun as direct source of energy.
Answer:

Fossil Fuel	Sun
It is non-renewable and exhaustible source of energy.	It is renewable and non-exhaustible source of energy.
It causes environmental pollution.	It is non-polluting source.
Fossil fuels have to be extracted for use.	It is easily available for most of the time and at most the places throughout the year in our country.
Sun is indirect source of energy in fossil fuel.	Nuclear fusion reactions are the source of energy in the sun.
It is conventional source of energy.	It is non-conventional source of energy.

Question 5.
Compare and contrast biomass and hydroelectricity as source of energy.
Answer:

Biomass	Hydroelectricity
It causes pollution.	It is pollution free source of energy.
It is less expensive.	It is expensive in terms of construction of dams.
It is in the form of wood, cow-dung cake, agricultural residue etc.	Potential energy of water is used to generate electricity.
A fuel gas (biogas) can be produced by using it.	No gas is produced by using it.

Question 6.
What are the limitations of extracting energy from (a) the wind? (b) waves? (c) tides?
Answer:

Energy Source	Limitations of extracting energy
(a) The wind	The required wind speed should be higher than 15 km/h, the initial cost of establishment of wind farm is high, large area is required, high level of maintenence for wind farm is needed.
(b) Waves	It is costly and difficult to manage, the waves are generated by strong winds blowing across the sea.
(c) Tides	There are limited locations where dams for to harness tidal energy can be built. Efficient commercial exploitation is difficult.

Question 7.
On what basis would you classify energy sources as…
(a) renewable and non-renewable?
(b) exhaustible and inexhaustible?
Are the options given in (a) and (b) the same?
Answer:

• Exhaustible : Fossil fuel, coal will get depleted some day.
• Inexhaustible : Wind, tidal, solar energy, etc. are in the form continuing or repetitive currents of energy.
• Renewable : Biomass, if we manage properly, it can give a constant supply of energy at a particular rate.
• Non-renewable : Fossil fuels as once used up, they are lost forever and cannot be renewed.
  Yes, options given in ( a) and (b ) are same.

Question 8.
What are the qualities of an ideal source of energy?
Answer:
A good source of energy has following properties :

• It would able to do a large amount of work per unit volume or mass.
• It can be easily accessible.
• It can be easy to store and transport.
• It can be economical.

It should be pollution free and should not leave any residue.

Question 9.
What are the advantages and disadvantages of using a solar cooker? Are there places where solar cookers would have limited utility?
Answer:
Using a solar cooker:

Advantages	Disadvantages
It does not cause any pollution.	It cannot be used at night and on cloudy days.
It uses renewable/ inexhaustible source of energy.	It takes comparati-vely more time for cooking
Food nutrients are maintained during cooking, because the food is cooked at a comparatively low temperature.	The position of miror which reflects sunrays need to be monitored and the direction has to be changed again and again.
	It cannot be used for frying and to cook chapattis.

Yes, the place where Sun shine/solar energy is insufficient, the utility of solar cooker would be limited. Also on a rainy and cloudy day solar cooker cannot work.

Question 10.
What are the environmental consequences of the increasing demand for energy? What steps would you suggest to reduce energy consumption?
Answer:
The energy demand is increasing day by day. Exploiting any source of energy may adversely affect the environment more or less.

For example, use of fossil fuels cause air pollution. It may lead to greenhouse effect, acid rain, etc. Use of Hydropower to generate electricity destroys large ecosystem.

Following steps are suggested to reduce energy consumption :

• Minimise the regular use of personal vehicles and use of public transport as far as possible.
• Maximise the use of pollution free source of energy.
• Use of eco-friendly fuels such as biogas, CNG, etc.
• Switch off the light, fan and other electric appliances, whenever not in use.
• Turn off the vehicles at traffic signal while waiting for green signal.
• Use of solar cooker, solar water heater.

Jharkhand Board Class 10 Science Sources of Energy InText Questions and Answers

Question 1.
What is a good source of energy?
Answer:
A good source of energy has following properties :

• It would able to do a large amount of work per unit volume or mass.
• It can be easily accessible.
• It can be easy to store and transport.
• It can be economical.

Question 2.
What is a good fuel?
Answer:
A good fuel is the one.

• Which burns completely without producing smoke or ash.
• Which produces large amount of heat while burning a small quantity of it.
• Which is easily available and economical.

Question 3.
If you could use any source of energy for heating your food, which one would you use and why?
Answer:
If we live in a village, gobar gas will be used as a fuel for heating our food because it has high calorific value and it is easily available and more economic.

If we live in a city, either LPG or microwave or oven can be used as fuel for heating our food, because LPG is pollution free. Microwave or oven is more preferable because nutritive value of food is maintained during heating our food.

Question 4.
What are the disadvantages of fossil fuels?
Answer:
The disadvantages of fossil fuels are :

• It is non-renewable source of energy.
• Air pollution is caused by burning of fossil fuels. Acidic oxides of nitrogen and sulphur are released on burning of fossil fuels which lead to acid rain.
• Gases like carbon dioxide causes green house effect and global warming.

Question 5.
Why are we looking at alternative sources of energy?
Answer:
We are looking at alternative sources of energy because the growing demand for energy was largely met by the fossil fuels. Our technologies were also developed for using fossil fuels i.e., coal and petroleum. The fossil fuels are non-renewable source of energy.

These fuels were formed over millions of years ago and there are only limited reserves. If we are to continue consuming these sources at such alarming rate, we would soon run out energy. To overcome this, alternative sources of energy are explored.

Question 6.
How has the traditional use of wind and water energy been modified for our convenience?
Answer:
The traditional use of wind energy has been modified by constructing wind fans and wind energy farm to generate electricity. The traditional use of water energy has been modified by constructing dams and convert the potential energy of falling water into electricity for our convenience.

Question 7.
What kind of mirror – concave, convex or plain – would be best suited for use in a solar cooker? Why?
Answer:
Use of concave mirror is best suited for solar cooker because it is a converging mirror that converges large amount of sun rays into the solar cooker.

Question 8.
What are the limitations of the energy that can be obtained from the oceans?
Answer:
The limitations of energy obtained from oceans are as follows :

• The locations, where dams can be built for tidal energy are limited.
• The wave energy is obtained only where strong winds are blowing accross the sea.
• Efficient commercial exploitation of ocean thermal energy is difficult.

Question 9.
What is geothermal energy?
Answer:
The heat energy which can be exploited from the steam trapped in rocks near the hot springs is called geothermal energy.

Question 10.
What are the advantages of nuclear energy?
Answer:
The advantages of nuclear energy are :

• It produces much more energy than the other conventional sources. For example, the fission of an atom of uranium produces 10 million times the energy produced by the combustion of an atom of carbon from coal.
• As compared to the space required to harness hydro energy, thermal energy, etc. less space is required to harness nuclear energy.

Question 11.
Can any source of energy be pollution free? Why or why not?
Answer:
Solar energy, wind energy, geothermal energy, etc. are considered as pollution free. But every time a source of energy is exploited or used, it causes envirnomental pollution. So, no source of energy can be pollution free.

Question 12.
Hydrogen has been used as a rocket fuel. Would you consider it a cleaner fuel than CNG? Why or why not?
Answer:
Yes, hydrogen is a cleaner fuel than CNG because hydrogen burnt in presence of oxygen produces water vapours (H₂O_(g)) whereas CNG that contain methane burns to produce carbon dioxide and carbon monoxide.

Question 13.
Name two energy sources that you would consider to be renewable. Give reasons for your choices.
Answer:

• Hydropower, since the water in the reservoir would be refilled each time it rains.
• Wind power, as wind keeps blowing due to unequal heating of the landmass and water bodies by solar radiation.

Question 14.
Give the names of two energy sources that you would consider to be exhaustible. Give reasons for your choices.
Answer:
Fossil fuels, i.e., coal and petroleum are exhaustible because once they are used up they are lost forever.

Activity 14.1 [T. B. Pg. 242]

Questions:

Question 1.
List four forms of energy that you use from morning, when you wake up, till you reach the school.
Answer:

• Muscular energy – To brush, exercise, take bath, to ride bicycle
• Electrical energy – To turn on geyser, to iron uniform, to put on the fan
• Fuel energy/PNG – To boil milk, cooking food; Transport – Going to school by bus / car
• Chemical energy / food – Breakfast, lunch

Question 2.
From where do we get these different forms of energy?
Answer:

Form of energy	Sources
Muscular energy	Energy stored in muscle cells in form of ATP
Electrical energy	From power plant
Fuel energy	From gas station
Chemical energy	From food

Question 3.
Can we call these sources of energy? Why or why not?
Answer:
Yes, we can call all these as sources of energy.

Activity 14.2 [T. B. Pg. 243]

Consider the various options we have when we choose a fuel for cooking our food.

Questions :

Question 1.
What are the criteria you would consider when trying to categorise something as a good fuel?
Answer:
The criteria to categorise good fuel for cooking our food are as follows :

• It should be easily available.
• It should be economical.
• It should not produce smoke or ash.
• It should have high efficiency.

Question 2.
Would your choice be different if you lived …
(a) in a forest?
(b) in a remote mountain village or small island?
(c) in New Delhi?
(d) lived five centuries ago?
Answer:

We live in	Our choice will be
(a) in a forest	wood, dry leaves
(b) in a remote mountain village or small island	wood, dry leaves, cow-dung cake
(c) in New Delhi	LPG, PNG
(d) lived five centuries ago	wood

Question 3.
How are the factors different in each case?
Answer:
In each case, the factors are different on the basis of its availability.

Activity 14.3 [T. B. Pg. 244]

To demonstrate the process of thermoelectric production with a model.

Apparatus and materials: Pressure cooker, pipe, tennis ball, metal sheet, dynamo, bulb.

Procedure:

• Take a table-tennis ball and make three slits into it.
• Put semicirucular fins cut out of a metal sheet into these slits.
• Pivot the tennis ball on an axle through its centre with a straight metal wire fixed to a rigid support.
• Ensure that the tennis ball rotates freely about the axle.
• Now connect a cycle dynamo to this.
• Connect a bulb in series.
• Direct a jet of water or steam produced in a pressure cooker at the fins.
• Note down your observation.
  

Observation : The bulb gets lighted.

Questions :

Question 1.
Which energy conversion do you think is taking place in this model?
Answer:
Heat energy gets converted to electrical energy in this model.

Question 2.
Which structure in the model acts as a turbine?
Answer:
Tennis ball fitted with metal sheets in the model acts as a turbine.

Question 3.
How electricity generated in this simple model?
Answer:
The heat given to pressure cooker produces steam. The steam is used to turn the tennis ball (the turbine). The tennis ball is further linked to a dynamo. Therefore, armature of a dynamo rotates and generates electricity.

Question 4.
What do the simplest turbines have?
Answer:
The simplest turbines have one moving part, a rotor assembly.

Question 5.
Which form of energy is essential in today’s life?
Answer:
Electrical energy is essential in today’s life.

Question 6.
What is your conclusion from this activity?
Answer:
Energy can be converted from one form to another.

Activity 14.4 [T. B. Pg. 248]

Find out from your grandparents or other elders

Questions :
(a) how did they go to school?
(b) how did they get water for their daily needs when they were young?
(c) what means of entertainment did they use?
Compare the above answers with how you do these tasks now.
Is there a difference? If yes, in which case more energy from external sources is consumed?
Answer:

Our grand parents	We are
(a) They went to school by walking.	(a) We use activa, a car or a school bus.
(b) They got water from wells, river, etc.	(b) We get water from bore by using submercible water pump.
(c) Social gathering, group meeting, drama, fairs, festival celebration, etc. were the means of their entertainment.	(c) We are using mobile phones, computers most of times. Besides it television, watching

Yes, today we consume much more energy. This is used for transport and day-to-day living.

Activity 14.5 [T. B. Pg. 249]

To demonstrate, “black surface absorbs more heat as compared to a white surface”.

Apparatus – Materials :
Conical flasks, water, thermometer

Procedure :

• Take two conical flasks and paint one white and the other black.
• Fill water in the both flask.
• Place the conical flasks in direct sunlight for half an hour to one hour.
• Measure the temperature of the water in both conical flask with a thermometer.

Questions :

Question 1.
If you touch the conical flasks, which one is hotter?
Answer:
Conical flask painted black is hotter.

Question 2.
Which property is used in solar cooker and solar water heater?
Answer:
A black surface absorbs more heat as compared to a white or a reflecting surface under identical conditions.

Question 3.
In which ways this finding can be used in our daily life?
Answer:
Wearing black coloured clothes in winter and white colour clothes in summer. White paint on the outer wall as well as white coating on terrace helps to maintain 2 – 3 °C low temperature inside the house during summer.

Activity 14.6 [T. B. Pg. 249]

To study the structure and working of a solar cooker and/or water heater.

Activity is done for understanding how the solar equipments are insulated and how does maximum heat absorption is ensured.

Property: A black surface absorbs more heat.

Structure :

• It consists of an insulated metal box or a wooden box which is painted black from inside.
• The box has a thick glass sheet as a cover over the box.
• A plane or concave mirror is attached to the box that acts as a reflector.
• Mirror focuses the rays of the sun into the box.

Questions :

Question 1.
Why is a glass sheet used?
Answer:
A glass sheet is used to creates greenhouse effect.

Question 2.
How is heat reflected into the box?
Answer:
By using mirror, rays of sun reflect the heat into the box.

Question 3.
State energy conversion in solar cooker/solar water heater.
Answer:
Light energy / solar energy in converted to heat energy.

Question 4.
What temperature is achieved in solar cooker / solar water heater?
Answer:
Typically solar cooker is designed to achieve 65 °C (150 °F) temperature. The temperature of water in the solar water heater is determined by the combination of collector area and the tank capacity. Typically it would be 50-60°C, which is much hotter than the bathing water temperature (around 40 °C).

Question 5.
State the advantages and limitations of using the solar cooker or solar water heater.
Answer:

Advantages	Limitations
1. Use of solar energy, which is available free of cost,	1. These devices are useful only at certain times during the day.
2. We can save fuel gas by using it.	2. These devices are not useful in cloudy days and at night.

Activity 14.7 [T. B. Pg. 252]

Discuss in the class following questions:

Question 1.
What is the ultimate source of energy for biomass, wind and ocean thermal energy?
Answer:
Sun / Solar energy is the ultimate source of energy for biomass, wind and ocean thermal energy.

Question 2.
Is geothermal energy and nuclear energy different in this respect? Why?
Answer:
Yes, geothermal energy is obtained from steam generated in regions of hot spots due to geological changes.
Nuclear energy is obtained by a process called nuclear fission and nuclear fusion of radioactive substances.

Question 3.
Where would you place hydroelectricity and wave energy?
Answer:
Hydroelectricity and wave electricity can be placed under renewable energy sources.

Activity 14.8 [T. B. Pg. 253]

To collect the information about various energy sources and how each one affects the environment.

Debate the merits and demerits of each source and select the best source of energy on this basis.

Sources of energy	Adverse effects on environment
1. Fossil fuel	Air pollution, acid rain, greenhouse effect
2. Thermal power	Air pollution, water pollution
3. Hydropower	Large eco-systems destroy agricultural land get submerged.
4. Biomass	Smoke, ash, and gaseous substances pollute air
5. Wind power	No adverse efffect
6. Solar energy	No adverse efffect
7. Tidal / Wave / Ocean thermal energy	No adverse efffect
8. Geothermal energy	No adverse efffect
9. Nuclear energy	Radioactive pollution, radiation

Based on this, solar energy is best energy soure. wind, ocean energy sources ultimately derive their energy from the sun. Solar energy is inexhaustible.

Activity 14.9 [T. B. Pg. 254]

Debate the following two issues in class: 9uestions:
(a) The estimated coal reserves are said to be enough to last us for another two hundred years. Do you think we need to worry about coal getting depleted in this case? Why or why not?
Answer:
Coal is used in large amount in thermal power stations for to generate electricity. The use of coal generates pollutants as the coal burns. Coal is also non-renewable resource, once depleted, it cannot be restored back. It will last only for 200 years, but formation of coal has taken millions of years and therefore we should use it with great care.

(b It is estimated that the sun will last for another five billion years. Do we have to worry about solar energy getting exhausted? Why or why not?
Answer:
We have not to worry about solar energy getting exhausted because the sun will last for another five billions years. We will have
to develop advanced technology for to trap and store more and more solar energy.

(c) On the basis of the debate, decide which energy sources can be considered –

• Exhaustible
• Inexhaustible
• Renewable
• on-renewable.

Give your reasons for each choice.
Answer:

• Exhaustible : Fossil fuel, coal will get depleted some day.
• Inexhaustible : Wind, tidal, solar energy, etc. are in the form continuing or repetitive currents of energy.
• Renewable : Biomass, if we manage properly, it can give a constant supply of energy at a particular rate.
• Non-renewable : Fossil fuels as once used up, they are lost forever and cannot be renewed.

Jharkhand Board JAC Class 10 Science Solutions Chapter 16 Management of Natural Resources Textbook Exercise Questions and Answers.

JAC Board Class 10 Science Solutions Chapter 16 Management of Natural Resources

Jharkhand Board Class 10 Science Management of Natural Resources Textbook Questions and Answers

Question 1.
What changes would you suggest in your home in order to be environment-friendly?
Answer:
Certain changes can be applied in our daily routine at home to be environment- friendly.

• Check the wastage of water. Not letting the water run while brushing, soaping or washing.
• Turning off lights and fans when not in use.
• Avoid wastage of water, food and energy.
• Reusing the materials whenever possible.
• Use solar water heater and cookers.
• Reduce the garbage.
• Make small rainwater harvesting system if possible.

Question 2.
Can you suggest some changes in your s school which would make it environment-friendly?
Answer:
A school can become environment-friendly by following ways:

• Growing plants and trees all around the play ground.
• Making a rainwater harvesting system.
• Arranging solar cell pannel if possible.
• Making compost of biomass waste collected e.g., food waste, fallen leaves, etc.

Question 3.
We saw in this chapter that there are four main stakeholders when it comes to forests and wildlife. Which among these should have the authority to decide the management of forest produce? Why do you think so?
Answer:
Out of the four main stakeholders, the local people living near the forest areas should given the authority to decide the management of forest produce.

Because these people know the traditional methods to use the natural resources in sustainable manner. These local people have been using the forest and wildlife resources since the ancient times without causing any damage to environment.

Question 4.
How can you as an individual contribute or make a difference to the management of (a) Forests and wildlife, (b) Water resources and (c) Coal and petroleum?
Answer:
Contribution of an individual to the management of:
(a) Forests and wildlife : Do not waste paper, use less paper, recycle the waste paper, minimise the wooden furniture, etc. help into save trees. Any animal products like fur, skin, tusk, horn, etc. should not be used by killing them.

(b) Water resources : Stop the wastage of water in daily routine. Instead of shower, use buckets to take bath, to wash the car. Close the taps properly. Do not let the water run while brushing, soaping or washing.

(c) Coal and petroleum : To walk or to use a bicycle over a short distance, use of public transport switching of unnecessary electrical appliances.

Question 5.
What can you as an individual do to reduce your consumption of the various natural resources?
Answer:
At an individual level, I will do following activities that can help to reduce the consumption of the various natural resources :

• Switch off electric appliances when not in use.
• Turn off tap when not in use.
• Minimum use of auto-vehicles.
• No wastage of food.
• No wastage of paper.
• Say no to plastic.

Question 6.
List five things you have done over the last one week to –
(a) conserve our natural resources.
(b) increase the pressure on our natural resources.
Answer:
(a) To conserve our natural resources:

• Turned off tap while brushing, soaping, etc.
• Walking to the nearby places.
• Switched off lights when not in use.
• Reusing envelopes by turning them.
• Using cracked crockery for growing the plant.

(b) To increase the pressure on our natural resources :

• Frequent use of plastic bags and throwing these anywhere.
• Often wastage of food
• Buying leather belt, purse and shoes.
• Leaving the light / lamp on even when not needed.
• Tearing the pages of notebook.

Question 7.
On the basis of the issues raised in this chapter, what changes would you incorporate in your lifestyle in a move towards a sustainable use of our resources?
Answer:
The Ganga Action Plan was launched in 1985 to improve the quality of water in Ganga and remove the pollution caused by disease causing microorganisms. Faecal coliform bacteria were found in Ganga water indicating contamination.

Jharkhand Board Class 10 Science Management of Natural Resources InText Questions and Answers

Question 1.
What changes can you make in your habits to become more environment-friendly?
Answer:
We can make following changes in our habits to become more environment-friendly:

• Use of paper bags, jute bags instead of plastic bags.
• Walk or cycle to cover short distance.
• Do not throw garbage anywhere.
• Use of renewable resources and biodegradable substances.
• Switching off electrical appliances when not required.
• Do not pollute and waste water.

Question 2.
What would be the advantages of exploiting resources with short-term aims?
Answer:
The advantages of exploiting resources with short-term aims are as follows :

• We will able to meet current basic human needs.
• It will be beneficial for the present generation.
• There will be rapid industrial growth, agricultural growth and hence, economic development.

Question 3.
How would these advantages differ from the advantages of using a long-term perspective in managing our resources?
Answer:
Using a long-term perspective is to reap the profit in a sustainable manner so that the natural resources will last for generations to come and will not merely be exploited to hit short-term gains. All the sections of society should be made aware about conservation and protection of the environment.

Question 4.
Why do you think that there should be equitable distribution of resources? What forces would be working against an equitable distribution of our resources?
Answer:
There should be equitable distribution s of resources so that both rich as well as poor, will get benefitted. Powerful and rich people take advantage of their influence and get more benefit as compared to weak and poor people. Money and power are important factors which work against the proper distribution of resources.

Question 5.
Why should we conserve forests and wildlife?
Answer:
We should conserve forests because they,

• Provide raw materials for various industries.
• Provide fruits, vegetables, fodder, grass, etc.
• Provide medicines, herbs, gum, resin, catechu, etc.
• Provide habitat to animals.
• Prevent soil erosion and flood.
• Provide sources for economic and social growth.
• Play an important role in maintaining CO₂ – O₂ balance in the atmosphere. Regulate earth’s average temperature.

Importance of wildlife conservation :

• To maintain forest ecosystem and ecological balance in nature.
• It helps into maintain forests by facilitating growth of plants in different places by dispersing seeds.
• Flow of energy in trophic levels maintained and biodiversity increases.

Question 6.
Suggest some approaches towards conservation of forests.
Answer:
Approaches towards conservation of forests are :

• Indiscriminate felling of trees for the purpose of timber must be reduced.
• The forest ecosystem must be protected from fuel starved villages, fodder-starved cattles and commercial exploitation.
• Replantation of trees and also plantation of indiginous species to develop forests in all available land.
• Participation of local people and villagers must be taken in conservation of forests.
• Scientific research, monitoring and spreading awareness about conservation of forests through education.

Question 7.
Find out about the traditional systems of water harvesting / management in different regions of India.
Answer:

Region	Traditional water harvesting system
Rajasthan	Khadins, tanks and nadis
Maharashtra	Bandharas and tals
Madhya Pradesh and Uttar Pradesh	Bundhis
Bihar	Ahars and pynes
Himachal Pradesh	Kulhs
Kandi belt of Jammu	Ponds
Tamil Nadu	Eris (tanks)
Karnataka	Kattas
Kerala	Surangams

Question 8.
Compare the traditional water harvesting system with the probable systems in hilly mountainous area or plains or plateau regions.
Answer:
Traditional water harvesting system in hilly and mountainous area is different from plains and from plateau region.

Example: In hilly areas like Himachal Pradesh there is a local system of irrigation called Kulhs. The water flowing in the streams is diverted into man-made channels which takes this water to numerous villages down the hillside.

Whereas water in plains is collected in check dams or tanks, tals or bundhis.

Question 9.
Find out the source of water in your region / locality. Is water from this source available to all people living in that area?
Answer:
The source of water in our region is municipal corporation supply of water and from ground water source. There is scarcity of water during summer and most people have to wait in long queues at the nearest municipal water tap to collect water for their daily consumption.

Activity 16.1 [T. B. Pg. 266]

To find out the international norms to regulate the emission of carbon dioxide.

• Carbon dioxide (CO₂) is the primary greenhouse gas emitted through human activity.
• The international norms to regulate the emission CO₂ are based on Kyoto Protocol.
• This protocol was negotiated in December, 1997 at the city of Kyoto, Japan and came into force on February 16th, 2005.
• As of December 2006, a total of 169 countries have signed the agreement.
• Under this protocol, industrialised countries must reduce their collective emissions of CO₂ and other greenhouse gases to an average of 5 % against 1990 levels.

Questions:

Question 1.
Why is it necessary to regulate the emission of carbon dioxide?
Answer:
Carbon dioxide is a major greenhouse gas that causes global warming. Which results into climate change. So, it is necessary to regulate the emission of carbon dioxide.

Question 2.
Which is the simplest way to regulate the CO₂ level in environment?
Answer:
Plantation of trees and conservation of green cover on the earth is the simplest way to regulate the CO₂ level in environment.

Question 3.
What is global warming?
Answer:
Global warming is the increase in average temperature of the earth’s environment due to increase in amount of greenhouse gases.

Activity 16.2 [T. B. Pg. 267]

To find out the names of organisations involved in spreading awareness about the conservation of natural resources.

NGO (Non Government Organisations) such us SEACOLOGY and Mera Desh Foundation spread awareness about the environment and promote activities that lead to conservation of natural resources.

Some other organisations that work for the conservation of the environment.

• CSE – The Centre for Science and Environment
• TER – The Energy and Resources Institute
• WTI – Wildlife Trust of India.

Questions:

Question 1.
Which organisation(s) is/are active to spread awareness about conservation of our environment and natural resources in your village / town / city?
Answer:

• Natural resource management
• Core environmental NGOs
• Centre for Environment and Social Concerns.

Question 2.
How you can contribute towards the conservation of our environment and natural resources.
Answer:
We can contribute towards the conservation of our environment and natural resources by following:

• Limit the use personal vehicle, instead use public transport.
• Minimise the wooden furniture at our home.
• Do not waste paper and other natural resources.
• Do not pollute water reservoirs.
• Do not use insecticides / pesticides.
• Do not cut trees, do not clear forests.

Activity 16.3 [T. B. Pg. 268]

To check the pH of tap water and compare it with pH of the water in the local water body.

Apparatus: Test tubes or beaker

Materials : Universal pH indicator / litmus paper

Procedure:

• Take water sample from tap of your home in a test tube.
• Add few drops of the universal indicator into the test tube containing tap water.
• Observe the water sample in the test tube for colour change.
• Test pH using a litmus paper, in the water sample placed in a beaker.
• Dip the litmus paper into the water in the local water body.
• Observe colour changes of the litmus paper.

Observation:

• Test tube containing tap water, turns yellow colour with addition of pH indicator.
• Litmus paper does not show colour change with tap water.
• Water sample collected from pond shows red colour with pH indicator.
• Litmus paper turns red as it is dipped in water of local water body.

Conclusion:
The uncontaminated tap water is neutral while water of local water body is acidic.

Questions:

Question 1.
What is the cause for acidic water in water body?
Answer:
Pollution is the main cause for acidic water in water body.

Question 2.
Can aquatic life survive in acidic water of water body?
Answer:
No, aquatic life cannot survive in acidic water.

Question 3.
Can you say whether the water is polluted or not on the basis of your observations?
Answer:
Yes, tap water is clean and non-polluted. Water of water body is polluted.

Activity 16.4 [T. B. Pg. 269]

To visit a town or village after a few years of absence, note down the major developmental changes there.

Major developmental changes in a village are

• New roads have been built
• New houses have been constructed.
• Factory and new market have been setup.

Questions :

Question 1.
Make a list of the materials for making roads and buildings with their probable sources.
Answer:

Materials	Probable sources
Granite	Rocks
Cement	Factories. Raw materials obtained from nature.
Bricks	Soil
Steel	Mined from the soil
Wood	Forest

Question 2.
What are the ways in which the materials used in construction can be reduced?
Answer:

• Roads can be built with cement or with polymer plastic mix instead of coaltar.
• By using cement and iron to make beams and by using aluminium or fibreglass to make windows and doors, we can reduce the usage of wood.

Activity 16.5 [T. B. Pg. 270]

To prepare a report on traditional practices for conservation of nature in day-to-day life.

Traditional practices for conservation of nature :

• Tulsi, Calotropis, Asopalav, Ficus (Pipal), Khejdo, Banyan trees and various other trees are planted, which are considered sacred and worshipped by the people in . majority of Indian villages and towns.
• Several birds, cow and even snakes have been considered sacred. Therefore, they are protected.
• The concept of cultural landscape such as sacred forests, sacred corridors and a variety of ethno-forestry practices are the means for protection of nature and natural resource. They are observed by people.
• Several rivers have been considered sacred.

Activity 16.6 [T. B. Pg. 271]

To know about our dependency on forest resources.

Questions :

Question 1.
Make a list of forest products that we commonly use.
Answer:
Wood, bamboo, fuel, timber, fruits, herbs, paper, medicine and many other products that we commonly use come from forests.

Question 2.
What do you think a person living near a forest would use?
Answer:
A person living near a forest might use wood, fodder, dry leaves, bamboo, fruits, vegetables, herbs, etc.

Question 3.
What do you think a person living in a forest would use?
Answer:
A person living in a forest is almost entirely dependent on the forest for all basic needs, i.e., fire wood, small timber, fodder and grass, bamboo, nuts and medicines, food, clothing, shelter, fishing, hunting, etc.

Question 4.
How these needs depend upon the person’s area in which they live?
Answer:
People who live in a village or town are less dependent on forest than those who live in or near the forest.

Activity 16.7 [T. B. Pg. 272]

To name any two products of forest used in an industry and find out a sustainable alternative for such products.

• Tendu leaves are used in Bidi industry. Essential oils are used in the manufacture of soaps and cosmetic industry.
• Over exploitation of forests is not sustainable in the long run. We need to control our consumption. Plantation of trees is necessary.

Activity 16.8 [T. B. Pg. 275]

To debate following topics which are concerned with the damage caused to forests:

• Building rest houses for tourists in national parks.
• Grazing domestic animals in national parks.
• Tourists throwing plastic bottles / covers and other litter in national parks.

Conclusion:
(a) The rest houses made in national park for tourists become hub of activities, which damage ecology of forests. Tourists see forest as travel destination. They cause pollution and disturb natural environment of forests.

(b) Over grazing of domestic animals leaves little or no grass for the herbivores animals that live in forests. Thus their number slowly decreases, in turn disturbs the upper trophic levels of the food chains in the forests.

(c) Tourists throwing plastic wastes in national parks cause pollution. Plastic is non-biodegradable wastes.

Decomposers cannot decompose it and such dumped waste piles up. Biotic components of national parks are adversely affected.

Activity 16.9 [T. B. Pg. 275]

In Maharashtra, the village suffering from chronic water shortage surround a water theme park to get water for their needs.

Debate whether they get optimum use of available water or they should search for alternative rain water harvesting.

Conclusion:

• No, the construction of a water theme park in the region of water scarce area in wrong decision.
• Water is a basic need for all living organisms. If people are suffering due to lack of drinking water and water for other uses too, how will they amuse themselves with a water park.
• This is unequal distribution of water resources. People should oppose this and the authorities should first provide adequate supply of water to people. People should also search for alternative rain water harvesting.

Activity 16.10 [T. B. Pg. 275]

Study the pattern of rainfall in various parts of India from atlas.

Do by yourself.

Conclusion :
Rainfall more than 200 cm in North-East part of India and the regions of water scarcity where rainfall is less than 25 cm are part of Rajasthan and Gujarat.

Activity 16.11 [T. B. Pg. 279]

To find ways to reduce our consumption of coal and petroleum.

Some ways to reduce our consumption of coal and petroleum are as follows :

• Take a public transport instead of using your personal vehicle.
• Use CFL instead of bulbs.
• Install solar water heater.
• Walk or cycle to nearby places instead of using motorised vehicles.
• Switch off vehicles at red lights.
• Use pressure or solar cookers to cook food, keep proper air pressure in tires, etc.
• Wearing an extra sweater instead of using heater or sigri in cold days.
• Taking the stairs instead of using the lift up to 3-4 floors.
• Using efficient engines that ensures complete combustion of fuels.

Activity 16.12 [T. B. Pg. 279]

To find out the norms for emission from vehicles.

• Euro norms refer to the permissible emission level from petrol and diesel vehicles, which have been implemented in Europe.
• These norms require manufacturers to reduce pollution emission levels in an efficient manner by making technical changes in the vehicles they manufacture.
• Under the Euro norms, emissions of carbon monoxide (CO), burnt hydrocarbons (HCs), oxides of nitrogen (NOx) and suspended particulate matter (SPM) are regulated.
• The European Union has been upgrading emission norms as well as the fuel quality standards in stages :
  Norms Implemented
  Euro I → 1992 – 93
  Euro II → 1996 – 97
  Euro III → 2000
  Euro IV → 2005
• The enforcement of these norms help in reducing air pollution due to vehicular s emissions.

Jharkhand Board JAC Class 10 Science Solutions Chapter 9 Heredity and Evolution Textbook Exercise Questions and Answers.

JAC Board Class 10 Science Solutions Chapter 9 Heredity and Evolution

Jharkhand Board Class 10 Science Heredity and Evolution Textbook Questions and Answers

Question 1.
A Mendelian experiment consisted of breeding tall pea plants bearing violet flowers with short pea plants bearing white flowers. The progeny all bore violet flowers, but almost half of them were short. This suggests that the genetic make-up of the tall parent can be depicted as …………..
A. TTWW
B. TTww
C. TtWW
D. TtWw
Answer:
A. TTWW

Question 2.
An example of homologous organs is …………..
A. our arm and a dog’s foreleg.
B. our teeth and an elephant’s tusks.
C. potato and runners of grass.
D. all of the above
Answer:
D. all of the above

Question 3.
In evolutionary terms, we have more in common with …………..
A. a Chinese school-boy.
B. a chimpanzee.
C. a spider.
D. a bacterium.
Answer:
A. a Chinese school-boy.

Question 4.
A study found that children with light coloured eyes are likely to have parents with light coloured eyes. On this basis, can we say anything about whether the light eye colour trait is dominant or recessive? Why or why not?
Answer:
No, we cannot say anything about whether the light eye colour trait is dominant or recessive on the basis of given information because information regarding cross between two traits, i.e., light colour with black eye colour is essential to determine it. In general population light coloured eyes are in much less proportion as compared to dark eyes. This indicates that it may be recessive trait.

Question 5.
How are the areas of study – evolution and classification – interlinked?
Answer:
One can work out the evolutionary relationships of the species by identifying hierarchies of characteristics between them.
Similarities among organisms will allow us to group them together. If the characteristics between two species are more common, then they are more closely related and they had a recent common ancestor.

Thus, build up small group of species with recent common ancestors, then super group with more distant common ancestors and so on. By this one can interlink the study of evolution and classification.

Question 6.
Explain the terms analogous and homologous organs with examples.
Answer:
Analogous organs : Organs with different structure and components but have common function.
Examples: The wing of a bat and the wing of a bird.

Homologous organs : Organs with similar basic structure but modified to perform different functions.
Examples : Fore limbs of frog, lizard, bird and human.

Question 7.
Outline a project which aims to find the dominant coat colour in dogs.
Answer:

So, black coat is dominant trait in dog. [If in F₁ generation all progeny are with white coat then white coat can be considered as dominat trait.]

Question 8.
Explain the importance of fossils in deciding evolutionary relationships.
Answer:
Fossils are the remains or impressions of the dead animals and plants that lived in the past. Fossils are the direct evidence of evolution. If the fossils are found closer to the surface then they are more recent than the fossils found in deeper layers. One can find evolutionary relationships between ancient and present organisms by study of fossils. We also come to know the time period of evolutionary process by studying fossils.

Question 9.
What evidence do we have for the origin of life from inanimate matter?
Answer:
Stanley L. Miller and Harold C. Urey:
In 1953, they assembled an experiment in which primitive atmosphere was simulated. It had compounds like ammonia, methane, hydrogen and water vapour but no oxygen. This was maintained at a temperature just below 100°C and electric sparks were passed through the mixture of gases to simulate lightning. At the end of a week, 15% of the carbon had been converted to simple compounds of carbon including amino acids which are monomers of protein molecules.

Question 10.
Explain how sexual reproduction gives rise to more visible variations than asexual reproduction. How does this affect the evolution of those organisms that reproduce sexually?
Answer:
(1) Sexual reproduction gives rise to more visible variations than asexual reproduction because in sexual reproduction, each new generation is the combination of the DNA copies from two pre-existing individuals.

(2) Even during gamete formation new combination of genes occurs during meiosis. From more visible variations during sexual reproduction, certain favourable variation can be selected by natural selection.

Whereas in asexual reproduction generally offsprings are exact copies of their single parent.

Question 11.
How is equal genetic contribution of male and female parents ensured in the progeny?
Answer:
Male and female parents produce male gametes (Sperms) and egg cells (Ovum) respectively through a process of meiosis. It reduces the number of chromosomes and amount of DNA to half in gametes.

Such germ cells from two individuals, i. e., male and female parents are fused to form zygote. This ensures the equal genetic contribution of male and female parents in the progeny that develop from zygote.

Question 12.
Only variations that confer an advantage to an individual organism will survive in a population. Do you agree with this statement? Why or why not?
Answer:
Yes, because of useful inherited / genetic variations of an individual organism can adapt and survive. Moreover they leave behind more offsprings and populations with such genetic variations will survive.

Jharkhand Board Class 10 Science Heredity and Evolution InText Questions and Answers

Question 1.
If a trait A exists in 10 % of a population of an asexually reproducing species and a trait B exists in 60 % of the same population, which trait is likely to have arisen earlier?
Answer:
A trait B which exist in 60 % of population of an asexually reproducing species, must have arisen earlier than trait A.

Because in asexually reproducing species there are chances of appearance of very few new traits due to small inaccurancies during DNA copying. So, populations having trait A have arisen later.

Question 2.
How does the creation of variations in a species promote survival?
Answer:
The creation of variations in a species is either due to inaccuracies in DNA copying or during sexual reproduction.

• Depending on the nature of variations, different individuals have different kinds of advantages.
• The individuals with useful variations can adapt to the prevailing environment and show better survival.
• The individuals with useful variations then increase in numbers.

Question 3.
How do Mendel’s experiments show that traits may be dominant or recessive?
Answer:
Mendel’s conducted, cross fertilisation between pure tall plant (TT) and pure short plant (tt) which resulted in all tall (Tt) plant in F₁ generation.

This shows that single copy of T is enough to make the plant tall. It shows that one trait which is expressed in the presence of its contrasting form. This is dominant trait and the other remains unexpressed in the presence of its contrasting form is recessive trait.

Question 4.
How do Mendel’s experiments show that traits are inherited independently?
Answer:
Mendel performed dihybrid experiment on pea plants. A tall plant with round seeds was crossed with a short plant with wrinkled seeds. Fx progeny plants were all tall with round seeds. F₁ progeny are used to generate F₂ progeny by self-pollination. Along with parental combinations, F₂ progeny showed new combinations too. Some of them were tall with wrinkled seeds while some others were short with round seeds.

It means factors (genes) controlling for seed shape and height of plant recombine to form new combinations in F₂ offsprings. Thus, tall/short trait and the round seed / wrinkled seeds trait are inherited independently.

Question 5.
A man with blood group A marries a woman with blood group O and their daughter has blood group O. Is this information enough to tell you which of the traits blood group A or O is dominant? Why or why not?
Answer:
No, the given information is not enough to tell us whether the trait of blood group A or blood group O is dominant.

• Blood group trait is controlled by genes and inherited from parents.
• Daughter has blood group O and two copies of genes as it inherited one each from the father and the mother.

For Information:
Trait blood group A is dominant and blood group O is recessive. This fact derived from the study of genotype.
Gene I^A for blood group trait A
Gene i recessive for blood group trait O.

Question 6.
How is the sex of the child determined in human beings?
Answer:
The sex of the child will be determined in human beings by the sex chromosome inherited from father. Father has XY sex chromosomes in each of his cell. Two types of gametes (sperms) are produced based on chromosomes, 50 % sperms are with X chromosome and 50 % sperms are with Y chromosome.

When sperm bearing X chromosome fertilise the egg, girl is born and when sperm bearing Y chromosome fertilise the egg, son is born.

Question 7.
What are the different ways in which individuals with a particular trait may increase in a population?
Answer:
A particular trait may increase in a population by following ways:

• Natural selection – directing evolution with a survival advantage.
• Genetic drift – provides diversity without any adaptations.

Question 8.
Why are traits acquired during the life-time of an individual not inherited?
Answer:
Traits acquired during the life-time of an individual may not be inherited because they may be changes in non-reproductive tissues which cannot be passed on to the DNA of the germ cells and hence cannot be passed on its progeny.

Question 9.
Why are the small numbers of surviving tigers a cause of worry from the point of view of genetics?
Answer:
The small numbers of surviving tigers is a cause of worry from the point of view of genetics because if they become extinct then the genes of this species will be lost forever. There will be no chance of getting this species back again to life in future.

Question 10.
What factors could lead to the rise of a new species?
Answer:
Factors that can lead to the rise of a new species are –

• Gene flow
• Genetic drift
• Natural selection and
• Reproductive isolation.

Question 11.
Will geographical isolation be a major factor in the speciation of a self-pollinating plant species? Why or why not?
Answer:
No, geographical isolation will not be a major factor in the speciation of a self-pollinating plant species because single parent is involved in it. There is no gene flow between two geographically isolated population.

Question 12.
Will geographical isolation be a major factor in the speciation of an organism that reproduce asexually? Why or why not?
Answer:
No, geographical isolation will not be a major factor to the speciation that reproduces asexually because single parent is involved in asexual reproduction due to which variations are not formed.

Question 13.
Give an example of characteristics S being used to determine how close two species? are in evolutionary terms.
Answer:
Homologous organs are the characteristics < being used to determine two species are close in evolutionary terms.

Example: The basic structure of the limbs of mammals, birds, reptiles and amphibians is similar though it has been modified to perform different functions.

Question 14.
Can the wing of a butterfly and the wing of a bat be considered homologous organs? Why or why not?
Answer:
No. they are not considered homologous organs because the wing of a butterfly and the wing of a bat are similar in function but the designs of two wings, their structure and components are very different. So, they can be considered as analogous organs and not homologous.

Question 15.
What are fossils? What do they tell us about the process of evolution?
Answer:
Fossils are the remains or impressions of the dead animals and plants that lived in the past.

Fossils are the direct evidence of evolution. If the fossils are found closer to the surface then they are more recent than the fossils found in deeper layers. One can find evolutionary relationships between ancient and present organisms by study of fossils.

We also come to know the time period of evolutionary process by studying fossils.

Question 16.
Why are human beings who look so different from each other in terms of size, colour and looks said to belong to the same species?
Answer:
All human beings look so different from each other in size, colour and looks due to environmental factors, new combination of genes during reproduction.

All they belong to Homo sapiens and have descended from a common ancestor in Africa. They have capacity of interbreeding which is an important criteria to categorize them as one species.

Question 17.
In evolutionary terms, can we say which among bacteria, spiders, fish and chimpanzees have a better body design? Why or why not?
Answer:
In evolutionary terms, we can say that chimpanzees have a better developed body design among remaining three because chimpanzees are highly complex as compared to remaining three.

Activity 9.1 [T. B. Pg. 143]

To suggest rule for inheritance of earlobe type.

Procedure / Method:

• The lowest part of the ear pinna called the earlobe, is closely attached to the side of the head, i.e., attached or not, i.e., free.
• Observe the earlobes of all the students In – the class. Prepare a list of students. Enter < the data about the earlobes whether they are free or attached.
• Find out about the earlobes of the parents of each student in the class.
• Correlate earlobe type of each student with that of their parents.
• In the column write F for free earlobe and A for attached earlobe.
  

No.	Details	Free Earlobe	Attached Earlobe
1.	Name of student….
	Mother
	Father
2.	Name of student….
	Mother
	Father

Answer the following questions on the basis of the collected data:

Questions :

Question 1.
Which expression of earlobe is observed more in number in your class?
Answer:
Expression of free earlobe is observed more in number in our class.

Question 2.
Are the types of earlobes hereditary?
Answer:
Yes, the type of earlobe is hereditary.

Question 3.
From your collected data state which expression is dominant and which one is recessive for earlobe.
Answer:
Free earlobe is dominant and attached earlobe is recessive expression.

Question 4.
Determine the percentage of free earlobe and attached earlobe in the students of your classroom.
Answer:
There are 60 students in the classroom. Out of which 51 students have free earlobe and 9 students have attached earlobe.
Percentage of free earlobe = \(\frac { 51 }{ 60 }\) x 100 = 85 %
Percentage of attached earlobe = \(\frac { 9 }{ 60 }\) x 100
= 15 %

Activity 9.2 [T. B. Pg. 144]

What experiment would we do to confirm that F₂ generation did infact have a 1:2:1 ratio of TT, Tt and tt trait combination?
We do Mendel’s monohybrid cross-experiment:

Jharkhand Board JAC Class 10 Science Solutions Chapter 15 Our Environment Textbook Exercise Questions and Answers.

JAC Board Class 10 Science Solutions Chapter 15 Our Environment

Jharkhand Board Class 10 Science Our Environment Textbook Questions and Answers

Question 1.
Which of the following groups contain only biodegradable items?
A. Grass, flowers and leather
B. Grass, wood and plastic
C. Fruit peels, cake and lime juice
D. Cake, wood and grass
Answer:
Grass, flowers and leather; Fruit peels, cake and lime juice; Cake, wood and grass.

Question 2.
Which of the following constitute a food chain?
A. Grass, wheat and mango
B. Grass, goat and human
C. Goat, cow and elephant
D. Grass, fish and goat
Answer:
Grass, goat and human

Question 3.
Which of the following are environment-friendly practices?
A. Carrying cloth-bags to put purchases in while shopping
B. Switching off unnecessary lights and fans
C. Walking to school instead of getting your mother to drop you on her scooter.
D. All of the above
Answer:
All of the above

Question 4.
What will happen if we kill all the organisms in one trophic level?
Answer:
If we kill all the organisms in one trophic level then organisms of next trophic level will not get food (chemical energy) and the entire food chain gets disturbed. All the organisms which are dependent on these are died.

On the other hand, the organisms at the lower trophic level will increase in abundance. Due to this, ecosystem will be in imbalance.

Question 5.
Will the impact of removing all the organisms in a trophic level be different for different trophic levels? Can the organisms of any trophic level be removed without causing any damage to the ecosystem?
Answer:
The impact of removing all the organisms in a trophic level will be different for different trophic levels. Removal of producers will affect all the organisms of successive trophic levels. It will be a threat the survival. The removal of organisms at higher trophic level will lead to increase in organisms of lower trophic level. Removal of organisms of any trophic level will cause the damage to the ecosystem.

Question 6.
What is biological magnification? Will the levels of this magnification be different at different level of the ecosystem?
Answer:
Successively increasing concentration of some substance (e.g., pesticides) at various trophic levels of a food chain of organisms is known as biological magnification.

The level of biological magnification will be different at different trophic levels of the ecosystem. Maximum concentration will be at the third and fourth trophic level and concentration of chemical will be less at lower trophic levels.

Question 7.
What are the problems caused by the non-biodegradable wastes that we generate?
Answer:
The problems caused by the generated non-biodegradable wastes are as follows :

• It causes biological magnification.
• They keep on accumulating in nature causing pollution.
• They prevent growth of vegetation when dumped underground.
• They may be inert and simply persist in the environment for a long time and may harm various members of the ecosystem.
• There is imbalance of the food chains causing problems in ecosystem.

Question 8.
If all the wastes we generate is biodegradable, will this have no impact on the environment?
Answer:
If all the waste we generate is biodegradable and if properly allowed it to decompose, then it will have no impact on the environment. They should be properly managed.

Question 9.
Why is damage to the ozone layer a cause for concern? What steps are being taken to limit this damage?
Answer:
Ozone layer absorbs ultraviolet radiation of the sun which is very harmful to living organisms.

Damage to the ozone layer is a cause for concern because depletion of ozone layer allows harmful ultraviolet radiation to reach to the surface of earth, which may lead to skin cancer, cataract, etc.

To reduce the damage to the ozone layer, use of chlorofluorocarbons has been minimised. In 1987, the UNEP-United Nations Environment Programme has passed an agreement to freeze CFC production at 1986 levels. This will protect the ozone layer and subsequent effects of radiation.

Jharkhand Board Class 10 Science Our Environment InText Questions and Answers

Question 1.
What are trophic levels? Give an example of a food chain and state the different trophic levels in it.
Answer:
Successive levels of nourishment in the food chain are known as trophic levels.

• It shows transfer of energy in an ecosystem.
• Food chain is a sequential list of prey-predator
  

Question 2.
What is the role of decomposers in the ecosystem?
Answer:
Decomposers feed on the excretory substances as well as dead bodies of plants and animals.
Bacteria and fungi are decomposers.

• They breakdown the complex organic substances into simple inorganic substances.
• Such simple inorganic substances are used up by the plants again.
• So, they play an important role in cyclic pathway of the elements.

Question 3.
Why are some substances bio¬degradable and some non-biodegradable?
Answer:
Some substances such as paper, vegetables, peels, etc. are acted upon by decomposers and get converted into simple form are called biodegradable. Biodegradable substances are natural substances.

Some synthetic substances such as plastic, polythene, etc. cannot be degraded by microbial activity are called non-biodegradable.

Question 4.
Give any two ways in which biodegradable substances would affect the environment.
Answer:

• Biodegradable substances get degraded by microbial activity releasing simple component back to nature. These components help to sustain the life of other organisms.
• Diming the biodegradation certain gases may be released in atmosphere causing pollution.

Question 5.
Give any two ways in which non-biodegradable substances would affect the environment.
Answer:

• Non-biodegradable substances such as pesticides cause soil and water polluton. It may cause biological magnification.
• Non-biodegradable substances block the functions of an ecosystem, i.e., transfer of energy and elements may stop.

Question 6.
What is ozone and how does it affect any ecosystem?
Answer:
Ozone is a molecule formed by three atoms of oxygen in the presence of UV (Ultraviolet) rays. Ozone performs an essential function at the higher levels of the atmosphere. However, at ground level it is a deadly poison. Ozone absorbs shorter wavelength UV rays from the sun. Thus it protects the living system on earth.

Question 7.
How can you help in reducing the problem of waste disposal? Give any two methods.
Answer:
We can help in reducing the problem of waste disposal by following methods :

• Biodegradable domestic wastes such as left-over food, fruit and vegetable peels, dry leaves and other wastes of gardens, etc. can be hurried in a pit. They are converted into compost and used as manure.
• Waste materials such as tin, cans, paper, glass, metallic articles are recycled. Through the process of recycling such materials are reused to form new products.

Activity 15.1 [T. B. Pg. 256-257]

To make an aquarium.

Materials:
Large jar of glass, water, pebbles, fish food, aerator (oxygen pump), small fishes, aquatic plant

Procedure:

• Take a large jar of glass. Keep some pebbles in it.
• Fill the jar with water.
• Add few aquatic plants such as algae in the jar.
• Add few small fishes in it.
• Arrange oxygen pump in such a way that we can provide oxygen in the jar.
• Provide fish food regularly which is available in the market.
• Add few small aquatic animals other than fishes in the jar.

Questions :

Question 1.
How can an aquarium become self-sustaining system by adding a few aquatic plants and animals?
Answer:
Aquatic plants performs photosynthesis. They are producers on which animals depend for their nutrition. Plants release oxygen during photosynthesis. Oxygen is utilised by animals in respiration and release carbon dioxide in the water. Carbon dioxide is available to plants for photosynthesis. Thus, aquarium becomes self-sustaining system.

Question 2.
Can we leave the aquarium as such after it is set up?
Answer:
No, we cannot leave the aquarium as it is because metabolic wastes are released in water make it polluted. Therefore, finally change of water is needed.

Question 3.
Why does aquarium have to be cleaned once in a while?
Answer:
Metabolic wastes produced by aquatic organisms make the water polluted. So, it should be cleaned once in a while.

Question 4.
Do we need to clean lakes or ponds in the same manner? Why or why not?
Answer:
Yes, because sometimes excretory wastes accelerate the growth of algae. The lake or pond gets covered with algal growth. Some toxic substances are released and dissolved 02 in water body will be depleted. This will lead to death of all aquatic life and such ecosystem may destructed.

Activity 15.2 [T. B. Pg. 257]

To find more about aquarium.
Material:
An aquarium

Questions:

Question 1.
While creating an aquarium did you take care not to put an aquatic animal which would eat others? What would have happened otherwise?
Answer:
Yes, while creating an aquarium care was taken predator aquatic animals were not used. Otherwise such predators can feed on other organisms and destroy. All small aquatic animals will be consumed by carnivores which later would all die.

Question 2.
Write the aquatic organisms in order of who eats whom and form a chain of at least three steps.
Answer:

Question 3.
Would you consider any one group of organisms to be of primary importance? Why or why not?
Answer:
Yes, plants (producers) should be given primary importance because they form first trophic level and all the consumers directly or indirectly depend on plants for their food (energy) requirement.

Question 4.
Explain the components of an ecosystem.
OR
Explain the groups of organisms based on their role in an ecosystem.
Answer:
Each ecosystem consists of two main components:
(1) Abiotic components : All the non-living constituents of an ecosystem are included in the abiotic components.

Abiotic components are the physical factors like temperature, rainfall, wind, soil, light, minerals, etc.

(2) Biotic components : All living organisms of an ecosystem are included in the biotic components.
Organisms can be grouped as producers, consumers and decomposers according to their food habit. Their mode of sustenance forms the trophic relationship in the environment.

(i) Producers : Those organisms which can make organic compounds like sugar and starch from inorganic substances using solar energy in presence of chlorophyll are called producers.
Example: Certain bacteria, various kinds of algae and all green plants.

(ii) Consumers : Those organisms which consume the food produced either directly from
producers or indirectly by feeding on other consumers are called consumers.

Example: Non-chlorophyllous and heterotrophic organisms.
Consumers can be divided into four categories.

(iii) Decomposers : The microorganisms which breakdown the complex organic substances into simple inorganic substances are called decomposers.
Example: Certain bacteria and fungi, breakdown the dead remains and waste products of organisms.

Question 5.
Write a short note on : Consumers
Answer:
Consumers : Those organisms which consume the food produced either directly from producers or indirectly by feeding on other consumers are called consumers.

Example: Non-chlorophyllous and heterotrophic organisms.
Consumers can be divided into four categories

Activity 15.3 [T. B. Pg. 260]

Newspaper reports about pesticides level in ready-made food items are often seen these days and some states have banned these products.

Questions :

Question 1.
What would be the source of pesticides in ready-made food items?
Answer:
Pesticides are non-biodegradable substances. They are excessively used for controlling the pest in crop field. They enter in food items through food chain.

Question 2.
Could pesticides get into our bodies from food products other than ready-made food?
Answer:
Yes, pesticides can get into our bodies from the food grains, vegetables, fruits, milk, etc. that contain residues of pesticides.

Question 3.
What methods could be applied to reduce our intake of pesticides?
Answer:

1. Pesticides in the crop fields should be s judiciously used.
2. Monitoring of pesticides level in agricultural products should be tested at regular interval.

Question 4.
Why some states have banned on some ready-made food products?
Answer:
Some states have banned on some ready-made food products because the level of pesticides is high in it, thus causing hazard to our health.

Activity 15.4 [T. B. Pg. 261]

To find harmful chemicals for ozone layer.
Materials:
Library, internet, newspaper reports

Questions:

Question 1.
Which chemicals are responsible for the depletion of the ozone layer?
Answer:
Ozone depleting substances such as chlorofluorocarbons (CFCs), hydrofluoro-carbons (HFCs) and oxides of nitrogen are resonsible for depletion of the ozone layer.

Question 2.
Find out if the regulations to control the emission of ozone depleting chemicals have succeeded in reducing the damage to the ozone layer. Has the size of the hole in the ozone layer changed in recent years?
Answer:
In 1987, the United Nations Environment Programme (UNEP), succeeded in forging an agreement to freeze CFC production at half of the 1986 levels.

Yes, by reducing the continuous use of ozone depleting chemicals, the size of the hole in the ozone layer has reduced in recent years.

Activity 15.5 [T. B. Pg. 261]

Collect waste materials from your home. (kitchen wastes, waste paper, torn clothes and its pieces, empty cartons, milk packets, empty bottles, its lid, used tea leaves, empty medicine bottles/strips/bubble packs, broken footwear, etc.)

• Bury these materials in a pit near your home.
• Keep these moist by spraying water on it.
• Fill the pit with moist clay and cover the waste.
• Dig the pit and observe at 15-day intervals.

Questions:

Question 1.
What are the materials that remain unchanged over long periods of time?
Answer:
The materials that remain unchanged over long periods of time are empty medicine bottles, bubble packs, milk packets, broken plastic footwear.

Question 2.
What are the materials which change their form and structure over time?
Answer:
The materials that change their form and structure are food, vegetable peels, used tea leaves, empty cartons, waste paper, torn clothes, broken leather footwear.

Question 3.
What are the materials that change the faster?
Answer:
The materials that are changed faster are vegetable peels, used tea leaves, spoilt food, etc.

Activity 15.6 [T. B. Pg. 262]

To find more about biodegradable and non-biodegradabie substances.

Questions:

Question 1.
How long are various non-biodegradable substances expected last in our environment?
Answer:
Non-biodegradable substances such as plastic wastes can be acted upon by physical factors such as heat and pressure. But under the ambient conditions found in our environment, non-biodegradable wastes persist for a long time.

Question 2.
Find out whether biodegradable plastic do or do not harm the environment.
Answer:
Polymer fabrics and dental implants are examples of biodegradable plastics. Biodegradable plastics do not cause any harm to the environment.

Activity 15.7 [T. B. Pg. 263]

To find about the disposal of waste generated at home,

Questions

Question 1.
Find out what happens to the waste generated at home? Is there a system in place to collect this waste?
Answer:
The waste generated at home is collected in dustbins daily. The municipal corporation of our city has set up a system to remove such garbage on daily basis. Large collection containers are set up at specific sites to collect the household waste.

In some areas, municipal corporation has set up a system to collect household waste from door to door.

Question 2.
Find out how the local body (panchayat, municipal corporation, resident welfare association) deals with the waste.
Are there mechanisms in place to treat the biodegradable and non-biodegradable wastes separately?
Answer:
Biodegradable and non-biodegradable wastes are separated at the source. In some villages, biogas plants have been established to use biodegradable waste to generate biogas and manure.

In cities, municipal corporation collects waste and finally send to specific vacant site on the extreme edge of the city and dump it there.

Biodegradable wastes should not be burnt as burning causes air pollution. They are converted into manure by composting. Non-biodegradable wastes such as plastics, glass, metals are collected separately and send to respective recycling units.

Question 3.
Calculate how much waste is generated at home in a day. How much of this waste is biodegradable?
Answer:
Large amount of waste is generated at home in a day. Most of it is biodegradable. Some of it is non-biodegradable.

Question 4.
Calculate how much waste is generated in a classroom in a day. How much of this waste is biodegradable?
Answer:
In a classroom, large amount of waste which is mostly biodegradable waste generated.

Question 5.
Suggest the ways of dealing with the waste.
Answer:
Biodegradable wastes should be burried in a pit. After few days they change into manure / compost due to action of decomposers. It can be used in garden.

Activity 15.8 [T. B. Pg. 263]

To find about how the sewage is treated.

Questions:

Question 1.
Find out how the sewage in your locality is treated. Are there mechanisms in place to ensure that local water bodies are not polluted by untreated sewage?
Answer:
We have underground drainage system in our city. The interconnected drainage lines take away sewage to distantly place where sewage treatment plant is located. Here it is treated.

Sewage is not allowed to pollute the local water bodies. It is first treated in sewage treatment plant, during this the treated water is disinfected through chlorination, water is then used for irrigation.

Question 2.
Find out how the local industries in your locality treat their wastes.
Are there mechanisms in place to ensure that the soil and water are not polluted by this water?
Answer:
Local industries are legaly bound to treat their industrial waste before releasing/discarding them in local water bodies.

But, these industries do not follow the rules and the norms set for them. Due to it, the industrial wastes that come out from industries have still pollutants and pollute our water bodies.

Activity 15.9 [T. B. Pg. 264]

To find out hazardous materials in disposed electronic items.
Materials:
Internet, books from library.

Questions:

Question 1.
What hazardous materials have to be dealt with while disposing the electronic items? How would these materials affect the environment?
Answer:
When we dispose off electronic wastes, the hazardous materials in them include plastics and electronic chips made of silicon. Such materials are non-biodegradable and will remain unchanged for a long time in the environment.

Question 2.
How are plastics recycled? Does the recycling process have any impact on the environment?
Answer:
Plastic wastes are isolated from garbage and sent to recycling unit. Plastics are melted in the unit and then remoulded to form various plastic items for reuse.

The recycling process reduces plastic wastes from the environment. Recently, plastic wastes are being used to construct polymer plastic road.

Jharkhand Board JAC Class 10 Science Solutions Chapter 3 Metals and Non-metals Textbook Exercise Questions and Answers.

JAC Board Class 10 Science Solutions Chapter 3 Metals and Non-metals

Jharkhand Board Class 10 Science Metals and Non-metals Textbook Questions and Answers

Question 1.
Which of the following pairs will give displacement reactions?
(a) NaCl solution and copper metal
(b) MgCl₂ solution and aluminium metal
(c) FeSO₄ solution and silver metal
(d) AgNO₃ solution and copper metal
Answer:
AgNO₃ solution and copper metal

Question 2.
Which of the following methods is suitable for preventing an iron frying pan from rusting?
(a) Applying grease
(b) Applying paint
(c) Applying a coating of zinc
(d) All of the above
Answer:
Applying a coating of zinc

Question 3.
An element reacts with oxygen to give a compound with a high melting point. This compound is also soluble in water. The element is likely to be…
(a) calcium
(b) carbon
(c) silicon
(d) iron
Answer:
calcium

Question 4.
Food cans are coated with tin and not with zinc because …
(a) zinc is costlier than tin.
(b) zinc has a higher melting point than tin.
(c) zinc is more reactive than tin.
(d) zinc is less reactive than tin.
Answer:
zinc is more reactive than tin.

Question 5.
You are given a hammer, a battery, a bulb, wires and a switch.
(a) How could you use them to distinguish between samples of metals and non-metals?
(b) Assess the usefulness of these tests in distinguishing between metals and non-metals.
Answer:
(a) Metals can be hammered Into thin sheets hence, metal possesses property of malleability while non-metals cannot be beaten into thin sheets.
Arrange the battery, bulb, wires and switch in a proper circuit and by passing the electric current, if the bulb glows, then it must be a metal, because metal is a good conductor of electricity. But if the bulb does not glow, then it Is a sample of non-metal, because non-metal is a non-conductor of electricity.

(b) First experiment shows that metal possesses property of malleability and ductility, while second experiment justifies that metals are good conductors of electricity while non-metals are non-conductor of electricity.

Question 6.
What are amphoteric oxides? Give two examples of amphoteric oxides.
Answer:
Metal oxides which react with both acids and bases to forms salt and water are called amphoteric oxides.
Examples:

• Aluminium oxide (Al₂O₃)
• Zinc oxide (ZnO)

Aluminium oxide reacts with an acid and a base in the following manner :
Al₂O₃ + 6HCl → 2AlCl₃ + 3H₂O

Zinc oxide reacts with an acid and a base in the following manner :
ZnO + 2HCl → ZnCl₂ + H₂O

Question 7.
Name two metals which will displace hydrogen from dilute acids and two metals which will not.
Answer:
Two metals which displace hydrogen from dilute acids are :

• zinc (Zn) and
• aluminium (Al).

Two metals which cannot displace hydrogen from dilute acids are :

• copper (Cu) and
• mercury (Hg).

Question 8.
In the electrolytic refining of a metal M, What would you take as an anode, the cathode and the electrolyte?
Answer:
In the electrolytic refining, Impure metal (M) is taken as an anode and thin strip of pure metal (M) Is taken as a cathode and solution of soluble salt of metal (M) Is taken as an electrolyte.

Question 9.
Pratyush took sulphur powder on a spatula and heated it. He collected the gas evolved by inverting a test tube over it as shown in figure below:
(a) What will be the action of gas on
(i) dry litmus paper?
(ii) moist litmus paper?
(b) Write a balanced chemical equation for the reaction taking place.

When sulphur powder is heated in air, it forms sulphur dioxide, which is acidic in nature and its aqueous solution is called sulphurous acid (H₂SO₃).
(a) Action of gas :

• There will be no effect of gas on dry litmus paper.
• Moist blue litmus paper changes its colour to red due to H⁺ ions present in the aqueous solution of H₂SO₃ formed from SO₂ obtained after burning sulphur.

(b) Balanced chemical equation for the above activity is as given below:
S(s) + O₂(g) → SO₂(g)

Question 10.
State two ways to prevent the rusting of iron.
Answer:
The rusting of iron can be prevented by painting, oiling, greasing, chrome plating, anodising or by making alloys.

Question 11.
What type of oxides are formed when non¬metals combine with oxygen?
Answer:
Non-metals combines with oxygen to form acidic oxides. For example, SO₂, SO₃, CO₂, Cl₂O₇, etc.

Question 12.
Give reasons :
(a) Platinum, gold and silver are used to make jewellery.
Answer:
Platinum, gold and silver are used to make jewellery, because these metals possesses lustre. They are malleable and ductile, as a result, jewellery of different shapes can be made. Moreover, these metals do not chemically react with water or air. Due to these properties platinum, gold and silver are used to make jewellery.

(b) Sodium, potassium and lithium are stored under oil.
Answer:
Sodium, potassium and lithium are highly reactive metals. They release hydrogen gas, when they react with air or moisture in air. Hydrogen gas is highly combustible and it catches fire. To prevent accidental fire, lithium, sodium and potassium are stored under oil.

(c) Aluminium is a highly reactive metal, yet it is used to make utensils for cooking.
Answer:
Aluminium is highly reactive metal. It reacts with oxygen of air to form aluminium oxide, which gets deposited as thin layer on the surface of aluminium. This layer acts as protective layer and prevents further reaction of aluminium with oxygen. Moreover it is light in weight and a good conductor of heat. Also, it’s manufacturing cost is very low in comparison to other metals. Therefore, most of the utensils for cooking are made from aluminium.

(d) Carbonate and sulphide ores are usually converted into oxides during the process of extraction.
Answer:
It is easier to obtain a metal from its oxide. Hence, it is essential to convert carbonate and sulphide ores into oxide ores during the extraction of metal. Extraction of metal by reduction of oxide ores is easier than extraction from its carbonate or sulphide ores.

Question 13.
You must have seen tarnished copper vessels being cleaned with lemon or tamarind juice. Explain why these sour substances are effective in cleaning the vessels.
Answer:
Copper vessels are tarnished and corroded due to formation of copper oxide layer on its surface. The green coating on copper vessels is due to formation of basic copper carbonate. On cleaning with lemon or tamarind juice, the citric acid present in them neutralises the basic copper carbonate and dissolves the layer, there by helps to regain the lustre of copper utensils.

Question 14.
Distinguish between metal and non-metal on the basis of their chemical properties.
Answer:

Metals	Non-metals
1. They are electro-positive elements.	1. They are electro-negative elements.
2. Aqueous solutions of metal oxides are basic.	2. Aqueous solutions of non-metal oxides are acidic.
3. Hydrogen gas is evolved when metals reacts with dilute acid.	3. When non-metal react with dilute acid hydrogen gas is not evolved.
4. Oxides of metals are basic in nature. For example, Na2O	4. Oxides of non-metals are acidic in nature. For example, SO2, CO
5. Atoms of metal possess one, two or three electrons in their outermost shell.	5. Atoms of non-metals possesses more than three electrons in their outermost shell.

Question 15.
A man went door to door posing as a goldsmith. He promised to bring back the glitter of old and dull gold ornaments. An unsuspecting lady gave a set of gold bangles to him which he dipped in a particular solution. The bangles sparkled like new but their weight was reduced drastically. The lady was upset but after a futile argument the man beat a hasty retreat. Can you play the detective to find out the nature of the solution he had used?
Answer:
That man was using a solution of aqua regia; which is a mixture of concentrated hydrochloric acid and concentrated nitric acid in the ratio of 3 : 1 by volume. Gold dissolves in aqua regia.

Question 16.
Give reason : Why copper is used to make hot water tanks and not steel (an alloy of iron.)?
Answer:
Copper does not react with cold and hot water nor it reacts with steam of water. Hence copper can be used to make hot water tanks.

But, steel is an alloy of an iron and it reacts with steam of water, hence, iron in steel is slowly corroded.
Therefore, copper is used for making hot water tanks and not steel.

Jharkhand Board Class 10 Science Metals and Non-metals InText Questions and Answers

Question 1.
Give an example of a metal which …
(i) is a liquid at room temperature.
(ii) can be easily cut with a knife.
(iii) is the best conductor of heat.
(iv) is a poor conductor of heat.
Answer:
(i) Mercury is a liquid at room temperature.
(ii) Sodium, potassium can be easily cut with a knife.
(iii) Silver and copper are good conductor of heat.
(iv) Lead is a poor conductor of heat.

Question 2.
Explain the meaning of malleable and ductile.
Answer:

1. Malleable : Malleable indicates a tendency of a metal to be brought in sheet form by hammering.
2. Ductile: Ductile indicates a tendency of a s metal to be brought in thin wire form.

Question 3.
Why is sodium kept immersed in kerosene oil?
Answer:
Sodium is highly reactive metal. It reacts with oxygen of air at room temperature. This reaction is highly exothermic. Thus, to prevent the reaction of sodium with oxygen, it is kept immersed in kerosene oil.

Question 4.
Write equations for the reactions of (i) Iron with steam.
(ii) Calcium and potassium with water.
Answer:
(i) Iron with steam:
4H₂O(g) + 3Fe(s) → Fe₃O₄(s) + 4H₂(g)

(ii) Calcium and potassium with water :

Question 5.
Samples of four metals A, B, C and D were taken and added to the following solution one by one. The results obtained have been tabulated as follows:

Metal	Iron (II) sulphate	Copper (II) sulphate	Zinc sulphate	Silver nitrate
A	No reaction	Displacement
B	Displacement		No reaction
C	No reaction	No reaction	No reaction	Displacement
D	No reaction	No reaction	No reaction	No reaction

Use the table above to answer the following questions about metals A, B, C and D:
(1) Which is the most reactive metal?
(2) What would you observe if B is added to a solution of copper (II) sulphate?
(3) Arrange the metals A, B, C and D in the order of decreasing reactivity.
Answer:
(1) Metal B is the most reactive metal.

(2) Blue colour of copper (II) sulphate solution disappears and reddish brown copper metal is < deposited on the metal B.

(3) Decreasing order of reactivity is s B > A > C > D.

Question 6.
Which gas is produced when dilute hydrochloric acid is added to a reactive metal? Write the chemical reaction when iron reacts with dilute H₂SO₄.
Answer:
When a reactive metal reacts with dilute hydrochloric acid, it forms hydrogen gas. The reactive metal displaces the hydrogen from acid releasing hydrogen gas.

Question 7.
What would you observe when zinc is added to a solution of iron (II) sulphate?
Write the chemical reaction that takes place.
Answer:
Zinc (Zn) is more reactive than iron (Fe). Hence, when it is added to iron (II) sulphate, it displaces the iron metal. As a result, the colour of the solution fades from green to colourless due to formation of zinc sulphate, and the greyish black coloured iron metal gets displaced.
Zn(s) + FeSO₄(aq) → ZnSO₄(aq) + Fe(s)

Question 8.
Name two metals which displace hydrogen from dilute acid and name two metals which cannot displace hydrogen from dilute acids.
Answer:
Two metals which displace hydrogen from dilute acids are:

• zinc (Zn) and
• aluminium (Al).

Two metals which cannot displace hydrogen from dilute acids are:

• copper (Cu)
• mercury (Hg).

Question 9.
Explain the electronic configuration of noble gases (He, Ne, Ar); metals (Na, Mg, Al, K, Ca) and non-metals (N, O, F, E S, Cl).
Answer:
Electronic Configurations of Some Elements

Question 10.
What are ionic compounds or electrovalent compounds? Explain with example.
OR
Explain the formation of sodium chloride (NaCl).
Answer:
The compounds formed by the transfer of electrons from a metal to a non-metal are known as Ionic compounds or electrovalent compounds. Atomic number of sodium is 11. Sodium atom has one electron in its outermost shell (M-shell). Sodium atom loses the electron from its M-shell and forms sodium cation (Na⁺) and acquires stable complete octet structure of noble gas neon (Ne).

Similarly, atomic number of chlorine is 17. Chlorine atom has seven electrons in its outermost shell (M-shell). Chlorine atom gains one e^– which is lost by sodium atom and forms chloride anion (Cl^–) and acquires stable complete octet structure of noble gas argon (Ar).

Sodium cation (Na⁺) and chloride anion (Cl^–) being oppositely charged attract each other and are held by strong electrostatic forces of attraction and exists as sodium chloride (NaCl).

Sodium chloride exists as a group of oppositely charged ions.

Question 11.
State the formation of magnesium chloride by the transfer of electrons.
Answer:
Atomic number of magnesium is 12. It loses its two electrons from outermost shell and acquires complete octet stable structure.

Similarly, atomic number of chlorine is 17. It gains one electron and acquires complete octet stable structure.

Thus, two electrons lost by magnesium atom are gained by two chlorine atoms (each one gets one electron) and forms magnesium chloride.

Question 12.
Explain the electronic configuration of noble gases (He, Ne, Ar); metals (Na, Mg, Al, K, Ca) and non-metals (N, O, F, P, S, Cl).
Answer:
Electronic Configurations of Some Elements

Question 13.
What are ionic compounds or electrovalent compounds? Explain with example.
OR
Explain the formation of sodium chloride (NaCl).
Answer:
The compounds formed by the transfer of electrons from a metal to a non-metal are known as Ionic compounds or electrovalent compounds. Atomic number of sodium is 11. Sodium atom has one electron in its outermost shell (M-shell). Sodium atom loses the electron from its M-shell and forms sodium cation (Na⁺) and acquires stable complete octet structure of noble gas neon (Ne).

Similarly, atomic number of chlorine is 17. Chlorine atom has seven electrons in its outermost shell (M-shell). Chlorine atom gains one e^– which is lost by sodium atom and forms chloride anion (Cl^–) and acquires stable complete octet structure of noble gas argon (Ar).

Sodium cation (Na⁺) and chloride anion (Cl^–) being oppositely charged attract each other and are held by strong electrostatic forces of attraction and exists as sodium chloride (NaCl).

Sodium chloride exists as a group of oppositely charged ions.

Question 14.
State the formation of magnesium chloride by the transfer of electrons.
Answer:
Atomic number of magnesium is 12. It loses its two electrons from outermost shell and acquires complete octet stable structure.

Similarly, atomic number of chlorine is 17. It gains one electron and acquires complete octet stable structure.

Thus, two electrons lost by magnesium atom are gained by two chlorine atoms (each one gets one electron) and forms magnesium chloride.

Activity 3.1 [T. B. Pg. 37]

Aim : To study the lustrous properties of metals.

Activity:

• Take samples of iron, copper, aluminium and magnesium. Note the appearance of surface of each sample.
• Clean the surface of each sample by rubbing them with sand paper and note their appearance again.

Questions :

Question 1.
How does the surface of samples of metal appear?
Answer:
The surface of samples of metal appear dull.

Question 2.
What happens when the surface of sample of metal is rubbed with sand paper?
Answer:
When the surface of sample of metal is rubbed with sand paper, it appears shiny.

Question 3.
How does the surface of metal appear in their pure form?
Answer:
Metals have shining surface in their pure form.

Question 4.
Name the shining property of metal.
Answer:
The property of shining surface of metal is known as metallic lustre.

Activity 3.2 [T. B. Pg. 37]

Aim : To study the hardness of metals.

Caution : Always handle sodium metal with care.

Activity:

• Take small pieces of iron, copper, aluminium and magnesium.
• Try to cut these metals with a sharp knife and note your observations.
• Hold a piece of sodium metal with a pair of tongs, put it on a watch-glass and try to cut it with a knife.
  What do you observe?

Questions:

Question 1.
Can we cut the metals such as an iron, copper, aluminium and magnesium with a knife?
Answer:
No, we can’t cut.

Question 2.
Can sodium metal be cut with a knife?
Answer:
Yes, we can cut sodium metal into pieces.

Question 3.
Which property of metal is seen in the above activity?
Answer:
Metals are hard and their hardness varies from metal to metal.

Activity 3.3 [T. B. Pg. 38]

Aim : To study that metals are malleable.

Activity:

• Take pieces of iron, zinc, lead and copper?
• Place any one metal on a block of iron and strike it four to five times with a hammer.
• What do you observe?
• Repeat the same with other metals.
• Record the change in the shape of these < metals.

Questions:

Question 1.
Which property of metal is observed in this activity?
Answer:
Metals can be hammered into thin sheets.

Question 2.
What is malleability of metals?
Answer:
The property of metals to be converted into thin sheets when hammered is known as ‘Malleability of metals’.

Question 3.
Name the metals which are most malleable in nature.
Answer:
Gold and silver.

Activity 3.4 [T. B. Pg. 38]

Aim: To study that metals are ductile.

Activity:
Take samples of metals such as iron, copper, aluminium and lead.

Questions:

Question 1.
What is meant by ductility?
Answer:
The ability of metals to be drawn into? thin wires (filaments) is called ductility.

Question 2.
Which metal is most ductile?
Answer:
Gold is most ductile metal.

Question 3.
What is the maximum length of wire which (can be drawn from one gram of gold?
Answer:
2 km length

Question 4.
Why the metals can be given different shapes?
Answer:
Metals can be given different shapes due to their properties like malleability and ductility.

Activity 3.5 [T. B. Pg. 38]

Aim : To show that metals are good conductors of heat and possesses high melting point.

Activity:

• Take an aluminium or copper wire. Clamp this wire on a stand as shown in the figure 3.1.
• Fix a pin to the free end of the wire using wax.
• Heat the wire with a spirit lamp, candle or a burner near the place where it is clamped.
• What do you observe after some time?
• Note your observations.
• Does the metal wire melt?
  

Questions:

Question 1.
What happens to a pin attached to the wire?
Answer:
Due to heating, metal wire expands and the pin is displaced forward. This indicate that heat flows through the wire and melts the wax but wire does not melt.

Question 2.
Which property of metal is observed in the above activity?
Answer:
The above activity shows that metals are good conductors of heat and have high melting point.

Question 3.
Which metal is used as the best conductor of heat?
Answer:
Silver and copper are best conductors of heat.

Question 4.
Which metals are poor conductors of heat?
Answer:
Lead and mercury are poor conductors of heat.

Activity 3.6 [T. B. Pg. 39]

Aim: To study the property of electrical conductivity of metals.

Activity:

• Set up an electric circuit as shown in the figure 3.2.
• Place the metal to be tested in the circuit between terminals A and B as shown.
  

Questions :

Question 1.
Which property of metal is observed in the above activity?
Answer:
It is observed that metals are good conductors of electricity.

Question 2.
Which coating is used on electric wire?
Answer:
The coating of polyvinyl chloride is formed on electric wire.

Question 3.
Does the bulb glow? What does it indicate?
Answer:
The bulb glows. It indicate that electric current flows through the metal.

Activity 3.7 [T. B. Pg. 39]

Aim : To study the properties of non-metals.

Activity:

• Collect the samples of carbon (coal or graphite), sulphur and iodine.
• Carry out the activities 3.1 to 3.4 and 3.6 with these non-metals and record your observations.

Questions:

Question 1.
State the physical states of non-metals.
Answer:
Non-metals exist in solid, liquid and gaseous states at room temperature.

Question 2.
State the common properties of non-metals based on the above activities.
Answer:

• Non-metals are non-conductors of heat and electricity.
• Boiling points of non-metals are comparatively lower.
• Non-metals do not possess property of malleability and ductility.

Activity 3.8 IT. B. Pg. 40]

Aim: To test the acidity and basicity of oxides.

Activity:

• Take a magnesium ribbon and some sulphur powder.
• Burn the magnesium ribbon. Collect the ashes formed and dissolve it in water.
• Test the resultant solution with red and blue litmus paper.
• Is the product formed on burning magnesium ribbon acidic or basic?
• Now, burn sulphur powder. Place a test tube over the burning sulphur to collect the fumes produced.
• Add some water to the above test tube and shake.
• Test this solution with blue and red litmus paper.
• Is the product formed on burning sulphur acidic or basic?
• Can you write equations for these reactions?

Questions :

Question 1.
What is formed on burning magnesium?
Answer:
Magnesium oxide (MgO) is formed on burning magnesium.

Question 2.
Is the magnesium oxide acidic or basic?
Answer:
Magnesium oxide is basic in nature.

Question 3.
Which product is obtained on burning sulphur?
Answer:
Sulphur dioxide (SO₂) is obtained.

Question 4.
Is the product formed on burning sulphur acidic or basic?
Answer:
It is acidic.

Question 5.
Write the equations of chemical reactions which occur in the activity 3.8.
Answer:

• 2Mg(s) + O₂(g) → 2MgO(s)
• MgO(s) + H₂O(l) → Mg(OH)₂(aq)
• S(s) + O₂(g) → SO₂(g)
• SO₂(g) + H₂O(l) → H₂SO₃(aq)

Question 6.
Is the sulphur dioxide acidic or basic?
Answer:
It is an acidic.

Activity 3.9 [T. B. Pg. 41]

Aim: To study the burning of metals in air.

Caution:

• This activity needs the teacher’s assistance.
• It would be better, if students wear goggles for eye protection.

Activity:

• Take samples of metals such as aluminium, copper, iron, lead, magnesium, zinc and? sodium.
• Hold any sample of metal taken above with a pair of tongs and try burning over a flame.
• Repeat the same with the other metal samples.
• Collect the product, if formed.
• Let the products and the metal surface cool down.
• Which metals burn easily?
• What flame colour did you observe when the metal burnt?
• How does the metal surface appear after burning?
• Arrange the metals in the decreasing order of their reactivity towards oxygen.
• Are the products soluble in water?

Questions:

Question 1.
Which metal burns easily in air?
Answer:
Magnesium burns easily in air.

Question 2.
What colour does Na, Mg, Cu and Al impart to the oxidising flame?
Answer:

• Na → Yellow flame
• Mg → Dazzling white flame
• Cu → Greenish blue flame
• Al → White flame

Question 3.
How does the metal surface appear after burning?
Answer:
Silvery white.

Question 4.
What is the solubility of product in water obtained by the reaction of Cu, Fe, Zn, Al with oxygen?
Answer:
The products obtained by the reaction of Cu, Fe, Zn and Al with oxygen are insoluble in water.

Question 5.
Arrange the metals such as Al, Cu, Fe, Pb, Mg, Zn and Na in the decreasing order of their reactivity towards oxygen.
Answer:
Na > Mg > Al > Zn > Fe > Pb > Cu.

Question 6.
Which amongst the given metals forms water soluble product after heating it?
Answer:
Amongst the given metals, only sodium metal on heating forms oxide which is soluble in water.

Activity 3.10 [T. B. Pg. 42]

Aim : To study the reaction of water with metals.

Caution :
This activity requires the teacher’s help.

Activity:

• Take samples of metals such as aluminium, copper, iron, lead, magnesium, zinc, calcium, gold, silver, sodium and potassium.
• Put small pieces of the samples separately in beakers half filled with cold water.
• Which metals reacted with cold water? Arrange them in the increasing order of their reactivity with cold water.
• Did any metal produce fire on water?
• Does any metal start floating after some time?
• Put the metals that did not react with cold water in beakers half filled with hot water.
• For the metals that did not react with hot water, arrange the apparatus as shown in the figure 3.3 and observe their reaction with steam.
• Which metals did not react even with steam?
• Arrange the metals in the decreasing order of reactivity with water.
  

Questions:

Question 1.
Which metals react with cold water?
Answer:
Sodium, potassium and calcium react with cold water.

Question 2.
Which metals produce fire on water?
Answer:
Sodium and potassium produce fire on water.

Question 3.
Which metal floats on water?
Answer:
Calcium and magnesium float on water.

Question 4.
Which metal does not react with cold water, but reacts with hot water?
Answer:
Magnesium does not react with cold water but it reacts with hot water.

Question 5.
Which metal does not react with either cold or hot water but reacts with steam?
Answer:
Aluminium, iron and zinc do not react with cold and hot water, but they react with steam.

Question 6.
Arrange the given metals (in activity) in the decreasing order of their reactivity with water.
Answer:
Decreasing order of the reactivity of metals with water is given as follows:
K > Na > Ca > Mg > A1 > Zn > Fe
Pb, Cu, Ag and Au does not react with water.

Question 7.
Arrange sodium, potassium and calcium metals in the increasing order of their reactivity with water.
Answer:
Ca < Na < K Question 8. Which metals do not react with cold water and steam? Answer: Pb, Cu, Ag and Au do not react with cold water and steam.

Activity 3.11 [T. B. Pg. 44]

Aim : To study the reaction of metal with an acid.

Activity: Collect the samples of pieces of metals such as magnesium, aluminium, zinc, iron and copper. Put the samples separately in test tubes containing dilute hydrochloric acid. Suspend thermometers in the test tubes. Which metals reacted vigorously with dilute hydrochloric acid? With which metal did you record the highest temperahire? Arrange the metals in the decreasing order of their reactivity with dilute acids.

Questions:

Question 1.
Which metal reacts vigorously with dilute hydrochloric acid?
Answer:
Magnesium metal reacts vigorously with dilute hydrochloric acid.

Question 2.
Which metal does not react with hydrochloric acid?
Answer:
Copper (Cu) metal do not react with hydrochloric acid.

Question 3.
With which metal the rise of temperature is maximum during the reaction?
Answer:
The rise of temperature is maximum in case of magnesium during the reaction.

Question 4.
Arrange the metals Mg, Al, Zn and Fe in the decreasing order of their reactivity towards dilute hydrochloric acid.
Answer:
Mg > Al > Zn > Fe

Activity 3.12 [T. B. Pg. 44 – 45]

Aim : To study the reaction of metal with solutions of other metal salts.

Activity:

• Take a clean wire of copper and an iron nail.
• Put the copper wire in a solution of iron sulphate and the iron nail in a solution of copper sulphate taken separately in test tubes (figure 3.4).
• Record your observations after 20 minutes.
• In which test tube did you find that a reaction has occurred?
• On what basis can you say that a reaction has actually taken place?
• Write a balanced chemical equation for the reaction that has taken place.
• Name the type of reaction.
  

Questions:

Question 1.
In which test tube, reaction has occurred? Write the balanced chemical equation for the reaction.
Answer:
The following reaction occurred in a test tube containing iron nail dipped in a copper sulphate solution :
Fe(s) + CuSO₄(aq) → FeSO₄(aq) + Cu(s)
In this reaction, more reactive Fe displaces less reactive Cu.

Question 2.
On what basis can you say that a reaction has actually taken place?
Answer:
Blue colour of copper sulphate solution turns green during reaction. This change indicates that reaction has occurred.

Question 3.
Name the type of reaction occurring in the above activity.
Answer:
Displacement reaction occurs in the above acitivity.

Question 4.
Why does the reaction not occurred in the test tube containing copper wire dipped in a iron sulphate solution?
Answer:
Because, iron (Fe) is more reactive than copper (Cu); the reaction does not take place.

Activity 3.13 [T. B. Pg. 48]

Aim : To study the properties of ionic compounds, s

Activity:

• Take samples of sodium chloride, potassium s iodide and barium chloride.
• What is the physical state of these salts?
• Take a small amount of a sample on a metal ; spatula and heat it directly on the flame (figure 3.5). Repeat with other samples.
• What did you observe? Did the samples s impart any colour to the flame?
• Try to dissolve the samples in water, petrol and kerosene. Are they soluble?
• Make a circuit as shown in figure 3.6 and insert the electrodes into a solution of one salt, s
• What did you observe? Test the other samples too in this manner.
• What is your inference about the nature of these compounds?
  

Answers of the questions asked in the above activity are given in the following table :