Jharkhand Board JAC Class 10 Maths Solutions Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4 Textbook Exercise Questions and Answers.
JAC Board Class 10 Maths Solutions Chapter 3 Pair of Linear Equations in Two Variables Exercise 3.4
Question 1.
Solve the following pair of linear equations by the elimination method and the substitution method:
1. x + y = 5 and 2x – 3y = 4
2. 3x + 4y = 10 and 2x – 2y = 2
3. 3x – 5y – 4 = 0 and 9x = 2y + 7
4. \(\frac{x}{2}+\frac{2 y}{3}=-1\) and x – \(\frac{y}{3}\) = 3
Solution:
1. Elimination method:
x + y = 5 ………(1)
2x – 3y = 4 ……..(2)
We multiply equation (1) by 3 and equation (2) by 1 to get following equations:
3x + 3y = 15 ……(3)
2x – 3y = 4 ……(4)
Adding equations (3) and (4), we get
(3x + 3y) + (2x – 3y) = 15 + 4
∴ 5x = 19
∴ x = \(\frac{19}{5}\)
Substituting x = \(\frac{19}{5}\) in equation (1), we get
\(\frac{19}{5}\) + y = 5
∴ y = 5 – \(\frac{19}{5}\)
∴ y = \(\frac{6}{5}\)
Thus, the solution of the given pair of linear equations is x = \(\frac{19}{5}\), y = \(\frac{6}{5}\)
Substitution method:
x + y = 5 ……(1)
2x – 3y = 4 ……(2)
From equation (1), we get y = 5 – x.
Substituting y = 5 – x in equation (2), we get
2x – 3(5 – x) = 4
∴ 2x – 15 + 3x = 4
∴ 5x = 19
∴ x = \(\frac{19}{5}\)
Substituting x = \(\frac{19}{5}\) in y = 5 – x, we get
y = 5 – \(\frac{19}{5}\)
∴ y = \(\frac{6}{5}\)
Thus, the solution of the given pair of linear equations is x = \(\frac{19}{5}\), y = \(\frac{6}{5}\)
2. Elimination method:
3x + 4y = 10 ……(1)
2x – 2y = 2 ……(2)
We multiply equation (1) by 1 and equation (2) by 2 to get following equations:
3x + 4y = 10 ……(3)
4x – 4y = 4 ……(4)
Adding equations (3) and (4), we get
(3x + 4y) + (4x – 4y) = 10 +4
∴ 7x = 14
∴ x = 2
Substituting x = 2 in equation (1), we get
3(2) + 4y = 10
∴ 4y = 10 – 6
∴ 4y = 4
∴ y = 1
Thus, the solution of the given pair of linear equations is x = 2, y = 1.
Substitution method:
3x + 4y = 10 ……(1)
2x – 2y = 2 ……(2)
From equation (2), we get 2x = 2y + 2.
i.e., x = y + 1.
Substituting x = y + 1 in equation (1), we get
3(y + 1) + 4y = 10
∴ 3y + 3 + 4y = 10
∴ 7y = 7
∴ y = 1
Substituting y = 1 in x = y + 1, we get
x = 1 + 1
x = 2
Thus, the solution of the given pair of linear equations is x = 2, y = 1.
3. Elimination method:
3x – 5y – 4 = 0 ……(1)
9x = 2y + 7 ……(2)
i.e., 3x – 5y = 4 ……(3)
9x – 2y = 7 ……(4)
We multiply equation (3) by 3 and equation (4) by 1 to get following equations:
9x – 15y = 12 ……(5)
9x – 2y = 7 ……(6)
Subtracting equation (5) from equation (6),
we get
(9x – 2y) – (9x – 15y) = 7 – 12
∴ 9x – 2y – 9x + 15y = -5
∴ 13y = -5
∴ y = –\(\frac{5}{13}\)
Substituting y = –\(\frac{5}{13}\) in equation (5), we get
9x – 15(-\(\frac{5}{13}\)) = 12
∴ 9x + \(\frac{75}{13}\) = 12
∴ 9x = 12 – \(\frac{75}{13}\)
∴ 9x = \(\frac{75}{13}\)
∴ x = \(\frac{9}{13}\)
Thus, the solution of the given pair of linear equations is x = \(\frac{9}{13}\), y = –\(\frac{5}{13}\)
Substitution method:
3x – 5y – 4 = 0 ………(1)
9x = 2y + 7 ………(2)
From equation (2), we get x = \(\frac{2 y+7}{9}\).
Substituting x = \(\frac{2 y+7}{9}\) in equation (1).
we get
3(\(\frac{2 y+7}{9}\)) – 5y – 4 = 0
∴ \(\frac{2 y+7}{9}\) – 5y – 4 = 0
∴ 2y + 7 – 15y – 12 = 0 (Multiplying by 3)
∴ -13y – 5 = 0
∴ -13y = 5
∴ y = –\(\frac{5}{13}\)
Substituting y = –\(\frac{5}{13}\) in x = \(\frac{2 y+7}{9}\)
we get,

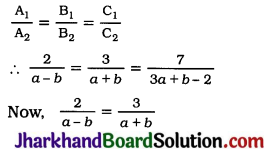
Thus, the solution of the given pair of linear equations is x = \(\frac{9}{13}\), y = –\(\frac{5}{13}\).
4. Elimination method:
\(\frac{x}{2}+\frac{2 y}{3}=-1\) ………(1)
\(x-\frac{y}{3}=3\) ………(2)
We multiply equation (1) by 3 and equation (2) by 6 to get following equations:
\(\frac{3}{2}\)x + 2y = -3 ………(3)
6x – 2y = 18 ………(4)
Adding equations (3) and (4), we get
(\(\frac{3}{2}\)x + 2y) + (6x – 2y) = -3 + 18
∴ \(\frac{15}{2}\)x = 15
∴ x = 2
Substituting x = 2 in equation (3), we get
\(\frac{3}{2}\)(2) + 2y = -3
∴ 3 + 2y = -3
∴ 2y = -6
∴ y = -3
Thus, the solution of the given pair of linear equations is x = 2, y = -3.
Substitution method:
\(\frac{x}{2}+\frac{2 y}{3}=-1\) ………(1)
\(x-\frac{y}{3}=3\) ………(2)
From equation (2), we get x = \(\frac{y}{3}+3\)
Substituting x = \(\frac{y}{3}+3\) in equation (1).
we get

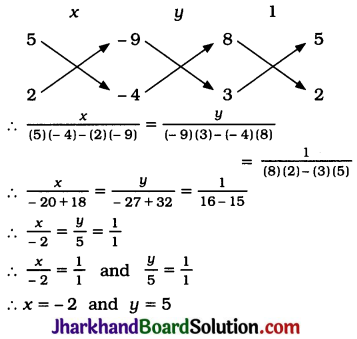
Substituting y = -3 in x = \(\frac{y}{3}+3\), we get
x = \(\frac{-3}{3}+3\)
∴ x = -1 + 3
∴ x = 2
Thus, the solution of the given pair of linear equations is x = 2, y = -3.
![]()
Form the pair of linear equations in the following problems and find their solutions (if they exist) by the elimination method:
Question 1.
If we add 1 to the numerator and subtract 1 from the denominator, a fraction reduces to 1. It becomes \(\frac{1}{2}\) if we only add 1 to the denominator. What is the fraction?
Solution:
Let the numerator of the required fraction be x and the denominator be y.
Then, the required fraction is \(\frac{x}{y}\)
From the first condition given, we get
\(\frac{x+1}{y-1}=1\)
∴ x + 1 = y – 1
∴ x – y = -2 …..(1)
From the second condition, we get
\(\frac{x}{y+1}=\frac{1}{2}\)
∴ 2x = y + 1
∴ 2x – y = 1 ………(2)
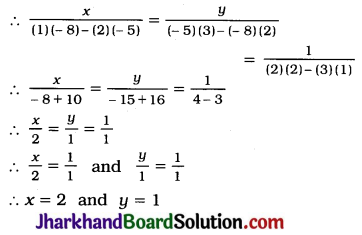
Now, subtracting equation (1) from equation (2), we get
(2x – y) – (x – y) = 1 – (-2)
∴ 2x – y – x + y = 3
∴ x = 3
Substituting x = 3 in equation (1), we get
3 – y = -2
∴ -y = – 2 – 3
∴ -y = -5
∴ y = 5
So, the fraction \(\frac{x}{y}=\frac{3}{5}\)
Thus, the pair of linear equations formed is x – y = -2 and 2x – y = 1; and the required fraction is \(\frac{3}{5}\)
Question 2.
Five years ago, Nuri was thrice as old as Sonu. Ten years later, Nuri will be twice as old as Sonu. How old are Nuri and Sonu ?
Solution:
Let the present age of Nuri be x years. and the present age of Sonu be y years.
Five years ago, the age of Nuri was (x – 5) years and the age of Sonu was (y – 5) years.
Then, from the first condition given, we get
(x – 5) = 3(y – 5)
∴ x – 5 = 3y – 15
∴ x – 3y = -10 ……….(1)
Ten years later the ages of Nuri and Sonu will be (x + 10) years and (y + 10) years respectively.
Then, from the second condition given, we get
(x + 10) = 2(y + 10)
∴ x + 10 = 2y + 20
∴ x – 2y = 10 ………..(2)
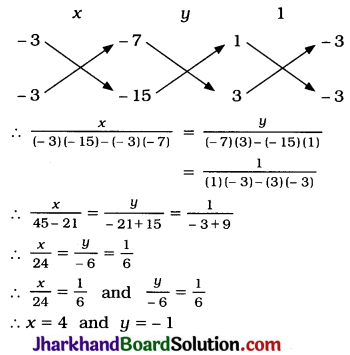
Subtracting equation (1) from equation (2).
we get
(x – 2y) – (x – 3y) = 10 – (-10)
∴ x – 2y – x + 3y = 10 + 10
∴ y = 20
Substituting y = 20 in equation (2).
we get
x – 2 (20) = 10
∴ x – 40 = 10
∴ x = 50
So, the present ages of Nuri and Sonu are 50 years and 10 years.
Thus, the pair of linear equations formed is x – 3y = -10 and x – 2y = 10 and the present ages of Nuri and Sonu are 50 years and 20 years respectively.
![]()
Question 3.
The sum of the digits of a two digit number is 9. Also, nine times this number is twice the number obtained by reversing the order of the digits. Find the number.
Solution:
Let the digit at tens place be x and the digit at units place be y in the original number.
Then, the original number = 10x + y.
From the first condition given, we get x + y = 9 ………..(1)
If the digits are reversed, in the new number the digit at tens place is y and the digit at units place is x.
Then, the new number = 10y + x.
From the second condition given, we get
9(10x + y) = 2(10y + x)
∴ 90x + 9y = 20y + 2x
∴ 88x – 11y = 0
∴ 8x – y = 0 (Dividing by 11) ……….(2)
Adding equations (1) and (2), we get
(x + y) + (8x − y) = 9 + 0
∴ 9x = 9
∴ x = 1
Substituting x = 1 in equation (1), we get
1 + y = 9
∴ y = 8
So, the original number = 10x + y
= 10(1) + 8 = 18
Thus, the pair of linear equations formed is x + y = 9 and 8x – y = 0; and the original number is 18.
Question 4.
Meena went to a bank to withdraw ₹ 2000. She asked the cashier to give her ₹ 50 and ₹ 100 notes only. Meena got 25 notes in all. Find how many notes of ₹ 50 and ₹ 100 she received.
Solution:
Suppose Meena received x notes of ₹ 50 and y notes of ₹ 100.
So, the total amount received by her = ₹ (50x + 100y)
From the first condition given, the total amount is ₹ 2000. So, we get
50x + 100y = 2000
∴ x + 2y = 40 (Dividing by 50) ……(1)
From the second condition given, we get
x + y = 25 ……(2)
Subtracting equation (2) from equation (1),
we get
(x + 2y) – (x + y) = 40 – 25
∴ y = 15
Substituting y = 15 in equation (2), we get
x + 15 = 25
∴ x = 10
Hence, Meena received 10 notes of ₹ 50 and 15 notes of ₹ 100.
Thus, the pair of linear equations formed is x + 2y = 40 and x + y = 25, and Meena received 10 notes of ₹ 50 and 15 notes of ₹ 100.
![]()
Question 5.
A lending library has a fixed charge for the first three days and an additional charge for each day thereafter. Saritha paid ₹ 27 for a book kept for seven days, while Susy paid ₹ 21 for the book she kept for five days. Find the fixed charge and the charge for each extra day.
Solution:
Let the fixed charge for first three days be ₹ x and the additional charge for each day exceeding the first three days be ₹ y. Saritha kept the book for 7 days.
So, she has to pay the fixed charge plus the additional charge for 4(7 – 3) days. Hence, we get the following equation for Saritha:
x + 4y = 27 …………(1)
Similarly, Susy has to pay the fixed charge plus the addition charge for 2 (5 – 3) days.
Hence, we get the following equation for Susy:
x + 2y = 21 ……….(2)
Subtracting equation (2) from equation (1),
we get
(x + 4y) – (x + 2y) = 27 – 21
∴ 2y = 6
∴ y = 3
Substituting y = 3 in equation (1), we get
x + 4(3) = 27
∴ x + 12 = 27
∴ x = 15
Hence, the fixed charge for first three days is ₹ 15 and the addition charge for each day thereafter is ₹ 3.
Thus, the pair of linear equations formed is x + 4y = 27 and x + 2y = 21; and the fixed charge and the additional charge per day are ₹ 15 and ₹ 3 respectively.
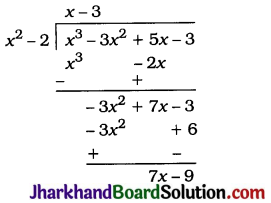

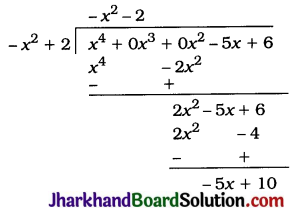
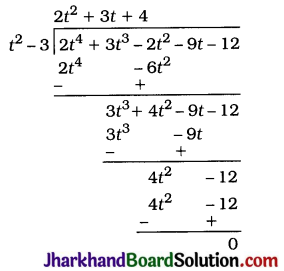
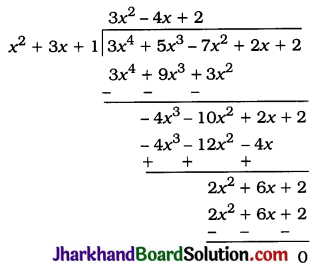

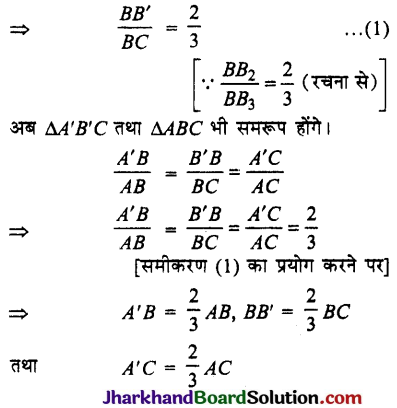
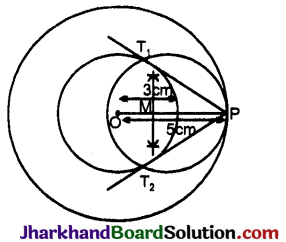
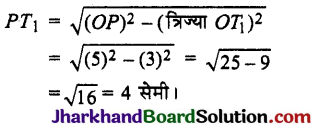
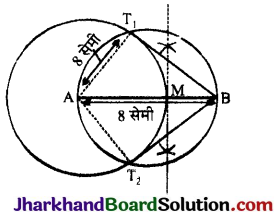


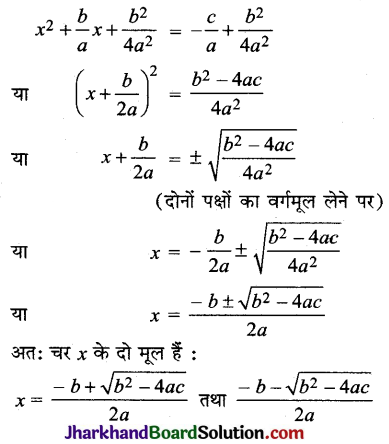
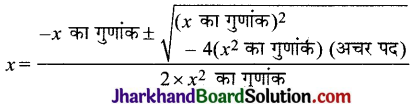
 जोड़ने पर बायाँ पक्ष पूर्ण वर्ग बन जाएगा।
जोड़ने पर बायाँ पक्ष पूर्ण वर्ग बन जाएगा।