Month: September 2024

JAC Board Class 9th Science Important Questions Chapter 10 Gravitation

Multiple Choice Questions 

Question 1.
The mass of a body on moon is 40 kg. What is its weight on the earth?
(a) 240 kg
(b) 392 N
(c) 240 N
(c) 400 kg
Answer:
(b) 392 N

Question 2.
The gravitational force between two objects is
(a) attractive at large distance only
(b) attractive at small distance only
(c) attractive at all distances
(d) attractive at large distance but repulsive at small distance
Answer:
(c) attractive at all distances

Question 3.
A body of mass 1 kg is attracted by the earth with a force equal to
(a) 9.8 N
(b) 1 N
(c) 6.67 × 10¹¹ N
(d) 9.8 m/s
Answer:
(a) 9.8 N

Question 4.
The earth attracts a body with a force of 10 N. With what force does that the body attract the earth?
(a) 10N
(b) 1 N
(c) \(\frac{1}{10 \mathrm{~N}}\)
(d) 2 N
Answer:
(a) 10N

Question 5.
The SI unit of G is
(a) Nm² kg^-1
(b) Nm² kg^-2
(c) Nm² kg
(d) N^-1 m² kg^-2
Answer:
(b) Nm² kg^-2

Question 6.
The gravitational force of attraction between two bodies of 1 kg each and 1 m apart is
(a) 6.67 × 10^-11 N
(b) 6.67 × 10^-8 N
(c) 6.67 × 10¹¹ N
(d) 6.67 × 10⁸ N
Answer:
(a) 6.67 × 10^-11 N

Question 7.
The value of acceleration due to gravity near the earth’s surface is
(a) 8.9 m^-2
(b) 9.8 ms^-2
(c) 8.9 cms^-2
(d) 9.8 cms^-2
Answer:
(b) 9.8 ms^-2

Question 8.
Consider an elevator moving downwards with an acceleration a The force exerted by a passenger of mass m on the floor of the elevator is
(a) ma
(b) ma – mg
(c) mg – ma
(d) mg + ma
Answer:
(c) mg – ma

Question 9.
If the earth suddenly shrinks to half of its present size, the value of acceleration due to gravity will
(a) become twice
(b) remain unchanged
(c) become half
(d) become four times
Answer:
(d) become four times

Question 10.
The weight of a body would not be zero
(a) at the centre of the earth
(b) during a free fall of an elevator
(c) in interplanetary space
(d) on a frictionless surface
Answer:
(d) on a frictionless surface

Question 11.
Newton’s law of gravitation
(a) can be verified in a laboratory
(b) cannot be verified but is true
(c) is valid only on earth
(d) is valid only in the solar system
Answer:
(a) can be verified in a laboratory

Question 12.
10 kg weight is equal to
(a) 9.8 N
(b) 98 N
(c) 980 N
(d) \(\frac{1}{9.8}\) N
Answer:
(b) 98 N

Question 13.
The weight of a body cannot be expressed in
(a) kg wt
(b) N
(c) dyne
(d) kg
Answer:
(d) kg

Question 14.
A stone is dropped from a cliff. Its speed after it has fallen 100m is
(a) 9.8 m/s
(b) 44.2 m/s
(c) 19.8 m/s
(d) 98 m/s
Answer:
(b) 44.2 m/s

Question 15.
At which of the following locations, the value of g is the largest?
(a) On top of the Mount Everest
(b) On top of Qutub Minar
(c) At a place on the equator
(d) A camp site in Antarctica
Answer:
(d) A camp site in Antarctica

Analysing & Evaluating Questions

Question 16.
An apple falls from a tree because of gravitational attraction between the earth and apple. If Ft is the magnitude of force exerted by the earth on the apple and F2 is the magnitude of force exerted by the apple on the earth, then
(a) F₁ is very much greater than F₂
(b) F₂ is very much greater than F₁
(c) F₁ is only a little greater than F₂
(d) F₁ and F₂ are equal
Answer:
(d) F₁ and F₂ are equal

Question 17.
An object is put one by one in three liquids having differei dnsities. The object floats with\(\frac{1}{9}\), \(\frac{2}{11}\) and \(\frac{3}{7}\) part of its volume outside dit liquid surface in liquids of densities d₁, d₂ and d₃ respectively. Which of the following is correct?
(a) d₁ > d₂ > d₃
(b) d₁ >d₂ <d₃
(c) d₁ < d₂ > d₃
(d) d₁ < d₂ < d₃
Answer:
(d) d₁ < d₂ < d₃

Question 18.
An object weighs 10N in air. When immersed fully in water, it weighs only 8N. The weight of the liquid displaced by the object will be
(a) 2 N
(b) 8 N
(c) 10 N
(d) 12
Answer:
(a) 2 N

Assertion Reason Questions

Directions: In the following questions, the Assertions and the Reasons have been put forward. Read the statements carefully and choose the correct alternative from the following:
(A) Both the assertion and the reason are correct and the reason is the correct explanation of the assertion.
(B) The assertion and the reason are correct but the reason is not the correct explanation of the assertion.
(C) The assertion is true but the reason is false.
(D) Both the statements are false.
1. Assertion: The law of gravitation is not applicable on the two bodies lying on the surface of the earth.
Reason: Law of gravitation is applied to celestial bodies only.
Answer:
(D) Both the statements are false.

2. Assertion: Mass of a body remains same at all the places.
Reason: Mass of a body is independent of acceleration due to gravity.
Answer:
(A) Both the assertion and the reason are correct and the reason is the correct explanation of the assertion.

3. Assertion: Value of acceleration due to gravity is greater at the poles than at the equator.
Reason: Distance between pole and the centre of the earth is less than the distance between the equator and the centre of the earth.
Answer:
(A) Both the assertion and the reason are correct and the reason is the correct explanation of the assertion.

4. Assertion: One can jump higher on the surface of the moon than on the earth. Reason: The value of g (acceleration due to gravity) on the moon is greater than that on the earth.
Answer:
(C) The assertion is true but the reason is false.

5. Assertion: Fluids exert an upthrust on the objects immersed in them.
Reason: The upthrust is equal to the weight of the liquid displaced.
Answer:
(B) The assertion and the reason are correct but the reason is not the correct explanation of the assertion.

Very Short Answer Type Questions

Question 1.
Define the term ‘gravitation’.
Answer:
Every object in this universe attracts every other object with a force known as ‘force of gravitation’. Gravitation is the force of attraction between any two bodies in the universe.

Question 2.
Give the SI unit and value of G.
Answer:
SI unit of G = \(\frac{\mathrm{Nm}^{2}}{\mathrm{~kg} \cdot \mathrm{kg}}\) = N m² kg^-2
Its value = 6.67 × 10^-11 N m² kg^-2.

Question 3.
g = GM/R², what do the symbols in this formula denote?
Answer:
g = Acceleration due to gravity
G = Gravitational constant
M = Mass of the earth
R = Radius of the earth

Question 4.
Name the scientist who first determined the value of G experimentally.
Answer:
Henry Cavendish first determined the value of G experimentally, in the year 1778, using a sensitive balance.

Question 5.
Why is G called a universal gravitational constant’?
Answer:
The value of constant G is same for any pair of objects in the universe. Also, its value does not depend on the nature of the intervening medium. That is why constant G is called ‘universal’, whether the bodies are big or small, or whether they are celestial or terrestrial.

Question 6.
State any two properties of gravitational force.
Answer:

1. It is always attractive in nature.
2. It does not depend on the nature of the medium between the two bodies.

Question 7.
Which force is responsible for the stability of our universe?
Answer:
The force of gravitation.

Question 8.
How is ‘g’ on the surface of the earth related to ‘G’?
Answer:
g = \(\frac{\mathrm{GM}}{\mathrm{R}^{2}}\) = \(\frac{1.67 \times\left(1.74 \times 10^{6}\right)^{2}}{6.67 \times 10^{-11}}\)

where M = mass of the earth,
R = radius of the earth.

Question 9.
How is weight of an object related to its mass?
Answer:
Weight (w) = mass (m) × acceleration due to gravity (g).

Question 10.
What is the SI unit of pressure?
Answer:
The SI unit of pressure = N/m² = Pascal.

Question 11.
Define thrust. What is the SI unit of thrust?
Answer:
The net force exerted by a body in a particular direction is called thrust. The SI unit of thrust is newton.

Question 12.
Why does a truck or a motorbike have much wider tyres?
Answer:
A truck or a motorbike has much wider types so that the pressure exerted by it can be distributed to more surface area of the road and avoid the wear and tear of tyres.

Question 13.
In what sense does the moon fall towards the earth? Why does it not actually fall on the earth’s surface?
Answer:
At each point of its orbit, the moon falls towards the earth instead of going straight. It does not fall on the earth because it is moving in a circular orbit. The moon is kept in its circular orbit by the centripetal force provided by the force of attraction of the earth.

Question 14.
The earth attracts an apple from the tree and it falls but the earth does not appear to move towards the apple. Why?
Answer:
The mass of the earth is extremely large as compared to that of the apple. So the acceleration produced is very small as compared to that in the apple. Hence, the motion of the earth towards the apple is not noticeable.

Question 15.
What do you mean by the term ‘free fall’?
Answer:
The motion of a body under the influence of the force of gravity alone is called a ‘free fall’.

Question 16.
Suppose a planet exists whose mass and radius, both are half that of the earth. Calculate the acceleration due to gravity on the surface of this planet.
Answer:
On the surface of the earth, g = \(\frac{\mathrm{GM}}{\mathrm{R}^{2}}\)
On the surface of the planet, \(g^{\prime}=\frac{G\left(\frac{M}{2}\right)}{\left(\frac{R}{2}\right)^{2}}=\frac{2 G M}{R^{2}}=2 g\)

Question 17.
Define mass of a body. What is its SI unit?
Answer:
The mass of a body represents the quantity of matter contained in the body. It gives a measure of inertia of the body. The greater the mass of a body, the greater is its inertia. The SI unit of mass is kilogram (kg).

Question 18.
Define one kilogram – weight. How many newtons are there in 1kg wt?
Answer:
One kilogram – weight (kg – wt)
= 1kg × 9.8 m/s² = 9.8 N.

Question 19.
State Archimedes’ principle.
Answer:
This principle states that when a body is immersed fully or partially in a fluid, it experiences an upward thrust equal to the weight of the fluid displaced by it.

Analysing & Evaluating Questions

Question 20.
A beam balance is used for measuring the mass of a body, whereas a spring balance gives the weight of a body. What is measured by a digital balance?
Answer:
Digital balance measures the weight of a body.

Question 21.
Under what conditions do the two hollow balls – one of aluminium and the other of iron, experience equal upthrust when placed in water?
Answer:
The two balls will experience equal upthrust in water when their volumes inside the water are equal.

Question 22.
The density of liquid B is greater than that of liquid A. A hydrometer is placed one by one in the two liquids. In which liquid will the hydrometer sink to the greater depth?
Answer:
The hydrometer will sink deeper in liquid A.

Short Answer Type Questions

Question 1.
During free – fall of an elevator, what is the weight of a body inside it? Give reasons for your answer.
Answer:
During free – fall of an elevator the apparent weight of the object becomes zero. This is because both the body and the elevator are in free fall, and have a downward acceleration of ‘g’. In this situation normal reaction on the body becomes zero. Since normal reaction is responsible for the sensation of weight in a body, the apparent weight of the body in this case becomes zero.

Question 2.
What is relative density?
Answer:
The relative density of a substance is the ratio of its density to that of the water at 4°C. Relative density Density of the substance Density of water at 4°C

Question 3.
Differentiate between ‘g’ and ‘G’ in tabular form.
Answer:

Acceleration due to gravity ‘g’	Universal gravitational constant ‘G’
(a) It is the acceleration acquired by a body due to earth’s gravitational pull on it.	(a) It is numerically equal to the force of attraction between two masses of 1kg each separated by a distance of 1 m.
(b) ‘g’ is not a universal constant. It is different at different places on the surface of the earth. Its value varies from one celestial body to another.	(b) ‘G’ is a universal constant and its value is same, i.e., 6.67 × 10-11 N m2 kg-2 everywhere in the universe.
(c) It is a vector quantity.	(c) It is a scalar quantity.

Question 4.
Why is it easier to swim in sea water than in river water?
Answer:
The density of sea water is high due to dissolved salts in it as compared to the density of river water. Hence, the buoyant force applied on the swimmer by the sea water is high which helps in floating and makes swimming simpler.

Question 5.
A ship made of iron does not sink but an iron nail sinks in water. Why?
Answer:
The iron nail sinks because of its high density and less buoyant force acting on it due to lesser surface area. Whereas, the surface area of a ship is greater and thus experiences a higher buoyant force. Due to this fact, a ship floats but an iron nail sinks.

Question 6.
A stone and feather are thrown from a tower. Both the objects should reach the ground at the same time but it does not happen. Give reasons.
Answer:
As per the motion of objects due to gravitational pull of the earth, both the bodies are acted upon by the same force of the earth but the stone will fall first and then the feather. Feather being lighter, experiences greater air resistance, so it will reach later.

Question 7.
The relative density of gold is 18.3. The density of water is 10³ kg/m³? What is the density of gold in S.I units?
Answer:
The relative density of gold is 18.3.
Relative density of gold = \(\frac{Density of gold}{Density of water}\)
That is, density of gold = Relative density of gold × Density of water = 18.3 × 10³ kg/m³ = 18300 kg/m³.

Question 8.
The acceleration due to gravity at the moon’s surface is 1.67 m/s². If the radius of the moon is 1.74 × 106m, calculate the mass of the moon.
Answer:
Here, G = 6.67 × 10^-11 Nm²/kg²
g = 1.67 m/s²
R= 1.74 × 10⁶ m.
Mass of the moon is
M = \(\frac{\mathrm{gR}^{2}}{\mathrm{G}}\) = \(\frac{1.67 \times\left(1.74 \times 10^{6}\right)^{2}}{6.67 \times 10^{-11}}\)
= 7.58 × 10²² kg.

Question 9.
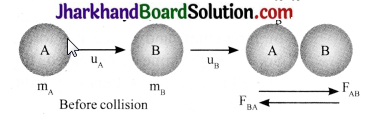
Derive the formula for the gravitational force acting between two objects.
Answer:
Let two objects A and B of masses ‘M’ and’m’, lie at a distance d from each other as shown in the figure.

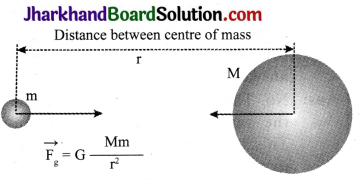
Let the force of attraction between two objects be F.
According to the universal law of gravitation, the force between two objects is directly proportional to the product of their masses.
F ∝ Mm … (1)
And the force between two objects is inversely proportional to the square of the distance between them,
F ∝ \(\frac{1}{\mathrm{~d}^{2}}\) …………(2)
F ∝ \(\frac{\mathrm{Mm}}{\mathrm{d}^{2}}\)
where G is universal gravitational constant

Question 10.
Find the value of ‘g’, acceleration due to gravity.
Answer:
g = \( \frac{\mathrm{GM}}{\mathrm{R}^{2}}\)
G = 6.67 × 10^-11 Nm²/kg² (constant)
M = 6 × 10²⁴ kg (mass of the earth)
= \( \frac{6.7 \times 10^{-11} \mathrm{Nm}^{2} / \mathrm{kg}^{2} \times 6 \times 10^{24} \mathrm{~kg}}{\left(6.4 \times 10^{6} \mathrm{~m}\right)^{2}}\)
= 9.8 m/s²

Question 11.
Camels can walk easily on desert sand but we are not comfortable walking on the sand. Why do you think so?
Answer:
The surface area of a camel’s feet is broad and large. Thus, the pressure exerted is low. However, when we walk, our legs sink because the pressure exerted by our body is not equally distributed but is directed towards the legs.

Question 12.
Define lactometer and hydrometer.
Answer:
Lactometer is a device used to find the purity of milk. Hydrometer is a device used to find the density of a liquid.

Question 13.
Two wood pieces of same size and mass are dipped in two beakers containing water and oil. One wood floats on water, but the other one sinks in oil. Why?
Answer:
The wood floats on water because the density of wood is lower than the density of water, and the other wood sinks in the oil because the density of wood is more than that of the oil.

Question 14.
What are fluids? Why is Archimedes’ principle applicable only for fluids? Give the applications of Archimedes’ principle.
Answer:
Liquids are the substances which can flow. Archimedes principle is based on the upward force exerted by fluids on any object immersed in them. Hence, it is applicable only for fluids. Applications of Archimedes’ principle are as follows:

1. It is used to design ships and submarines.
2. To determine the purity of milk using lactometers which are designed based on the Archimedes’ principle.
3. To make hydrometers which are used to determine the density of liquids.

Question 15.
The volume of 60g of a substance is 10cm². If the density of water is 1g/cm², find out whether the substance will float or sink in water.
Answer:
Mass = 60g
Volume = 10 cm³
The density of water is 1g/cm². As the density of the given substance is more than the density of water, the substance will get submerged in water.

Question 16.
What is meant by buoyancy?
Answer:
When a body is submerged in a fluid, the fluid exerts an upward force on the submerged body. This upward force is equal to the weight of the fluid displaced by the submerged body and is called the buoyant force. In other words, it is the force exerted by the fluid when an object is submerged in it.

Analysing & Evaluating Questions

Question 17.
Identical packets are dropped from two aeroplanes, one over the equator and the other over the north pole, both from the height h. Assuming that all conditions are identical, explain if those packets take same time to reach the surface of earth or not. Justify your answer.
Answer:
No. The two packets will take different time intervals to reach the earth’s surface. This is because the acceleration due to gravity (g) is greater at the poles than that at the equator. The packet will fall slowly at the equator than that at the north pole.

Question 18.
You must have seen two types of balances. A grocer has a balance with two platforms or pan, and a needle moving on a scale. Some junk dealers (kabadiwalas) may be using a spring balance to weigh used newspapers. Suppose, the two balances give the same measure for a given body on the earth. Now, you take the balances to the moon. Would the two balances give the same measure? Explain your answer.
Answer:
No. The two balances will not give the same measure on the moon. This is because, the spring balance measures the weight, and the grocer’s balance measures the mass. The weight of a body depends upon the acceleration due to gravity at the place of measurement. The acceleration due to gravity on the moon is not equal to that on the earth. Therefore, spring balance will give different readings relative to the grocer’s balance on the moon.

Long Answer Type Questions

Question 1.
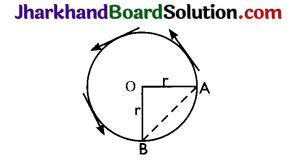
What is centripetal force? From where does the moon get the centripetal force required for its motion around the earth?
Answer:

1. Centripetal force When a body moves along a circular path with a uniform speed, its direction of motion changes at every point. The change in direction involves change in velocity or acceleration.
2. The force that provides this acceleration and keeps the body moving along the circular path, acts towards the centre. This force is called centripetal (centre seeking) force.
3. Therefore, a force which is required to make a body move along a circular path with uniform speed is called centripetal force. Centripetal force always acts along the radius and towards the centre of the circular path.
   
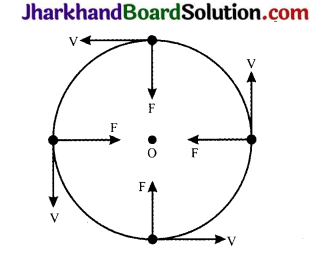
4. The figure given here shows that it is the centripetal force which continuously deflects a particle from its straight line path to make it move along a circle.
5. Example: The moon needs a centripetal force for its circular motion around the earth. This centripetal force is provided by the gravitational attraction exerted by the earth on the moon.

Question 2.
Show that the weight of an object on the moon is 1/6,h its weight on the earth. Given that the mass of the earth is 100 times the mass of the moon and its radius is 4 times that of the moon.
Answer:
Suppose, mass of the object = m
mass of the earth = M_e
mass of the moon = M_m
radius of the earth = R_e
radius of the moon = R_m
Then, M_e = 100 Mm and Re = 4Rm
Weight of an object of mass m on the earth is
We = the force with which the earth
attracts the object = G \(\frac{\mathrm{M}_{\mathrm{e}} \mathrm{m}}{\mathrm{R}_{\mathrm{e}}^{2}}\)
Weight of the object on the moon is
W_m = The force with which the moon
attracts the object = \( \mathrm{G} \frac{\mathrm{M}_{\mathrm{m}} \mathrm{m}}{\mathrm{R}_{\mathrm{m}}^{2}}\)
\(\frac{W_{m}}{W_{e}}\) = \(\frac{\left(G \frac{M_{m} m}{R_{m}^{2}}\right)}{\left(G \frac{M_{m} m}{R_{e}^{2}}\right)}\)
= \(\frac{M_{m}}{M_{e}} \times \frac{\left(R_{e}\right)^{2}}{\left(R_{m}\right)^{2}}\)
= \(\frac{\mathrm{M}_{\mathrm{m}}}{100 \mathrm{M}_{\mathrm{m}}} \times \frac{4 \mathrm{R}_{\mathrm{m}}^{2}}{\mathrm{R}_{\mathrm{m}}^{2}}\) = \(\frac{16}{100} \approx \frac{1}{6}\)
Thus, the weight of an object on the moon is about one – sixth of its weight on the earth.

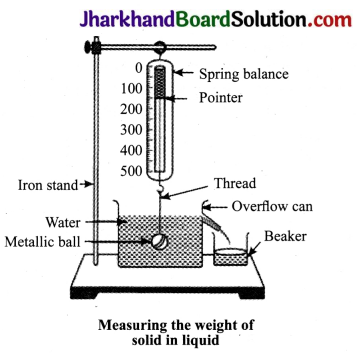
Question 3.
Briefly explain how can Archimedes’ principle be verified experimentally.
Answer:
According to Archimedes’ principle, when a body is immersed in a liquid, completely or partially, it loses its weight. The loss in weight is equal to the weight of liquid displaced by the body. The loss in weight of a body is due to the presence of upthrust which is equal to the weight of liquid displaced.
Thus, a Loss in weight = Weight of body in air – Weight of body immersed in water =
W W₁ = upthrust in water on the body = Weight of liquid displaced.

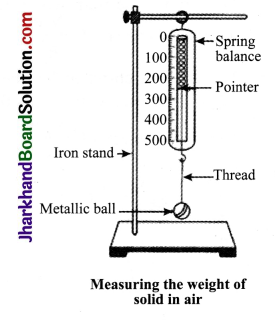
As shown in figure, measure the weight of a solid, say a metallic ball, in air using spring balance. Weigh the empty beakers using spring balance. Set the spring balance, overflow can with tap water and beaker. Now, allow the bob to immerse completely in water in overflow can. Note down the new position of the pointer of the spring balance.

This will give you the weight of the brass bob in tap water. It is found to be less than the weight of bob in air. Weigh the beaker containing displaced water which is collected from the overflow can while immersing the bob in it completely. It is observed that the loss in weight of the bob is equal to the weight of the water collected in the beaker, i.e., weight of the water displaced.

Question 4.
With the help of an activity, prove that the force acting on a lesser area exerts a larger pressure.
Answer:
Consider a block of wood kept on a tabletop. The mass of the wooden block is 5kg. Its dimensions are 30 cm × 20 cm × 10 cm.
Now, we have to find the pressure applied by the wooden block on the tabletop by keeping it vertically and horizontally.

The mass of the wooden block = 5kg
Weight of the wooden block applies a thrust on the tabletop.
Thrust = F = mg = 5kg × 9.8 m/s² = 49 N
(Case a) : When the wooden box is kept vertically with sides 20 cm × 10 cm,
Area of a side = length × breadth = 20 cm × 10 cm = 200 cm² = 0.02m²
Pressure = \(\frac{Thrust}{Area}\) = \(\frac{49 \mathrm{~N}}{0.02 \mathrm{~m}^{2}}\)
= 2450 N/m²

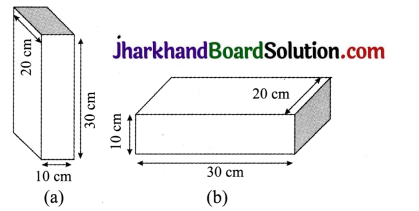
(Case b): When the block is kept horizontally with side 30 cm × 20 cm, Area = length × breadth = 30 cm × 20 cm = 600 cm² = 0.06 m²

Pressure = \(\frac{Thrust}{Area}\) = [/latex] \frac{49 \mathrm{~N}}{0.02 \mathrm{~m}^{2}}[/latex]
= 816.7 N/m²
The pressure exerted by the box in case (a) in more as compared to the pressure exerted in case (b). The area is reduced and the pressure exerted is more.
This shows that pressure ∝= \( \frac{1}{\text { Area }}\)
Hence, pressure will be larger if the area is reduced.

Analysing & Evaluating Questions

Question 5.
(a) A cube of side 5 cm is immersed in water and then in saturated salt solution. In which case it will experience a greater buoyant force. If each side of the cube is reduced to 4 cm and then immersed in water, what will be the effect on the buoyant force experienced by the cube as compared to the first case for water.

(b) A ball of weight 4 kg and density 4000 kg m³ is completely immersed in water of density 10³ kg m³. Find the force of buoyancy on it. (Given g = 10 ms^-2)
Answer:
(a) The cube will experience greater buoyant force in salt solution. When the side of the cube is reduced to 4 cm, it will experience lesser buoyant force as compared to the first case.
This is because the volume of water displaced by the smaller cube is lesser than that displaced by the original cube,

(b) Volume of water displaced by the ball = Volume of the ball = \(\frac{Mass}{Density}\)
= \(\frac{4 \mathrm{~kg}}{4000 \mathrm{~kg} \mathrm{~m}^{-3}}\) = 10^-3 m³
Weight of water displaced by the ball = Volume of water × Density × g
= 10^-3 m³ × 10³ kg m³ × 10 ms^-2 = 10 N
So, Buoyant force acting on the ball = Weight of water displaced = 10 N

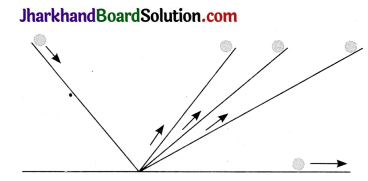
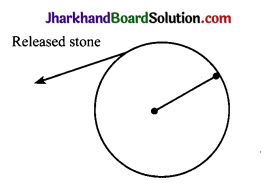
Activity 1

• Take a piece of thread.
• Tie a small stone at one end. Hold the other end of the thread and whirl it round.
• Note the motion of the stone.
• Now release the thread.
• Again, note the direction of motion of the stone.
• Record your observations and draw conclusion about the direction of the stone.
  
• If thread is released when stone is here, stone goes straight towards A not towards B Top view A stone describing a circular path

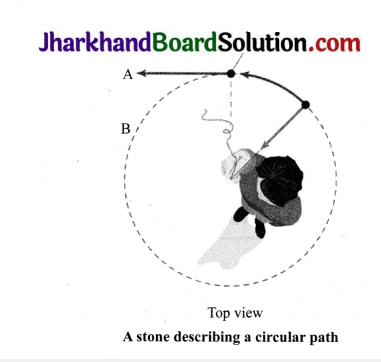
Observations

• The motion gets accelerated and stone moves in a circular path.
• When the thread is released, the stone makes a tangent to the circle and falls down.

ACTIVITY 2

• Take a sheet of paper and a stone. Drop them simultaneously from the first floor of a building.
• Observe whether both of them reach the ground simultaneously or not.

Observations

• We see that the paper reaches the ground a little later than the stone. This happens because of air resistance. The air offers resistance due to friction to the motion of the falling objects. The resistance offered by air to the paper is more than the resistance offered to the stone.
• If we do the experiment in a glass jar from which air has been sucked out, the paper and the stone would fall at the same rate.

ACTIVITY 3

• Take an empty plastic bottle. Close the mouth of the bottle with an airtight stopper. Put it in a bucket filled with water and observe.
• Push the bottle into the water and observe again. Try to push it further down.
• Now, release the bottle. Record your observations.
• Does the force due to the gravitational attraction of the earth act on this bottle? If so, why doesn’t the bottle stay immersed in water after it is released? How can you immerse the bottle in water?

Gravity

Observations

• The plastic bottle floats on water. When it is pushed deeper, the upward force on the bottle increases.
• When released, the bottle bounces back to the surface because the upthrust on the bottle was larger than the downward pull of gravity
• Take a piece of stone and tie it to one end of a rubber string or a spring balance.

ACTIVITY-4

• Take a piece of stone and tie it to one end of a rubber string or a spring balance.
  Thread
  
• Suspend the stone by holding the balance or the string as shown in the figure (A).
• Note the elongation of the string or the reading on the spring balance due to the weight of the stone.
• Now, slowly dip the stone in the water in a container as shown in Figure (B).
• Observe what happens to the elongation of the string or the reading on the balance.

Observations

• In fig. A, the elongation of the string is more.
• In fig. B, when the stone is dipped in water, the length of the string is reduced.
• The length of the string in case (B) decreases due to the upward force exerted by water on the stone called buoyant force.

Value Based Questions

Question 1.
Priya had a bad experience during the take off of the plane when she boarded it for the first time. Her friend assisted and helped her during the landing of plane. She told her to fasten the seat belt and involved her in gossip. Priya faced less problems while landing of the plane.
1. Why do we tie our seat belts in moving cars or during take off of the plane?
2. What is free fall?
3. What value of Priya’s friend is seen in this set?
Answer:

1. We tie our seat belts to remain intact on the seat so that our body does not fall forward.
2. When an object falls towards the earth under the gravitational force alone, we say that the object is in free fall.
3. Priya’s friend showed the value of concerned, sympathetic, responsible and caring friend.

Question 2.
A goldsmith measured the purity of gold by using a special measuring device. He told the customer that there was impurity present in gold ornament that he wanted to buy and was not 22 carat but 18 carat jewellery.
1. How can we find the purity of gold?
2. What is the unit of relative density?
3. What value of goldsmith is reflected in this act?
Answer:

1. The purity of gold can be obtained by knowing the density of the gold.
2. Relative density does not have any unit.
3. Goldsmith showed the value of honesty and trustworthiness.

JAC Class 9 Science Important Questions

Jharkhand Board JAC Class 10 Sanskrit Solutions Shemushi Chapter 9 सूक्तयः Textbook Exercise Questions and Answers.

JAC Board Class 10th Sanskrit Solutions Shemushi Chapter 9 सूक्तयः

JAC Class 10th Sanskrit सूक्तयः Textbook Questions and Answers

प्रश्न 1.
एकपदेन उत्तरं लिखत। (एक शब्द में उत्तर लिखिये)
(क) पिता पुत्राय बाल्ये किं यच्छति? (पिता पुत्र के लिये बचपन में क्या देता है?)
उत्तरम् :
विद्याधनम् (विद्यारूपी धन)।

(ख) विमूढधीः कीदृशीं वाचं परित्यजति? (मूढ़ कैसी वाणी त्यागता है?)
उत्तरम् :
धर्मप्रदाम् (धर्म प्रदान करने वाली)।

(ग) अस्मिन् लोके के एव चक्षुष्मन्तः प्रकीर्तिताः? (इस लोक में कैसे व्यक्ति आँखों वाले कहलाते हैं?)
उत्तरम् :
विद्वान्सः (विद्वान लोग)।

(घ) प्राणेभ्योऽपि कः रक्षणीयः? (प्राणों से भी कौन रक्षा करने योग्य है?)
उत्तरम् :
सदाचारः (सदाचरण)।

(ङ) आत्मनः श्रेयः इच्छन् नरः कीदृशं कर्म न कुर्यात्?
(अपना श्रेय चाहने वाले व्यक्ति को कैसा कर्म नहीं करना चाहिये?)
उत्तरम् :
परेभ्योऽहितम् (दूसरों के लिये अहित)।

(च) वाचि किं भवेत्? (वाणी कैसी हो?)
उत्तरम् :
धर्मप्रदा।

प्रश्न 2.
स्थूलपदानि आधृत्य प्रश्न निर्माणं कुरुत (मोटे पदों के आधार पर प्रश्न निर्माण कीजिये।)
यथा – विमूढधीः पक्वं फलं परित्यज्य अपक्वं फलं भुङ्क्ते?
कः पक्वं फलं परित्यज्य अपक्वं फलं भुङ्क्ते।
(क) संसारे विद्वांसः ज्ञानचक्षुभिः नेत्रवन्तः कथ्यन्ते।
उत्तरम् :
संसारे के ज्ञानचक्षुभिः नेत्रवन्तः कथ्यन्ते?

(ख) जनकेन सुताय शैशवे विद्याधनं दीयते।
उत्तरम् :
जनकेन कस्मै शैशवे विद्याधनं दीयते?

(ग) तत्वार्थस्य निर्णयः विवेकेन कर्तुं शक्यः।
उत्तरम् :
कस्य निर्णयः विवेकेन कर्तुं शक्यः?

(घ) धैर्यवान् लोके परिभवं न प्राप्नोति।
उत्तरम् :
धैर्यवान् कुत्र परिभवं न प्राप्नोति?

(ङ) आत्मकल्याणम् इच्छन् नरः परेषाम् अनिष्टं न कुर्यात्।
उत्तरम् :
आत्मकल्याणम् इच्छन् नरः केषाम् अनिष्टं न कुर्यात्।

प्रश्न 3.
पाठात् चित्वा अधोलिखितानां श्लोकानाम् अन्वयम् उचित पदं क्रमेण पूरयत –
(पाठ से छाँटकर उचित पद के क्रम से निम्नलिखित श्लोकों के अन्वयों की पूर्ति कीजिए-)
(क) पिता ………… बाल्ये महत् विद्याधनम् यच्छति अस्य पिता किं तपः तेपे इत्युक्तिः ……….. ।
उत्तरम् :
पिता पुत्राय बाल्ये महत् विद्याधनम् यच्छति अस्य पिता किं तपः तेपे इत्युक्तिः कृतज्ञता ।

(ख) येन ………… यत् प्रोक्तं तस्य तत्वार्थ निर्णयः येन कर्तुं ………… भवेत्, सः ……….. इति ……… ।
उत्तरम :
येन केनापि यत् प्रोक्तं तस्य तत्वार्थ निर्णयः येन कर्तुं शक्यो भवेत. सः विवेक इति ईरितः ।

(ग) य आत्मनः श्रेयः …………. सुखानि च इच्छति, परेभ्य अहितं ………… कदापि च न ………. ।
उत्तरम् :
यः आत्मनः श्रेयः प्रभूतानि सुखानि च इच्छति, परेभ्य अहितं कर्म कदापि च न कुर्यात् ।

प्रश्न 4.
अधोलिखितम् उदाहरणद्वयं पठित्वा अन्येषां प्रश्नानाम् उत्तराणि लिखत – (निम्न दो उदाहरणों को पढ़कर अन्य प्रश्नों के उत्तर लिखिए-)

प्रश्न 5.
मञ्जूषायाः तद्भावात्मकसूक्ती: विचित्य अधोलिखितकथनानां समक्षं लिखत – (मंजूषा से उस भाव की सूक्ति छाँटकर निम्न कथनों के सामने लिखिए-)
(क) विद्याधनं महत। …………….
(ख) आचारः प्रथमो धर्मः ……………
(ग) चित्ते वाचि च अवक्रता एव समत्वम्। ………………
[मञ्जूषा – आचरेण तु संयुक्तः सम्पूर्ण फल भाग्भवेत्। मनसि एक वचसि एक कर्मणि एकं महात्मनाम्। विद्याधनं सर्वधन प्रधानम्। सं वो मनांसि जानताम्। विद्याधनं श्रेष्ठं तन्मूलमितरद्धनम्। आचारप्रभवो धर्मः सन्तश्चाचारलक्षाः]
उत्तर :
(क) विद्याधनं महत।
(i) विद्याधनं सर्वधन प्रधानम्।
(ii) विद्याधनं श्रेष्ठं तन्मूलमितरद्धनम्।

(ख) आचारः प्रथमो धर्मः
(i) आचरेण तु संयुक्तः सम्पूर्ण फल भाग्भवेत्।
(ii) आचार प्रभवो धर्मः सन्तश्चाचारलक्षणाः

(ग) चित्ते वाचि च अवक्रता एव समत्वम्।
(i) मनसि एक वचसि एक कर्मणि एकं महात्मनाम्
(ii) सं वो मनांसि जानताम्।

प्रश्न 6.
(अ) अधोलिखितानां शब्दानां पुरतः उचितं विलोम शब्द कोष्ठकात् चित्वा लिखत
(निम्नलिखित शब्दों के सामने उचित विलोम शब्द कोष्ठक से छाँटकर लिखिए-)
(क) पक्वः ……………. । (परिपक्व, अपक्व, क्वथितः)
(ख) विमूढधीः …………… । (सुधी, निधिः मन्दधी:)
(ग) कातरः ……………. । (अकरुणः, अधीरः, अकातरः)
(घ) कृतज्ञता ……………. (कृपणता, कृतघ्नता, कातरता)
(ङ) आलस्यम् ……………. । (उद्विग्नता, विलासिता, उद्योगः)
(च) परुषा ……………. । (पौरुषी, कोमला, कठोरा)
उत्तरम् :
(क) अपक्व
(ख) सुधी
(ग) अकातरः
(घ) कृतघ्नता
(ङ) उद्योगः
(च) कोमला।

(आ) अधोलिखितानां शब्दानां त्रयः समानार्थकाः शब्दाः मञ्जूषायाः चित्वा लिखन्ताम्।
(निम्न शब्दों के तीन समानार्थक शब्द मंजूषा से छाँटकर लिखिए-)

उत्तरम् :
(क) प्रभूतम् – भूरि, विपुलम्, बहु
(ख) श्रेयः – शुभम्, शिवम्, कल्याणम्
(ग) चित्तम् – मनः, मानसम्, चेतः
(घ) सभा – परिषद्, संसद, सभा
(ङ) चक्षुष – लोचनम्, नेत्रम्, नयनम्
(च) मुखम् – ‘ आननम्, वदनम्, वक्त्रम्

प्रश्न 7.
अधस्तात् समास विग्रहाः दीयन्ते तेषां समस्त पदानि पाठाधारण दीयन्ताम्।
(नीचे समास विग्रह दिए हैं, उनके समस्त पद पाठ के आधार पर दीजिए-)
उत्तरम् :

JAC Class 10th Sanskrit सूक्तयः Important Questions and Answers

शब्दार्थ चयनम् –

अधोलिखित वाक्येषु रेखांकित पदानां प्रसङ्गानुकूलम् उचितार्थ चित्वा लिखत –

प्रश्न 1.
पिता यच्छति पुत्राय बाल्ये विद्याधनं महत्।
(अ) दीप्ति
(ब) अशनम्
(स) महत्
(द) ददाति
उत्तरम् :
(द) ददाति

प्रश्न 2.
पिताऽस्य किं तपस्तेपे इत्युक्तिस्तत्कृतज्ञता –
(अ) विद्या
(ब) तस्य
(ब) तस्य
(स) जनक
(द) उक्तिः
उत्तरम् :
(स) जनक

प्रश्न 3.
अवकता यथा चित्ते तथा वाचि भवेद् यदि –
(अ) वक्रता
(ब) ऋजुता
(स) सुचि
(द) वृत्त
उत्तरम् :
(ब) ऋजुता

प्रश्न 4.
तदेवाहुः महात्मानः समत्वमिति तथ्यतः –
(अ) यथार्थरूपेण
(ब) यदात्मनः
(स)
(द) इत्याहुः
उत्तरम् :
(अ) यथार्थरूपेण

प्रश्न 5.
त्यक्त्वा धर्मप्रदां वाचं परुषां योऽभ्युदीरयेत्।
(अ) परित्यज्य
(ब) विमूढ़धीः
(स) तथ्यतः
(द) अपक्वं
उत्तरम् :
(अ) परित्यज्य

प्रश्न 6.
विद्वांस एव लोकेऽस्मिन् चक्षुष्मन्तः प्रकीर्तिताः –
(अ) जिह्वः
(ब) नेत्रवंतः
(स) अन्येषाम्
(द) चक्षुर्नामनी
उत्तरम् :
(ब) नेत्रवंतः

प्रश्न 7.
यत् प्रोक्तं येन केनापि तस्य तत्त्वार्थनिर्णयः।
(अ) तेन
(ब) परिणामम्
(स) परिणति
(द) विवेक
उत्तरम् :
(स) परिणति

प्रश्न 8.
वाक्पटुधैर्यवान् मंत्री सभायामप्यकातर:
(अ) प्रतिभाशालिनः
(ब) अकातरः
(स) नपरिभूयते
(द) धृतिमान्
उत्तरम् :
(द) धृतिमान्

प्रश्न 9.
य इच्छत्यात्मनः श्रेयः प्रभूतानि सुखानि च –
(अ) कल्याणम्
(ब) इच्छति
(स) परेभ्य
(द) कदापि
उत्तरम् :
(अ) कल्याणम्

प्रश्न 10.
आचारः प्रथमो धर्मः इत्येतद् विदुषां वचः –
(अ) तस्मात्
(ब) प्रज्ञानां
(स) प्राणेभ्यः
(घ) सदाचारम्
उत्तरम् :
(ब) प्रज्ञानां

संस्कृतमाध्यमेन प्रश्नोत्तराणि –

एकपदेन उत्तरत (एक शब्द में उत्तर दीजिए)

प्रश्न 1.
पिता पुत्राय किं यच्छति?
(पिता पुत्र के लिये क्या देता है?
उत्तरम् :
विद्याधनम् (विद्यारूपी धन)।

प्रश्न 2.
पिता कस्मै विद्याधनं यच्छति?
(पिता किसके लिये विद्या धन देता है?)
उत्तरम् :
पुत्राय (पुत्र के लिये)।

प्रश्न 3.
यथा अवक्रता चित्ते तथैव कुत्रापि भवेत्।
(जैसी सरलता चित्त में होती है वैसी ही कहाँ होनी चाहिए?)
उत्तरम् :
वाचि (वाणी में)।

प्रश्न 4.
चित्ते वाचि च किं गुणः भवेत्?
(चित्त और वाणी में क्या गुण होना चाहिये?)
उत्तरम् :
अवक्रता (सरलता)।

प्रश्न 5.
बुद्धिमन्तः पुरुषाः कीदृशीं वाणी वदन्ति?
(बुद्धिमान लोग कैसी वाणी बोलते हैं?)
उत्तरम् :
धर्मप्रदाम् (धर्मयुक्त)।

प्रश्न 6.
कीदृशीं वाचं न वदेत्?
(कैसी वाणी नहीं बोलनी चाहिये।)
उत्तरम् :
परुषाम् (कठोर)।

प्रश्न 7.
अस्मिन् लोके चक्षुमन्तः के?
(इस लोक में नेत्रों वाला कौन हैं?)
उत्तरम् :
विद्वांस (विद्वान लोग)।

प्रश्न 8.
विद्वांसः एव अस्मिन् लोके के प्रकीर्दिना?
(विद्वान लोग ही इस लोक में क्या कहलाता है?)
उत्तरम् :
चक्षुष्मन्तः (आँखों वाले)।

प्रश्न 9.
येन तत्वार्थ निर्णयः कर्तुं शक्यो भवेत् सः किं कथ्यते?
(जिसके द्वारा यथार्थ निर्णय किया जाना समर्थ है, वह क्या कहलाता है?)
उत्तरम् :
विवेकः।

प्रश्न 10.
विवेकः कीदृशः निर्णयं कर्तुं शक्यो भवेत?
(विवेक कैसा निर्णय करने में समर्थ होता है?)
उत्तरम् :
तत्वार्थ (यथार्थ)।

प्रश्न 11.
वाक्पटुः मंत्री कैः न परिभूयते?
(वाक्पटु मंत्री किनसे पराजित नहीं होता?)
उत्तरम् :
परैः (शत्रुओं से)।

प्रश्न 12.
मन्त्रिपदस्थस्य एकं गुणं लिखत।
(मन्त्रि पद पर स्थित व्यक्ति का एक गुण लिखिये।)
उत्तरम् :
वाक्पटुता।

प्रश्न 13.
यः आत्मनः श्रेयः इच्छति तेन परेभ्यः किं न करणीय?
(अपना भला चाहता है, उसे अन्य व्यक्तियों के साथ क्या नहीं करना चाहिये?)
उत्तरम् :
अहितम् (अहित)।

प्रश्न 14.
अहितं कर्म केभ्यः न कुर्यात्।
(अहित कर्म किसके लिये नहीं करना चाहिये।)
उत्तरम् :
परेभ्यः (अन्यों के लिये)।

प्रश्न 15.
कः प्रथमः धर्मः? (प्रथम धर्म क्या है?)
उत्तरम् :
आचारः।

प्रश्न 16.
विशेषतः प्राणेभ्योऽपि किं रक्षेत्?
(विशेषतः प्राणों से भी किसकी रक्षा करनी चाहिये?)
उत्तरम् :
सदाचारम्।

पूर्णवाक्येन उत्तरत (पूरे वाक्य में उत्तर दीजिए)

प्रश्न 17.
के प्रति कृतज्ञो भवेत् ?
(किसके प्रति कृतज्ञ होना चाहिये?)
उत्तरम् :
पितरं प्रति। (पिता के प्रति।)

प्रश्न 18.
समत्वं किमुच्यते? (समत्व क्या कहलाता है?)
उत्तरम् :
मनसि वाचि च अवक्रता समत्वमिति कथ्यते।
(मन और वाणी में सरलता भी समत्व कहलाता है।)

प्रश्न 19.
परुषां वाचं त्यक्त्वा कीदृशी वाणी वदेत्?
(कठोर वाणी को त्यागकर कैसी वाणी बोलनी चाहिये?
उत्तरम् :
परुषां वाचं त्यक्त्वा धर्मप्रदां वाचं वदेत्।
(कठोर वचनों को त्यागकर धर्मप्रद वाणी बोलनी चाहिए।)

प्रश्न 20.
चक्षुर्नामनी के मते ? (चक्षुर्नामनी क्या माने जाते हैं?)
उत्तरम् :
वदने ये नेत्रे ते तु चक्षुर्नामनी मते।
(चेहरे पर जो नेत्र हैं वे तो नाम मात्र की आँखें हैं।)

प्रश्न 21.
मन्त्री कैः गुणैरुपेतो भवेत्।
(मन्त्री किन गुणों से युक्त होना चाहिये?)
उत्तरम् :
मन्त्री वाक्पटुता, धैर्य सभायां वीरतादिभिः गुणैरुपेतो भवेत् ।
(मंत्री वाक्पटुता, धैर्य, सभा में वीरता आदि गुणों से युक्त होना चाहिए।)

प्रश्न 22.
‘कथमपि’ इति पदस्य हेतोः किं पदं प्रयुक्तम्?
(‘कथमपि’ पद के लिये किस पद का प्रयोग किया है?)
उत्तरम् :
केनापि प्रकारेण (कैसे भी या किसी प्रकार से)।

प्रश्न 23.
मानवः संसारे किं लब्धम् इच्छति?
(मानव संसार में क्या प्राप्त करना चाहता है?)
उत्तरम् :
मानवः संसारे श्रेयः सुखानि च लब्धुम् इच्छति।
(मानव संसार में कल्याण और सुख प्राप्त करना चाहता है?)

प्रश्न 24.
कस्मात् रक्षेत् सदाचारम्? (किसलिये सदाचार की रक्षा करनी चाहिये?)
उत्तरम् :
आचार: प्रथमः धर्मः तस्मात् सदाचारं रक्षेत्।
(आचार पहला धर्म है, अतः सदाचार की रक्षा करनी चाहिये।)

प्रश्न 25.
का उक्तिः कृतज्ञता? (क्या कथन कृतज्ञता है?)
उत्तरम् :
‘पिताऽस्य किं तपस्तेपे’ इति उक्तिः कृतज्ञता।
(पिता ने इसके लिये कितना तप किया, यह उक्ति ही कृतज्ञता है)

प्रश्न 26.
चित्ते वाचि च अवक्रता कस्य समत्वं भवति?
(चित्त और वाणी में सरलता किसका समत्व होता है?)
उत्तरम् :
तत्महात्मनः तथ्यतः समत्वमिति कथ्यते।

प्रश्न 27.
ये परुषां वाणीं वदन्ति ते कीदृशः मनाः ?
(जो कठोर वाणी बोलते हैं वे कैसे लोग हैं?)
उत्तरम् :
ये जनाः परुषां वाणी वदन्ति ते मूढधियः भवन्ति।
(जो छ । कठोर वाणी बोलते हैं वे विमूढ़मति होते हैं।)

प्रश्न 28.
अन्येषां ये वदने नेत्रे ते के मते ?
(अन्य लोगों के चेहरे पर जो आँखें हैं वह क्या माने गये हैं?)
उत्तरम् :
अन्येषां ये वदने नेत्रे ते तु चक्षुर्नामनी मते।
(दूसरों के चेहरे पर जो नेत्र हैं वे तो मात्र आँखें ही मानी गई हैं।)

प्रश्न 29.
मंत्री कीदृशः भवेत्? (मन्त्री कैसा होना चाहिये?)
उत्तरम् :
मन्त्री: वाक्पटुः धैर्यवान् सभायामपि अकातरः भवेत्।
(मन्त्री वाक्पटु, धैर्यवान् और सभा में भी वीर या साहसी होना चाहिये)।

प्रश्न 30.
यः अन्येभ्योः अहितं न करोति सः किं लभते?
(दूसरों के लिये जो अहित नहीं करता वह क्या पाता है?)
उत्तरम् :
यो अन्येभ्यः अहितं न करोति स: श्रेयः प्रभूतानि सुखानि लभते।
(जो अन्यों के लिये अहित नहीं करता, वह श्रेय और बहुत से सुख प्राप्त करता है।)

प्रश्न 31.
आचारः प्रथमः धर्मः इति केषां वचः?
(आचार प्रथम धर्म है, यह किसका वचन है?)
उत्तरम् :
आचार: प्रथमः धर्मः इति विदुषां वचः।
(आचार प्रमुख धर्म है, यह विद्वानों का वचन है।)

अन्वय-लेखनम् –

अधोलिखितश्लोकस्यान्वयमाश्रित्य रिक्तस्थानानि मञ्जूषातः समुचितपदानि चित्वा पूरयत।
(नीचे लिखे श्लोक के अन्वय के आधार पर रिक्तस्थानों की पूर्ति मंजूषा से उचित पद चुनकर कीजिए ।)

1. पिता यच्छति पुत्राय ………………………………. इत्युक्तिस्तत्कृतज्ञता॥
मञ्जूषा – पिता, तत्, तपः, विद्याधनं।
बाल्ये (i)…… पुत्राय महत् (ii)……यच्छति। अस्य पिता किं (iii)…… तेपे इति उक्तिः (iv)……कृतज्ञता।
उत्तरम् :
(i) पिता (ii) विद्याधनं (iii) तपः (iv) तत् ।

2. अवकता यथा चित्ते ……………. समत्वमिति तथ्यतः।
मञ्जूषा – महात्मनः, अवक्रता, इति, वाचि।।
यथा (i)…… चित्ते तथा यदि (ii)…… भवेत् तदैव (अवक्रता) (iii)…… तथ्यतः समत्वम् (iv)……आहुः। उत्तरम् : (i) अवक्रता (ii) वाचि (iii) महात्मनः (iv) इति।

3. त्यक्त्वा धर्मप्रदां …………………………………… विमूढधीः।
मञ्जूषा – त्यक्त्वा, विमूढधीः, फलं, फलम्।
यो (i)…… धर्मप्रदां वाचं (i)…… परुषामभ्युदीरयेत सः पक्वं (iii)…… परित्यज्यं अपक्वं (iii)……भुङ्क्ते।
उत्तरम् :
(i) विमूढधी: (ii) त्यक्त्वा (iii) फलम् (iv) फलं।

4. विद्वास एवं ……………………………………………………….. चक्षुर्नामनी मते ॥
मञ्जूषा – चक्षुर्नामनी, लोके, अन्येषां, चक्षुष्मन्तः।
अस्मिन् (i)…… विद्वान्सः एव (ii)…… प्रकीर्तिताः। (iii)……वदने तु ये (iv)….. मते।
उत्तरम् :
(i) लोके (ii) चक्षुष्मन्तः (iii) अन्येषां (iv) चक्षुर्नामनी।

5. यत् प्रोक्तं येन केनापि ………………………….. विवेक इतीरितः॥
मञ्जूषा – शक्यो, तत्वार्थ, विवेक, केनापि। येन (i) ……… यत् प्रोक्तम् तस्य (ii) ……….. निर्णयः येन कर्तुं (iii) ………. भवेत् सः (iii)…… इति ईरितः।
उत्तरम् :
(i) केनापि (ii) तत्वार्थ (iii) शक्यो (iv) विवेक।

6. वाक्पटुधैर्यवान् मंत्री ……………………………….. परैर्न परिभूयते।।
मञ्जूषा – धैर्यवान्, परैः, केनापि, अकातरः।
वाक्पटुः (i) …………. सभायाम् अपि (ii) ………… सः (iii)…… प्रकारेण (iv)…… न परिभूयते।
उत्तरम् :
(i) धैर्यवान् (ii) अकातरः (iii) केनापि (iv) परैः।

7. य इच्छत्यात्मनः श्रेयः …………………….. परेभ्यः कदापि च ।
मणूणा – परेभ्यः, सुखानि, कलापि, आत्मना यः (i)…… श्रेयः प्रभूतानि (ii)…… च इच्छति, (iii)…… अहितं कर्म (iv)…… च न कुर्यात्।
उत्तरम् :
(i) आत्मनः (ii) सुखानि (iii) परेभ्यः (iv) कदापि।

8. आचारः प्रथमो धर्मः …………………… प्राणेभ्योऽपि विशेषतः।।
आचारः (i) ……….. धर्मः इति एतद् (i) ………. वचः तस्मात् (iii)…… सदाचारं प्राणेभ्योऽपि (iii) ………..।
उत्तरम् :
(i) प्रथमो (ii) विदुषां (iii) विशेषतः (iv) रक्षेत्।

प्रश्ननिर्माणम् –

अधोलिखित वाक्येषु स्थूलपदमाधृत्य प्रश्ननिर्माणं कुरुत –

1. पितापुत्राय बाल्ये विद्याधनं यच्छति। (पिता पुत्र को बचपन में विद्या ही धन देता है।)
2. अवक्रता यथा चित्ते तथा वाचि भवेत् । (सरलता जैसी चित्त में होती है, वैसी वाणी में होनी चाहिए।)
3. त्यक्त्वा धर्मप्रदां वाचं परुषां चः वदति। (धर्मनिष्ठ विद्या को त्याग कर जो कठोर वाणी बोलता है।)
4. विमूढधीः भुङ्क्तेऽपक्वं फलम्। (मूर्ख व्यक्ति कच्चा फल खाते हैं।)
5. विद्वान एव लोकेऽस्मिन् चक्षुष्मन्तः प्रकीर्तितः। (विद्वान ही संसार में नेत्रवान कहलाते हैं।)
6. वाक्पटुः मन्त्री परैर्नः परिभूयते। (वाक्पटु मंत्री शत्रुओं द्वारा अपमानित नहीं होता है।) ।
7. यः इच्छति प्रभूतानि सुखानि न कुर्यात् अहितं परेभ्यः। (जो बहुत से सुख चाहता है, उसे दूसरों के लिये अहित कार्य नहीं करने चाहिये।)
8. आचारः परमोधर्मः। (आचार परम धर्म है।)
9. तस्मात् रक्षेत् सदाचारं प्राणेभ्योऽपि विशेषतः।
10. तदेवाहुः महात्मन् समत्वमिति तथ्यतः। (वही महापुरुष का यथार्थ समत्व कहलाता है।)
11. पिता पुत्राय विद्याधनं ददाति।
12. तदेवाहुः महात्मानः समत्वमिति तथ्यतः।
13. भुङ्क्तेऽपक्वं विमूढधीः।
14. विद्वांस एव लोकेऽस्मिन् चक्षुष्मन्तः।
15. स केनापि प्रकारेण परैः न परिभूयते।
उत्तराणि :
1. पिता पुत्राय कदा विद्याधने यच्छति?
2. अवक्रता यथा चित्ते तथा कस्याम् भवेत् ?
3. कीदृशीं वाणी त्यक्त्वा यः पुरुषां य वदति?
4. कः भुङ्क्तेऽपक्वं फलम्।
5. के एव लोकेऽस्मिन् चक्षुष्मन्तः प्रकीर्तिता?
6. कीदृशः मन्त्री परैः नः परिभूयते?
7. यः इच्छति प्रभूतानि सुखानि किं न कुर्यात् परेभ्यः?
8. कः परमोधर्म:?
9. तस्मात् प्राणेभ्योऽपि विशेषतः किम् रक्षेत्?
10. तदेवाहुः कस्य समत्वमिति तथ्यत:?
11. पिता कस्मै विद्याधनं ददाति?
12. तदेवाहुः कान् समत्वमिति तथ्यतः?
13. भुङ्क्तेऽपक्वं कः?
14. के एव लोकेऽस्मिन् चक्षुष्मन्तः?
15. स केनापि प्रकारेण कैः न परिभूयते?

भावार्थ-लेखनम –

अधोलिखित पद्यांश संस्कृते भावार्थं लिखत –

1. पिता यच्छति पुत्राय …………………………… इत्युक्तिस्तत्कृतज्ञता॥

संस्कृत व्याख्या: – बाल्यकाले जनक : स्व-आत्मजाय श्रेष्ठं ज्ञान धनं ददाति। अमुष्य जनकः किं तपस्यां कृतवान् इति कथनं एव तस्योपकारः कृतज्ञता वा।

2. अवक्रता यथा चित्ते ………………………. समत्वमिति तथ्यतः।।

संस्कृत व्याख्या: – येन प्रकारेण ऋजुता मनसि भवति तेनैव प्रकारेण चेत् वाच्याम् स्यात् तदैव ऋजुता महापुरुषस्य यथार्थरूपेण समता इति उच्यते।

3. त्यक्त्वा धर्मप्रदां वाचं ………………… भुङ्क्तेऽपक्वं विमूढधीः।।

संस्कृत व्याख्या: – यः मूर्खः धार्मिकभावोत्पादिकां वाणी परित्यज्य कटुकां वाणी कठोर वचनानि वा वदति असौ तु पक्वं फलं त्यक्त्वा अपक्वं फलमेव परिणामेव भुनक्ति।

4. विद्वांस एव लोकेऽस्मिन् …………………………. चक्षुर्नामनी मते।।

संस्कृत व्याख्या:- एतस्मिन् संसारे, समाजे वा प्राज्ञा एव यथार्थतः नेत्रवन्तः कथ्यन्ते, अपरेषाम् आनने तु नाममात्रस्यैव नेत्रे स्त: मन्यते वा।

5. यत् प्रोक्तं येन ………………….. विवेक इतीरितः॥

संस्कृत व्याख्याः – येन केनापि यत्किञ्चित् अकथत् अमुष्य समता यथार्थतः परिणति कर्तुं निर्णय वा कर्तुम् समर्थः यः च कर्तुं शक्नोति असावेव विचारशीलता विवेकः वा कथ्यते।

6. वाक्पटुधैर्यवान् मंत्री …………………………………….. परैर्न परिभूयते।।

संस्कृत व्याख्याः – वाण्यां भाषणे वा चतुरः यश्च धृतिमान्, संसदि चापि यः साहसी वीरः प्रगल्भो वा असौ पुरुषः कथमपि अरिभिः नाभिभूयते अर्थात् सः कदापि न पराजयते।

7. य इच्छत्यात्मनः श्रेयः ……………………….. परेभ्यः कदापि च ।।

संस्कृत व्याख्या: – यः (मानवः) स्वकीयं कल्याणम् बहूनि सुखानि च ईहते सः अन्येभ्यः अहित कार्य न कुर्यात्। अर्थात् तेन अन्येभ्यः अहित कार्य कदापि न कर्त्तव्यम्।

8. आचारः प्रथमो धर्मः ……………………. प्राणेभ्योऽपि विशेषतः।।

संस्कृत व्याख्या: – सदाचारः सदाचरणं वा मानवस्य प्रमुखो धर्म कर्त्तव्य वा। एवं प्राज्ञानां वचनानि। अनेन प्रकारेण सद्व्यवहारं सदाचरणं वा प्राणपणेनापि रक्षणीयम् भवति।

सूक्तयः Summary and Translation in Hindi

पाठ-परिचय – यह पाठ मूलत: तमिल भाषा के ‘तिरक्कुरल’ नामक ग्रंथ से लिया गया है। यह ग्रन्थ तमिल भाषा का वेद कहलाता है। इसके रचनाकार ‘तिरुवल्लुवर’ हैं। प्रथम शताब्दी इसका रचना काल स्वीकार किया गया है। धर्म, अर्थ और काम का प्रतिपाद्य है यह ग्रंथ। यह तीन भागों में विभक्त है। तिरु शब्द श्रीवाचक है अर्थात् तिरु का अर्थ है ‘श्री’। अतः तिरक्कुरल शब्द का अभिप्राय होता है- श्री से युक्त वाणी। इस ग्रंथ में मानवों के लिये जीवनोपयोपी सत्य को सरल और सुबोधगम्य पद्यों द्वारा प्रतिपादित किया गया है।

मूलपाठः, अन्वयः,शब्दार्थाः, सप्रसंग हिन्दी-अनुवादः

1. पिता यच्छति पुत्राय बाल्ये विद्याधनं महत्।
पिताऽस्य किं तपस्तेपे इत्युक्तिस्तत्कृतज्ञता॥1॥

अन्वयः – बाल्ये पिता पुत्राय महत् विद्याधनं यच्छति। अस्य पिता किं तपः तेपे इति उक्तिः तत् कृतज्ञता।

शब्दार्थाः – बाल्ये = बाल्यकाले (बचपन में). पिता = जनकः (पिता ने), पत्राय = आत्मजस्य कते (पत्र के लिये), महत् = श्रेष्ठं (उत्तम, महान), विद्याधनं = ज्ञान रूपं धनं (विद्या धन), यच्छति = ददाति (देता है), तस्य = अमुष्य (उसका), पिता = जनक (पिता), किं तपः तेपे = कि तपस्यां कृतवान (क्या तपस्या की), इति उक्तिः = एवं कथनं (ऐसा कहना), तत् कृतज्ञता = तस्य उपकृति (उसका अहसान है)।

सन्दर्भ-प्रसङ्गश्च – यह श्लोक हमारी ‘शेमुषी’ पाठ्यपुस्तक के ‘सूक्तयः’ पाठ से लिया गया है। इस श्लोक में कवि कहता है कि पिता ही बाल्यकाल में शिक्षक होता है, जिसका मानव को कृतज्ञ होना चाहिये।

हिन्दी-अनुवादः – बाल्यकाल में पिता ही पुत्र को महान् विद्यारूपी धन प्रदान करता है। पिता इसके लिये कितनी तपस्या करता है। यह उसका अहसान है।

2. अवक्रता यथा चित्ते तथा वाचि भवेद् यदि।
तदेवाहुः महात्मानः समत्वमिति तथ्यतः।।2।।

अन्वयः – यथा अवक्रता चित्ते तथा यदि वाचि भवेत् तदैव (अवक्रता) महात्मनः तथ्यतः समत्वम् इति आहुः।

शब्दार्थाः – यथा = येन प्रकारेण (जिस प्रकार), अवकता = ऋजुता, सरलता (सीधापन), चित्ते = यदि (यदि), वाधि = वाच्याम, वाण्याम् अपि (वाणी में भी), भवेत् – स्यात् (हो), अवकता – सरलता, ऋजुता (सीधापन), यदात्मनः = महापुरुषस्य (महात्मा को), तथ्यता – यथार्थरूपेण (वास्तव में), समत्वम् – समता (समानता), इत्याहुः = इत्युच्यते (इस प्रकार कहलाती है।)

सन्दर्भः प्रसङ्गश्च – यह पद्य हमारी ‘शेमुषी’ पाठ्यपुस्तक के सूक्तयः पाठ से लिया गया है। यह पाठ मूलतः तिरुवल्लुवर कृत ‘तिरक्कुरल’ के संस्कृत अनुवाद से लिया गया है। इस पद्य में अवक्रता और समता भाव के महत्व को प्रतिपादित किया है।

हिन्दी-अनुवादः – सरलता जैसे मन में हो वैसी ही वाणी में भी होनी चाहिये। वास्तव में यही महापुरुष या महान् आत्मा का समत्व कहलाता है।

3. त्यक्त्वा धर्मप्रदां वाचं परुषां योऽभ्युदीरयेत्।
परित्यज्य फलं पक्वं भुङ्क्तेऽपक्वं विमूढधीः।।3।।

अन्वयः – यो विमूढ़धीः धर्मप्रदां वाचं त्यक्त्वा परुषामभ्युदीरयेत सः पक्वं फलम् परित्यज्यं अपक्वं फलं भुङ्क्ते।

शब्दार्थाः – यः = यो जनः (जो व्यक्ति), विमूढधीः = मूर्खः, बुद्धिहीनः (मूर्ख या बुद्धिहीन, अज्ञानी), धर्मप्रदां = धार्मिकभावोत्पादिकां (धर्म भाव पैदा करने वाली), वाचं = वाणीम् (वाणी को), त्यक्त्वा = परित्यज्य (त्यागकर), परुषाम् = कटुकं, कर्कशां (कठोर), अभ्युदीरयेत = प्रयुज्यते, वदति (बोलता है, प्रयोग करता है) सः = असौ (वह), पक्वं फलं = (पके फल से), परित्यज्य = त्यक्त्वा (त्यागकर) अपक्वं = न पक्वं (कच्चे) फलम् = परिणामं (फल को) भुक्ते = खादति। –

सन्दर्भ-प्रसङ्गश्च – यह श्लोक हमारी ‘शेमुषी’ पाठ्यपुस्तक के सूक्तयः पाठ से लिया गया है। यह पाठ मूलतः तिरुवल्लुवर कृत ‘तिरक्कुरल’ इस काव्य के संस्कृत अनुवाद से संकलित है। इस श्लोक में धर्मप्रदा वाणी का महत्व प्रतिपादित किया गया है।

हिन्दी-अनुवादः – धर्म प्रदान करने वाली वाणी को त्याग कर जो कठोर वाणी बोलता है वह मूढ़मति पके हुए फल . को त्याग कर कच्चे फल को ही खाता है।

4. विद्वांस एव लोकेऽस्मिन् चक्षुष्मन्तः प्रकीर्तिताः।
अन्येषां वदने ये तु ते चक्षुर्नामनी मते।। 4।।

अन्वयः – अस्मिन् लोके विद्वान्सः एव चक्षुष्मन्तः प्रकीर्तिताः। अन्येषां वदने तु ये चक्षुर्नामनी मते ।

शब्दार्थाः – अस्मिन् लोके = एतस्मिन् संसारे, समाजे वा (संसार या समाज में), विद्वांस एव = प्राज्ञः एव (विद्वान् ही) चक्षषमन्तः = नेत्रवंतः (नेत्रों वाले), प्रकीर्तिता = मताः, कथ्यन्ते (कहलाते हैं) अन्येषाम् = अपरेषां (दूसरे) मुखे = आनने (मुँह, पर तो), चक्षुर्नामनी = नाममात्र चक्षु (नाममात्र की आँख), मते = मन्वते (मानी जाती है)।

सन्दर्भ-प्रसङ्गश्च – यह श्लोक हमारी ‘शेमुषी’ पाठ्यपुस्तक के सूक्तयः पाठ से लिया गया है। यह पाठ मूलतः तमिल कवि तिरुवल्लुवर कृत ‘तिरक्कुरल’ के संस्कृत अनुवाद से संकलित है। इस पद्य में कवि ने विद्वानों को ही नेत्रों वाला बताया गया है।

हिन्दी-अनुवादः – विद्वान लोगों को ही इस लोक में आँखों वाला कहा गया है। अन्य के मुख (आनन) पर जो आँखें होती हैं, वे तो नाम की आँखें मानी गई हैं।

5. यत् प्रोक्तं येन केनापि तस्य तत्त्वार्थनिर्णयः।
कर्तुं शक्यो भवेद्येन स विवेक इतीरितः॥5॥

अन्वयः – येन केनापि यत् प्रोक्तम् तस्य तत्वार्थ निर्णयः येन कर्तुं शक्यो भवेत् सः विवेक इति ईरितः।।

शब्दार्थाः – येन केनापि यत् प्रोक्तम् = यः कश्चित् यदकथयत् (जिस किसी से जो कहा), तस्य = अमुष्य (उसका), तत्वार्थ = यथार्थ (जैसा का तैसा), निर्णयः = परिणति (निर्णय करना) येन कर्तुं शक्यो भवेत् = यः कर्तुम् शक्नोति (जो कर सकता है), सः = असौ (वह), विवेक = ज्ञानं (सोच-समझ), इति ईरितः = इति कथ्यते (ऐसा कहलाता है)।

सन्दर्भ-प्रसङ्गश्च- यह पद्य हमारी ‘शेमुषी’ पाठ्यपुस्तक के ‘सूक्तयः’ पाठ से लिया गया है। मूलतः यह पाठ तमिल कवि तिरुवल्लुवर कृत ‘तिरक्कुरल’ के संस्कृतानुवाद से संकलित है। इस श्लोक में कवि कहता है कि तत्वार्थ निर्णय विवेक से ही सम्भव है।

हिन्दी-अनुवादः – जिस किसी के द्वारा जो कहा गया है वह उसका यथार्थ निर्णय है, वह करने में समर्थ होना चाहिये, वह विवेक कहलाता है।

6. वाक्पटुधैर्यवान् मंत्री सभायामप्यकातरः।
स केनापि प्रकारेण परैर्न परिभूयते ।।6।।

अन्वयः – वाक्पटुः धैर्यवान् सभायाम् अपि अकातरः सः केनापि प्रकारेण परैः न परिभूयते।

शब्दार्थाः – वाक्पटु = वाचि, सम्भाषणे वा पटुः (बातचीत में चतुर), धैर्यवान् = धृतिमान् (धैर्य रखने वाला), सभायामपि = संसदि अपि (सभा में भी), अकातरः = वीरः, साहसी च (वीर और साहसी), सः = असौ (वह), केनापिप्रकारेण = कथमपि (ये भी), परैः = शत्रुभिः, अरिभिः (शत्रुओं द्वारा), नपरिभूयते = नाभिभूतये पराभूत (पराजित नहीं होगा)।

सन्दर्भ-प्रसङ्गश्च – यह पद्य ‘शेमुषी’ पाठ्यपुस्तक के सूक्तयः पाठ से लिया है। मूलतः यह तमिल कवि रचित तिरुक्कुरल ग्रंथ के संस्कृत अनुवाद से संकलित है। इस श्लोक में कवि कहता है कि जिसका मंत्री वाक्कुशल, धैर्यवान व सभा में निर्भय होता है, वह शत्रुओं से कभी भी पराजित नहीं होता।

हिन्दी-अनुवादः – वाणी में चतुरता, धैर्ययुक्त, सभा में भी जो साहसी हो, ऐसा मंत्री किसी भी प्रकार से शत्रुओं द्वारा पराजित नहीं किया जा सकता है।

7. य इच्छत्यात्मनः श्रेयः प्रभूतानि सुखानि च।
न कुर्यादहितं कर्म स परेभ्यः कदापि च ।।7।।

अन्वयः – यः आत्मनः श्रेयः प्रभूतानि सुखानि च इच्छति, परेभ्यः अहितं कर्म कदापि च न कुर्यात्।

शब्दार्थाः – यः = यो (जो), आत्मनः = स्वकीयं (अपने), श्रेयः = कल्याणम् (कल्याण को) (तथा), प्रभूतानि = बहूनि (बहुत से), सुखानि = सुखसाधनानि (सुखों को), इच्छति = ईहते (चाहता है), सः = उसे, परेभ्य = अन्येभ्यो (दूसरों का), अहितं = नुकसान (हानि), कार्यम् = करणीयम् (काम), कदापि न कुर्यात् = कदापि न कर्त्तव्यम् (कभी नहीं करना चाहिये।)

सन्दर्भ-प्रसङ्गश्च – यह पद्य हमारी ‘शेमुषी’ पाठ्य-पुस्तक के ‘सूक्तयः’ पाठ से लिया गया है। मूलतः यह पाठ तमिल ल्लुवरकृत ‘तिरुकुरुल’ के संस्कृत अनुवाद से संकलित है। इस श्लोक में कवि कहता है कि जो मनुष्य अपना भला चाहता है उसे दूसरों का अहित नहीं करना चाहिये।

हिन्दी-अनुवादः – जो व्यक्ति अपना भला तथा बहुत सारे सुख चाहता है उसे दृ मरे के लिये कभी कोई अहित कर्म नहीं करना चाहिये।

8. आचारः प्रथमो धर्मः इत्येतद् विदुषां वचः।
तस्माद् रक्षेत् सदाचारं प्राणेभ्योऽपि विशेषतः।।8।।

अन्वयः – आचारः प्रथमो धर्मः इति एतद् विदुषां वचः तस्मात् विशेषतः सदाचारं प्राणेभ्योऽपि रक्षेत्।

शब्दार्थाः – आचारः = सदाचारः (आचरण), प्रथमः धर्मः = प्रमुखो धर्मः (पहला धर्म है), इति = एव (इस प्रकार), विदुषां – प्रज्ञानां (विद्वान में), वचन = वचनानि, (उक्ति), तस्मात् = अनेन कारणेन (इसी वजह से), सदाचारम् = सद्व्यवहारं (सदाचार की), प्राणेभ्यः = प्राणपणेन (प्राणों की बाजी लगाकर), रक्षेत् = त्रायेत (रक्षा करनी चाहिये)।

सन्दर्भ-प्रसङ्गश्च – यह पद्य हमारी ‘शेमुषी’ पाठ्य-पुस्तक के ‘सूक्तयः’ इस ग्रन्थ से उद्धृत है। यह पाठ मूलतः तमिल कवि तिरुवल्लुवर विरचित ‘तिरुकुरुल’ के संस्कृत-अनुवाद से सङ्कलित है। इस श्लोक में कवि आचार को प्रथम धर्म कहते हुए सदाचार का महत्व कहता है।

हिन्दी-अनुवादः – सदाचार पहला धर्म है। यह विद्वानों का वचन है, इसलिये विशेष रूप से प्राणों की बाजी लगाकर भी सदाचार की रक्षा करनी चाहिये।

JAC Board Class 9th Social Science Important Questions Civics Chapter 5 Democratic Rights

I. Objective Type Questions

1. Which of the following countries had a major terrorist attack an 11 September 2001?
(a) United states of America
(b) China
(c) India
(d) South Africa
Answer:
(a) United states of America

2. Which of the following countries is ruled by a dynasty?
(a) China
(b) Saudi Arabia
(c) Japan
(d) India
Answer:
(b) Saudi Arabia

3. Kosovo was a province of which of the following countries before its split?
(a) Egypt
(b) Yugoslabia
(c) USA
(d) Saudi Arabia
Answer:
(b) Yugoslabia

4. Which one of the following is a Fundamental Right in India?
(a) Right to freedom of religion
(b) Right against Exploitation
(c) Cultural and Educational rights
(d) All of these
Answer:
(d) All of these

5. When was the national human rights commission established in India?
(a) 1947
(b) 1950
(c) 1993
(d)2019
Answer:
(c) 1993

II. Very Short Answer Type Questions

Question 1.
What was the reason given by United States of America for imprisoning people at guantanamo bay?
Answer:
United States of America considered the people as their enemies and linked them to the attack on New York on 11th September, 2001.

Question 2.
Which body exposed to the world that prisoners at guantanama bay were being tortured in ways that violated the US law.
Answer:
Amnesty International.

Question 3.
What was Milosavic’s attitude towards the albanians?
Answer:
Milosavic was a narrow-minded Serb nationalist, who wanted serbs to dominate the albanians as his government was hostile to the kosovo albanions.

Question 4.
What is meant by the term‘Right’.
Answer:
Rights are reasonable claims of person recognised by society and sanctioned by law.

Question 5.
What is the need of rights in a democracy. Give a reason.
Answer:
Rights are necessary for the very systenance of democracy.

Question 6.
Define Fundamental Rights.
Answer:
Basic human rights guaranteed in the constitution are called Fundamental Rights. Articles 12 to 35 of the constitution deal with fundamental Rights.

Question 7.
How many fundamental Rights does the Indian constitution provide?
Answer:
Indian constitution provides six fundamental rights.

Question 8.
Which articles of the constitution deal with Fundamental Rights?
Answer:
Articles 12 to 35.

Question 9.
According to which fundamental right, the public places should be accessible to all the persons?
Answer:
Under right to equality, the public places should be accessible to all the persons.

Question 10.
Mention any four freedoms given in the constitution of India.
Answer:

1. Freedom of speech and expression,
2. Assembly for peaceful manner.

Question 11.
What do you understand by human trafficking?
Answer:
gale and purchase of human beings‘is called human trafficking?

Question 12.
What is meant by the term‘Begar’?
Answer:

Question 13.
‘Begar’ is a practice where the worker is forced to render service to the ‘master’ free
Answer:
The fundamental rights, which ensures the ban on bounded labour is ‘Right against exploitation’.

Question 14.
What is the minimum age below which no one can employ a child?
Answer:
The constitution prohibits child labour. No one can employ a child below the age of 14 to work in any factory.

Question 15.
What is a secular state?
Answer:
ln a secular state, no religion can get any privilege or favour and there is no official

Question 16.
If our fundamental rights are violated, where can we seek the remedy?
Answer:
if our fundamental rights are violated, we seek remedy from the supreme court or high court.

Question 17.
Who called the right to constitutional remedies, the heart and soul of our constitution?
Answer:
Dr. B.R. Ambedkar called the right to constitutions remedies, ‘the heart and soul’ of our constitution.

Question 18.
Can a law violating fundamental rights be made?
Answer:
There can be no law or action that violates the fundamental rights.

Question 19.
What is writs?
Answer:
A formal document containing an order of the court to the government issued only by high court or the supreme court is called writs.

Question 20.
“From time to time, the courts gave judgements to expand the scope of rights.” What are these rights?
Answer:

1. Right to freedom of press
2. Right to informations
3. Right to education
4. Right to food.

Question 21.
Who has the responsibility to provide free and compulsory education to all children up to the age of 14 years?
Answer:
The governments are responsible for providing free and compulsory education to all children up to the age of 14 years.

Question 22.
Name any two political rights.
Answer:

1. Right to vote,
2. Right to contest election.

Question 23.
Give any twro features of fundamental rights.
Answer:
Two important features of fundamental rights are that these are limited and these can be suspended during emergency.

Question 24.
What are Human Rights?
Answer:
The Human Rights are such essential conditions of life, without which no person can be a human being in the real sense. These are natural to man like the breath in his nostrils. Any denial of these human rights means to the denial of existence of man himself.

III. Short Answer Type Questions

Question 1.
Who was Milosevic? What was his government’ policy towards the albanians?”
Answer:
Milosevic was a narrow-minded serb nationalist leader who won elections in
Yugoslavia. His government behaved very harshly towards the Albanians of kosovo. The population as over whelming ethnic albanians in kosovo. But the serb people were in abundance throughout the country. He wanted the serbs to have complete control over the country.

Question 2.
What are rights? Why is it said that right comes with an obligation to respect others rights? Explain.
Answer:
Right: Rights are the reasonable claims of persons recognised by society and sanctioned by law. Rights come with an obligation to respect other’s rights as.

1. All of us want to live happily, without fear and without being subjected to degraded treatments .
2. For this we expect others to behave in such a way that does not harm us.
3. So a right is possible when we make a claim that is equally possible for others.
4. The claims should be reasonable and such that can be available to others in equal measure.

Question 3.
Why do we need rights in a democracy? Describe it.
Answer:
Rights are necessary for the very sustance of a democracy. Without rights, democracy will become meaningless and ineffective. For democratic elections, it is necessary that citizens should have the right to express their opinions, form political parties and take part in political activities. Rights protect minorities from the oppression of majority.

They ensure the majority cannot do whatever it likes. The government is expected to protect citizen’s rights. But sometimes elected government may not protect or may even attack the rights of their own citizens. So, some rights need to be placed higher than the government so that it cannot violate them.

Question 4.
Mention the Fundamental Rights which have been provided to the citizens by the constitution.
Answer:

1. Right to Equality (Articles 14 to 18)
2. Right to Freedom (Articles 19 to 22)
3. Right against Exploitation (Articles 23 and 24)
4. Right to Freedom of Religion (Articles 25 to 28)
5. Cultural and Educational Rights (Articles 29 and 30)
6. Right to constitutional Remedies (Articles 32)

Question 5.
Mention any two exceptions to the Right to equality.
Answer:
The Right to Equality contains two exceptions, which are as follows:

1. The state can make special provisions for women and children, scheduled castes and scheduled tribes and backward classes. These sections of our society need special protection because, often, they have been the victims of unequal treatment.
2. The state can reserve some posts in the government offices of SCs, STs and OBCs.

Question 6.
What are the various aspects of ‘Right to Equality’?
The various aspects of ‘Right to Equality’ are as follows:
1. Equality before Law:
The constitution guarantees that all citizens are equal before law. There is no discrimination on the basis of race, caste, sex or
place of birth. The state cannot discriminate against anyone in the matter of employment.

2. Protection of Weaker Sections:
The right to equality gives special provisions for women and children.

3. Reservation:
In legislatures, educational institution and government offices, some seats are reserved for the persons belonging to the weaker sections of society.

4. Ban on Untouchability:
Untouchability has been made an offence. Anyone who practises untouchability is liable to punishment. No citizen can be denied across to shops, public restaurants, hotels and places of public entertainment

Question 7.
“Right to Freedom” is a cluster of six rights. Mention these rights.
Answer:
The Article 19 gives six fundamental freedoms, which are given below:

1. Freedom of speech and expression.
2. Freedom to assemble peacefully and without arms.
3. Freedom to form association or unions.
4. Freedom of movement throughout India.
5. Freedom to reside and settle in any part of India.
6. Freedom to practice any profession or to carry on any occupation.

Question 8.
What are the rules to be followed while arresting or detaining a person?
Answer:
The following rules have to be followed while arresting or detaining a person.

1. A person who is arrested and detained in custody will have to be informed of the reasons for such arrest and detention.
2. A person who is arrested and detained shall be produced before the nearest
   magistrate within a period of 24 hours of arrest.
3. Such a person has the right to cousult a lawyer or engage a lawyer for his defence.

Question 9.
Which provisions are made in the constitution to prevent exploitation of the weaker sections.
Answer:
Following are the three provisions made in constitution to prevent exploitation of the weaker sections.

1. The constitution prohibits traffic in human beings especially the weaker sections.
2. The constitution also prohibits child labour. No one can employ a child below the age of 14 to work in any factory or mine or any hazardous work.
3. The constitution prohibits forced labour or begar in any form because begar is a practice where the worker is forced to render service to the master free of charge or at a nominal remuneration.

Question 10.
Mention any three features of Right to Freedom of Religion.
OR
‘India is a secular state’. Justify by giving examples.
Answer:
India is a secular state because the Indian constitution provides us Right to Freedom of Religion under Article 25 to 28 with the objective to sustain the principle of secularism in the country. Right to Freedom of Religion provides religious freedom to all the citizens of India. The main declaration of this Right are as under:

1. All religions are equal before the state and no religion will be given preference over the other.
2. Citizens are free to practice their respective religions.
3. No state can run institutions which can give religious education. Religious communities can set up charitable institutions of their own. However, there could be activities in such institutions are performed according to the laws laid down by the government.

Question 11.
Explain the Right to Cultural and Educational rights.
Answer:
India is a multi-religious, multi-lingual and multi-cultural nation, each having its own identity and characteristics. To preserve these diversities, it is natural to grant the following cultural and Educational Rights to the minorities and other communities.

1. Right to conserve the Language, Script and Culture (Article 29):
Under this Right minorities have the right to conserve their distinct language, script or culture, and to join state-owned or state-aided institution without discriminations.

2. Right to Establish Educational Institutions (Article 30):
Under this, each minority group has a right to establish and administer educational institutions of its choice. The state shall not discriminate between minorities while granting aid to their educational institutions.

Question 12.
How does constitution safeguard Fundamental Rights?
OR
Explain the Right which protects all other Rights.
OR
What do you mean by the ‘Right to Constitutional Remedy’?
Answer:
Right to Constitutional Remedy is the Right which protects all other Rights.

1. The constitution States that the courts have a duty to protect citizens’ rights.
2. Every citizen has a right to go to a court to enforce his fundamental rights.
3. He can challenge any act of the Government against his fundamental rights.
4. Courts can issue orders to the government in case of violation of the fundamental rights.
5. These court orders are known as Writs.

Question 13.
The right to constitutional remedies is the ‘heart and soul’ of the Indian constitution”. Justify the statement.
OR
Why did Br. B.R. Ambedkar call the right to constitutional remedies the heart and soul of our constitution?
Answer:
The right to constitution remedies is the heart and soul of the indian constitution was said by Dr. B.R. Ambedkar because.

1. When any of our rights is violated we can seek remedy through courts. If it is a fundamental right, we can directly approach or the supreme court or the high court of the state.
2. If any act of legislature or executive takes already or limits any of the fundamental rights, it will be invalid. We can challenge such law of the central or the state government in the court of law.
3. The supreme court and the high court have the power to issue writs, orders or directions for the enforcements of the fundamental rights.
4. Right to constitutional remedies makes other rights effective. This right is the guardian of other rights.

Question 14.
What is National Human Rights Commission? What are its functions?
Answer:
National Human Rights Commission (NHRC) is an independent commission. It was set up in 1993 to perform the following functions:

1. It can make an independent and credible inquiry into any case of violation of human rights.
2. It can inquire into any case of violation or negligence in controlling it by any government officer.
3. It can take any step to promote human rights in the country.
4. It can summon witnesses and question any government official.
5. It can visit any prison for inspection or send its own team for on-the-spot inquiry.

Question 15.
Describe the near rights given to the citizens by the constitution of South Africa?
Answer:
In South Africa the following new rights have been given by the constitution to its
citizens.

1. Right to privacy So that citizens or their home cannot be searched, their phones cannot be tapped, their communication can not be opened.
2. Right to an environment that is not harmful to their health or well being.
3. Right to have access to adequate housing.
4. Right to have access to health care services. Sufficient food and water; no one may be refused emergency medical treatment.

Question 16.
Explain the international covenant on economic, social and cultural rights.
Answer:
The following rights were accepted in the international convenant.

1. Right to work opportunity to everyone to earn livelihood by working.
2. Right to safe and healthy working conditions, fair wages that can provide decent standard of living for the workers and their families.
3. Right to adequate standard of living including adequate food, clothing and housing.
4. Right to health: medical care during illness, special care for worsen during child birth and prevention of epidemics.
5. Right to education: free and compulsory primary education, equal access to higher education.

IV. Long Answer Type Questions

Question 1.
What was the Amnesty International’s report regarding the prisoners in Guantanamo Bay? Describe
OR
Explain the condition of prisoners in Guantanamo Bay
Answer:
condition of prisoners in Guantanamo Bay About 600 people were secretly picked up the United States of America’s forces from all over the world and put in a prison Guantanamo Bay, and area near Cuba controlled by American Navy. The Americ, Government said they were enemies of the USA and linked them to the attack 01 New York (USA) on 11th September 2001.

In most cases, the governments of their countries were not asked or even informed about their imprisonment. Families of prisoners, media or even United Nations Organization’s representatives were not allowed to meet them. The USA army arrested them, interrogated them and decided whether to keep them there or not. There was no trial before any magistrate in the USA. Even the prisoners could not approach courts in their own country.

Amnesty International’s Report The amnesty International reported that the prisoners were being tortured in ways that violated the united nations organization’s laws. They were being denied the treatment that even prisoners of war must get as per international treaties. Prisoners were not released even after they were officially declared not guilty. An independent inquiry by the UN supported these findings.

Question 2.
The rights of citizens are being violated in Saudi Arabia justify this statement.
Answer:
The rights of citizens are being violated in Saudi Arabia. This is clear from the following facts.

1. The country is ruled by a hereditary king and the people hance no role in electing or changing their rulers.
2. The king selects the legislature as well as the executive. He appoints the judges and can change any of their decisions.
3. Citizens cannot form political parties or any political organizations. Media cannot report anything that the monarch does not like.
4. There is no freedom of religions. Every citizen is required to be muslim. Non- muslim residents can follow their religion in private, but not in public.
5. Women are subjected to many public restrictions. The testimony of one man is considered equal to that of two women.

Question 3.
What fundamental rights are given by the Indian constitution to the citizens? Describe in detail.
Answer:
The Indian constitution has given 6 fundamental rights to the citizens.
1. Right to equality (Articles. 14-18)
2. Right to freedom (Articles. 19-22)
3. Right against exploitation (Articles. 23-24)
4. Right to freedom of religion. (Articles. 25-28)
5. Cultural and Educational right (Articles. 29-30) and
6. Right to constitutional remedies. (Articles. 23-35)

1. The right to equality includes equality before the law the prohibition of discrimination on grounds of religion, race, caste, gender or place of birth, equality of opportunity in matters of employment, the abolition of untouchability and abolition of titles.

2. The right of freedom includes freedom of speech and expression, assembly, association or union or cooperatives, movement.
Residence, and right to practice any profession or oecupat ;on.

3. The right against exploitation prohibits all forms of forced labour, child labour and trafficking of human beings.

4. The right of freedom of religion includes freedom of conscience and free profession,
practice, and propagation of religion, freedom to manage religious affairs, freedom from certain taxes and freedom from religious instructions in certain educational institutes. .

5. Cultural and educational rights preserve the right of any section of citizens to conserve their culture, language or script, and right of minorities to establish and administer educational institutions of their choice.

6. The right to constitutional remedies is present for enforcement of fundamental right. This right makes other rights effective. When any of the fundamental rights are violated, then citizens can directly approach the supreme court or the high courts. Both courts have the power to writs for the enforcement of the rights.

Question 4.
What are the freedom provided by the Indian constitution to every citizen? Describe any one.
Answer:
The indian constitution has provided the following freedoms to every citizen:

1. Freedom of speech and expression.
2. Assembly in a peaceful manner.
3. Form associations and unions.
4. Move freely through out the country.
5. Reside in any part of the country.
6. Practice any profession, or to carry on any occupation, trade or business.

Freedom of speech and expression:
Freedom of speech and expression is a fundamental human right. It reinforces all other human rights, allowing society to develop and progress. Freedom of speech and expression means the right to express One’s own convictions and opinions freely by words of mouth, writing, printing, pictures or any other mode.

We may express our views through pamphlets, magzines, news papers, paintings, poetry or songs. How ever, we can not use this freedom to stimulate violence against others and excite people to rebel against government. Neither can we use it to against other’s by saying false and mean things that cause to a person’s reputation. “This right is available only to a citizen of India and not the foreign nationals.

JAC Class 9 Social Science Important Questions

JAC Board Class 9th Social Science Important Questions Civics Chapter 4 Working of  Institutions

I. Objective Type Questions

1. Who is the highest formal authority in our country?
(a) The President of India
(b) The Vice-President of India
(c) The Prime Minister on India
(d) The Chief Justice India
Answer:
(a) The President of India

2. The Indian parliament consists of how many houses?
(a) One
(b) Two
(c) Three
(d) Four
Answer:
(b) Two

3. Which is called as ‘upper chamber’ of the Indian parliament?
(a) Lok Sabha
(b) Rajya Sabha
(c) President’s office
(d) None of the above
Answer:
(b) Rajya Sabha

4. Which of the following is called as ‘Lower chamber’ of the parliament?
(a) Rajya Sabha
(b) Lok Sabha
(c) President’s office
(d) None of the above
Answer:
(b) Lok Sabha

5. Which one of the following is incorrect about the supreme court of India?
(a) It can hear appeals against the decisions of the high courts.
(b) It is the highest court of appeal in civil cases. –
(c) it cannot take up any dispute between citizens of the county.
(d) All of these.
Answer:
(d) All of these.

II. Very Short Answer Type Questions

Question 1.
Which are the three institutions in a Parliamentary democracy ?
Answer:

1. Legislature,
2. Executive and
3. Judiciary.

Question 2.
What do you understand by office memorandum?
Answer:
A communication issued by an appropriate authority stating the policy or decision of the government is called office memorandom.

Question 3.
What is the full form of SEBC?
Answer:
SEBC Socially and Educationally Backward classes.

Question 4.
Who are the decision makers in India?
Answer:
The president, the Prime Minister and the parliament are the decision makers.

Question 5.
Who is formally the country’s highest officer?
OR
Who is the head of the country in India?
Answer:
President.

Question 6.
Who is the head of government?
Answer:
Prime minister is the head of the government.

Question 7.
Which political institutions take all important policy decisions in our country?
Answer:
The Prime Minister and the cabinet are institutions that take all important policy decisions.

Question 8.
How many Houses of Indian parliament are there?
Answer:
The Indian parliament has two houses

1. Lok Sabha
2. Rajya Sabha.

Question 9.
When was the second backward classes commission set up?
Answer:
In 1979.

Question 10.
Who was the head of the second backward classes commission?
Answer:
B.P. Mandal.

Question 11.
Mention any one recommendation of the mantal commission.
Answer:
One recommendation is that 27% of government jobs be reserved for the socially and educationally backward classes.

Question 12.
Who is the final authority for making laws in any country?
Answer:
The parliament is the final authority for making laws in any country.

Question 13.
How many members are presently in Lok Sabha?
Answer:
There are 545 members on Lok Sabha presently.

Question 14.
Name the three components of the Indian Parliament.
Answer:

1. The President of the Union.
2. The Lok Sabha or the House of the People.
3. The Rajya Sabha or the Council of States.

Question 15.
What do you know about Lok Sabha?
Answer:
Lok Sabha is the lower house of the Indian parliament, which is directly elected by the people and exercises the real power on behalf of the people.

Question 16.
What is Rajya Sabha?
Answer:
Rajya Sabha is the upper house of the Indian parliament, which is indirectly elected by the people.

Question 17.
How many members are presently in Rajya Sabha?
Answer:
There are 245 memebers in Rajya Sabha presently.

Question 18.
What is the term of Lok Sabha?
Answer:
Five years.

Question 19.
What is the tenure of the members of Rajya Sabha?
Answer:
Six years.

Question 20.
Can this house be dissolved or is it permanent?
Answer:
Rajya Sabha is a parmanent house, so it can not be dissolved wheres Lok Sabha can be dissolved.

Question 21.
Which is the Upper House of the Parliament?
Answer:
The Rajya Sabha.

Question 22.
Which is the Lower house of the Parliament?
Answer:
The Lok Sabha.

Question 23.
In what ways Lok Sabha exercises supreme power over Rajya Sabha?
Answer:
Lok Sabha exercises more powers on money matter and during the joint session, final decision is taken by Lok Sabha because of its large number of members.

Question 24.
Who exercises more powers in money matters?
Answer:
The Lok Sabha.

Question 25.
Which house is better placed with regard to control over the executive in our country?
Answer:
Lok Sabha.

Question 26.
What do we mean by government?
Answer:
By government we refer to the executive.

Question 27.
In which house of the Indian parliament a money bill can be introduced?
Answer:
Lok Sabha.

Question 28.
Describe the type of executive.
Answer:

1. political executive
2. permanent executive.

Question 29.
Who are civil servants?
Answer:
Person, working in civil services are called civil sarvants.

Question 30.
What work do secretaries of different departments do?
Answer:
The secretaries provide the necessary back ground information to the ministers to . take decisions.

Question 31.
Which is the most important political institution in our country?
Answer:
Prime Minister is the most important political institution in our country.

Question 32.
Who appoints the Prime Minister in our country?
Answer:
The President appoints the Prime Minister in our country.

Question 33.
Whom does the President appoint as Prime Minister?
Answer:
The President appoints the leader of the majority party or the coalition of parties that commands a majority in the Lok Sabha.

Question 34.
Who is the leader of the Lok Sabha?
Answer:
The Prime Minister.

Question 35.
What is the tenure of the Prime Minister?
Answer:
The Prime Minister does not have a fixed tenur. He continues in power so long as he remain the leader of the majority party or coalition.

Question 36.
How are members of Council of Minister appointed india?
Answer:
The members of Council of Minister appointed by the president on the advice of the Prime Ministers.

Question 37.
A person who is not a memeber of any house of parliament is appointed as minister within what time he has to get elected to one of the house of the parliament?
Answer:
Within 6 months, he has to be elected to one of the house of the parliament.

Question 38.
Which types of ministers are included in the union council of ministers?
Answer:
Union council of ministers include cabinet ministers, ministers of state and ministers of state with independent charges.

Question 39.
Who presides over cabinet meetings?
Answer:
The Prime Minister presides over cabinet meetings.

Question 40.
State any two functions of the Prime Minister.
Answer:

1. Presiding over cabinet meetings.
2. Distibution and redistribution of work to ministers.

Question 41.
In which type of government the Prime Minister cannot take decisions as he likes?
Answer:
The Prime Minister of a coalition government can not take decisions as he likes.

Question 42.
The President of India is like the Queen of which country?
Answer:
The President of India is like the Queen of Britain whose functions are to a large extent ceremonial.

Question 43.
What is the term of office for the President?
Answer:
Five years.

Question 44.
Who supervises the functioning of all political institutions in the country?
Answer:
The President supervises the overall functioning of all the political institutions in the country.

Question 45.
How is the President elected in India?
Answer:
The President in India is not directly elected by the public. Members of the parliament and members of the legislative assemblies of the entire country are elected by him.

Question 46.
In whose name are international treaties and agruments made?
Answer:
All international treaties and agreements are made in the name of the president.

Question 47.
Who is the supreme commander of India’s defence forces?
Answer:
The President is the supreme commander of the defence forces of India.

Question 48.
A bill passed by parliament becames law only after whose approval?
Answer:
A bill passed by parliament becomes law only after the approval of the President.

Question 49.
State any two functions of the President.
Answer:

1. He appoints the Prime Minister.
2. He approves bills passed by the parliament.

Question 50.
When can the President exercise his discretionary powers in the matter of appointment of the Prime Minister?
Answer:
When a party or coalition does not get a majority in the Lok Sabha, the President can exercise his discretionary powers.

Question 51.
What is the highest Court in the country called?
Answer:
The Supreme Court.

Question 52.
Where is the Supreme Court located in India?
Answer:
Delhi.

Question 53.
Describe the structure of Indian judiciary.
Answer:
The Indian judiciary consists of a supreme court for the entire nation, High courts in the states, district courts and the courts at local level.

Question 54.
Who controls the judicial administration of the country.
Answer:
The supreme court controls the judicial administration of the country

Question 55.
Whose verdict is required by all the courts of the country?
Answer:
Of the supreme court.

Question 56.
All the courts of the country have to decide whose decision?
Answer:
Of the supreme court.

Question 55.
Which dispute can the supreme court hear?
Answer:
The supreme court can hear any of the following disputes.

1. Between citizens of the country,
2. Between citizens and government,
3. Between two or next more state government, and
4. Between government at the union and state level.

Question 58.
Name the institution where disputes between two or more state governments are finally settled in our country.
Answer:
The disputes between two or more state governments are finally settled in the supreme court.

Question 59.
Which is the highest court of appeal in civil and criminal cases?
Answer:
Supreme court.

Question 60.
Which institution can hear appeal against the decisions of the high courts?
Answer:
The supreme court can hear appeals against the decisions of the high courts.

Question 61.
What does independence of the judiciary means?
Answer:
Indepenence of the judiciary means that it is not under the control of the legislature or the executive.

Question 62.
What is the procedure for the removal of Judges of the Supreme Court ?
Answer:
A Judge can be removed by the President on the grounds of ‘proved misbehaviour or incapacity’ but the resolution for his impeachment should be passed by both the Houses.

Question 63.
Who have the power to interpret the constitution of the country?
Answer:
The supreme court and the high courts have the power to interpret the constitution of the country.

Question 64.
In what ways does the powers and independence of the Indian judiciary give him the ability to function ?
Answer:
The powers and the independence of the Indian judiciary allow it to act as the guardian of the fundamental right.

Question 65.
Why is the Supreme court called the ‘Guardian of the Constitution’?
Answer:
The written constitution of the country is the fundamental law of the land. It is the supreme law, above all men, matters and legislation. The Supreme Court has been given the power to interpret the constitution, declare the law and enforce the principle of and balances, prevalent in the constitution.

Question 66.
‘The Supreme Court is the Guardian of the Fundamental Rights’. Explain.
Answer:
The Supreme Court is the guardian of the Fundamental Rights. A citizen, whose fundamental right has been violated by any individual, government or institution, may move to the Supreme Court for the protection of his Fundamental Rights.

Question 67.
What is PIL?
Answer:
Public Interest Litigation (PIL) means litigation for the protection of public interest. It is the power given to the public by courts through Judicial activism. Such cases may occur when the victim does not have the necessary resources to commence litigation or his freedom to move court has been suppressed.

III. Short Answer Type Questions

Question 1.
Why do we need the political institutions? Give reasons?
Answer:
We need political institutions for the following:
1. To Take Decisions:
Institutions are required to take decisions regarding the policies, to collect taxes, spending money on administration, defence and development programmes.

2. Implementation of Decisions:
The decisions which are taken by one institution have to be implemented. Most of the decisions are implemented by permanent executives.

3. To Solve the Dispute:
In a democratic country disputes may occur over decisions. So, courts have been established to solve the disputes.

4. Right decisions:
Institutions provide solid base to democracy. They provide an opportunity for a wider set of people to be consulted in any decision. These institutions help the rulers to take right decisions.

Question 2.
Give any two differences between the Lok Sabha and the Rajya Sabha.
Answer:
The differences between the Lok Sabha and the Rajya Sabha are as follows.

Lok Sabha	Rajya Sabha
1. The members of the Lok Sabha are directly elected by the people.	1. Members of the Rajya Sabha are indirectly elected by the members of the legislative assemblies.
2. The tenure of Lok Sabha is 5 years. The house can be dissolved berfore the expiry of the tenure.	2. The tenure of Rajya Sabha members is 6 years but one-third members retire after every 2 years. It can not be dissolved, it is a parmanent house.

Question 3.
Lok Sabha is more powerful than Rajya Sabha’. Analyse the statement.
Answer:
Lok Sabha is more powerful than Rajya Sabha. This is clear from the following facts.

1. Any ordinary law needs to be passed by both the houses but in case of conflict, view of Lok Sabha prevails because it has large number of members.
2. Once the Lok Sabha passes the budget or any other money related law, Rajya Sabha cannot reject it. The Rajya Sabha can delay it for a maximum of 14 days or suggest changes in it which may or may not be accepted by the Lok Sabha.
3. Most importantly, the Lok Sabha controls the Council of Ministers. If the majority of the Lok Sabha members say that they have no confidence in the Council of Ministers, then all the ministers including the Prime Minister have to resign. Thus, it can be concluded that Lok Sabha is more powerful than Rajya Sabha.

Question 4.
Differentiate between a Money Bill and an Ordinary Bill? (Any three)
Answer:

Money Bill	Ordinary Bill
1. A bill deals with income, expenditure, loans, investments and taxes is a Money Bill.	1. Any bill which is not a Money Bill is an Ordinary Bill.
2. It can be introduced only in the Lok Sabha.	2. It may be introduced in either House of the Parliament.
3. Prior permission of the President is sought to introduce this bill.	3. It is not necessary to take any prior permission of the President to introduce this bill.
4. The Rajya Sabha cannot reject a Money Bill. It can only make recommendations for changes in the Bill.	4. It is necessary to pass this bill by Rajya Sabha also. If Rajya Sabha rejects this bill, it can not be passed.

Question 5.
Differentiate between political executive and permanent executives.
Answer:
The following are the differences between a political executive and a permanent executive

Political executive	Permanent executive
1. Elected by the people (voters) for specific period.	1. Appointed a long term basis.
2. Answerable to the people.	2. Answerable to the government.
3. Political leaders are political executives	3. Civil servants are the permanent executives.

Question 6.
Why does the political executive have more power than the non political executive?
Answer:
There are following reasons for this.

1. In democracy, the will of the people is paramount, political executives are the elected representative of the public. Therefore, they use all the power on behalf of the people.
2. Political executives (ministers) are ultimately accountable to the public for their policy and decision.

Question 7.
How is the election of Prime Minister in India?
Answer:
There is no direct election for the post of Prime Minister in India. The President appoints the Prime Minister. The President appoints the leader of the majority party or coalition parties in the Lok Sabha as the Prime Minister.

In case no single party or alliance gets a majority, the president appoints the person most likely to secure a majority support. The Prime Minister does not have a fixed tenure. He continues in power so long as he remains the leader of the majority party or coalitions.

Question 8.
Write any three constraints on the powers of the Prime Minister of a coalition government.
Answer:
Following are the three constraints on the powers of Prime Minister of coalition government.

1. The agenda and policies of the government are usually decided as a common minimum programme which includes all the views and demands of coalition partners.
2. The Prime Minister has to accommodate different groups and fraction in his party as well as his alliance partners.
3. He has to pay attention to the views and positions of his coalition partners for
   the survival of his government.

Question 9.
Briefly describe which classes of ministers are there in the council of ministers.
Answer:
The Council of Ministers consists of three classes of ministers.

1. Cabinet minister: They are usually for leaders of the ruling party or parties who are incharge of the major ministeries.
2. Ministers of state with independent charge: They are usually incharge at smaller ministries. They participate in the cabinet meetings only when specially invited.
3. Ministers of state: They are attached to and required to assist cabinet ministers.

Question 10.
Explain the collective responsibility of fhe Cabinet.
Answer:
The Council of Ministers is collectively responsible and accountable to the Lok Sabha. The cabinet owes collective responsibility for all the decisions taken by it. If the Lok Sabha rejects the policy of the government on a particular issue, it is not only the Minister responsible for that subject, but also the whole council of Ministers must accept the responsibility and resign.

Question 11.
The Prime Minister is the line of communication between the Cabinet and the President. Justify by giving two examples.
Answer:

1. The Prime Minister conveys cabinet’s decisions to the the President, and keeps him informed of all national or foreign matters of the government.
2. He advises the President to summon and progue the Parliament and dissolve the Lok Sabha.

Question 12.
Discuss the relations of the Prime Minister with the Parliament.
Answer:

1. The Prime Minister is the leader of the Lok Sabha.
2. The Prime Minister is the chief spokesman of the government in the Parliament.
3. The Prime Minister is free to choose ministers as long as they are the members of Parliament.

Question 13.
In recent years the rise of coalition politics has imposed certain constraints on the power of the the Prime Minister. Justify the statement.
OR
Coalition politics has acted to curb the powers of the Prime Minister. Discuss.
Answer:

1. The Prime Minister of coalition government can not take decisions as he likes,
2. He has to accommodate different groups and factions in his party as well as among alliance partners.
3. He has also to need to the view and positions of the coalition partners and other parties, on whose support the survival of the government depends.

Question 13.
Write any three legislative powers of the President of India.
Answer:
Following are the three legislative powers of the President of India.

1. The Presindent is not a member of either house of the parliament. But she is an integral part of the legislative process. He plays an important role in making of laws.
2. The President has the power to dissolved the Lok Sabha. He can summon joint sitting of both houses of parliament.
3. The president has the power to send messages to either house of the parliament either regarding any pending bill or any other matter.

IV. Long Answer Type Questions

Question 1.
Why was the Mandal Commission appointed? What did it recommend to the government?
Answer:
The government of India appointed the second backward classes commission in the year 1979. B.P mandal was the head of Mandal Commission. Thus, it was popularly known as mandal commission.
It was appointed in order to:
1. Determine the criteria to identify the socially and educationally backward classes (SEBCs) in India.

2. To recommend steps to be taken for their advancement. The commission gave its report in 1980 and made following recommendations 27% of the government jobs be reserved for the socially and educationally backward classes. The report and recommendations were discussed in the parliament. On 6th August 1990, the government of India took a formal decision to implement the recommendation.

Question 2.
Describe the “Indira Sawhney and others v/s union of India case” along with its major reforms.
Answer:
In 1990, the central government implemented 27% reservation for socially and educationally backward classes in government jobs in accordance with the recommendations of the mandal commission. Some persons and associations opposed the order of government of implementing reservation and filed a number of cases in the courts. People appealed to the courts to declare the order invalid and stop its implementation.

The supreme court of India bunched all these cases together and this case came to be known as the ‘India Sawhney case’. Eleven judges of the Supreme Courts heard arguments of both sides. By a majority, the Supreme Court judges in 1992 declared the order of government was valid.

Supreme Court modified the orginal order and declared. That well to-do persons among the backward classes should be excluded from getting benefit of the reservation. Hence, the department of personnel and Training issued another office memorandum on 8th September, 1993.

Question 3.
Why do we need parliament? Explain in detail.
Answer:
We need parliament for the following reasons.
1. Parliament is the final authority for making laws in any country. This task of law making or legislation is so important that these assemblies are called legislatures. Parliaments all over the world can make new laws, change existing laws, or abolish existing laws and make new ones in their place.

2. Parliament all over the world exercise some control over those who run the government. In same countries like India this control is direct and full. Those who run the government can take decisions only so long as they enjoy support of parliament.

3. Parliament is required to control public money, i.e., treasury. In most countries the public money can be spent only when parliament sanctions it.

4. Parliament is the highest forum of discussion and debate on public issues and national policy in any country. Parliament can seek information about any matter.

Question 4.
What is parliament? Explain the major powers and function of the parliament.
Answer:
Parliament A national assembly of elected representatives of India is called parliament. Following are the major powers and functions of the parliament of India.

1. The parliament elects Presidents, Vice-President, Speaker, Deputy Speaker, ViceChairman of Rajya Sabha, etc. It can also remove President, Vice-President, Judges of the Supreme Court and High courts by passing a resolution of impeachment against them.
2. The parliament is the final authority for making laws in any country. In India, it makes laws on the subjects of union list, concurrent list and in certain cases on state list.
3. The parliament can seek information from and ask questions to the government and its ministers on any matter.
4. The parliament can pass a resolution of no-confidence against the ministry and ask it to resign. Those who run the government can take decision only so long as they enjoy support of the parliament.
5. The parliament is the highest fourm of discussion and debate oh public issues and national policies.
6. The governmental budget is passed in the parliament and it controls all the money that government has.

Question 5.
Distinguish between political and permanent executive. Why does the political executive have more power than the non-political executive?
Answer:
1. The political excecutive consists of the political leaders who have been elected by the people for a specific period. They work till the House is dissolved or their five- year term is over. The permanent executive consists of those persons who have been oppointed on a long term basis. It constitutes of civil servants.

2. In a democracy, the will of the people is supreme. The political executive, i.e. the minister is elected by the people and thus is empowered to exercise the will of the people on their behalf. He is finally answerable to the people for all the consequences of his decisions.

That is why the minister takes all the final decisions. Though, he takes the advice of experts on all technical matters. But very often experts hold different opinions before him, i.e., more than one single option. Depending on what the overall objective is, the minister decides. Therefore, he has more power than the non-political or permanent executive.

Question 6.
Who appoints the Council of Ministers? also explain its composition?
Answer:
Appointment of Council of Ministers:
After the appointment of the Prime Minister, the president appoints the minister of the Council of Ministers on the advice of the Prime Minister. The ministers are usually from the party or the coalition
that has the majority in the Lok Sabha. In other words, the President appoints the Council of Ministers on the advice of the Prime Minister.

Composition of Council of Ministers:
The council of ministers is the official name for the body that includes all the ministers. It usually has 60 to 80 ministers of different ranks. The Council of Ministers have collective responsibility to the Lok Sabha, it includes three types of ministers.

1. Cabinet ministers:
They are usually top leaders of the ruling party or parties who are in charge of the major ministries. They meet to take decisions in the name of the council of ministers cabinet is the inner ring of the Council of Ministers.

2. Ministers of state with independent charge:
They are usually incharge at smaller ministers. They participate in the cabinet meetings only when specially invited.

3. Ministers of state:
They are attached to and required to assist cabinet ministers.

Question 7.
Describe the powers and functions of the Prime Minister.
Answer:
As the head of the government, the Prime Minister has wide ranging powers. The power and functions of the Prime Minister are as follows:

1. He is free to choose ministers. He distributes and redistributes work to the ministers.
2. All ministers work under his leadership.
3. He chairs cabinet meetings.
4. He coordinates the work of different departments. His decisions are “final in case disagreement aries between different departments.
5. He exercises general supervision of different ministers.
6. He also has the power to dismiss ministers. When the Prime Minister quits the entire ministry quits.
7. He communicates to the president all decisions of the council of ministers relating to the administration of union and proposals of legislation.
8. He is the leader of the nation. People always remain ready to hear his views on all political and economic issues.
9. He is the ex-office chairman of Niti aayog. Which supervises economic policies.
10. When he attends the international meetings, represents his nation and whatever he says there is on behalf of his country.

Question 8.
Explain the election of the President.
Answer:
1. The President is elected indirectly. Ordinary citizens play no part in this election. She is elected by the Electoral College, a joint body of elected M.Ps and M.L.As. It is a temporary body which is dissolved immediately after the election of the President is over.

2. All the members of the Electoral College are expected to indicate their preference by writing 1, 2, 3 against the names Presidential Candidates, according to their choice, on the ballot paper.

3. If any one of the candidates secures more than 50 per cent of the total number of votes polled, she is declared elected.

4. If none of the candidates secures more than 50 per cent of the total number of votes polled, the candidate with the least number of voters is eliminated. His votes are transferred to the candidate for whom the voters have indicated second preference.

Question 9.
Describe the functions and powers of the President.
Answer:
Following are the functions and powers of the President:

1. All governmental activities take place in the name of the President.
2. All laws and major policy decisions of the government are issued in his name.
3. All major appointments are made in the name of the President. These include the appointment of the Chief Justice of India, the judge of Supreme Court and the High courts of the states, the governours of the states, the Election Commissioners Ambassadors to the countries, etc.
4. All international treaties and agreements are made in the name of the President.
5. The president is the supreme commander of the defence forces of India. He appoints to the highest posts of the defence forces.
6. He appoints the Prime Minister and members of the Council of Ministers.
7. Any bill passed by the parliament becomes law only after the signature of the President.
8. Every Union Territory in India is under the President and it is administered by him. He’texercises this power through an administrator appointed by him.

Question 10.
Explain in detail the differences between the presidential system of government and the parliamentary system.
Answer:
The following are the differences between the presdiential system of government and the parliamentary system.

Presidential system	Parliamentary system
1. In the presidential system, the executive is elected by direct voting by the public for a fixed term.	1. In the parliamentary system, the exdhtive is elected indirectly by the public for a fixed period.
2. The executive does not require the support of parliament to remain in power in this system.	2. This system requires the support of the majority of parliament to remain in power.
3. In this system, often the president does not get the support of parliament for his policies. Example—United States of America.	3. In this system the executive gets the support of the parliament for its policies. Example India
4. In this system, there is no difference between the nominal and the real executive of the nation. Both are the same.	4. In this system, the nominal and real executive of the nation are different.
5. The president has a central role in this form of gevernment.	5. The Prime Minister has a central role in this form of government.

Question 11.
Describe the function and powers of the supreme court.
Answer:
Following are the functions and powers of the supreme court:

1. The supreme court is the highest court in the country. If controls the entire judicial administration of the country. Its decisions are binding on all other courts of the country.
2. It hears appeals for criminal and civil matters, it also hears against the decisions of the high courts.
3. If can hear any dispute which is
   (a) Between citizens of the country.
   (b) Between citizens and the government.
   (c) Between the government of India and one or more states.
   (d) Between the government of India and one or more states on one side and one or more states on the other.
4. Betweeen two or more states governments.
5. It interprets the constitution of the country.
6. It protects the fundamental rights of citizens.

Question 12.
What do you mean by the independence of judiciary?
Answer:
By the independence of judiciary we mean that the judiciary should not be under the influence of any individual or authority. This also means a fair, impartial, fearless and honest judiciary. Following points are given below to justify the independence of judiciary :

1. Appointment of Judges:
The Judges of the Supreme Court and High Courts are appointed by the President of India on the advice of the Prime Minister. But it consults the chief justice of the Supreme Court.

2. Security of Tenure:
A Judge can remain in office till he has attained the age of 65 (in case of supreme court) and 62 years (in case of the High Court). He can be removed by the President on the grounds of “proved misbehaviour or incapacity”. But the resolution of his impeachment should be passed by two-third members of the two houses of the parliament.

3. No Discussion with Respect to the Conduct of Any Judge:
No discussion shall ‘ take place in Parliament with respect to the conduct of any judge in the discharge of his duties when a motion for this removal is under consideration.

4. Punishment for the Contempt of Court:
Genuine criticism of a judgement is allowed, but nothing should be done to lower the authority or dignity of the court.

Question 13.
“The Indian judiciary is free from legislature and executive control”. Explain.
Answer:
The Indian judiciary is free from legislature and executive control. This is evident from the following points.

1. The judges of the supreme court and high courts are appointed by the president on the advice of the Prime Minister. But it also consults the chief justice of the supreme court.
2. The senior most judge of the supreme court is usually appointed the chief justice.
3. Once a person is appointed a judge at the supreme or high court, it is almost impossible to remove him from his post.
4. A judge can be removed only by an impeachment motion passed separately by two-thirds members of the two houses of the parliament. This is a very difficult task. It has never happened in the history of Indian democracy.

Question 14.
“The judiciary in India is also one of the most powerful in the world”. Explain.
Answer:
The judiciary in India is also one of the most powerful in the world. This is evident from the following points:

1. The supreme court and high courts of India are empowered to interpret the constitution of the country.
2. The judiciary of India can examine the legality of any act done by the executive and any law passed by the legislature.
3. The Indian judiciary can declare any such law or any such function of the executive passed by the legislature at the national or state level as against the provisions of the constitution.
4. The Indian judiciary also acts as protector of fundamental rights. If the public is harm by any act of the government, then any person can go to the court and get justice by presenting a public interest litigation.
5. The judiciary can interfere the government’s decision-making power is misused.
6. The judiciary prevents the corrupt conduct of government officials.

JAC Class 9 Social Science Important Questions

JAC Board Class 9th Social Science Important Questions Civics Chapter 3 Electoral Politics

I. Objective Type Questions

1. Name the party formed by Chaudhary Devi Lai.
(a) Lok Dal
(b) National Congress
(c) BJP
(d) All of these.
Answer:
(a) Lok Dal

2. Which of the following terms is used when fresh election is held only for constituency to fill the vacancy caused by death or resignation of a member?
(a) By election
(b) General election
(c) Parliament election
(d) Assembly election.
Answer:
(a) By election

3. Which of the following states has the largest number of Lok Sabha seats?
(a) Bihar
(b) Uttar Pradesh
(c) Rajasthan
(d) Haryana.
Answer:
(b) Uttar Pradesh

4. What is the minimum age for voting in India?
(a) 18 years
(b) 25 years
(c) 30 years
(d) 35 years.
Answer:
(a) 18 years

5. What does EVM stand for?
(a) Electronic Volt Machine
(b) Electric Vending Machine
(c) Electronic Voting Machine
(d) None of these.
Answer:
(c) Electronic Voting Machine

II. Very Short Answer Type Questions

Question 1.
Which movement did Chaudhary Devi Lai lead?
Answer:
Chaudhary Devi Lai led a movement called Nyaya Yudh (Struggle for Justice).

Question 2.
What do you mean by Elections?
Answer:
In a democracy, administration is run by the representatives who are elected by the people. The process by which people elect their representatives is called Elections.

Question 3.
What are constituencies?
Answer:
For election, the entire country is divided into fixed electoral areas with a body of registered voters. These areas are called constituencies.

Question 4.
Mention a merit of electoral competition.
Answer:
One merit of electoral competition is that it gives choice to voters to choose best among the contesting candidates.

Question 5.
What is ‘party-politics’?
Answer:
Different political parties and leaders often level allegations aganist one another. Parties and candidates often use dirty tricks to win election. Such malpractices are collectively termed as ‘party-politics’.

Question 6.
What are the ‘two types’ of elections?
Answer:

1. General Election,
2. By-Election.

Question 7.
What do you mean by General Election?
Answer:
Elections to the Lok Sabha and the Vidhan Sabha in the states are normally held every nve years, which is known as General Election. ‘

Question 8.
What do you understand by By-Elections?
Answer:
If a representative from a constituency dies while in office or if the office falls va-cant because of reasons like resignation, fresh elections are held in that particular constituency. Such an election is called by-election.

Question 9.
Into how many constituencies has the country been divided for Lok Sabha elections?
Answer:
The country has been divided into 543 constituencies for Lok Sabha elections.

Question 10.
What is the representative, elected from each parliamentary constitutency called?
Answer:
Member of Parliament (MP).

Question 11.
Differentiate between MP and MLA.
Answer:
For Lok Sabha, the country is divided into various constituencies. The representative elected from each constituency is called Member of Parliament (MP). On the other hand, each state is divided into certain Assembly constituencies. The representative elected from them is called Member of Legislative Assembly (MLA).

Question 12.
What is the motive behind reserved constituencies?
Answer:
The motive behind reserved constituencies is to ensure proper representation of the weaker sections of our society like scheduled castes and scheduled tribes.

Question 13.
How many seats are reserved for the Scheduled Castes in the Lok Sabha?
Answer:
84 Seats.

Question 14.
How many seats are reserved for the Scheduled Tribes in the Lok Sabha?
Answer:
47 Seats.

Question 15.
What is the official name of voters’ list?
Answer:
Electoral roll.

Question 16.
What is Electoral Roll?
Answer:
The list of eligible voters is known as Electoral Roll and is commonly known as Voters’ List.

Question 17.
Who can be denied the right to vote?
Answer:
Criminals and persons with an unsound mind can be denied the right to vote.

Question 18.
What does‘EPIC’stand for?
Answer:
Election Photo Identity Card.

Question 19.
What is election symbols?
Answer:
At the time of any election, certain symbols are alloted by Election Commission to political parties. They are called election symbols.

Question 20.
What is meant by Model Code of Conduct?
Answer:
Model Code of Conduct is a set of norms and guidelines to be followed by political parties and contesting condidates during election time.

Question 21.
what is ballot paper?
Answer:
A ballot paper is a sheet of paper on which the names of the contesting candidates alongwith party name and symbols are listed.

Question 22.
Nowadays, which machine is used to record votes?
Answer:
Electronic Voting Machine (EVM).

Question 23.
Who appoints the Chief Election Commissioner of India?
Answer:
The President of India.

Question 24.
What is a turnout?
Answer:
The percentage of eligible voters who cast their votes in an election is known as turnout.

III. Short Answer Type Questions

Question 1.
Why do weneed Elections?
Answer:
We need Elections because:

1. Elec ons give the voters the right to elect their representatives or rulers.
2. Through elections, voters can choose who will form the government and take major decisions. They can choose the party whose policies will guide the government and law-making.
3. Elect ions provide political equality.

Question 2.
“In an election, the voters make many choices.” Explain the statement.
Answer:
in an election, the voters make many choices. This statement can be explained as follows:

1. They can choose who will make laws for them,
2. They an choose who will form the government and take major decisions,
3. They can choose the party whose policies will guide the government and law-making.

Question 3.
What makes an election democratic?
Or
What are the minimum conditions required for democratic elections?
Answer:

1. Everyone should have one vote and every vote should have equal value.
2. Parties and candidates should be free to contest elections and should offer some real choice to the voters. The choice should be offered at regular inter¬vals. Elections must be held regularly after every few years.
3. Elections should be conducted in a free and fair manner where people can choose as they wish.

Question 4.
Mention any four demerits of electoral competition.
Answer:
Following are the four demerits of electoral competition:

1. An electoral competition creates a sense of disunity and ‘factionalism’ in every locality.
2. Different political parties and candidates often use dirty tricks to win elec-tions.
3. The pressure of winning electoral fights does not allow sensible long-term policies to be formulated.
4. Some good people who wish to serve the country do not enter this arena as they do not like this unhealthy competition in politics.

Question 5.
Distinguish between general election and by election.
Answer:
Differences between general election and by election are as follows:

General Election	By Election
1. Elections are held in all constituencies at the same time either on the same time either on the same day or within a fear days. This is called a general election.	1. Election is held only for one constituency to fill the vacancy caused by death of resignation of a member. This is called a by election.
2. This election are held regularly after every five years.	2. This election is held before the expiry of six months from the date of the seat falls vacant.
3. By this elections, the representatives are elected for a full term of five years.	3. By this election the representative is elected for the remaining period of the house and not for five years.

Question 6.
What is an Election Photo Identity Card?
Answer:
Every eligible voter whose name appears in the voters’ list, is issued an identity card by the government. This contains the photograph of the voter. The card serves , as a mark of identification at the time of polling of votes. Such card is called as an Election Photo Identity Card.

Question 7.
Who can contest an election? What is the procedure for the nomination of candidates?
Answer:
Any citizen over 25 years of age can file his nomination. Filing the nomination means he can present himself as a candidate. Every person who wishes to contest an election has to fill a ‘nomination form’ and give some amount as ‘security deposit’.

Recently, a new system of declearation has been introduced at the direction of the Supreme Court, according to which, every candidate has to make a legal declaration, giving full details of their assets and liabilities, their family and their educational qualifications. This information has to be made public. This provides an opportunity to the voters to make their decision on the basis of the information provided by the candidates.

Question 8.
What is the importance of symbols in elections?
Answer:
Political parties have usually well-known symbols. Normally, a person recognises a party instantly from its symbol. Symbols are allotted by the Election Commission so that every condidate has a different symbol and the voters do not get confused.

Question 9.
What details are needed to be given by the candidates in a legal declaration?
Answer:
The candidates in a legal declaration are expected to give full details of:

1. Serious criminal cases pending against them.
2. Assets and liabilities of the candidates and their families.
3. Educational qualifications of the candidates.

Question 10.
What are the corrupt election practices forbidden by law?
Answer:
Corrupt election practices forbidden by law are as follows:

1. To bribe or threaten the voters.
2. Using government money or other public resources to aid an election campaign.
3. Spending more than the allowed amount of money for a Lok Sabha or Assembly election, and appeal to voters in the name of religion or caste.

Question 11.
Mention the norms of a Model Code of Conduct for election campaigns.
Answer:

1. Use any place of worship for election propaganda;
2. Use government vehicles, aircrafts and officials for elections;
3. Once elections are announced, ministers shall not lay foundation stones of any projects, take any big policy decisions or make any promises of providing public facilities.

Question 12.
How are votes casted?
Answer:
On the election day, every person whose name is on the voters’ list can go to a nearby ‘polling booth’, situated usually in a local school or a government office. Once the voter goes inside the booth, the election officials identify him, put a mark on his finger and allow him to cast his vote. An agent of each candidate is allowed to sit inside the polling booth and ensure that the voting is taking place in a fair way.

Earlier the voters used to indicate who they wanted to vote for by putting a stamp on the ballot paper. Nowadays, Electronic Voting Machines (EVM) are used to record votes. The machine shows the names of the candidates and the party symbols. Independent candidates too have their own symbols, alotted by the election officials. All the voters has to do press the button against the name of the candidate he/she wants to give his/her vote.

Question 13.
How are the votes counted?
Answer:
Once the polling is over, all the EVMs are sealed and taken to a secure place. A few days later, on a fixed date, all the EVMs from a constituency are opened and the votes secured by each condidate are counted. The agents of all candidates are present there to ensure that the counting is done properly.

The candidate who secures the highest number of votes from a constituency is declared elected. In a general election, usually the counting of votes in all the constituencies takes place at the same time, on the same day. Television, radio and newspapers report this event. Within a few hours of counting, all the results are declared and it becomes clear as to who will form the next government.

Question 14.
Write any four allegations about unfair practices on election, reported in newspapers and television.
Answer:
Newspapers and television generally report the following four allegations about unfair practices in elections.

1. Inclusion of false names and exclusion of genuine names in the voters’ list.
2. Misuse of government facilities and officials by ruling party.
3. Excessive use of money by rich candidates and big parties.
4. Intimidation of voters and rigging on the polling day.

Question 15.
Explain any two provisions which ensure the independency of the Election Commission.
Answer:
Following are the two provisions which ensure the independency of the Election Commission.

1. The members of the Election Commission are appointed by the President of India, but the members are not answerable to the President of India.
2. It is virtually impossible for the government to remove the members even if their work is not liked by the government.

Question 16.
Explain any four functions of the Election Commission of India.
Answer:
Following are the four functions of the Election Commission of India:

1. Election Commission take decision on every aspect of conduct and control of elections.
2. It implements the code of conduct and can punish any candidate or party that violates it.
3. It controls the government officers who are rooted on the election duty.
4. During the election period, the Election Commission can order the govern-ment to follow some guidelines.

Question 17.
What are the measures taken by the Election Commission to ensure free and fair elections?
Answer:
The Commission takes many measures. Some of these are as follows:

1. It sends central observers to sensitive constituencies;
2. The Commission prescribes the code of conduct for the election campaign, and
3. The Commission creates conditions for a free and fair poll.

Question 18.
What factors affect citizens’ participation in electoral politics?
Answer:
The factors affecting citizens’ participation in electroal politics are:
1. The Literacy Factor:
A vast majority of voters may not be welleducated, but they have plenty of common sense and enthusiastically cast their vote.

2. Economic, Regional and Ethnic Factors:
Unemployment and poverty have made low-income groups conscious of their voting right. In western democracies, especially in the United States, the better educated and the rich, vote more than those having lower status, such as the blacks and the poor persons. In case of India, the situation is exactly opposite of that. The regional and ethnic factors considerably helped the DMK, ALA DMK, Shiv Sena, Akali Dal, Assam Gana Parishad and the Bahujan Samaj Party in India.

3. Participation of Women:
Women, as compared to men, are politically less informed but their participation has increased steadily election after election. The number of women candidates has also shown a rising trend. This is in contrast to western democracies where men vote more than women.

Question 19.
How has the interest of the voters in the election-related activities increased in recent years in India? Explain.
Answer:
The interest of the voters in the election related activities has been increasing over the years. During the 2004 election, more than one third voters took part in a campaign-related. activity. More than half of the people identify themselves as being close to one or the other political party. One out of every seven voters is a member of a political party.

Question 20.
“If elections are not free or fair, the outcome always favours the powerful.” Does the outcome of elections in India justify this statement?
Answer:
The outcome of elections in India speaks for itself:

1. The ruling parties routinely lose elections in India both at the national and state level. In fact, in every two out of the three elections held in the last 25 years, the ruling party lost. In India, about half of the sitting MPs or MLAs lose elections.
2. Candidates who are known to have spent a lot of money on ‘buying votes’ and those with known criminal connections, often lose elections.
3. Barring very few disputed elections, the electoral outcomes are usually accepted as ‘people’s verdict’ by the defeated party.

Question 21.
Explain any four challenges and limitation to free and fair elections in India.
Answer:
Following are the four challenges and limitation to free and fair elections in India:

1. Candidates and political parties with a lot of money enjoy a big and unfair advantage over smaller parties or independent candidates.
2. Candidates with criminal records have been able to push others out of electoral race and secure a ticket from major parties.
3. Some families tend to doominate political parties and secure tickes for their own family members.
   (iv) Smaller parties and independents suffer a huge disadvantage compared to bigger parties.

IV. Long Answer Type Questions

Question 1.
What are reserved constituSncies ? Why are they required?
Answer:
A true democracy provides equal opportunity to all the individuals and sections of the society to take part in elections, get elected and share the responsibility of gov-ernance of the country. In our country, the weaker section constitutes, by and large, Scheduled Castes (SCs), Scheduled Tribes (STs), Other Backward Castes (OBCs) and Women.

For elections to be meaningful, a level playing field is to be provided to both the weakers and the powerful sections of the society. With this in view, our constitution contains the provision of reserved constituency. By reserved constituency, we mean a constituency in which only a person belonging to that particular group can contest an election for which the seat has been rserved. For example, in a SC constituency, only a person belonging to one of the SCs can contest elections. Similarly, for a ST constituency, only a ST person can be the candidate.

Question 2.
Explain the entire election process.
Answer:
The entire election process can be briefy stated as follows:
1. Notification of Election Dates:
The elections process begins with the notifica¬tion of the election dates by the Election Commission.

2. Prepareation of Electoral Rolls:
Once the constituencies are decided the next step is to decide the voters who can vote. In a democratic elections, the list of those who are eligible to vote is prepared much before the elections. The list of eligible voters is known as Electoral Roll and is commonly known as Voters’ List.

3. Nomination of Candidates:
Political parties nominate their candidates who get the part}- symbol and support. Every person who wishes to contest an election has to file a ‘Nomination form’ and deposit some amount as security deposit.

4. Scrutinising of Nomination Papers:
The nomination papers are scrutinised on the fixed date. Incomplete or incorrect papers are rejected by the Commission.

5. Withdrawal of Nominations:
The candidates are given a date for withdrawal of their names after the withdrawal, the final list is printed. Election symbols are alloted to the candidates.

6. Election Campaign:
When the final list gets printed by the Returning Officer,
different political parties launch their election campaigns to canvass for their candidates. The political parties issue their election manifestos and propagate their programmes to the people through public meeting, processions, rallies, newspapers, booklets and radio etc.

7. Polling:
Public holiday is declared on the election day, so that each voter may exercise his/her vote.

8. Counting of Votes: After the election is over, the counting personnels are appointed by the Election Officer, who, at a fixed place and at the fixed time, start their counting work in the presence of the Returning Officer.

9. Declaration of Results: After the counting of votes is over, the election officer declares the candidate getting the maximum votes, elected.

10. Election Petitions:
After the declaration of election results, the defeated candidate of the constituency can file an election petition against the successful candidate within a fixed period to the concerned court or election tribunal. If the allegations are proved true, the court declares the election of the successful candidate invalid and it declares the defeated candidate as successful.

Question 3.
Why slogan are used by political parties? Mention any four successful slogans given by different political parties in various elections.
Answer:
In election campaign, various political parties use slogans to attract the voters and get them to vote for their party on that basis:
Following are the four successful slogans given by different political parties in various elections:
1. The congress party led by Indira Gandhi gave the slogan of Garibi Hatao (Remove poverty) in the Lok Sabha election of 1971. The party promised to reorient all the policies of the government to remove poverty from the country.

2. The Janata Party gave the slogan of Save Democracy in the Lok Sabha election of 1977, under the leadership of Jaya Prakash Narayan. The party promised to undo the atrocities committed during emergency and restore civil liberties.

3. The Left Front gave the slogan of land to the tiller in the West Bengal Assembly elections of 1977.

4. ‘Protect the Self Respect of the Telugus’ was the slogan used by N.T. Rama Rao, the leader of the Telugu Desam Party in Andhra Pradesh Assembly elections in 1983.

Question 4.
What maks elections in India democratic?
1. Independent Election Commission:
In our country the elections are conducted by an independent body, i.e., Election Commission. It enjoys same kind of independence that the judiciary enjoys. The Chief Election Commissioner is appointed by the President but once appointed he/she is not answerable to the President or the Government.

2. Popular Participation:
People’s participation in election is usually measures by voter turnout figures. Turnout indicates the percent of eligible voters who actually cast their vote. Over the last fifty years, the turnout in Europe and North America has declined. In India, the turnout has either remained stable or actually gone up.

3. Paticipation of Underprivileged:
In India, the poor, illiterate and under-privileged people vote in larger proportion as compared to the rich and privileged section. This is in contrast to western democracies.

4. Participation of People in Election-Related Activities:
It is not only the turnover and casting of votes but the people are also taking a lot of interest in election-related activities. According to a survey, one out of every seven voters is a member of a political party. During the general elections of 2004, more than one-third voters took part in campaign-related activities.

5. Acceptance of Election Outcome:
One final test of the free and fairness of election has in the outcome itself. If elections are not free and fair, the outcome
always favours the powerful ruling party. Usually, the ruling party does not accept the outcome of a rigged elections.

Question 5.
Mention the functions of Election Commission.
Answer:
1. Conduct of Elections:
The Election Commission is obliged to conduct all elec¬tions to the Parliament and State Assemblies. It also supervises and directs the elections to the office of the President and Vice-President of India.

2. Recognition of Political Parties:
The Election Commission grants recognition to political parties. For this purpose, it has evolved its own procedure.

3. Allotment of Symbols:
The Election Commission allots symbols to political parties and to independent candidates.

4. Ensuring Free and Fair Elections:
To ensure free and fair elections is one of the major functions of the Election Commission.

5. The Model Code of Conduct for Elections:
Another important function of the Election Commission is to prescribe a Model Code of Conduct for the candidates and political parties, which is ought to be followed by them.

6. Preparation of Electoral Rolls:
The Election Commission prepares electoral rolls and includes the names of the valid voters in the electoral rolls.

7. Appointment of Electoral Staff:
The Election Commission appoints Chief Electoral officers for every state and one Returning Officer for every constituency. These officers appoint several other officers to make arrangement for the elections.

Question 6.
State the limitations and challenges to the elections in India.
Answer:
The limitations and challenges to the elections in India are as follows:

1. Candidates and parties with a lot of money may not be sure of their victory, but they do enjoy a big and unfair advantage over smaller parties and independents.
2. In some parts of the country, candidates with criminal connection have been able to push others out of the electoral race and to secure a ‘ticket’ from major parties.
3. Some families tend to dominate political parties; tickets are distributed to relatives from these families.
4. Very often, elections offer little choice to ordinary citizens, for both the major parties are quite similar to each other, both in policies and practice.
5. Smaller parties and independent candidates suffer a huge disadvantage compared to bigger parties.
6. These deeper issues are matter of concern for those who believe in democracy. That is why, citizens, social activists and organisations have been demanding reforms in our electoral system.

JAC Class 9 Social Science Important Questions

JAC Board Class 9th Social Science Important Questions Civics Chapter 2 Constitutional Design

I. Objective Type Questions

1. On which island Nelson Mandela was kept in prison?
(a) Andman and Nicobar island
(b) Robben island
(c) Greenland island
(d) None of the above.
Answer:
(b) Robben island

2. The constitution of India was framed by:
(a) British Parliament
(b) Indian Parliament
(c) A Constituent Assembly
(d) All of these.
Answer:
(c) A Constituent Assembly

3. When was first meeting of the Consitituent Assembly held?
(a) 1946
(b) 1940
(c) 1935
(d) 1949.
Answer:
(a) 1946

4. Who was the Chairman of the Drafting Committee?
(a) Dr. Rajendra Prasad
(b) Dr. B.R. Ambedkar
(c) Mahatma Gandhi
(d) Jawaharlal Nehru.
Answer:
(b) Dr. B.R. Ambedkar

5. In 1931, who wrote about his expectation from the constitution in his magazine “Young India’?
(a) Jawaharlal Nehru
(b) Mahatma Gandhi
(c) Dr. B.R. Ambedkar
(d) Motilal Nehru.
Answer:
(b) Mahatma Gandhi

II. Very Short Answer Type Questions

Question 1.
Who said these words “I have fought against white domination and I have fought against black domination”?
Answer:
Nelson Mandela.

Question 2.
For what crime did the white government of South Africa prosecute Nelson Mandela?
Answer:
For the crime of demanding freedom.

Question 3.
Name the leader who led the struggle against racial discrimination?
Answer:
Nelson Mandela.

Question 4.
What is Apartheid?
Answer:
The official policy of racial separation and illtreatment of blacks followed by the government of South Africa between 1948 and 1989.

Question 5.
On the basis of what did the system of apartheid divide people?
Answer:
On the basis of skin colour, the system of apartheid divided people.

Question 6.
Name the umbrella organisation that led the struggle against the policies of segregation.
Answer:
African National Congress (ANC).

Question 7.
On which day did South Africa gain independence from the rule of the white minority?
Answer:
South Africa gained Independence from the rule of the white minority on 26th April, 1994.

Question 8.
Which constitution has inspired democrats all over the world?
Answer:
The constitution of South Africa has inspired democrats all over the world.

Question 9.
What is Constitution?
Answer:
The constitution is a fundamental legal document according to which the government of a country functions.

Question 10.
Which national leader tried to draft a constitution for India in 1928?
Answer:
The Constituent Assembly adopted the constitution on 20th November, 1949.

Question 11.
Define the Constituent Assembly.
Answer:
The drafting of the document called the constitution was done by an assembly of elected representatives called the Constituent Assembly.

Question 12.
Name the body which prepared the constitution of India.
Answer:
Constituent Assembly.

Question 13.
When were the elections to the Constituent Assembly held?
Answer:
The elections to the Constituent Assembly were held in July, 1946.

Question 14.
How many members of the Constituent Assembly wrote the Indian Constitution?
Answer:
299 members.

Question 15.
When did the Constituent Assembly adopt the constitution of India?
Answer:
The Constituent Assembly adopted the constitution on 26th November, 1949.

Question 16.
When was the Indian constitution came into effect?
Answer:
The Indian constitution come into effect on 26th January, 1950.

Question 17.
Who was the President of the Constituent Assembly?
Answer:
Dr. Rajendra Prasad.

Question 18.
Who was the Chariman of the Drafting Committee?
Answer:
Dr. Bhimrao Ramji Ambedkar.

Question 19.
What is meant by the term‘Preamble’?
Answer:
The Preamble is an introduction to the constitution which contains the ideals and . basic principles of the Indian Constitution.

Question 20.
Which part of the Indian constitution is called soul of the constitution?
Answer:
The preamble of the Indian constitution is called soul of the constitution.

Question 21.
Which guiding value of the constitution of India means people have the supreme right to make decision?
Answer:
Sovereign.

Question 22.
What is meant by the term ‘socialist’?
Answer:
The term ‘socialist’ in the contect of the Indian constitution means a person who believes that the wealth generated by society should be shared equally by all members of the society.

Question 23.
What is meant by declaring India as a Sovereign State?
Answer:
A sovereign state means that Indian people have supreme right to make decisions on internal as well as external matters. No external power can dictate the government of India.

Question 24.
“India is a secular country.” Justify.
Answer:
In India, there is no official religion. All citizens have the right to follow any religion. The Indian government treats all religious beliefs and practices with equal respect.

Question 25.
What proves that India is democratic? Give two points.
Answer:

1. India is democratic, as the government is elected by the people directly after every five years.
2. People enjoy equal political rights and hold their rulers accountable.

Question 26.
What is meant by the term ‘Republic’?
Answer:
If the head of the state is an elected person and not a hereditary position, then it is called as Republic.

Question 27.
Why is India called a Republic?
Answer:
India is called a Republic because the head of the state is an elected person and not from a hereditary position.

Question 28.
What does the term ‘liberty’ signify as mentioned in the preamble of the Indian constitution?
Answer:
It signifies that the citizens are free to express their opinions and there are no unreasonable restrictions on their liberty.

Question 29.
What do you mean by constitutional amendment?
Answer:
A change in the constitution made by the supreme legislative body in the country is known as constitutional amendment.

III. Short Answer Type Questions

Question 1.
What was the ‘Policy of Apartheid’? Explain.
Or
What do you understand by the ‘Policy of Apartheid’? How did this policy come to an end?
Answer:
Policy of Apartheid: The policy of apartheid was a system of racial discrimination unique to South Africa. It was imposed on the black by the white Europeans. In this system, the blacks were forbidden from living in white areas. They could work in white areas only if they had a permit.

Trains, buses, taxis, hotels, hospitals, schools, colleges, libraries, cinema halls, theatres, beaches, swimming pools, public toilets, etc. were all separate for the whites and the blacks. This policy came to an end on 26th April, 1994, when the Republic of South Africa was bom with a multifacial government.

Question 2.
“The partheid system in South Africa was particularly oppressive for the blacks”. Justify the statement with arguments.
Answer:
The following arguments are presented in support of this statement:

1. The blacks were forbidden from living in white areas. They could work in white areas only if they had a permit.
2. Trains, buses, taxis, hotels, hospitals, schools and colleges, libraries, cinema halls, theatres, beaches, swimming pools, public toilets, churchs, etc. were all separate for the whites and blacks.
3. Blacks could not form associations or protest against their terrible treatment.

Question 3.
Explain any three efforts made by the people of South Africa to struggle against the apartheid system :
Answer:
The following three efforts were made by the people of South Africa to struggle against the apartheid system.

1. From 1950 onwards, the blacks, coloured and Indians fought against the apartheid system. They launched protest marches and strikes.
2. The African national congress led the struggle against this policy of segregation. Many workers’ unions and the Communist Party joined this movement.
3. Even many sensitive whites joined the African National Congress and played a leading role in this movement.

Question 4.
Explain the role of Nelson Mandela in the struggle against apartheid.

1. Nelson Mandela was one of the most able, efficient and far-sighted leaders of the African National Congress (ANC).
2. It was under his leadership, that the struggle against apartheid reached its climax.
3. Due to the participation in the movement against apartheid, he was sentenced to life imprisonment in 1964. He spent the next 28 years in prison.
4. In the 90’s due to activities of the ANC and the support it got from other countries of the world, South African regime was completely isolated from the world.
5. In order to create a favourable atmosphere, Nelson Mandela was released in 1990.
6. In 1994, the first democratic elections were held and Nelson Mandela was elected the President of South Africa.

Question 5.
South Africans call themselves a ‘rainbow nation’. Can you guess why?
Answer:
South Africans call themselves a ‘rainbow nation’ because there are whites, blacks, coloured people and Indians in South Africa who have different skin colours. But they are living and working together as one people, unified like the colours of a rainbow.

Question 6.
What is the constitution? What does it explain?
Answer:
The constitution of a country is a set of written rules that are accepted by all people living together in a country. Constitution is the supreme law that determines the relationship among citizens and also the relationship between the people and government. The constitution explains:

1. the organisation and structures of the three organs of the government, namely, the Legislature, the Executive and the Judiciary.
2. their powers and functions.
3. their mutual relations.
4. their limitations, and
5. the rights and duties of the citizens.

Question 7.
What functions does the constitution perform?
Answer:
The constitution performs the following functions:

1. It generates a degree of trust and cordination that is necessary for different kind of people to like together.
2. It specifies how the government will be constituted, who will have power to take which decisions.
3. It lay down limits on the powers of the government and tells us what the right of the citizens are.
4. It expresses the aspirations of the people about creating a good society.

Question 8.
‘Formation of Indian constitution was no less difficult than that of South Africa.’ Do you agree ? Explain with five arguments. What were the difficulties faced during the making of Indian Constitution?
Answer:
The difficulties faced during the making of Indian constitution were as follows:

1. The making of the constitution for a huge and diverse country like India was not an easy task.
2. At that time, the people of India were emerging from the states of subjects to that of citizens.
3. The country was bom through a partition on the basis of religious differences.
4. The British had left it to the rulers of the princely states to decide whether they wanted to merge with India or with Pakistan or remain independent. The merger of these princely states was a difficult and uncertain task.
5. When the constitution was being written, the future of the country did not look as secure as it does today.

Question 9.
“The Constituent Assembly was a galaxy of outstanding leaders”. Explain.
Answer:
It was India’s good fortune that when it emerged as an independent country, it had the benefit of a galaxy of outstanding leaders. Some of these leaders, who were elected to the Constituent Assembly, were fully conscious about the enormity of the task before them.

Great men and women from different communities were entrusted with the momentous task of drafting the constitution of free India. They came to the Constituent Assembly from all parts of the country, making it a miniature India. The Assembly was dominated by the Indian National Congress but with leaders having different opinions.

Question 10.
What was the procedure adopted for making the Indian constitution ?
Answer:
The ‘Drafting Committee’ chaired by Dr. B. R. Ambedkar prepared a draft of the Indian constitution for discussion.
Several rounds of discussion took place on the draft constitution clause by clause. The members worked for 114 days spread over three years. More than two thousand amendments were considered. Every document presented and every work spoken in the Constituent Assembly has been recorded and preserved. These are called Constituent Assembly Debates.

Question 11.
“The working of the Constituent Assembly has given sanctity to the constitution.”Explain.
Answer:

1. The Constituent Assembly worked in a systematic, open and consensual manner.
2. First, some basic principles were decided and agreed upon. Then a drafting committee prepared a Draft Constitution for discussion.
3. Several rounds of through discussion took place on the Draft Constitution, clause by clause. More than two thousand amendments were considered. The members deliberated for 114 days, spread over three years.
4. Every document presented and every word spoken in the Constituent Assembly has been recorded and preserved. These are called the ‘Constituent Assembly Debates’.
5. Though the Constituent Assembly was not directly elected by the people, its sessions were open to the press and the people.

Question 12.
What is Preamble to our constitution? What is its importance.
Answer:
The Preamble contains the ideals and basic principles of the Indian Constitution. The Preamble is not a part of the constitution, but it has been described as ‘Key to the Constitution.’ It is not enforceable by the court of law. No one can go to the court and say that the Preamble has not been enforced by the government. Still, the preamble serves as the guiding light of the constitution. Importance of Preamble:

1. It contains the philosophy on which the entire constitution has been built.
2. It provides a standard to examine and evaluate any law and action of the government, to find out whether it is good or bad. It is the soul of the Indian constitution.

Question 13.
“Indian Constitution is both rigid and flexible.” Explain.
Answer:
Usually the federal constitutions are rigid, but our constitution presents a mixture of flexibility and rigidity. Some parts of it can be amended by parliament by a simple majority, while other parts are less flexible. They require, for their amendment, a special majority, that is two-thirds of the members of both Houses should vote for that legislation. Then there are Articles which fall under the third category. They require for their amendment a two-third majority of both Houses plus ratification by the legislatures of not less than one-half of the States.

III. Long Answer Type Questions

Question 1.
Explain the struggle of the South African People against apartheid.
Answer:
The policy of’apartheid was imposed by the whites over the blacks. Under this, people of South Africa were discriminated on the basis of colour and race.

1. The African National Congress (ANC) was the umbrella organisation that led the struggle against the policies of segregation. This included many workers’ unions and the Communist Party.
2. Many sensitive whites also joined the ANC to oppose apartheid, and played a leading role in this struggle.
3. Several countries including India denounced apartheid as unjust and racist, but the white racist government continued to rule by detaining, torturing and killing thousands of blacks and coloured people.
4. As protests and struggles against apartheid increased, the government realised that they could no longer keep the blacks under their rule through repression.
5. The white regime changed its policies. Discriminatory laws were repealed. Ban on political parties and restrictions on the media were lifted. After 28 years of imprisonment, Nelson Mandela walked out of the jail as a free man. Finally, at the midnight of 26th April, 1994, the new national flag of the Republic of South Africa was unfurled, making it a newly born democracy in the world.

Question 2.
How did the white minority and the black majority agree to draw up a common constitution for South Africa?
Answer:

1. Finally, at the midnight of 26th April, 1994 the new national flag of the Republic of South Africa was unfurled making the newly born democracy in the world.
2. After the emergence of the new democratic South Africa, black leaders appealed to fellow blacks to forgive the whites for the atrocities they had committed while in power. They said let us build a new South Africa based on equality of all races and men and women, on democratic values, social justice and human rights.
3. The party that ruled through oppression and brutal killings and the party that led the freedom struggle set together to draw up a common constitution.
4. After two years of discussion and debate, they came out with one of the finest constitution the world has ever had.
5. The new constitution gave to its citizens the most extensive rights available in any country.

Question 3.
What is a constitution? Why do we need a constitution?
Answer:
Constitution:
The constitution of a country is a set of written rules that are accepted by all people living together in a country. Constitution is the supreme law that determines the relationship among people living in a territory (called citizens) and also the relationship between the people and government.
Need of Constitution: We need a constitution because:

1. It generates a degree of trust and coordination that is necessary for different kinds of people to live together.
2. It specifies how the government will be constituted and who will have power to take which decisions.
3. It lays down limits to the powers of the government and tells us what are the rights of the citizens.
4. It expresses the aspirations of the people about creating a good society.
5. It grants universal adult franchise to all and establishes equality for all.
6. The Indian constitution safeguards the interests of minorities, the backward classes, poor and weaker sections of our society.

Question 4.
Explain major difficulties faced by the makers of the Indian Constitution.
Answer:
The following were the difficulties faced by the makers of the Indian Constitution:

1. The Indian people were emerging as free citizens from their earlier status as subjects. The constitution had to ensure that the people were given certain fundamental rights, which had not been provided earlier.
2. The country was born through a partition on the basis of religious differences. This was a traumetic experience for the people. At least 10 Lakh people were killed on the both sides of the border in partition-related violence.
3. The Britishers had left it to the princely states to decide whether to join either country or remain independent. This problem had to be kept in mind while framing the constitution.
4. India was a vast and varied country with diverse languages, religions, culture, customs and beliefs. This factor was a barrier in framing the constitution.
5. The makers of the Indian constitution had to ensure that the country remained united after the constitution came into force. This was another difficulty faced while framing the constitution.

Question 5.
Highlight the constitution assembly.
Answer:
The constitution of our country was framed by the constituent assembly which was constituted in 1946. This assembly originally had 389 members. Of them, most were elected by the provincial assemblies and some were nominated by the rulers of the native states. The first meeting of the constituent assembly was held in December, 1946.

Soon after, the country was divided into India and Pakistan. The Constituent As¬sembly was also divided into the Constituent Assembly of India and that of Pakistan. The Constituent Assembly that wrote the Indian constitution had 299 members. The assembly adopted the constitution on 26 November 1949 but it came into effect on 26 January 1950. To mark this day, we celebrate January 26 as Republic Day every year.

The Constituent Assembly included eminent persons, like Dr. Rajendra Prasad, Pt. Jawaharlal Nehru, Sardar Vallabhbhai Patel, Dr. B. R. Ambedkar, Jaipal Singh, Baldev Singh, Shyama Prasad Mukheijee, Somnath Lahiri, C. Rajagopalachari and others. Women members, like Sarojini Naidu and C. Durgabai Deshmukh also played an important role in the constituent assembly.

Question 6.
Describe any five major factors which contributed to making of Indian Constitution.
Answer:
The following were the major factors which contributed to the making of Indian Constitution:

1. At that time, people of India were emerging from the status of subjects to that of citizens. They had become conscious of their rights.
2. Our leaders gained confidence to learn from other countries, but on our own terms. Many of them were inspired by the ideals of the French Revolution.
3. They were also influenced by the practices of parliamentary democracy in Britain and the Bill of Rights in the United States of America.
4. Social Revolution in Russia inspired many Indians to think of shaping a system based on social and economic equality.
5. The makers of the constitution were not simply coping the constitution of other countries.
   At each step, they were questioning whether the values and ideals they accepted, suited or not suited to our country. We have modified those provisions to suit our needs.

Question 7.
Explain in detail the steps involved in the framing of Indian constitution.
Answer:
The drafting of the constitution was done by an assembly of elected representatives called the constituent assembly. Following were the steps involved in the framing of Indian constitution:
1. Elections to the Constituent Assembly were held in July 1946 and its first meeting was held in December 1946.

2. After partition, the Constituent Assembly was also divided into the Constituent Assembly of India and that of Pakistan.

3. The Constituent Assembly that wrote the Indian constitution had 299 members. The constitution does not only reflect the views of its members, but it also expresses a broad consensus of its time.

4. The Constituent Assembly represented the people of India, i.e., members from different language groups, castes, classes, religions and occupations.
Thus, the constitution written by the constituent assembly covered the interests of the diverse population of our country.

5. Finally, the manner in which the Constituent Assembly worked provided sanctity to the constitution. They worked in a systematic, open and consensual manner. drafting committee chaired by Dr. Bhimrao Ambedkar prepared a draft constitution. Several rounds of thorough discussion took place.

The members deliberated for 114 days, spread over almost three years. Every document was recorded and preserved. These are called as ‘Constituent Assembly Debate’ and are printed in 12 volumes. These are used to interpret the true meaning of the constitution.

6. The assembly adopted the constitution on 26th November, 1949 and it came into effect on 26th January, 1950. To mark this day we celebrate 26th January as the Republic Day of India every year.

Question 8.
Why should we accept the constitution made by Constituent Assembly more than six decades ago? Explain.
Answer:
Due to following reasons, we accept the constitution made by the Constituent Assembly more than six decades ago.
1. The constitution of India does not reflect the view of its members alone. It expresses a broad consensus of its time. Many countries of the world have had. to rewrite their constitution afresh because the basic rules were not acceptable to al1 major social groups or political parties. In some other countries, the cons tution exists as a mere piece of paper.

No one actually follows it. The expe ence of our constitution is different. Over the last half century, several grouj have questioned some provisions of the constitution. But no large social group or political party has ever questioned the legitimacy of the constitution itself. This is an unusual achievement for our constitution.

2. The constituent assembly represented the people of India. It was elected mainly by the members of the existing provincial legislatures. This ensured a fair geographical share of members from all the regions of the country. In social terms too, the assembly represented members from different language groups, castes, classes, religions and occupations.

3. The constituent assembly worked in a systematic, open and consensual manner. First, some basic principles were decided and agreed upon. Then a drafting committee chaired by Dr.B. R. Amebedkar prepared a draft constitution for discussion. Several rounds of thorough discussion took place on the draft constitution, clause by clause.

The members worked for 114 days spread over three years. More than two thousand amendments were considered. Every document presented and every word spoken in the constituent assembly has been recorded and preserved. These debates provide the rationale behind every provision of the constitution. These are used to interpret the meaning of the constitution.

Question 9.
Explain the meaning of each key words of the Preamble.
Answer:
1. We the people of India:
The constitution has been drawn up and enacted by the people through their representatives and not handed down to them by a king or any outside power.

2. Sovereign:
People have supreme right to make decisions on internal as well as external matters. No external power can dictate the Government of India.

3. Socialist:
Wealth is generated socially and should be shared equally by society. The government should regulate the owemship of land and industry to reduce socio-economic inequalities.

4. Secular:
Citizens have complete freedom to follow any religion, but there is no official religion. The government treats all religious belief and practices with equal respect.

5. Democratic:
A form of government where people enjoy equal political rights, elect their rulers and hold them accountable. The government runs according to some basic rules.

6. Republic:
The Head of the State is an elected person and he does not come from a heriditary position.

7. Justice:
Citizens cannot be discriminated on the grounds of caste. The government should work for the welfare of all, especially of the disadvantaged groups.

8. Liberty:
There are no unreasonable restrictions on the citizens in what they think, how they wish to express their thoughts and the way they wish to follow up their thoughts in action.

9. Equality:
All are equal before the law. The traditional social inequalities have to be ended. The government should ensure equal opportunity for all.

10. Fraternity:
All of us should live like members of the same family. No one should treat a fellow citizen as inferior.

JAC Class 9 Social Science Important Questions

JAC Board Class 9th Science Important Questions Chapter 9 Force and Laws of Motion

Multiple Choice Questions

Question 1.
The SI unit of force is
(a) kg m/s
(b) newton
(c) dyne
(d) kg wt
Answer:
(b) newton

Question 2.
The combined effect of mass and velocity is taken into account by a physical quantity called
(a) torque
(b) momentum
(c) moment
(d) force
Answer:
(b) momentum

Question 3.
Quantitative definition of force is given by
(a) Newton’s first law of motion
(b) Newton’s second law of motion
(c) Newton’s third law of motion
(d) Newton’s law of gravitation
Answer:
(b) Newton’s second law of motion

Question 4.
Momentum gives a measure of
(a) mass
(b) weight
(c) velocity
(d) motion
Answer:
(d) motion

Question 5.
An athlete runs some distance before taking a long jump because
(a) he gains energy to take himself through long distance
(b) it helps him to apply a larger force
(c) by running, action and reaction forces increase
(d) by running, the athlete gives himself larger inertia of motion
Answer:
(d) by running, the athlete gives himself larger inertia of motion

Question 6.
Rocket works on the principle of conservation of
(a) velocity
(b) energy
(c) linear momentum
(d) mass
Answer:
(c) linear momentum

Question 7.
The rate of change of momentum of an object is proportional to the
(a) mass of the body
(b) velocity of the body
(c) net force applied on the body
(d) none of these
Answer:
(c) net force applied on the body

Question 8.
A force of 50 N moves a body. Which of the following is correct?
(a) Frictional force exerted on the body is less than 50N
(b) Frictional force exerted on the body is more than 50N
(c) None of these
(d) Both (a) and (b)
Answer:
(a) Frictional force exerted on the body is less than 50N

Question 9.
If a football and a stone have same mass, then both will have
(a) same inertia
(b) same momentum
(c) different inertia
(d) different momentum
Answer:
(a) same inertia

Question 10.
The rate of change of momentum w. r. t time is measured in
(a) kg m
(b) kg
(c) kg ms^-1
(d) kg ms^-1
Answer:
(d) kg ms^-1

Question 11.
A block of mass M is pulled with a force F along a smooth horizontal surface with a rope of mass m. The acceleration of the block will be
(a) F/M
(b) F/m
(c) F/(M + m)
(d)F/(M – m)
Answer:
(c) F/(M + m)

Question 12.
Action and reaction forces act on two
(a) different objects
(b) same objects
(c) either (a) or (b)
(d) none of these
Answer:
(a) different objects

Question 13.
A man is standing in a boat in still water. If he tries to walk towards the shore, the boat will
(a) move away from the shore
(b) remain stationary
(c) sink
(d) move towards the shore
Answer:
(a) move away from the shore

Question 14.
When an object undergoes acceleration
(a) its speed always increases
(b) its velocity always increases
(c) it always falls towards the earth
(d) a force always acts on it
Answer:
(d) a force always acts on it

Question 15.
On applying a constant force to a body, it moves with uniform
(a) momentum
(b) speed
(c) acceleration
(d) velocity
Answer:
(c) acceleration

Analysing & Evaluating Questions

Question 16.
A goalkeeper in the game of football pulls his hands backwards after holding the ball shot at the goal. This enables the goal keeper to
(a) exert larger force on the ball
(b) reduce the force exerted by the ball on hands
(c) increase the rate of change of momentum
(d) decrease the rate of change of momentum
Answer:
(b) reduce the force exerted by the ball on hands

Question 17.
A passenger in a moving train tosses a coin which falls behind him. It means that motion of the train is
(a) accelerated
(b) uniform
(c) retarded
(d) along circular tracks
Answer:
(a) accelerated

Question 18.
An object of mass 2kg is sliding with a constant velocity of 4 ms^-1 on a frictionless horizontal table. The force required to keep the object moving with the same velocity is
(a) 32 N
(b) 0 N
(c) 2 N
(d) 8 N
Answer:
(b) 0 N

Assertion Reason Questions

Directions: In the following questions, the Assertions and the Reasons have been put forward Read the statements carefully and choose the correct alternative from the following:
(A) Both the assertion and the reason are correct and the reason is the correct explanation of the assertion.
(B) The assertion and the reason are correct but the reason is not the correct explanation of the assertion.
(C) The assertion is true but the reason is false.
(D) Both statements are false.
1. Assertion: A brick has more inertia than a hollow wooden block of the same shape and size.
Reason: Heavier the body, more is the inertia.
Answer:
(A) Both the assertion and the reason are correct and the reason is the correct explanation of the assertion.

2. Assertion: A body falling towards the earth also pulls the earth towards itself.
Reason: The forces always occur in pairs.
Answer:
(A) Both the assertion and the reason are correct and the reason is the correct explanation of the assertion.

3. Assertion: When a bus starts, the person inside it falls forward.
Reason: The bus pushes the person forward.
Answer:
(D) Both statements are false.

4. Assertion: The third law of motion defines acceleration.
Reason: The third law of motion states that the net force experienced by a body is proportional to the rate of change in momentum of the body.
Answer:
(D) Both statements are false.

5. Assertion: Action and reaction forces are always equal and opposite.
Reason: Action and reaction forces cancel each other.
Answer:
(C) The assertion is true but the reason is false.

Very Short Answer Type Questions

Question 1.
Define force.
Answer:
Force may be defined as a push or a pull which changes or tends to change the state of rest or of uniform motion or direction of motion of a body.

Question 2.
Define one Newton.
Answer:
One newton is the force which produces an acceleration of m/s² in an object of mass 1kg.
1N = 1kg m/s²

Question 3.
State the various effects of force.
Answer:
A force applied on an object can do five things:
(a) It can change the speed of the object.
(b) It can change the direction of motion of the object.
(c) It can change the shape and size of the object.
(d) It can set an object at rest in motion.
(e) It can bring a moving object to rest.

Question 4.
Define resultant force.
Answer:
The resultant force or resultant of several forces acting simultaneously on a body is that single force which produces the same effect on a body as all these forces produce together.

Question 5.
What is frictional force?
Answer:
The force that always opposes the motion of an object is called force of friction.

Question 6.
What is inertia?
Answer:
The natural tendency of an object to resist a change in their state of rest or of uniform motion along a straight line is called inertia.

Question 7.
State Newton’s first law of motion.
Answer:
An object remains in its state of rest or of uniform motion in a straight line unless acted upon by an external unbalanced force.

Question 8.
Place a water – filled tumbler on a tray. Hold the tray and turn around as fast as you can. We observe that the water spills. Why?
Answer:
The water spills when the tray turns around fastly, because initially, the water filled in the tumbler placed on the tray was in a state of rest. While turning, we apply force on the tray which starts moving but the water remains at rest due to its inertia, and hence, it spills out of the tumbler.

Question 9.
State Newton’s second law of motion.
Answer:
The rate of change of momentum of an object is proportional to the applied unbalanced force in the direction of the force.

Question 10.
State Newton’s third law of motion.
Answer:
To every action, there is an equal and opposite reaction and they act on two different bodies.

Question 11.
Why are shockers used in cars, scooters and motorcycles?
Answer:
Due to the shockers, the time interval of the jerk increases. As the rate of change of momentum will be smaller, comparatively less force acts on the passengers during the jerks.

Question 12.
Why is glass or chinaware packed with straw?
Answer:
The straw paper between the chinaware increases the time of experiencing the jerk during transportation. Hence, they strike against each other with a less force and are less likely to be damaged.

Question 13.
A bird hit the windscreen of a fast moving car and fell on the bonnet. Which of the two, the car or the bird, suffers greater change in momentum?
Answer:
By the law of conservation of momentum, both the car and the bird suffered equal and opposite change in momentum.

Question 14.
What decides the rate of change of momentum of an object?
Answer:
The rate of change of momentum of an object is proportional to the applied unbalanced force in the direction of force.

Question 15.
The diagram shows a moving truck. Forces A, B, C and D are acting on the truck. Name the type of each of these forces acting on the truck.

Answer:
The forces A, B, C and D acting on the truck are:
A driving force
B normal reaction
C frictional force
D weight gravitational force.

Analysing & Evaluating Questions

Question 16.
Two identical bullets are fired, one by a light rifle and another by a heavy rifle, with the same force. Which rifle will hurt the shoulder more and why?
Answer:
The velocity of recoil will be higher for ligher rifle. So lighter rifle will hurt the shoulder more.

Question 17.
When a fast moving carrom striker hits the carrom coin at the bottom of a pile, ¡t moves out without disturbing
the pile. Which kind of inertia of the pile is responsible for it?
Answer:
Inertia of rest of the pile is responsible for it.

Question 18.
There are three solid balls made up of aluminium, steel and wood, of the same shape and same volume. Which of them would have the highest inertia?
Answer:
Steel ball will have the highest inertia This is because the density of steel is higher than aluminium and wood As a result, the steel ball will have the highest mass and hence highest inertia

Short Answer Type Questions

Question 1.
Differentiate between balanced and unbalanced forces.
Answer:

Balanced forces	Balanced forces
1. The resultant of balanced forces is zero.	1. The resultant of balanced forces is zero.
2. These forces donot produce anyacceleration in the body.	2. These forces donot produce anyacceleration in the body.
3. Example: A book kept on the table (the downward force of gravity on the book is balanced by the normal reaction by the table on the book in upward direction).	3. Example: When the book that is kept on the table is pushed to the left side (the book moves as the push towards left is greater than the frictional force acting towards right).

Question 2.
Give examples to show that balanced forces can change the shape of an object.
Answer:
Consider a spring attached to a rigid support at one of its ends, as shown in the figure.

If we pull the free ends of the spring, it gets elongated Thus, on applying force, a spring expands. Similarly, if we hold a rubber ball between our palms and push the two palms against each other, we find that the ball is no longer round but oblong. The force exerted on the ball changes its shape, as shown in the figure. In both cases, the two forces, being equal and opposite, balance each other but change the shape of the object.

Question 3.
A football player kicks the ball which travels in the air for a while and lands on the ground where the ball travels on the ground for a short distance and then stops. What may be the reason behind it?
Answer:
The reason is frictional force. There is always a contact between the ball and the ground The opposing force always acts against the motion of the ball, thereby stopping the ball.

Question 4.
A table is moved across the floor with a constant velocity where the horizontal force acting on it is 400N. What will be the frictional force that will be acting on the table?
Answer:
According to Newton’s third law of motion, for every action there will be an equal and opposite reaction. The net force acting on the table is zero because the table moves with constant velocity in forward direction. Hence, an equal amount of frictional force must act in opposite direction as that of the table, i.e., F = – 400N.

Question 5.
When the car, we are moving in, makes a sharp turn at a very high speed, we tend to get thrown to one side. Explain the statement.
Answer:
The reason behind this is the law of inertia While moving in a car along a straight line, we tend to maintain our state of motion. But when the car takes a sharp turn, our bodies resist the change in direction and are thrown to the opposite side due to inertia.

Question 6.
Athletes always have a special posture by resting their right foot on a solid support, why?
Answer:
During the race, athletes have to run the heats and they rest their right foot on the solid support before the start, so that this support can give them a lot of force during the start of the race. They push the support backwards and get an equal and opposite forward push to get a very good start.

Question 7.
The safety belts in the car help in preventing accidents. Justify the statement.
Yes, safety belts help in preventing accidents. When a car is moving with a high speed, our body tends to be in movement due to inertia of motion in the forward direction. So when there is a sudden  collision, serious injuries can happen. However, seat belts exert a force on our body to slow down the forward motion and hence prevent injuries.

Question 8.
A karate player breaks the pile of tiles with a single blow. Give reason.
Answer:
A karate player strikes the pile of tiles by applying a very large velocity. The overall momentum of fast moving hand is reduced to zero in a very short interval. So this increases the rate of change of momentum which increases the force on the tiles and they eventually break.

Question 9.
Athletes are made to fall on a sand bed while performing a high jump. Give reason.
Answer:
During the high jump event, an athlete is made to fall on the sand bed because it increases the time to attain the rest
position. We know that, F = \(\frac{m(v-u)}{t}\)
So, force and time are inversely proportional to each other. If there is an increase in time, there is a decrease in rate of change of momentum and therefore the force or the impact is reduced This prevents injuries to the feet of athlete.

Question 10.
Explain the law of conservation of momentum.
Answer:
According to the law of conservation of momentum, the total momentum of the objects before collision is equal to the total momentum after collision, provided there is no external unbalanced force acting on them.

Question 11.
A bullet of mass 20g moving with a speed of 500 ms^-2 strikes a wooden block of mass 1kg and gets embedded in it. Find the speed with which the block moves along with the bullet.
Answer:
Let the final velocity of the block along with the bullet embedded in it be v.
For bullet, m₁ = 20g = 0.02 kg,
U₁ = 500 m/s, v₁ = v
For block, m₁ = 1kg, u₂= 0, v₂ = v
According to the law of conservation of momentum,
Total momentum before collision = Total momentum after collision
m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂
m₁u₁ + m₂u₂= (m₁ + m₂) v
v = \(\frac{m_{1} u_{1}+m_{2} u_{2}}{m_{1}+m_{2}}\)
= \(\frac{(0.02 \times 500)+(1 \times 0)}{(0.02+1)}\)
=\(\frac{10}{1.02}\) = \(\frac{1000}{102}\)
= 9.8 m/s.

Analysing & Evaluating Questions

Question 12.
Two balls of the same size but of different materials, rubber and iron, are kept on the smooth floor of a moving train. The brakes are applied suddenly to stop the train. Will the balls start rolling? If so, in which direction? Will they move with the same speed?

Answer:
The balls will start rolling in the forward direction. No. The two balls will move with different speed This is because the inertia of the two balls is not the same.

Question 13.
Two forces F₁ = 40N and F₂ = 60N are acting on an object as shown in the figure.

1. What is the net force acting on the object?
2. What is the direction of the net force acting on the object?
3. How much extra force is acting on the object if the object is not moving due to the application of these two forces? Name that force. Where does that force act and what is the direction of that force?
Answer:

1. Net force on the object = 60N – 40N = 20N
2. Net force of 20N acts in the direction of F₂ (i.e., towards left)
3. A force equal to 20N is acting on the object.
   This force is the frictional force. This force acts in the direction of F₁

Long Answer Type Questions

Question 1.
Describe Galileo’s experiments with inclined planes and state their conclusion.
Answer:
By observing the motion of objects on inclined planes, Galileo deduced that objects move with constant speed when no force acts on them.

1. In the first experiment, Galileo studied the motion of marbles on an inclined plane. He observed that when a marble rolls down an inclined plane, its velocity increases, as shown in the figure A.
2. Here the marble falls under the unbalanced force of gravity. The velocity of the marble decreases when it rolls up the inclined plane (against the force of gravity), as shown in the figure B.
3. From these observations, Galileo argued that the velocity of a marble rolling on a flat horizontal surface should remain constant. To test his idea, Galileo used double inclined planes.
4. He observed that when the inclinations of the planes on both sides were equal, the marble rolled down from one plane from a certain height will climb up to the same height on the other plane.
   
   side plane were gradually decreased, the marble would travel larger and larger distances to reach the same height.
5. Ultimately, if the right side plane were made horizontal, the marble would continue to travel forever to reach the same height from which it was released No unbalanced force acts on the marble in this case.
6. The above experiment suggests that an unbalanced force (external force) is required to change the motion of an object while no unbalanced force is needed to keep an object moving with a constant velocity.
7. In actual practice, the bodies stop due to the force of friction which always acts opposite to the direction of motion.

Question 2.
Mathematically explain the concept of conservation of momentum during collision between two bodies.
Answer:
Let us consider two bodies, viz., A and B, moving with initial velocities u_A and u_B respectively, such that u_A > u_B.
Let mass of body A be m_A and that of B be m_B.
Before collision
For body A, momentum = m_Au_A
For bodv B. momentum = m_Bu_B

After collision, the bodies A and B move with velocities, say, v_A and v_B respectively. Let the collision occur for a time, say, t.
After collision
For body A, momentum = m_Av_A
For body B, momentum = m_Bv_B
The rate of change of momentum for the body A = \(\frac{m_{A}\left(v_{A}-u_{A}\right)}{t}\)
The rate of change of momentum for the body A = \(\frac{m_{B}\left(v_{B}-u_{B}\right)}{t}\)

According to Newton’s third law of motion, The force F_AB exerted by ball A on ball B and the force F_BA exerted by the ball B on ball A must be equal and opposite to each other. Therefore
\(\left\{\frac{m_{A}\left(v_{A}-u_{A}\right)}{t}=-\left(\frac{m_{B}\left(v_{B}-u_{B}\right)}{t}\right)\right\}\)
m_Au_A + m_Bu_B= m_Av_A + m_Bv_B
Here, (m_Au_A + m_Bu_A) is the total momentum of the two bodies, A and B, before collision and (m_Av_A+ m_Bv_B) is the total momentum of the two bodies, A and B, after collision. So, the total momentum of the two bodies remains constant during collision, when no external force acts on them.

Question 3.
Give some examples of Newton’s third law of motion.
Answer:
Newton’s third law of motion states that: For every action, there is always an equal and opposite reaction acting on two different bodies.
Examples:

1. The person who is swimming, pushes the water backward (action), while water pushes the person forward (reaction).
2. When a bullet is fired from a gun, the gun exerts a force on the bullet (action), the bullet exerts an equal and opposite force on the gun (reaction),
3. When a rower jumps out of the boat in the forward direction (action), the boat moves backward (reaction).

Question 4.
Define the term ‘inertia’. Explain its types and state some of its examples in daily life.
Answer:

1. The tendency of a body to resist change in its state of rest or of uniform motion is called inertia.
   • Inertia of rest: The ability of a body to resist any change in its state of rest.
   • Inertia of motion: The ability of a body to resist any change in its state of uniform motion.
   • Inertia of direction: The ability of a body to resist any change in its direction of motion.
2. Examples of application of inertia in our day to day life are as follows:
   • When a carpet is beaten with a stick, the dust falls down.
   • When the car applies a sudden brake, we tend to fall forward.
   • When a bus suddenly takes a turn, we fall in the direction opposite to the turn.

Question 5.
Mathematically explain the concept of Newton’s second law of motion and also explain its importance in sports.
Answer:
Newton’s second law of motion: The rate of change of momentum of an object is directly proportional to the applied unbalanced force and it acts in the direction of force.

Example: Let us consider an object of mass (m), moving along a straight line with an initial velocity (u). Suppose, it attains a final velocity (v) in time (t).
Initial momentum of the object
= p₁ = mu
Final momentum of the object
= p₂ = mv
The change in momentum
= p₂ – p₁ = (mv) – (mu) = m (v – u)
The rate of change in momentum
= \(\frac{\mathrm{m}(\mathrm{v}-\mathrm{u})}{\mathrm{t}}\)
Therefore,
The applied force, F is
F ∝ \(\frac{\mathrm{m}(\mathrm{v}-\mathrm{u})}{\mathrm{t}}\)
F = \(\frac{\mathrm{mk}(\mathrm{v}-\mathrm{u})}{\mathrm{t}}\) …………..(i)
(where k is proportionality constant)
From the first equation of motion,
v = u + at
Substituting (ii) in (i),
So, F = kma
The unit of force is so chosen that the value of k becomes one.
Hence, F = kma or
F = ma
The second law is related to sports in the following examples:
(a) In a cricket match, a fielder who is trying to attempt a catch, generally pulls his hands along the moving ball because it consumes time to bring the momentum of the ball to zero. This decreases the rate change of mometum, and hence the force. So, when the time is increased, the overall force reduces, thus saving the hand from getting injured.

(b) In a high jump athletic event, the athletes are made to fall on a sand bed to increase the time of the athlete’s fall to stop after making the jump.
This decreases the rate of change of momentum and hence the force,

(c) A karate expert breaks a slab of ice with a single blow as he moves his hand very fast and then stops it in a very short time. Since the rate of change of momentum increases, the force also increases and the ice slab breaks.

Analysing & Evaluating Questions

Question 6.
A test tube containing little water is corked and suspended from two strings in a slanting position. Afterwards, the test tube is heated Answer the following:

1. What happens to the cork?
2. In which direction does the test tube move?
3. Compare the velocities of the cork and of the recoiling test tube.
Answer:

1. On heating, the water inside the test tube vaporises and the cork is blown out.
2. The test tube moves in the direction opposite to the direction in which the cork has blown out.
3. Cork blows out with greater velocity than the recoiling test tube.

Activity 1

• Make a pile of similar carom coins on a table, as shown in the figure.
• Attempt a sharp and strong horizontal hit at the bottom of the pile using another carom coin or striker.

Observations

• If the hit is strong enough the bottom coin moves out quickly.
• Once the lowermost coin is removed, the inertia of the other coins makes them fall vertically on the table.

Activity 2

• Set a five – rupee coin on a stiff card covering an empty glass tumbler standing on a table as shown in the figure.
• Give the card a sharp horizontal flick with a finger.

Observations

• If we do it fast, the card shoots away, allowing the coin to fall vertically into the glass tumbler due to its inertia.
• The inertia of the coin tries to maintain its state of rest even when the card is flown away.
• The force applied on the card due to flicking changes the inertia of the card but the coin resists this change and stays at rest, i.e, due to the inertia and gravity, the coin falls down into the tumbler.

Activity 3

• Request two children to stand on two separate carts as shown in the figure.
  
• Give them a bag full of sand or some other heavy object.
• Ask them to play a game of catch with the bag and observe.
• You can paint a white line on cartwheels to observe the motion of the two carts when the children throw the bag towards each other.

Observations

• In this case, each of them receives an instantaneous reaction as a result of throwing the sand bag.
• This activity explains Newton’s third law of motion, i.e., a force is exerted in forward direction in throwing the bag full of sand and the person who is throwing it, gets pushed backward.

Activity 4

• Take a big rubber balloon and inflate it fully.
• Tie its neck using a thread Also, using adhesive tape, fix a straw on the surface of this balloon.
• Pass a thread through the straw and hold one end of the thread in your hand or fix it on the wall.
• Ask your friend to hold the other end of the thread or fix it on a wall at some distance.
• The arrangement is shown in the figure.
• Now, remove the thread tied on the neck of the balloon and let the air escape from the mouth of the balloon.
• Observe the direction in which the straw moves.

Observations.

• When the air escapes out from the balloon, the straw moves in the direction opposite to the direction in which the air moved out of the balloon. This activity explains the law of conservation of momentum.
• This activity is also based on Newton’s third law of motion, i.e., for every action, there is always an equal and opposite reaction between two bodies.

Value Based Questions

Question 1.
Class IX students were playing cricket with cork ball in the school campus. Abhishek, senior student, told them about the accidents that can occur due to cork ball in the campus and also advised them to bring a soft cosco ball to play the game.
1. Why is it safe to play with a soft ball and not with a hard cork ball?
2. A player pulls his hands backwards while catching the ball shot at high speed Why?
3. What value of Abhishek is seen in this act?
Answer:

1. The soft ball has less inertia as compared to the heavy ball and it would not hurt the players.
2. By pulling the hand backwards, it reduces the force exerted by the ball
   on the hand.
3. Abhishek showed the value of being responsible and helpful by nature.

Question 2.
Rahul saw his karate expert friend breaking a brick. He tried to break the brick but his friend stopped him from doing so and told him that it would hurt him as one needs a lot of practice in doing so.
1. How can a karate expert break the brick without any injury to his hand?
2. What is Newton’s third law of motion?
3. What value of Rahul’s friend is seen in the above case?
Answer:

1. A karate expert applies the force with a large velocity in a very short interval of time on the brick, therefore, a large force is exerted on the brick and it breaks.
2. To every action there is an equal and opposite reaction, both act on different bodies.
3. Rahul’s friend showed the value of being responsible and caring friend.

JAC Class 9 Science Important Questions

JAC Board Class 9th Science Important Questions Chapter 8 Motion

Multiple Choice Questions

Question 1.
Which of the following is correct for a car which travels a distance of 100 km in 2 hours?
(a) Its average speed is 50 km/h
(b) The car did not travel at 50 km/h all the time
(c) The car travelled at 50 km/h all the time
(d) All of the above
Answer:
(a) Its average speed is 50 km/h

Question 2.
The distance-time graph of an object shown in the given figure represents that the object is
(a) at rest position
(b) moving with constant speed
(c) moving with constant velocity
(d) moving with constant acceleration
Answer:
(a) at rest position

Question 3.
The SI unit of velocity is
(a) ms^-1
(b) ms^-2
(c) ms^-3
(d) Nn^-1
Answer:
(a) ms^-1

Question 4.
Deceleration of a body is expressed in
(a) m
(b) ms^-1
(c) ms^-2
(d) ms^-3
Answer:
(c) ms^-2

Question 5.
The initial velocity of a body is ‘u’. It is under uniform acceleration ‘a’. Its velocity ‘v’ at any time ‘t’ is given by
(a) v = u + at²
(b) v = u + \(\frac{1}{2}\) at²
(c) v = u + at
(d) v = u
Answer:
(c) v = u + at

Question 6.
A wooden slab starting from rest, slides down a 10m long inclined plane with an acceleration of 5 ms^-2 What would be its speed at the bottom of the inclined plane?
(a) 10 ms^-1
(b) 12ms^-1
(c) 10 cm^-1
(d) 12 cm^-1
Answer:
(a) 10 ms^-1

Question 7.
The velocity of a particle increases from ‘u’ to ‘v’ in time’t’ during which it covers a distance ‘s’. If the particle has a uniform acceleration, which of the following equations does not apply to the motion?
(a) 2s = (v + u) t
(b) v² = u² – 2as
(c) a = v – u/t
(d) s = (u + \(\frac{1}{2}\) at) t
Answer:
(b) v² = u² – 2as

Question 8.
In 12 minutes, a car whose speed is 35 km/h travels a distance of
(a) 7 km
(b) 3.5 km
(c) 2.4 km
(d) none of these
Answer:
(a) 7 km

Question 9.
The area under the distance – time graph gives
(a) uniform speed
(b) non – uniform speed
(c) velocity and speed
(d) both (a) and (b)
Answer:
(d) both (a) and (b)

Question 10.
The odometer of a car measures
(a) speed
(b) velocity
(d) acceleration
(d) distance
Answer:
(d) distance

Question 11.
If a particle moves with a constant speed, the distance time graph is a
(a) straight line
(b) curved line
(c) straight line parallel to time axis
(d) straight line parallel to velocity axis
Answer:
(a) straight line

Question 12.
An object moving with uniform circular motion shows
(a) constant acceleration in speed
(b) constant velocity
(c) constant acceleration in direction
(d) constant change in type of motion
Answer:
(c) constant acceleration in direction

Question 13.
The slope of speed – time graph gives
(a) speed
(b) velocity
(c) acceleration
(d) momentum
Answer:
(c) acceleration

Question 14.
The distance travelled by a freely falling body is proportional to the
(a) mass of body
(b) square of the time of fall
(c) square of the acceleration due to
Answer:
(b) square of the time of fall

Question 15.
The figure shows the displacement time graph of a body moving in a straight line. The velocity of the body during the

(a) 2 ms^-2
(b) zero
(c) 3 ms^-1
(d) 2.5 ms^-1
Answer:
(b) zero

Analysing & Evaluating Questions

Question 16.
In which of the following cases of motion, the distance moved and the magnitude of displacement are equal?
(a) If the car is moving on a straight road
(b) If the car is moving in a circular path
(c) The pendulum is moving to and fro
(d) The earth is revolving around the Sun
Answer:
(a) If the car is moving on a straight road

Question 17.
Four cars A, B, C and D are moving on a levelled road. Their distance versus time graphs are shown here. Choose the correct statement.

(a) Car A is faster than car D
(b) Car B is the slowest
(c) Car D is faster than car C
(d) Car C is the slowest
Answer:
(b) Car B is the slowest

Question 18.
Suppose a boy is enjoying a ride on a merry – go – round which is moving with a constant speed of 10 ms^-1. It implies that the boy is
(a) at rest
(b) moving with no acceleration
(c) in accelerated motion
(d) moving with uniform velocity
Answer:
(c) in accelerated motion

Assertion Reason Questions

Directions: In the following questions, the Assertions and the Reasons have been put forward. Read the statements carefully and choose the correct alternative from the following:
(A) Both the assertion and the reason are correct and the reason is the correct explanation of the assertion.
(B) The assertion and the reason are correct but the reason is not the correct explanation of the assertion.
(C) The assertion is true but the reason is false.
(D) Both the statements are false.

1. Assertion: Velocity is a scalar quantity.
Reason: Velocity cannot be zero.
Answer:
(D) Both the statements are false.

2. Assertion: A body moving in a circular path is in non – uniform motion.
Reason: The direction of a body moving in a circular path changes at every point.
Answer:
(A) Both the assertion and the reason are correct and the reason is the correct explanation of the assertion.

3. Assertion: A stone thrown vertically upwards has negative acceleration.
Reason: The acceleration of the stone thrown upward is in the direction opposite to the direction of its motion.
Answer:
(A) Both the assertion and the reason are correct and the reason is the correct explanation of the assertion.

4. Assertion: A freely falling body is in uniform motion.
Reason: A freely falling body covers equal distances in equal intervals of time.
Answer:
(D) Both the statements are false.

5. Assertion: An object under acceleration can have a uniform speed.
Reason: Both speed and acceleration are scalar quantities.
Answer:
(C) The assertion is true but the reason is false.

Very Short Answer Type Questions

Question 1.
Define uniform motion.
Answer:
If an object covers equal distances in equal intervals of time, however small the time intervals may be, the motion of the object is said to be uniform motion.

Question 2.
Define non – uniform motion.
Answer:
If an object covers unequal distances in equal intervals of time, it is said to be in ‘non – uniform motion’.

Question 3.
Define speed.
Answer:
It is the distance travelled by a body per unit time.
Speed. = \(\vec{a}\)

Question 4.
Define average speed.
Answer:
The total distance travelled by an object divided by the total time taken is called its average speed.

Question 5.
Define acceleration.
Answer:
It is defined as the rate of change of velocity with time.
Acceleration = \(\frac{Change in velocity}{Time Taken}\)
or a = \(\frac{v-\mathbf{u}}{t}\)
The SI unit of acceleration is m/s².

Question 6.
Draw a distance – time graph that represents uniform speed.
Answer:

Question 7.
With the help of a distance – time graph show that the object is stationary.
Answer:

Question 8.
Define uniform circular motion.
Answer:
When a body moves in a circular path with uniform speed, its motion is called uniform circular motion.

Question 9.
Define scalar quantities. Give examples.
Answer:
The physical quantities which require only magnitude, and not the direction, for their complete description are called ‘scalars’ or ‘scalar quantities’. Distance, speed, time, area, etc., are all scalar quantities.

Question 10.
Define vector quantities. Give examples.
Answer:
The physical quantities which need both magnitude and direction for their complete description are called ‘vectors’ or vector quantities. Displacement, velocity, force, etc., are all vector quantities.

Question 11.
What is the SI unit of displacement?
Answer:
Metre (m).

Question 12.
A particle moves over three – quarters of a circle of radius. What is the magnitude of its displacement?
Answer:
When the particle covers three quarters of a circle, the magnitude of its displacement is

AB = \(\sqrt{\mathrm{OA}^{2}+\mathrm{OB}^{2}}\) = \(\sqrt{r^{2}+r^{2}}\)
= \(\sqrt{2 r}\) = √2 r

Question 13.
Can the average speed of a moving body ever be zero?
Answer:
Speed is a scalar quantity and is always positive. So the average speed of a moving body can never be zero.

Question 14.
What is the relationship between the distance travelled and the time elapsed for motion with uniform velocity?
Answer:
Distance is directly proportional to the time elapsed. In fact,
Distance travelled = Uniform velocity × Time elapsed

Question 15.
What is the SI unit of acceleration?
Answer:
The SI unit of acceleration is m/s².

Question 16.
What is acceleration of a body moving with uniform velocity?
Answer:
The acceleration of a body moving with uniform velocity is zero.

Question 17.
State a relationship connecting u, v, a and t for an accelerated motion. Give an example of motion in which acceleration is uniform.
Answer:
The relationship between u, v, a and t is v = u + at A body falling freely towards the earth has a uniform acceleration of 9.8 ms^-2.

Question 18.
Express the velocity of a body in uniform circular motion in terms of its time period T.
Answer:
Suppose a body of mass m rotates in a circle of radiusr with velocity v. It completes one revolution in time T. Then,
Velocity = \(\frac{Distance}{Time}\)[/latex] = \(\frac{Circumference}{Time period }\)[/latex]
or v = \(\frac{2 \pi \mathrm{r}}{\mathrm{T}}\)

Analysing & Evaluating Questions

Question 19.
The cars A, B, C and D are moving on a levelled road. Their distance versus time graphs are shown in the figure. Which car is the slowest?
Answer:
Speed = Slope of distance – time graph. The smaller the slope, the smaller is the speed

From the given figure, slope is minimum for car D. So, D is the slowest car.

Question 20.
A rubber ball dropped from a certain height bounce to certain height. This height keeps decreasing in subsequent bounces. What type of motion does the ball exhibit?
Answer:
The ball exhibits non – uniform motion.

Question 21.
A vehicle is moving at a constant velocity of 10 km/h in the north – south direction. It turns and starts moving towards east. What is the velocity of the vehicle towards east before taking the turn?
Answer:
Zero. The component of velocity at right angle to the original direction of motion is zero.

Short Answer Type Questions

Question 1.
Define the term rest and motion.
Answer:

• Rest: If a body does not change its position with respect to its surroundings, the body is said to be at rest, e.g., a table lying in a room is at rest with respect to the walls of the room.
• Motion: A body is said to be in motion if it changes its position with respect to its surroundings, e.g., a car running on the road is in motion with respect to the lamp posts, trees or bus stop on the roadside.

Question 2.
What is meant by a point object? Give some examples.
Answer:
Whenever the size of the object is much smaller than the distance it moves in a given time interval, the size of the object can be neglected. The object can be regarded as a point object in such cases.
Examples:

1. A car covering a distance of 10km can be treated as a point object.
2. Earth can be regarded as a point object for studying its motion around the sun.

Question 3.
Give some examples of straight line motion.
Answer:
Examples:

1. A bus moving on a straight road
2. A train moving on a straight track
3. A runner running along a straight track
4. A ball moving along a straight path
5. An object falling vertically downwards towards the surface of the earth.

Question 4.
How can we specify the position of an object?
Answer:
1. The position of an object can be specified by choosing:

• a fixed point called ‘origin’ or reference point, and
• a fixed line passing through the origin, called reference axis.

2. So the position of an object can be fully described by knowing:

• its distance from origin O and
• the angle 0 which the line joining the origin ‘O’ and the object makes with the reference axis.

In the figure shown below, the position of an object located at point P is 6 cm from the origin and 30° north of east. v (Scale: 1cm = 1m)

Question 5.
A body moves in a circle of radius ‘2R’. What is the distance covered and displacement of the body after 2 complete rounds?
Answer:
Distance covered after 2 complete rounds = 2 × circumference
= 2 × 2π (2R) = 8πR
Displacement = zero, because initial and final positions of the body are the same and displacement is the shortest distance between initial and final positions.

Question 6.
Define the term velocity. What is its SI unit? Is it a scalar or a vector quantity?
Answer:
Velocity is a physical quantity that gives both speed and direction of motion of the body.
Definition: Velocity of a body is defined as the displacement produced per unit time. Velocity is also defined as the speed of a body in a givendirection. If ‘s’ is the distance travelled by a body in a given direction and ‘t’ is the time taken to travel that distance, then the velocity V is given by,
Velocity = \(\frac{Displacement}{Time}\)[/latex]
SI unit of velocity is m/s. Velocity is a vector quantity because it requires both magnitude and direction of a body.

Question 7.
Give the difference between distance and displacement.

Distance	Displacement
(a) It is the total path covered by an object.	(a) It is the shortest path from initial position to the final position.
(b) It does not have any direction.	(b) It has direction.
(c) It cannot be zero.V	(c) It can be positive, negative or zero.

Question 8.
Differentiate between speed and velocity.
Answer:

Speed	Velocity
(a) It is the distance travelled by an object per unit of time.	(a) It is the displacement of the body per unit time.
(b) It is a scalar quantity, direction not required.	(b) It is a vector quantity, direction is required.
(c) It is always positive.	(c) It can be positive, negative or zero.

Question 9.
A cheetah is the fastest land animal and can achieve a peak velocity of 100 km/h upto distances less than 500 m. If a cheetah spots his prey at a distance of 100 m, what is the minimum time it will take to get its prey, if the average velocity attained by it is 90 km/h?
Answer:
Here v = 90Km/h = \(\frac{90 \times 1000 \mathrm{~m}}{3600 \mathrm{~s}}\)
= 25m/s
s = 100m
Minimum time, t = \(\frac{s}{v}\) = \(\frac{100}{25}\) = 4s

Question 10.
The Rajdhani Express travels a distance of 1384 km from Mumbai to Delhi. It starts from Mumbai at 4.00 p.m. and reaches Delhi at 9.00 a.m. the next day. What is its average speed?
Answer:
Total distance travelled = 1384 km
Total time taken = 17 hours
Average speed =\(\frac{Total distance travelled}{Total time taken}\)= \(\frac{1384}{17}\)
= 81.4 km/h

Question 11.
A body starts initially with a velocity ‘u’ and is accelerated at constant rate ‘a’. Find an expression for final velocity after time ‘t’
Answer:
First equation of motion : Let a body start with initial velocity ‘u’ and after time ‘t’, its velocity becomes ‘v’ due to uniform acceleration ‘a’. From the definition of acceleration,
Acceleration = \(\frac{Change in velocity }{Time taken}\)
= \(\frac{Final velocity – Initial velocity}{Time taken}\)
a = \(=\frac{\mathrm{V}-\mathrm{u}}{\mathrm{t}}\)
at = v – u
v = u + at.

Question 12.
Deduce the expression for the distance travelled by a body moving with uniform acceleration in a given time.
Answer:
Second equation of motion:
Suppose a body starts with initial velocity ‘u’ and due to uniform acceleration ‘a’ its final velocity becomes ‘v’ after time ‘t’ Then,
Average velocity = \(\frac{Initial velocity + Final velocity}{2}\)
=\(\frac{u+v}{2}\)
So, the distance covered by the body in time t is
s = Average velocity × Time =\(\frac{u+v}{2}\) × t = \(\frac{\mathrm{u}+(\mathrm{u}+\mathrm{at}) \times \mathrm{t}}{2}\)
= \(\frac{2 u t + a t^{2}}{2}\)
or s = ut + \(\frac{1}{2}\) at 2

Question 13.
Establish the relation v² – u² = 2as, where ‘u’ is the initial velocity, ‘v’ is the final velocity, ‘a’ is the uniform acceleration and ‘s’ is the distance covered by the body.
Answer:
Third equation of motion:
Let a body start with initial velocity ‘u’ and after covering distance ‘s’ under uniform acceleration ‘a’, its velocity becomes ‘v’ in ‘t’ seconds. Then
Average velocity = \(\frac{\mathrm{u}+\mathrm{v}}{2 \mathrm{}}\)
So the distance covered in time t is given by
s = Average velocity x Time
= \(\frac{u+v}{2}\) × t
or v + u = \(\frac{2 s}{t}\) …………..(1)

Using the first equation of motion: v = u + at
or v – u = at … (ii)

Multiplying equations (i) and (ii), we get
(v + u) (v – u) = \(2 \frac{s}{t}\) × at
or v² – u² = 2as

Question 14.
Show that the slope of distance – time graph gives velocity of the body.

Answer:
Given figure shows distance – time graph for a body moving with uniform velocity. Clearly, it covers distances s₁ and s₂ in times t₁ and t₂ respectively.
Slope of line AB = tan θ = \(\frac{\mathrm{BC}}{\mathrm{AC}}\)
= \(\frac{s_{2}-s_{1}}{t_{2}-t_{1}}\) = \(\frac{Displacement}{Time}\) = velocity
Hence, the slope of the distance-time graph gives velocity of the body.

Question 15.
Show that the slope of velocity time graph gives acceleration of the body.
Answer:
Given figure shows the velocity – time graph for a body in uniform acceleration. It is a straight line inclined to the time – axis. Body has velocities ‘u’ and ‘v’ at times ‘t₁’ and ‘t₂’ respectively
Img-1
Slope of line AE = tan θ = \( \frac{E D}{A D}\)
\(\frac{v-u}{t_{2}-t_{1}}\) = \( \frac{Change in velocity}{Time taken}\)
= Acceleration of the body
Hence, the slope of the velocity – time graph gives the acceleration of the body.

Question 16.
Draw velocity – time graph for a body moving with uniform velocity. Hence show that the area under the velocity time graph gives the distance travelled by the body in a given time interval.
Answer:
In the given figure, line PQ is the velocity – time graph of a body moving with a uniform velocity such that OP = v Area of rectangle ABCD = AD × AB
= OP × AB
= v × (t₂ – t₁)
= Velocity × Time
= Distance travelled in time interval (t₂ – t₁)

Hence, the area under the velocity – time graph gives the distance travelled by the body in the given time interval.

Question 17.
The odometer of a car reads 1800 km at the start of a trip and 2400 km at the end of the trip. If the trip took 10h, calculate the average speed of the car in km/h and m/s.
Answer:
Distance covered by the car (s)
= 2400 – 1800 = 600 km.
Trip time = 10h
Average speed = ?

(i) V_av = \(\frac{s}{t}\) = \(\frac{600}{10}\) km = 60 km/h

(ii) In m/s : (60 km/h) = \(\frac{60 \times 1000}{60 \times 60}\)
= 16.7 m/s
The average speed of the car in km/h is 60 km/h and in m/s is 16.7 m/s.

Question 18.
Draw velocity – time graphs to show the following:
(a) Uniform velocity
(b) Uniform acceleration
(c) Non – uniform acceleration
Answer:

Analysing & Evaluating Questions

Question 19.
The table given below shows distance (in km) travelled by bodies A, B and C. Read the data carefully and answer the following questions.

	Distance (in km) covered by different bodies
Time (s)	Body(A)	Body (B)	Body (C)
1st second	20	20	20
2nd second	20	36	60
3rd second	20	24	100
4th second	20	30	140
5 th second	20	48	180

(a) Which of the bodies is moving with
(i) constant speed?
(ii) constant acceleration?
(b) Which of the bodies covers
(i) maximum distance in 3rd second?
(ii) minimum distance in 3rd second?
Answer:
(a) (i) Body A
(ii) Body C

(b) (i) Body C
(ii) Body A

Question 20.
An electron moving with a velocity of 5 × 10⁴ms^-1 enters a uniform electric field and acquires a uniform acceleration of 10⁴ms^-2 in the direction of its initial motion.
(a) Calculate the time in which the electron would acquire a velocity double of its initial velocity.
(b) How much distance the electron would cover in this time?
Answer:
(i) Acceleration = \(\frac{ Change in velocity}{Time taken}\)
or Time taken, t = \(\frac{5 \times 10^{4} \mathrm{~m} \mathrm{~s}^{-1}}{10^{4} \mathrm{~m} \mathrm{~s}^{-2}}\)
= 5 s

(ii) s = ut + \(\frac{1}{2}\) at²
= (5 × 10⁴ m s^-1 × 5 s) + \(\frac{1}{2}\) × 10⁴m s^-2 × (5s)²
= 37.5 × 104 m

Long Answer Type Questions

Question 1.
Describe the various types of motion observed in bodies.
Answer:
Various types of motion as observed in bodies are:

1. Translatory motion: When a body moves, as a whole, along a straight or curved path, it is said to be in translatory motion. Translatory motion is again of two types:
   • Rectilinear motion : Here a body moves as a whole along a straight path. For example, a train moving on a straight track has translatory rectilinear motion.
   • Curvilinear motion : In this case a body moves as a whole along a curved path. For example, motion of a bicycle taking a turn along a curved path.
2. Rotatory motion: When a body rotates about a fixed point or axis. it exhibits a rotatory motion. For example, motion of a flywheel about a shaft
3. Vibratory or oscillatory motion: When a body moves to and fro about a mean position, the motion is said to be vibratory or oscillatory motion. For example, the motion of the pendulum of a wall – clock.
4. Complex motion: When the motion of a body may be a combination of more than one type of motion, it is said to be a complex motion. For example, a ball rolling down an inclined plane has both translatory and rotatory motions.

Question 2.
Define average velocity when the velocity of a body changes at a non – uniform rate and a uniform rate.
Answer:
Average velocity : When the velocity of a body changes at a non – uniform rate, its average velocity is defined as the net displacement covered divided by the total time taken.
Average velocity = \(\frac{Net displacement}{Total time taken}\)
When the velocity of a body changes at a uniform rate, the average velocity is given by the arithmetic mean of initial velocity and final velocity for a given period of time.
Average velocity = \(\frac{Initial velocity + Final velocity}{2}\) :
If u is the initial velocity and v is the final velocity, the average velocity v_ay is given by,
v_ay = \(=\frac{u+v}{2}\)

Question 3.
Explain the difference regarding the nature of acceleration of the three moving bodies as expressed by the following velocity – time graphs:

(a) Uniform acceleration: A body increases velocity by equal amounts in equal intervals of time.
(b) Non – uniform acceleration: A body travels unequal distances in equal intervals of time.
(c) Uniform motion or zero acceleration: A body moves with constant

Analysing & Evaluating Questions

Question 4.
Two stones are thrown vertically upwards simultaneously with their initial velocities u₁ and u₂ respectively. Prove that the heights reached by them would be in the ratio of \(\mathbf{u}_{1}^{2}\) : \(\mathbf{u}_{2}^{2}\) (Assume upward acceleration is – g and downward acceleration to be + g).
Answer:
Stone 1
Initial velocity = u₁
Acceleration = – g
Height = h,
Final velocity, v = 0
Using the equation, v² – u² =2as
0 – \(\vec{a}\) = 2 (- g) × h₁
h₁ = \(\frac{-u_{1}^{2}}{-2 g}\) = \(\frac{u_{1}^{2}}{2 g}\) ……..(1)

Stone 2
Initial velocity = u₁
Acceleration = – g
Height = h2
Final velocity, v = 0
0² \(-u_{2}^{2}\) = 2 (- g) × h₂
h₂ = \(\frac{-u_{2}^{2}}{-2 g}\) =\(\frac{u_{2}^{2}}{2 g}\) ……..(ii)
This gives; h₁ : h₂ = \(\vec{a}\) : \(\vec{a}\)
= \(=\frac{u_{1}^{2}}{2 g}\) : \(\frac{\mathrm{u}_{2}^{2}}{2 \mathrm{~g}}\)
=\(u_{1}^{2}\) : \(\vec{a}\)

Activity 1

1. In your everyday life, you come across a range of motions in which:
   • acceleration is in the direction of motion
   • acceleration is against the direction of motion
   • acceleration is uniform
   • acceleration is non – uniform
2. Observe these motions carefully and identify one example each of the above type of motions.

Observations

• A car moving on a road.
• A ball thrown up.
• Fan blades rotating
• Windmill at time moves fast when the wind speed is more and becomes slow when the wind speed decreases.

Activity 2

• Take piece of thread and tie a small piece of stone at one of its end. Move the stone to describe a circular path with constant speed by holding the thread at the other end.
  
• Now, let the stone go by releasing the thread.
• Observe the direction in which the stone moves after it is released.
• By repeating the activity for a few more times and releasing the stone at different positions of the circular path, carefully observe whether the direction in which the stone moves remains the same or not.

Observations

• When the stone is released, it moves along a straight line, tangential to the circular path.
• By repeating the activity and releasing the stone at different positions of the circular path, the direction in which the stone moves does not remain the same. It changes every time.

Value Based Questions

Question 1.
The speed limits are prescribed for vehicles running on highways. Why is it essential to follow the speed limit rules?
Answer:
Speed limits are prescribed on the highways/expressways because:
1. When a vehicle is made to run at height speeds, its tyres get hot (due to friction). This makes them softer and the air inside the tubes hotter Both. these factors may lead to bursting of tyre/tube.

2. Reflex time varies from person to person. Any running vehicle needs a certain time period to stop after the brakes are applied. At higher speeds, this time period becomes shorter.

3. As a result, the chances of collision increase. Because of these reasons, one should strictly follow the speed limit regulations. Also, it is safer to drive in your lane depending upon the speed of your vehicle.

4. Moral: Drive below the speed limit and in your lane.

Question 2.
Most drivers involved in road accidents are found to be drunk. Give reason.
Answer:
The reflex time of a person increases when drunk, (average reflex time of a normal person is about 1/15s). Therefore, such a driver of vehicle will take more time in applying brakes. As a result, the vehicle may not stop well in time and cause an accident.

JAC Class 9 Science Important Questions

Jharkhand Board JAC Class 9 Maths Solutions Chapter 10 Circles Ex 10.1 Textbook Exercise Questions and Answers.

JAC Board Class 9th Maths Solutions Chapter 10 Circles Ex 10.1

Page-171

Question 1.
Fill in the blanks:
(i) The centre of a circle lies in …………….. of the circle. (exterior/ interior)
(ii) A point, whose distance from the centre of a circle is greater than its radius lies in …………. of the circle, (exterior/ interior)
(iii) The longest chord of a circle is a …………. of the circle.
(iv) An arc is a ………… when its ends are the ends of a diameter.
(v) Segment of a circle is the region between an arc and …………… of the circle.
(vi) A circle divides the plane, on which it lies, in …………….. parts.
Answer:
(i) The centre of a circle lies in interior of the circle.
(ii) A point, whose distance from the centre of a circle is greater than its radius lies in exterior of the circle.
(iii) The longest chord of a circle is a diameter of the circle.
(iv) An arc is a semicircle when its ends are the ends of a diameter.
(v) Segment of a circle is the region between an arc and chord of the circle.
(vi) A circle divides the plane, on which it lies, in three parts.

Question 2.
Write True or False: Give reasons for your answers.
(i) Line segment joining the centre to any point on the circle is a radius of the circle.
(ii) A circle has only finite number of equal chords.
(iii) If a circle is divided into three equal arcs, each is a major arc.
(iv) A chord of a circle, which is twice as long as its radius, is a diameter of the circle.
(v) Sector is the region between the chord and its corresponding arc.
(vi) A circle is a plane figure.
Answer:
(i) True.
All the line segments from the centre to the circle are of equal length.

(ii) False.
We can draw infinite number of equal chords.

(iii) False.
We get major and minor arcs for unequal arcs. So, for equal arcs on circle we can’t say it is major arc or minor arc.

(iv) True.
A chord which is twice as long as radius must pass through the centre of the circle and is diameter of the circle.

(v) False.
Sector is the region between the arc and the two radii of the circle.

(vi) True.
A circle can be drawn on the plane.

JAC Board Class 9th Social Science Solutions Economics Chapter 2 People as Resource

JAC Class 9th Economics People as Resource InText Questions and Answers 

Page No. 17

Question 1.
Looking at the photograph can you explain how a doctor, teacher, engineer and a tailor are an asset to the economy?
Answer:
In the given picture a doctor is providing healthcare service to a woman. These types of serivces are essential for a healthier population. The teachers provide essential services in the field of education. They make people literate, imparting them good education and increasing their mental skill. A healthy and literate person helps in the growth of GDP of the nation.

An engineer provides his services in the field of infrastructure. He manufactures various types of machines, engines, builds roads and bridges which contribute in the development of the economy. The tailor stitches clothes and mends it. From the above, we can see that doctor, engineer, teacher and tailor are an asset to the economy because all are helpful in strengthening the nation.

Page No. 18

Question 1.
Do you notice any difference between the two friends ? What are those?
Answer:
Yes, we noticed some differences between the two friends. These differences are as follows:

1. Sakai’s parents gave him higher education while Vilas was unable to get education as he lost his father at the age of 2 years.
2. Sakai was an active boy while Vilas lost his health due to his inactive approach.
3. Sakai provided his services for a computer firm while Vilas sold fish like his mother to earn his livelihood.

Page No. 21

Study the graph and answer the following questions:

[Source : Economic survey, 2017-18.]

Question 1.
Have the literacy rates of the population increased since 1951?
Answer:
Yes, the literacy rates of the population have increased since 1951.

Question 2.
In which year India has the highest literacy rates?
Answer:
In the year 2011, India has the hightest literacy rates.

Question 3.
Why literacy rate is high among the males of India?
Answer:
Literacy rate is high among the males of India because the opportunity to get education is more for males as compared to females due to male-dominated society in India.

Question 4.
Why are women less educated than men?
Answer:
Women are less educated then man due to gender inequality and some social boundations in India. Parents do not send their girls to schools because they feel that they should look after the domestic work while boys should go to school.

Question 5.
How would you calculate literacy rate in India?
Answer:
The literacy rate can be calculated on the basis of the number of literate people divided by the population multiplied by 100.
Literacy Rate = \(\frac { Number of literate people in India }{ Population of India }\) = 100

Question 6.
What is your projection about India’s literacy rate in 2020?
Answer:
My projection about India’s literacy rate in 2020 is approximately 90 per cent.

Page No. 23

Discuss this table in the classroom and answer the following questions:
Table Number of Institutions of higher Education, Enrolment and faculty

Year	Number of colleges	Number of Universities	Students & Colleges	Teachers in University
1950-51	750	30	2,63,000	24,000
1990-91	7,346	177	49,25,000	2,72,000
1998-99	11,089	238	74,17,000	3,42,000
2010-11	33,023	523	186,70,050	8,16,966
2012-13	37,204	628	228,02,938	9,25,396
201-15	40,760	711	265,85,437	12,61,350
2015-16	41,435	753	284,84,741	14,38,000
2016-17	42,338	795	294,27,158	14,70,190

Source: UGC Annual Report 2010-11, 2012-13, 2013-14, 2015-16 and selected educational statistics, Ministry of HRD, www.ugc. ac. in (Anual Report. 2016-17 pdf).

Question 1.
Is the increase in the number of colleges adequate to admit the increasing number of students?
Answer:
No, the increasing number of colleges is not adquate to admit the increasing number of students.

Question 2.
Do you think we should have more number of Universities?
Answer:
Yes, I think, we should have more number of Universities to encourage higher education in India.

Question 3.
What is the increase noticed among the teachers in the year 1998-99?
Answer:
In the year 1998-99, the increase noticed is 70,000 teachers since 1990-91.

Question 4.
What is your idea about future colleges and universities?
Answer:
My idea about future colleges and universities is that there should some more professional colleges and Universities with more job-oriented and vocational courses offered to the students.

JAC Class 9th Economics People as Resource Textbook Questions and Answers 

Question 1.
What do you understand by ‘people as a resource’?
Answer:
‘People as a resource’ is a way of referring to a country’s working people in terms of their existing productive skills and abilities. It emphasises the ability of people to contribute to the creation of the Gross National Product. Population is a resource which also called Human Resource.

Question 2.
How is human resource different from other resources like land and physical capital?
Answer:
Human resource is different from other resources like land and physical capital in the following ways :

1. Human resource is an active and living resource.
2. Human resource is an indispensable resource.
3. Human resource makes use of other resources like land, physical capital, etc.
4. There are many forms of human resource as they work as manager, entrepreneur, worker, etc.

Question 3.
What is the role of education in human capital formation?
Answer:
Education plays an important role in human capital formation. It helps the people to make better use of economic opportunities available before them. It increases the quality of labour and helps to enhance the total productivity. This results in the growth of the economy.

Question 4.
What is the role of health in human capital formation?
Answer:
Health has a vital role in human capital formation. The health of a person helps him to realise his full potential. Health is an indispensable basis for realising one’s well being. Economic growth can be achieved at a faster rate, if population of the country is healthy and competent. Only a healthy person can do full justice to his job. So, we can say that health has an important role in human capital formation.

Question 5.
What part does health play in the individual’s working life?
Answer:
Health is a very important aspect of life. It includes the physical, mental, social, emotional and economic well-being of an individual. It is rightly said that ‘a sound mind lives in a sound body’. An unhealthy person is a liability for his family, organisation and country. No organisation will employ such a person who cannot work efficiently due to ill-health.

Question 6.
What are the various activities undertaken in the primary sector, secondary sector and tertiary sector?
Answer:
Following activities are undertaken in the primary sector, secondary sector and tertiary sector :

1. Primary Sector: Farming, forestery, animal husbandry, fishing, poultry farming, mining, quarrying, etc.
2. Secondary Sector: Manufacturing, processing, etc.
3. Tertiary Sector: Trade, Transport, Communication, Banking, Education, Health, Tourism, Services, Insurance, etc.

Question 7.
What is the difference between economic activities and non-economic activities?
Answer:
Activities which are performed to earn money are called economic activities. These activities add value to the National Income.
On the other hand, activities which are not performed to earn money are called non-economic activities. These activities are performed only for self-consumption. Example: If a father is a teacher in a school he gets salary for it. This is an economic activity. But when he teaches his own children, he is performing a non-economic activity.

Question 8.
Why are women employed in low-paid work?
Answer: Women are employed in low paid work due to the following reasons :

1. A majority of women have lesser education and low skill sets.
2. Generally, women are considered physically and emotionally weak.
3. Most women work in the unorganised sector.
4. Women are generally unaware of their legal right.

Question 9.
How will you explain the term unemployment?
Answer:
Unemployment is a term referring to individuals who are employable and seeking a job but are unable to find a job. Furthermore, it is those people in the workforce or pool of people who are available for work who do not have an appropriate job. Usually measured by the unemployment rate, which is dividing the number of unemployed people by the total number of people in the workforce, unemployment serves as one of the in¬dicators of an economy’s status.

Question 10.
What is the difference between disguised unemployment and seasonal unemployment?
Answer:
Disguised Unemployment: In disguised unemployment people appear to be employed but their productivity is zero. Such kind of unemployment is mostly found in agriculture. Seasonal Unemployment : In seasonal unemployment people get jobs during some months of the year. For the rest months of the year they do not get jobs and sit idle. People dependent upon agriculture usually face this kind of unemployment.

Question 11.
Why is educated unemployed, a peculiar problem of India?
Answer:
Educated unemployment is posing a great threat to our country. It has become a common phenomenon in India. Lakhs of educated persons are unemployed. This is a peculiar problem of India in the following ways:

1. It leads to wastage of educated manpower resource.
2. The youth feel hopeless and dejected.
3. It tends to increase economic overload.
4. The quality of life of an individual as well as of society is adversely affected.
5. Unemployment has a detrimental impact on the overall growth of an economy.

Question 12.
In which field do you think India can build the maximum employment opportunity?
Answer:
I think, India can build the maximum employment opportunity in the service
sector or say tertiary sector. In this sector, various new services are now emerging like biotechnology, IT (information technology) and so on.

Question 13.
Can you suggest some measures in the education system to mitigate the problem of the educated unemployed?
Answer:
The following measures can help to mitigate the problem of the educated unemployed :

1. The education system should be job-oriented.
2. Education should be planned and executed properly.
3. Higher education should be restricted to meritorious students only.
4. Education should encourage people to become self-reliant.

Question 14.
Can you imagine some village which initially had no job opportunities but later came up with many?
Answer:
Yes, we can imagine not only one but many villages which initially had no job opportunities but later came up with many. The villagers started to provide education to their children. These educated youngsters got absorbed in many professions in the villages like teaching, agro-engineering, etc. They created various types of employment in their villages. As a result, villages developed soon. Now these villages have better education facilities, health facilities, proper supply of water and electricity.

Question 15.
Which capital would you consider the best-land, labour, physical capital and human capital? Why?
Answer:
We shall consider human capital the best capital because:

1. Human capital makes efficient use of other resources land, labour and physical capital. These resources cannot be useful on their own.
2. Human capital can be developed to any extent.
3. Human capital activates other resources.

JAC Class 9 Social Science Solutions

JAC Board Class 9th Social Science Notes Civics Chapter 1 What is Democracy? Why Democracy?

→ Introduction

• Democracy is the most prevalent form of government in the world today and it is expanding in even more countries.
• The aim here to understand clearly the bare minimum features of a democratic form of government.
• In this chapter, we will discuss about the meaning, definition and features of democracy.
• After going through this chapter, we would be able to distinguish a democratic form of government from a non-democratic government.

→ What is Democracy?

• The word democracy comes from the Greek word ‘Demokratia’, in which ‘demos’ means people and ‘kratia’ means rule, i.e., rule by the people.
• ‘Democracy is government of the people, by the people and for the people’ Abraham Lincoln.
• Definition of Democracy-Democracy is a form of government in which the rulers are elected by the people.
• From this definition, we can clearly separate non-democratic governments from democratic ones.
• For instance, army rulers of Myanmar are not elected by the people, and are, thus, non-democratic.
• Dictators like Pinochet of Chile are not elected by the people. This is also applicable to the kings of Saudi Arabia and Nepal.
• If we accept this definition, it implies that almost every government is a democracy that holds an election. But it would be very misleading.
• We need to carefully distinguish between a government that is a democracy and one that pretends to be a democracy.

→ Features of Democracy

• Rulers elected by the people take all the major decisions.
• Elections offer a choice and fair opportunity to the people to change the current rulers.
• This choice and opportunity is available to all the people on an equal basis.
• The exercise of this choice leads to government limited by basic rulers of the constitution and citizens rights.

→ Mbgor Decisions by Elected Leaders

• In Pakistan, General Pervez Musharraf led a military coup in October 1999.
• He overthrew a democratically-elected government and declared himself the chief executive of the country. Later, he changed his designation to President.
• In Pakistan, elected representatives had some power but the final power was in the hands of military officers and General Musharraf himself.
• This condition cannot be termed as people’s rule.
• Thus, first feature of democracy is that, in a democracy, the final decision-making power must rest with those elected by the people’.

→ Free and Fair Electoral Composition

• The example of China and Mexico will give us an understanding of this feature of democracy.
• In China, elections are regularly held after every five years for electing the country’s parliament called Quanguo Renmin
• Daibiao Dahui (National People’s Congress).
• The National People’s Congress has the power to appoint the President of the country.
• Before contesting elections, each candidate needs the approval of the Chinese Communist Party.
• The government is always formed by the Communist Party.
• In Mexico, elections are held after every six years to elect its President.
• Mexico has never been under military or dictator’s rule.
• Till 2000, all the Presidential elections were won by the PRI (Institutional Revolutionary Party).
• Opposition parties did contest elections, but never managed to win.
• The PRI was known to use many dirty tricks to win elections.
• Conclusion of both the cases. In China, the elections do not offer the people any serious choice. They take to choose the ruling party and the candidates approved by it.
• In the Mexican example, people seemed to really have a choice, but in practice, they
  had no choice.
• There was no way the rulling party could be defeated, even if people were against it. These are not fair elections.
• Thus, another feature of democracy is that, “democracy must be based on a free and fair election where those currently in power have a fair chance of losing.”

→ One Person, One Vote, One Value

• The principle of universal adult franchise is accepted by almost all over the world. But there are many instances of denial of this equal right to vote.
• In Saudi Arabia, women do not have the right to vote.
• Estonia has made its citizenship rules in such a way that people belonging to Russian minority find it difficult to get the right to vote.
• In Fiji, the electoral system is such that the vote of an indigenous Fijian has more value than that of an Indian Fijian.
• Democracy is based on a fundamental principle of political equality.
• This gives us the third feature of democracy; ‘In a democracy, each adult citizen must have one vote and each vote must have one value.’

→ Rule of Law and Respect for Rights

• From the case of Zimbabwe, this feature of democracy can be understood in a better way.
• ZANU-PF party leader, Robert Mugabe, has been the president of the country since independence.
• Election are always won by this party through violence and harassment of the opposition politicians and journalists.
• Thus, there is no rule of law and citizen’s rights are not respected.
• Robert Mugabe was forced out of office in 2017.
• The example of Zimbabwe shows that popular approval of the rulers is necessary in a democracy, but it is not sufficient. Popular governments can be undemocratic.
• The state should respect the basic rights of citizens, like-freedom of expression, freedom to protest and freedom to take political action. Further, these rights should be protected by the judiciary whose orders should be obeyed by all.
• Thus another feature of democracy is that ‘a democratic government rules within i nmt s set by constitutional law and citizens’ rights.’

→ Summary Definition

• Through a series of examples, we marked out various features of democracy as a form of government.
• Accordingly, democracy is a form of government in which: (i) Rulers elected by the people take all the major decisions; (ii) Elections offer a choice and fair opportunity to the people to change the current rulers; (iii) This choice and opportunity is available to all the people on an equal basis; and (iv) The exercise of this choice leads to a government limited by basic rules of the constitution and citizens’ rights.

→ Arguments against Democracy

• Leaders keep changing in a democracy. This leads to instability.
• Democracy is all about political competition and power-play. There is no scope for morality.
• Democracy leads to delays.
• Elected leaders do not know the best interest of the people. It leads to bad decisions.
• Democracy leads to corruption, for it is based on electoral competition.
• Ordinary people don’t know what is good for them; they should not decide anything.

→ Arguments for Democracy

• A democratic government is a better government because it is a more accountable form of government.
• Democracy improves the quality of decision-making.
• Democracy provides a method to deal with various differences and conflicts.
• Democracy enhances the dignity of citizens.
• Democracy is better than other forms of government because it allows us to correct its own mistakes.
• The most common form that democracy taken in our times is that of a representative democracy. A majority is allowed to take decisions on behalf of all the people.

→ Broader Meanings of Democracy

• A democratic decision involves consultation with and consent of all those who are affected by that decision.
• Those who are not powerful have the same way in taking the decision as those who are powerful. This can apply to a government or a family or any other organization.
• Thus, democracy is also a principle that can be applied to any sphere of life.
• In a democracy, every citizen must be able to play an equal role in decision making.

→ Democracy: Democracy is a form of government in which the rulers are elected by the people.

→ Democratic government: A democratic government is a government in which citizens have
right to practice their fundamental rights and also their is full transparency between citizens of the country and the ruling government. Some examples of democratic government are India, USA, UK, France, etc.

→ Non-democratic government: A non-democratic government is a government run by officials who are not elected by citizens and are not accountable to citizens.

→ Monarchy: (i) The system of government or rule by a king or queen, (ii) a country that is governed by a king or queen.

→ Referendum: A public opinion poll seeking public aproval/disapproval to a specific proposal.

→ Dictatorship: A system of government in which all powers vest with one person.

→ Universal adult franchise: Universal adult franchise means all citizens who are 18 years and above have the right to vote, irrespective of their caste or education, religion, colour, race and economic conditions.

→ Autocratic: Autocratic describes a way of rulling, but not in a nice way. An autocratic leader is one who rules with an iron fist. In other words, someone who behaves like a dictator.

→ Corruption: Corruption is a form of dishonesty or criminal offence undertaken by a person or orgarlization entrusted with a position of authority, to acquire illicit benefit or abuse power for one’s own gain.

→ Decision-making: Decision-making is the process of making choices by identifying, a decision, gathering information and assessing alternative resolutions.

JAC Class 9 Social Science Notes