Bhagya

Students should go through these JAC Class 9 Maths Notes Chapter 10 वृत्त will seemingly help to get a clear insight into all the important concepts.

JAC Board Class 9 Maths Notes Chapter 10 वृत्त

→ वृत्त (Circle) : वृत्त एक समतल में स्थित उन बिन्दुओं का समुच्चय (Set) होता है, जो समतल में दिए गए एक स्थिर बिन्दु से दी हुई नियत दूरी पर होते हैं।
स्थिर बिन्दु को वृत्त का केन्द्र (Centre) और उस केन्द्र से वृत्त के प्रत्येक बिन्दु की नियत दूरी को वृत्त की त्रिज्या (Radius) कहते हैं।
(i) वृत्त की परिभाषा एक बिन्दुपध के रूप में भी दी जा सकती है।
परिभाषा : यदि एक समतल में कोई बिन्दु इस तरह गतिमान होता है कि समतल में दिए गए एक स्थिर बिन्दु से उसकी दूरी सदा ही नियत रही है, तो उस बिन्दु के पथ को वृत्त कहते हैं।
(ii) समुच्चय संकेतन में वृत्त को इस प्रकार लिखा जाता है:
C(O, r) = {X : OX = r}
(iii) एक वृत्त की सभी त्रिज्याएँ समान होती हैं। आकृति में,
OX = OY = OZ = r

→ वृत्त का अन्तः और बाह्य भाग (Interior and Exterior of a Circle) :
बिन्दु को, जहाँ OP <r, वृत्त का अन्तः बिन्दु कहते हैं। वृत्त के अन्तःबिन्दु को I₁ से प्रदर्शित करते हैं। बिन्दु Q को, जहाँ OQ > r वृत्त का बाह्य बिन्दु कहते हैं। वृत्त के बाह्य भाग को I₂ से दशति हैं।
सांकेतिक रूप में,
I₁ = {P: OP < r } तथा
I₂ = {P: OP > r}

→ गोल चक्रिका (Circular Disc) वृत्त C (O, r) के अन्तःभाग और वत्त पर स्थित बिन्दुओं के समुच्चय को केन्द्र O तथा त्रिज्या r वाली एक गोल चक्रिका कहते हैं।

→ संकेन्द्रीय वृत्त (Concentric Circles): एक ही केन्द्र वाले दो या दो से अधिक वृत्तों को संकेन्द्रीय वृत्त कहते हैं।

→ वृत्त का चाप (Arc of Circle) : यदि PQ वृत्त C (O, r) पर कोई दो बिन्दु हों तो वृत्त दो भागों में बँट जाता है, जिनमें से प्रत्येक भाग को वृत्त का चाप कहते हैं। छोटे भाग को लघु चाप (Minor arc) तथा बड़े भाग को दीर्घ चाप (Major arc) कहते हैं। चाप को प्रायः से प्रदर्शित करते हैं। आकृति में \(\overparen{P X Q}\) लघु चाप तथा \(\overparen{P Y Q}\) दीर्घ चाप है।

→ दक्षिणावर्त दिशा और वामावर्त दिशा (Clockwise Direction and Counter Clockwise or Anticlockwise Direction) : जिस दिशा में घड़ी की मिनट वाली सुई घूमती है, उसे दक्षिणावर्त दिशा तथा उसकी उलटी दिशा को वामावर्त दिशा कहते हैं। आकृति में P से Q की ओर की दिशा वामावर्त दिशा तथा Q से P की ओर की दिशा दक्षिणावर्त दिशा है।

→ वृत्त की जीवा (Chord of a Circle): वृत्त के दो बिन्दुओं को मिलाने वाले रेखाखण्ड को वृत्त की जीवा कहते हैं। आकृति में वृत्त पर स्थित प्रदत्त दो बिन्दुओं P तथा Q से खींची गयी रेखा जीवा PQ है।

→ वृत्त का व्यास (Diameter of a Circle) : वृत्त के केन्द्र से होकर जाने वाली जीवा को वृत्त का व्यास कहते हैं। आकृति में XY वृत्त का व्यास है। यदि वृत्त C (O, r) का व्यास हो, तो
d = 2r.
नोट:

• एक वृत्त के अनेक व्यास होते हैं।
• वृत्त के समस्त व्यास लम्बाई में समान होते हैं।
• वृत्त का व्यास उस वृत्त की सबसे बड़ी जीवा होती है।
• वृत्त का व्यास वृत्त की त्रिज्या का दोगुना होता है।

→ अर्द्धवृत्त (Semicircle) : व्यास वृत्त को दो बराबर चापों में विभाजित करता है। प्रत्येक चाप अर्द्धवृत्त कहलाता है।

आकृति में \(\overparen{P Q}\) तथा \(\overparen{Q P}\) अर्द्धवृत्त हैं।

→ वृत्तखण्ड (Segment) वृत्त की जीवा वृत्ताकार चक्रिका को दो भागों में विभक्त करती है उन दो भागों में से प्रत्येक भाग को वृत्तखण्ड कहते हैं। छोटे भाग को लघु वृत्तखण्ड (Minor segment) और बड़े भाग को दीर्घ वृत्तखण्ड (Major segment) कहते हैं।

इन खण्डों में से प्रत्येक खण्ड को दूसरे खण्ड का एकान्तर वृत्तखण्ड (Alternate segment) कहते हैं।

→ त्रिज्यखण्ड (Sector) किसी वृत्त के चाप तथा उसके अन्त्यबिन्दु से जाने वाली त्रिज्याओं से बनी आकृति त्रिज्यखण्ड कहलाती है। आकृति में OAQB लघु त्रिज्यखण्ड (Minor Sector) तथा OAPB दीर्घ त्रिज्यखण्ड (Major Sector) है।

→ चाप का अंशमाप (Degree Measure of an Arc) मान लीजिए C(O, r) एक वृत्त है, तो उस कोण को जिसका शीर्ष O है, वृत्त का केन्द्रीय कोण (Central angle) कहा जाता है। वृत्त की अंश माप 365° तथा अर्द्धवृत्त की अंशमाप 180° होती है चाप की अंश माप को M \(\overparen{A B}\) से प्रकट करते हैं। यदि चाप द्वारा केन्द्र पर अन्तरित कोण अंशों में दिया हों अथवा अंशों में ज्ञात किया जाए तो वह कोण चाप का अंशमाप कहलाता है।
अतः m\(\overparen{A B}\) = ∠AOB का मान अंशों में।

→ सर्वांगसम वृत्त (Congruent Circles) ऐसे दो या दो से अधिक वृत्त सर्वांगसम वृत्त कहलाते हैं, जिनकी त्रिज्याओं की माप समान हों। आकृति में, OA = O’C।

→ सर्वांगसम चाप (Congruent Arcs) : दो सर्वांगसम वृत्तों के ऐसे चाप जिनके अंशमाप समान हों, सर्वागसम चाप कहलाते हैं। आकृति में चाप AB, चाप CD के सर्वागसम है इसे “\(\overparen{A B}\) ≡ \(\overparen{C D}\)” भी लिखा जा सकता है।

→ वृत्त की परिधि (Circumference of a Circle) : वृत्त के परिमाप को परिधि कहते हैं।
यदि त्रिज्या r होता
परिधि = 2πr ⇒ πd
यहाँ π = \(\frac{22}{7}\) या 3.1416.

→ चक्रीय चतुर्भुज (Cyclic Quadrilateral) : किसी चतुर्भज को चक्रीय चतुर्भुज कहते हैं, यदि उसके चारों शीर्ष बिन्दु वृत्त पर स्थित हों। आकृति में ABCD चक्रीय चतुर्भुज है।

Students should go through these JAC Class 9 Maths Notes Chapter 2 बहुपद will seemingly help to get a clear insight into all the important concepts.

JAC Board Class 9 Maths Notes Chapter 2 बहुपद

चर / अचर : चर का मान स्थिर नहीं होता है। इसे हम स्वेच्छा से बदल सकते हैं जबकि अचर का मान स्थिर रहता है। हम चरों को अक्षरों x, y, z आदि से व्यक्त करते हैं तथा अचरों को वास्तविक संख्याओं या अक्षरों a, b, c द्वारा व्यक्त करते हैं।
बीजीय व्यंजक = [(एक अचर) × चर], कुछ निश्चित चर तथा अचर राशियों के योग, अन्तर, गुणन, भाग के आधार पर बने व्यंजक को बीजीय व्यंजक कहते हैं।
एक चरीय बहुपद : सभी बीजीय व्यंजकों में केवल एक चर हो तथा उस चर के घातांक पूर्ण संख्या हों, तो इस रूप के व्यंजकों को एक चरीय बहुपद कहते हैं।
एक चर वाला बहुपद p(x) निम्न रूप का x में बीजीय व्यंजक है :
p(x) = a₀ + a₁x + a₂x² + a₃x³ + …….. + a_nxⁿ
जहाँ a₀, a₁, a₂, ……… a_n अचर हैं और (a ≠ 0) तथा इन्हें x⁰, x¹, x², x³, ……… xⁿ के गुणांक भी कहते हैं।
बहुपद : यदि बीजीय व्यंजक में x (चर) की सभी घातें धनात्मक पूर्ण संख्या हों, उसे बहुपद कहते हैं।
उदाहरण के लिए, y^-2 बहुपद नहीं है क्योंकि चर y की घात ऋणात्मक है जबकि y² बहुपद है।

बहुपद के प्रकार : (A) पदों के आधार पर :
(i) एकपदी (Monomial) व्यंजक : वह बहुपद जिसमें केवल एक पद हो, एकपदी बहुपद कहलाता है।
जैसे- 2x, 2, 5x³, y और u⁴ आदि।
(ii) द्विपदीय (Binomial) व्यंजक : वह बहुपद जिसमें दो पद हों, द्विपदी बहुपद कहलाता है।
जैसे- x + 1, x² + 3, y¹⁰ + 1, x² + u¹⁰ आदि।
(iii) त्रिपदीय (Trinomial) व्यंजक : वह बहुपद जिसमें तीन पद हों, त्रिपदी बहुपद कहलाता है।
जैसे- x⁴ + x³ + 2, 5x² + 4 + 3x आदि ।

(B) घात के आधार पर :
(i) रैखिक बहुपद : वह बहुपद जिसकी घात एक हो, रैखिक बहुपद कहलाता है। उदाहरण के लिए,
p(x) = 4x + 5, q(y) = 2y, r(t) = t + \(\sqrt{2}\) आदि ।
(ii) द्विघाती बहुपद : वह बहुपद जिसकी घात दो हो, द्विघाती बहुपद कहलाता है। उदाहरण के लिए,
2x² + 5, 5x² + 3x + π, x² + \(\frac{2}{5}\)x आदि ।
किसी व्यंजक की सबसे बड़ी घात को बहुपद की घात कहते हैं।

शून्य बहुपद : यदि a₀ = a₁ = a₂ = a₃ ……… a_n = 0
अर्थात सभी अचर शून्य हों तो बहुपद शून्य बहुपद कहलाता है। शून्य बहुपद की घात को परिभाषित नहीं किया जा सकता है।

• एक चर वाले प्रत्येक रैखिक बहुपद में एक शून्यांक होता है।
• स्थिर (अचर) बहुपद का कोई शून्यांक नहीं होता है।
• प्रत्येक वास्तविक संख्या शून्य बहुपद का शून्यांक होता है।

बहुपद का शून्यक: किसी बहुपद में चर के स्थान पर किसी वास्तविक संख्या को प्रतिस्थापित करने पर यदि बहुपद का मान शून्य आता है वह वास्तविक संख्या उस बहुपद का शून्यक कहलाती है।
शेषफल प्रमेय : माना बहुपद p(x) एक या एक से अधिक घात वाला एक बहुपद है। p(x) को रैखिक बहुपद (x – a) से भाग देने पर शेषफल p(a) के बराबर होता है तथा p(a) = 0।

• यदि p(a) = 0 हो, तो (x – a) बहुपद p(x) का एक गुणनखण्ड होता है।
• यदि p(a) ≠ 0 हो, तो (x – a) बहुपद p(x) का एक गुणनखण्ड है।

बीजीय सर्वसमिकाएँ :

1. (x + y)² = x² + y² + 2xy
2. (xy)² = x² + y² – 2xy
3. (x² – y²) = (x + y) (x – y)
4. (x + y + z)² = x² + y² + z² + 2xy + 2yz + 2zx
5. (x + y)³ = x³ + y³ + 3(x + y)xy = x³ + y³ + 3x²y + 3xy²
6. (x – y)³ = x³ – y³ – 3(x – y)xy = x³ – y³ – 3x²y + 3xy²
7. x³ + y³ + z³ – 3xyz = (x + y + z) (x² + y² + z² – xy – yz – zx)
8. (x + a) (x + b) = x² + (a + b)x + ab

Students should go through these JAC Class 9 Maths Notes Chapter 9 समान्तर चतुर्भुज और त्रिभुजों के क्षेत्रफल will seemingly help to get a clear insight into all the important concepts.

JAC Board Class 9 Maths Notes Chapter 9 समान्तर चतुर्भुज और त्रिभुजों के क्षेत्रफल

→ क्षेत्रफल : “तल का वह भाग जो एक आकृति की सीमाओं के अन्दर घिरा रहता है, उस आकृति का क्षेत्रफल (area) कहलाता है।” क्योंकि क्षेत्रफल द्वि-आयामी संकल्पना है अतः इसका मात्रक वर्ग इकाई (मी², सेमी², आदि) होता है।”

→ “वह समतल एवं संवृत्त आकृति, जो तीन रेखाखण्डों से बनी होती है त्रिभुज (Triangle) कहलाती है।”

→ “बहुभुज एवं बहुभुज के अभ्यन्तर (Interior) के सम्मिलन को बहुभुज प्रदेश (Polygonal Region) कहते है।”

→ “समान्तर चतुर्भुज की कोई भी एक भुजा इसका आधार (Base) कहलाती है।”

→ “समान्तर चतुर्भुज के प्रत्येक आधार के संगत शीर्षलम्ब (Altitude) वह रेखाखण्ड है जो आधार के किसी बिन्दु से सम्मुख भुजा को अणविष्ट करने वाली रेखा पर लम्ब है।”

→ “त्रिभुज की माध्यिका उसे दो समान क्षेत्रफल वाले त्रिभुजों में विभाजित करती है।”

→ “एक ही आधार तथा समान समान्तर रेखाओं के मध्य त्रिभुजों के क्षेत्रफल बराबर होते हैं।”

→ “यदि एक त्रिभुज तथा समान्तर चतुर्भुज एक ही आधार तथा समान समान्तर रेखाओं के मध्य स्थित हैं तो त्रिभुज का क्षेत्रफल समान्तर चतुर्भुज के क्षेत्रफल का आधा होता है।”

→ “यदि एक आयत तथा एक समान्तर चतुर्भुज एक ही आधार तथा समान समान्तर रेखाओं के मध्य स्थित हो तो उनके क्षेत्रफल समान होते हैं।”

→ “समस्त सर्वांगसम आकृतियाँ क्षेत्रफल में समान होती हैं, किन्तु यह आवश्यक नहीं है कि क्षेत्रफल में समान आकृतियाँ सर्वांगसम हों।”

→ “समान आधार और समान लम्बाइयों के लम्ब वाले त्रिभुज क्षेत्रफल में समान होते हैं।”

→ “समान आधार और समान ऊँचाई वाले समान्तर चतुर्भुज क्षेत्रफल में भी समान होते हैं।”

→ “समान क्षेत्रफल वाले दो त्रिभुजों की यदि एक-एक भुजा बराबर हो तो उनकी ऊँचाइयाँ भी समान होती हैं।”

→ किसी भी Δ का क्षेत्रफल उसके आधार तथा शीर्ष लम्ब के गुणनफल का आधा होता है। अर्थात् Δ का क्षेत्रफल = \(\frac{1}{2}\) × आधार × लम्ब

→ किसी आयत का क्षेत्रफल उसकी लम्बाई तथा चौड़ाई के गुणनफल के बराबर होता है।” अर्थात् आयत का क्षेत्रफल = लम्बाई × चौड़ाई

→ “किसी चतुर्भुज का क्षेत्रफल उसके एक विकर्ण की लम्बाई तथा उस पर शेष दो शीर्षों से डाले गए लम्बों के योगफल के गुणनफल के आधे के बराबर होता है।”
चतुर्भुज का क्षेत्रफल = \(\frac{1}{2}\) × SQ × (PM + NR)

→ “किसी समलम्ब का क्षेत्रफल उसकी समान्तर भुजाओं की लम्बाइयों के योगफल तथा उनके बीच की दूरी के गुणनफल का आधा होता है।
समलम्ब का क्षेत्रफल = \(\frac{1}{2}\)(SR + PQ) × SD

Students should go through these JAC Class 9 Maths Notes Chapter 8 चतुर्भुज will seemingly help to get a clear insight into all the important concepts.

JAC Board Class 9 Maths Notes Chapter 8 चतुर्भुज

चतुर्भुज : किसी एक ही समतल में स्थित चार सरल रेखाओं से घिरी बन्द आकृति को चतुर्भुज कहते हैं।

• किसी चतुर्भुज में चार भुजाएँ, चार शीर्ष तथा चार कोण होते है।
• चतुर्भुज के सम्मुख शीर्षों को मिलाने वाली रेखाखण्ड को विकर्ण कहते हैं। प्रत्येक चतुभुर्ज में दो विकर्ण होते हैं।
• चतुर्भुज के अन्तः कोणों का योग 4 समकोण या 360° होता है।
• किसी चतुर्भुज का परिमाप उसके विकर्णों के योग से अधिक होता है।

चतुर्भुज की सम्मुख भुजाएँ : चतुर्भुज की वे दो भुजाएँ, जिनका कोई उभयनिष्ठ बिन्दु न हो, सम्मुख भुजाएँ कहलाती हैं।
चतुर्भुज की क्रमागत भुजाएँ : चतुर्भुज की वे दो भुजाएँ, जिनका एक उभयनिष्ठ अंत्य बिन्दु (end-point) हो, क्रमागत भुजाएँ कहलाती हैं।
चतुर्भुज के सम्मुख कोण : चतुर्भुज के वे दो कोण. जिनको अन्तरित करने वाली भुजाओं में कोई भुजा उभयनिष्ठ न हो, सम्मुख कोण कहलाते हैं।
चतुर्भुज के क्रमागत कोण : चतुर्भुज के वे दो कोण, जिनको अंतरित करने वाली भुजाओं में से एक भुजा उभयनिष्ठ हो, क्रमागत कोण कहलाते हैं।
समलम्ब चतुर्भुज : यदि किसी चतुर्भुज की सम्मुख भुजाओं का एक युग्म समान्तर हो, तो उस चतुर्भुज को समलम्ब चतुर्भुज कहते हैं।
समान्तर चतुर्भुज : यदि किसी चतुर्भुज की सम्मुख भुजाओं का प्रत्येक युग्म समान्तर हो, तो उसे समान्तर चतुर्भुज कहते हैं। इसे संक्षेप में ||^gm से निरूपित करते हैं।

गुणधर्म :
(i) यदि समान्तर चतुर्भुज के दो सम्मुख शीर्षों को मिला दिया जाये, तो बना विकर्ण चतुर्भुज को दो सर्वांगसम त्रिभुजों में विभाजित करता है।
(ii) समान्तर चतुर्भुज के सम्मुख कोण बराबर होते हैं।
(iii) समान्तर चतुर्भुज की सम्मुख भुजाएँ बराबर होती हैं।
(iv) समान्तर चतुर्भुज के विकर्ण एक-दूसरे को समद्विभाजित करते हैं।

एक ऐसा समान्तर चतुर्भुज जिसकी सम्मुख भुजाएँ बराबर हों तथा प्रत्येक कोण समकोण हो, आयत (rectangle) कहलाती है।
एक ऐसा समान्तर चतुर्भुज जिसकी सम्मुख भुजाएँ लम्बाई में समान हों, समचतुर्भुज (rhombus) कहलाता है।
एक ऐसा समान्तर चतुर्भुज जिसकी चारों भुजाएँ बराबर हों और चारों कोण समकोण हो, उसे वर्ग (square) कहते हैं।

यदि एक समतल में दो रेखाएँ l व m ( समान्तर या प्रतिच्छेदी) दी हुयी हों और यदि एक तीसरी रेखा x उन्हें भिन्न बिन्दुओं P और O पर प्रतिच्छेदित करती हो, तो रेखाखण्ड PQ को दी गयी रेखाओं द्वारा इस रेखा (x) पर बनाया गया अन्तः खण्ड कहते हैं।

Jharkhand Board JAC Class 10 Maths Solutions Chapter 13 Surface Areas and Volumes Ex 13.4 Textbook Exercise Questions and Answers.

JAC Board Class 10 Maths Solutions Chapter 13 Surface Areas and Volumes Exercise 13.4

Use π = \(\frac{22}{7}\), unless stated otherwise.

Question 1.
A drinking glass is in the shape of a frustum of a cone of height 14 cm. The diameters of its two circular ends are 4 cm and 2 cm. Find the capacity of the glass.
Solution:

Question 2.
The slant height of a frustum of a cone is 4 cm and the perimeters (circumference) of its circular ends are 18 cm and 6 cm. Find the curved surface area of the frustum.
Solution:
C.S.A. of the frustum = πl(r₁ + r₂)
2πr₁ = 18
r₁ = \(\frac{18}{2 \pi}=\frac{9}{\pi}\)
2πr₂ = 6
r₂ = \(\frac{6}{2 \pi}=\frac{3}{\pi}\)
C.S.A. of the frustum = xl(r₁ + r₂)
= π × \(4\left[\frac{9}{\pi}+\frac{3}{\pi}\right]\)
= π × 4 × \(\frac{12}{\pi}\)
= 48 cm².3

Question 3.
A fez, the cap used by the Turks, is shaped like the frustum of a cone. If its radius on the open side is 10 cm, radius at the upper base is 4 cm and its slant height is 15 cm, find the area of material used for making it.

Solution:
r₁ = 4, r₂ = 10, l = 15
Area of the material used = C.S.A. of the frustum + Area of the circular top
= πl(r₁ + r₂) + πr₁²
= \(\frac{22}{7}\) × 15 (4 + 10) + π × 4²
= \(\frac{22}{7}\) × 15 × 14 + \(\frac{22}{7}\) × 16
= \(\frac{22}{7}\)(210 + 16)
= \(\frac{22}{7}\) × 226
= \(\frac{4972}{7}\)
= 710\(\frac{2}{7}\) cm².

Question 4.
A container, opened from the top and made up of a metal sheet, is in the form of a frustum of a cone of height 16 cm with radii of its lower and upper ends as 8 cm and 20 cm, respectively. Find the cost of the milk which can completely fill the container, at the rate of Rs. 20 per litre. Also find the cost of metal sheet used to make the container, if it costs Rs. 8 per 100 cm². (Take π = 3.14).
Solution:
Volume of the milk = Volume of the container = Volume of the frustum
= \(\frac{1}{3}\)πh(r₁² + r₂² + r₁r₂)   [r₁ = 20, r₂ = 8, h = 16]
= \(\frac{1}{3}\) × 3.14 × 16[(20)² + (8)² + (20 × 8)]
= \(\frac{1}{3}\) × 3.14 × 16[400 + 64 + 160]
= \(\frac{1}{3}\) × 3.14 × 16 × 624 c.c. [1 litre 1000 cc]
= \(\frac{1}{3}\) × \(\frac{3.14 \times 16 \times 624}{1000}\) litres.
Cost of the milk = \(\frac{3.14 \times 16 \times 624}{3 \times 1000}\)
= \(\frac{3.14 \times 1664}{25}\)
= 3.14 × 66.56
= 208.99 = Rs. 209.
Area of the metal sheet used = πl(r₁ + r₂) + πr₂² [r₁ = 20, r₂ = 8, h = 16]

Area of the metal sheet used = πl(r₁ + r₂) + πr₂²
= 3.14 × 20(20 + 8) + 3.14 × 8²
= 3.14 × 20 × 28 + 3.14 × 64
= 3.14[(20 × 28) + 64]
= 3.14(560 + 64)
= 3.14 × 624 sq. cms.
Cost of the metal sheet = Area × Rate
= 3.14 × 624 × rate
= \(\frac{3.14 \times 624 \times 8}{100}\)
= 3.14 × 624 × 0.08
= 3.14 × 49.92
= 156.7488
= Rs. 156.75.

Question 5.
A metallic right circular cone 20 cm high and whose vertical angle is 60° is cut into two parts the middle of its height by a plane parallel to its base. If the frustum so obtained be drawn into a wire of diameter \(\frac{1}{6}\) cm, find the length of the wire.
Solution:

JAC Jharkhand Board Class 12th History Important Questions in Hindi & English Medium

JAC Board Class 12th History Important Questions in Hindi Medium

• Chapter 1 ईंटें, मनके तथा अस्थियाँ : हड़प्पा सभ्यता Important Questions
• Chapter 2 राजा, किसान और नगर : आरंभिक राज्य और अर्थव्यवस्थाएँ Important Questions
• Chapter 3 बंधुत्व, जाति तथा वर्ग : आरंभिक समाज Important Questions
• Chapter 4 विचारक, विश्वास और इमारतें : सांस्कृतिक विकास Important Questions
• Chapter 5 यात्रियों के नज़रिए : समाज के बारे में उनकी समझ Important Questions
• Chapter 6 भक्ति-सूफी परंपराएँ : धार्मिक विश्वासों में बदलाव और श्रद्धा ग्रंथ Important Questions
• Chapter 7 एक साम्राज्य की राजधानी : विजयनगर Important Questions
• Chapter 8 किसान, ज़मींदार और राज्य : कृषि समाज और मुगल साम्राज्य Important Questions
• Chapter 9 शासक और इतिवृत्त : मुगल दरबार Important Questions
• Chapter 10 उपनिवेशवाद और देहात : सरकारी अभिलेखों का अध्ययन Important Questions
• Chapter 11 विद्रोही और राज : 1857 का आंदोलन और उसके व्याख्यान Important Questions
• Chapter 12 औपनिवेशिक शहर : नगर-योजना, स्थापत्य Important Questions
• Chapter 13 महात्मा गांधी और राष्ट्रीय आंदोलन : सविनय अवज्ञा और उससे आगे Important Questions
• Chapter 14 विभाजन को समझना : राजनीति, स्मृति, अनुभव Important Questions
• Chapter 15 संविधान का निर्माण : एक नए युग की शुरुआत Important Questions

JAC Board Class 12th History Important Questions in English Medium

• Chapter 1 Bricks, Beads and Bones: The Harappan Civilisation Important Questions
• Chapter 2 Kings, Farmers and Towns: Early States and Economies Important Questions
• Chapter 3 Kinship, Caste and Class: Early Societies Important Questions
• Chapter 4 Thinkers, Beliefs and Buildings: Cultural Developments Important Questions
• Chapter 5 Through the Eyes of Travellers: Perceptions of Society Important Questions
• Chapter 6 Bhakti-Sufi Traditions: Changes in Religious Beliefs and Devotional Texts Important Questions
• Chapter 7 An Imperial Capital: Vijayanagara Important Questions
• Chapter 8 Peasants, Zamindars and the State: Agrarian Society and the Mughal Empire Important Questions
• Chapter 9 Kings and Chronicles: The Mughal Courts Important Questions
• Chapter 10 Colonialism and the Countryside: Exploring Official Archives Important Questions
• Chapter 11 Rebels and the Raj: 1857 Revolt and its Representations Important Questions
• Chapter 12 Colonial Cities: Urbanisation, Planning and Architecture Important Questions
• Chapter 13 Mahatma Gandhi and The Nationalist Movement: Civil Disobedience and Beyond Important Questions
• Chapter 14 Understanding Partition: Politics, Memories, Experiences Important Questions
• Chapter 15 Framing the Constitution: The Beginning of a New Era Important Questions

JAC Jharkhand Board Class 12th History Solutions in Hindi & English Medium

JAC Board Class 12th History Solutions in Hindi Medium

• Chapter 1 ईंटें, मनके तथा अस्थियाँ : हड़प्पा सभ्यता
• Chapter 2 राजा, किसान और नगर : आरंभिक राज्य और अर्थव्यवस्थाएँ
• Chapter 3 बंधुत्व, जाति तथा वर्ग : आरंभिक समाज
• Chapter 4 विचारक, विश्वास और इमारतें : सांस्कृतिक विकास
• Chapter 5 यात्रियों के नज़रिए : समाज के बारे में उनकी समझ
• Chapter 6 भक्ति-सूफी परंपराएँ : धार्मिक विश्वासों में बदलाव और श्रद्धा ग्रंथ
• Chapter 7 एक साम्राज्य की राजधानी : विजयनगर
• Chapter 8 किसान, ज़मींदार और राज्य : कृषि समाज और मुगल साम्राज्य
• Chapter 9 शासक और इतिवृत्त : मुगल दरबार
• Chapter 10 उपनिवेशवाद और देहात : सरकारी अभिलेखों का अध्ययन
• Chapter 11 विद्रोही और राज : 1857 का आंदोलन और उसके व्याख्यान
• Chapter 12 औपनिवेशिक शहर : नगर-योजना, स्थापत्य
• Chapter 13 महात्मा गांधी और राष्ट्रीय आंदोलन : सविनय अवज्ञा और उससे आगे
• Chapter 14 विभाजन को समझना : राजनीति, स्मृति, अनुभव
• Chapter 15 संविधान का निर्माण : एक नए युग की शुरुआत

JAC Board Class 12th History Solutions in English Medium

• Chapter 1 Bricks, Beads and Bones: The Harappan Civilisation
• Chapter 2 Kings, Farmers and Towns: Early States and Economies
• Chapter 3 Kinship, Caste and Class: Early Societies
• Chapter 4 Thinkers, Beliefs and Buildings: Cultural Developments
• Chapter 5 Through the Eyes of Travellers: Perceptions of Society
• Chapter 6 Bhakti-Sufi Traditions: Changes in Religious Beliefs and Devotional Texts
• Chapter 7 An Imperial Capital: Vijayanagara
• Chapter 8 Peasants, Zamindars and the State: Agrarian Society and the Mughal Empire
• Chapter 9 Kings and Chronicles: The Mughal Courts
• Chapter 10 Colonialism and the Countryside: Exploring Official Archives
• Chapter 11 Rebels and the Raj: 1857 Revolt and its Representations
• Chapter 12 Colonial Cities: Urbanisation, Planning and Architecture
• Chapter 13 Mahatma Gandhi and The Nationalist Movement: Civil Disobedience and Beyond
• Chapter 14 Understanding Partition: Politics, Memories, Experiences
• Chapter 15 Framing the Constitution: The Beginning of a New Era

Students should go through these JAC Class 9 Maths Notes Chapter 2 Polynomials will seemingly help to get a clear insight into all the important concepts.

JAC Board Class 9 Maths Notes Chapter 2 Polynomials

Polynomials:
An algebraic expression f(x) of the form f(x) = a₀ + a₁x + a₂x² + …… + a_nxⁿ, where a₀, a₁, a₂ ……, a_n are real numbers and all the index of x’ are nonnegative integers is called a polynomial in x.
→ Degree of a Polynomial: Highest Index of x in algebraic expression is called the degree of the polynomial, here a₀, a₁x, a₂x² ….. a_nxⁿ, are called the terms of the polynomial and a₀, a₁, a₂, …… a_n are called various coefficients of the polynomial f(x).
Note: A polynomial in x is said to be in standard form when the terms are written either in increasing order or decreasing order of the indices of x in various terms.

→ Different Types of Polynomials: Generally, we divide the polynomials in the following categories.
→ Based on degrees:
There are four types of polynomials based on degrees. These are listed below:

• Linear Polynomials: A polynomial of degree one is called a linear polynomial. The general form of linear polynomial is ax + b, where a and b are any real constant and a ≠ 0.
• Quadratic Polynomials: A polynomial of degree two is called a quadratic polynomial. The general form of a quadratic polynomial is ax² + bx + c, where a ≠ 0, a, b, c ∈ R.
• Cubic Polynomials: A polynomial of degree three is called a cubic polynomial. The general form of a cubic polynomial is ax³ + bx² + cx + d, where a ≠ 0 and a, b, c, d ∈ R.
• Biquadratic (or quadric) Polynomials: A polynomial of degree four is called a biquadratic (quadric) polynomial. The general form of a biquadratic polynomial is ax⁴ + bx³ + cx² + dx + e, where a ≠ 0 and a, b, c, d, e are real numbers.

Note: A polynomial of degree five or more than five does not have any particular name. Such a polynomial usually called a polynomial of degree five or six or ….etc.

→ Based on number of terms:
There are three types of polynomials based on number of terms. These are as follow:

• Monomial: A polynomial is said to be monomial if it has only one term. e.g. x, 9x², 5x³ all are monomials.
• Binomial: A polynomial is said to be binomial if it contains only two terms e.g. 2x² + 3x, \(\sqrt{3}\)x + 5x³, -8x³ + 3, all are binomials.
• Trinomial: A polynomial is said to be a trinomial if it contains only three terms.e.g. 3x³ – 8x + \(\frac{1}{2}\), \(\sqrt{7}\) x¹⁰ + 8x⁴ – 3x², 5 – 7x + 8x⁹, are all trinomials.

Note: A polynomial having four or more than four terms does not have particular name. These are simply called polynomials.

→ Zero degree polynomial: Any non-zero number (constant) is regarded as polynomial of degree zero or zero degree polynomial. i.e. f(x) = a. where a ≠ 0 is a zero degree polynomial, since we can write f(x) = a, as f(x) = ax⁰.

→ Zero polynomial: A polynomial whose all coefficients are zero is called as zero polynomial i.e. f(x) = 0, we cannot determine the degree of zero polynomial.

Algebraic Identities:
An identity is an equality which is true for all values of the variables.
Some important identities are:
(i) (a + b)² = a² + 2ab + b²
(ii) (a – b)² = a² – 2ab + b²
(iii) a² – b² = (a + b)(a – b)
(iv) a³ + b³ = (a + b)(a² – ab + b²)
(v) a³ – b³ = (a – b)(a² + ab + b²)
(vi) (a + b)³ = a³ + b³ + 3ab (a + b)
(vii) (a – b)³ = a³ – b³ – 3ab (a – b)
(viii) a⁴ + a²b² + b⁴ = (a² + ab + b²)(a² – ab + b²)
(ix) a³ + b³ + c³ – 3abc = (a + b + c)(a² + b² + c² – ab – bc – ac)

Special case: if a + b + c = 0 then a³ + b³ + c³ = 3abc.
Other Important Identities
(i) a² + b² = (a + b)² – 2ab,
if a + b and ab are given
(ii) a² + b² = (a – b)² + 2ab
if a – b and ab are given
(iii) a + b = \(\sqrt{(a-b)^2+4 a b}\)
if a – b and ab are given
(iv) a – b = \(\sqrt{(a+b)^2-4 a b}\)
if a + b and ab are given

Factors Of A Polynomial:
→ If a polynomial f(x) can be written as a product of two or more other polynomials f₁(x), f₂(x), f₃(x)…. then each of the polynomials f₁(x), f₂(x), f₃(x)….. is called a factor of polynomial f(x). The method of finding the factors of a polynomial is called factorisation.

Zeroes Of A Polynomial:
→ A real number α is a zero of polynomial f(x) = a_nxⁿ + a_n-1x^n-1 + a_n-2x^n-2 + ….. +a₁x + a₀, if f(α) = 0. i.e. a_nαⁿ + a_n-1α^n-1 + a_n-2α^n-2+ ….. + a₁α + a₀ = 0.
For example x = 3 is a zero of the polynomial f(x) = x³ – 6x² + 11x – 6, because f(3) = (3)³ – 6(3)² + 11(3) – 6 = 27 – 54 + 33 – 6 = 0.
but x = -2 is not a zero of the above mentioned polynomial,
∵ f(-2) = (-2)³ – 6(-2)² + 11(-2) – 6
f(-2) = -8 – 24 – 22 – 6
f(-2) = -60 ≠ 0.

→ Value of a Polynomial: The value of a polynomial f(x) at x = a is obtained by substituting x a in the given polynomial and is denoted by f(a). Eg if f(x) = 2x³ – 13x² + 17x + 12 then its value at x = 1 is
f(1) = 2(1)³ – 13(1)² + 17(1) + 12
= 2 – 13 + 17 + 12 = 18.

Remainder Theorem:
Let ‘p(x)’ be any polynomial of degree greater than or equal to one and ‘a’ be any real number and if p(x) is divided by (x – a). then the remainder is equal to p(a). Let q(x) be the quotient and r(x) be the remainder when p(x) is divided by (x – a), then
Dividend = Divisor × Quotient + Remainder
∴ p(x) = (x – a) × q(x) + [r(x) or r], where r(x) = 0 or degree of r(x) < degree of (x – a). But (x – 2) is a polynomial of degree 1 and a polynomial of degree less than 1 is a constant. Therefore, either r(x) = 0 or r(x) = Constant. Let r(x) = r, then p(x) = (x – a)q(x) + r.
Putting x = a in above equation, p(a)
p(a) = (a – a)q(a) + r = 0 × q(a) + r
p(a) = 0 + r
⇒ p(a) = r
This shows that the remainder is p(a) when p(x) is divided by (x – a).
Remark: If a polynomial p(x) is divided by (x + a),(ax – b), (ax + b), (b – ax) then the remainder is the value of p(x) at x.
= \(-a, \frac{b}{a},-\frac{b}{a}, \frac{b}{a} \text { i.e. } p(-a)\)
\(p\left(\frac{b}{a}\right), p\left(-\frac{b}{a}\right), p\left(\frac{b}{a}\right)\) respectively.

Factor Theorem:
Let ‘p(x)’ be a polynomial of degree greater than or equal to 1 and ‘a’ be a real number such that p(a) = 0, then (x – a) is a factor of p(x). Conversely, if(x – a) is a factor of p(x). then p(a) = 0.

Factorisation Of A Quadratic Polynomial:
→ For factorisation of a quadratic expression ax² + bx + c where a ≠ 0, there are two methods.
→ By Method of Completion of Square:
In the form ax² + bx + c where a ≠ 0, firstly we take ‘a’ common in the whole expression then factorise by converting the expression \(a\left\{x^2+\frac{b}{a} x+\frac{c}{a}\right\}\) as the difference of two squares, which is

→ By Splitting the Middle Term:
→ x² + lx + m = x² + (a + b)x + ab
Where l = a + b and m = ab, such that a and b are real numbers
= x² + ax + bx + ab
= x (x + a) + b (x + a)
= (x + a) (x + b)
Method: We express l as the sum of two such numbers whose product is m.

→ ax² + bx + c = prx² + (ps + qr)x + qs
where b = ps + qr, a = pr, c = qs
so that (ps) (gr) (pr) (qs) = ac
∴ prx² + (ps + qr)x + qs
= prx² + psx + qrx + qs
= px (rx + s) + q(rx + s)
= (px + q) (rx + x)
Method: We express b as the sum of two such numbers whose product is ac.

→ Integral Root Theorem:
If f(x) is a polynomial with integral coefficient and the leading coefficient is 1, then any integral root of f(x) is a factor of the constant term. Thus if f(x) = x³ – 6x² + 11x – 6 has an Integral root, then it is one of the factors of 6 which are ±1, ±2, ±3, ±6.
Now in fact,
f(1) = (1)³ – 6(1)² + 11(1) – 6 = 1 – 6 + 11 – 6 = 0
f(2) = (2)³ – 6(2)² + 11(2) – 6
= 8 – 24 + 22 – 6 = 0
f(3) = (3)³ – 6(3)² + 11(3) – 6
27 – 54 + 33 – 6 = 0
Therefore Integral roots of f(x) are 1, 2, 3.

→ Rational Root Theorem:
Let \(\frac{b}{c}\) be a rational fraction in lowest terms. If \(\frac{b}{c}\) is a rational root of the polynomial f(x) = a_nxⁿ + a_n-1x^n-1 +…+ a₁x + a₀, an ≠ 0 with integral coefficients, then b is a factor of constant term a₀, and C is a factor of the leading coefficient a_n.
For example: If \(\frac{b}{c}\) is a rational root of the polynomial f(x) = 6x³ + 5x² – 3x – 2, then the values of b are limited to the factors of -2, which are ±1, ±2 and the values of care limited to the factors of 6, which are ±1, ±2, ±3, ±6. Hence, the possible rational roots of f(x) are ±1, ±2, \(\pm \frac{1}{2}, \pm \frac{1}{3}, \pm \frac{1}{6}, \pm \frac{2}{3}\). In fact -1 is an integral root and \(\frac{2}{3}\), –\(\frac{1}{2}\) are the rational roots of f(x) = 6x³ + 5x² – 3x – 2.
Note: (i) n^th degree polynomial can have at most n real roots.
→ Finding a zero of polynomial f(x) means solving the polynomial equation f(x) = 0. It follows from the above discussion that if f(x) = ax + b, a ≠ 0 is a linear polynomial, then it has only one zero given by
f(x) = 0 i.e. f(x) = ax + b = 0
⇒ ax = -b
⇒ x = –\(\frac{b}{a}\)
Thus, x = –\(\frac{b}{a}\) is the only zero of f(x) = ax + b.
→ If a polynomial of degree n has more than n zeros then all the coefficients of powers of x including constant term of polynomial are zero.

Students should go through these JAC Class 9 Maths Notes Chapter 8 Quadrilaterals will seemingly help to get a clear insight into all the important concepts.

JAC Board Class 9 Maths Notes Chapter 8 Quadrilaterals

Quadrilateral
A quadrilateral is a closed figure obtained by joining four points (with no three points collinear) in an order.
→ Since, ‘quad’ means ‘four’ and ‘lateral’ is for ‘sides therefore quadrilateral means a figure bounded by four sides’
→ Every quadrilateral has:
(A) Four vertices
(B) Four sides
(C) Four angles and
(D) Two diagonals.
→ A diagonal is a line segment obtained on joining the opposite vertices.

Sum of the Angles of a Quadrilateral:

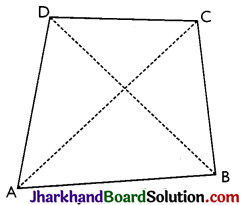
Consider a quadrilateral ABCD as shown in figure. Join A and C to get the diagonal AC which divides the quadrilateral ABCD into two triangles ABC and ADC.
We know the sum of the angles of each triangle is 180°
∴ In ΔABC; ∠CAB + ∠B + ∠BCA = 180°
and In ΔADC; ∠DAC + ∠D + ∠DCA = 180°
On adding, we get:
(∠CAB + ∠DAC) + ∠B + ∠D + (∠BCA + ∠DCA) = 180° + 180°
⇒ ∠A + ∠B + ∠D + ∠C = 360°
Thus, the sum of the angles of a quadrilateral is 360°.

Types of Quadrilaterals:
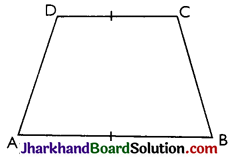
→ Trapezium: It is a quadrilateral in which one pair of opposite sides are parallel and one pair is unparallel. In the quadrilateral ABCD, drawn alongside, sides AB and DC are parallel and AD and BC are unparallel therefore it is a trapezium

→ Parallelogram: It is a quadrilateral in which both the pairs of opposite sides are equal and parallel. The figure shows a quadrilateral ABCD in which AB is parallel and equal to DC and AD is parallel and equal to BC, therefore ABCD is a parallelogram.

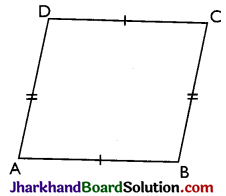
Here, (A) ∠A = ∠C and ∠B = ∠D
(B) AB = CD and AD = BC
(C) AB || CD and AD || BC
→ Rectangle: It is a parallelogram whose each angle is 90°.
(a) ∠A + ∠B = 90° + 90° = 180°
⇒ AD || BC, also AD = BC.
(b) ∠B + ∠C = 90° + 90° = 180°
⇒ AB || DC, also AB = DC.
(c) Diagonals AC and BD are equal.

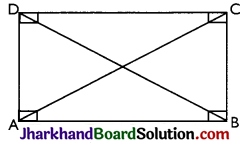
Rectangle ABCD is also a parallelogram.
→ Rhombus: It is a also parallelogram whose all the sides are equal and diagonals are perpendicular to each other. The figure shows a parallelogram ABCD in which AB = BC = CD = DA; AC ⊥ BD.; therefore it is a rhombus.

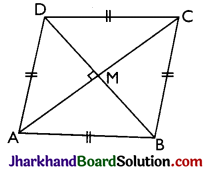
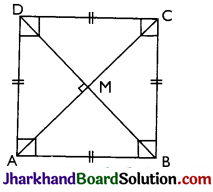
→ Square: It is a parallelogram whose all the sides are equal and each angle is 90°. Also, diagonals are equal and perpendicular to each other. The figure shows a parallelogram ABCD in which AB = BC = CD = DA, ∠A = ∠B = ∠C = ∠D = 90°, AC ⊥ BD and AC = BD, therefore ABCD is a square.

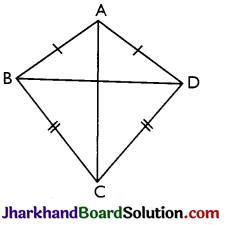
→ Kite: It is not parallelogram in which two pairs of adjacent sides are equal The figure shows a quadrilateral ABCD in which adjacent sides AB and AD are equal i.e. AB = AD and also the other pair of adjacent sides are equal i.e., BC = CD; therefore it is a kite or kite shaped figure.

Remarks:

• Square, rectangle and rhombus are all parallelograms.
• Kite and trapezium are not parallelograms.
• A square is a rectangle.
• A square is a rhombus.
• A parallelogram is a trapezium.

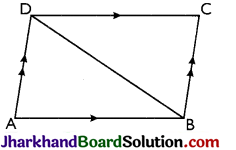
Parallelogram Theorems
A parallelogram is a quadrilateral in which both the pairs of opposite sides are equal and parallel.

Theorem 1.
A diagonal of a parallelogram divides the parallelogram into two congruent triangles.
Proof:
Given: A parallelogram ABCD.
To Prove: A diagonal divides the parallelogram
into two congruent triangles i.e., if diagonal AC is drawn then ΔABC ≅ ΔCDA and if diagonal BD is drawn
then ΔABD ≅ ΔCDB.
Construction: Join A and C
Proof: Since, ABCD is a parallelogram
AB || DC and AD || BC
In ΔABC and ΔCDA
∠BAC = ∠DCA [Alternate angles]
∠BCA = ∠DAC [Alternate angles]
And, AC = AC [Common side]
∴ ΔABC ≅ ΔCDA [By ASA]
Similarly, we can prove that
ΔABD ≅ ΔCDB

Theorem 2.
In a parallelogram, opposite sides are equal.

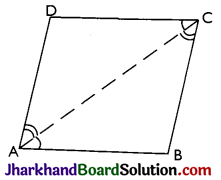
Proof:
Given: A parallelogram ABCD in which
AB || DC and AD || BC.
To Prove: Opposite sides are equal i.e.. AB = DC and AD = BC
Construction: Join A and C
Proof: In ΔABC and ΔCDA
∠BAC = ∠DCA [Alternate angles]
∠BCA = ∠DAC [Alternate angles]
AC = AC [Common]
∴ ΔABC ≅ ΔCDA [By ASA]
⇒ AB = DC and AD = BC [By CPCT]
Hence, proved.

Theorem 3.
If each pair of opposite sides of a quadrilateral is equal, then it is a parallelogram.

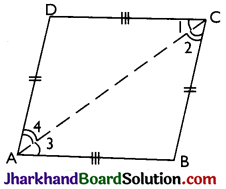
Proof:
Given: A quadrilateral ABCD in which AB = DC and AD = BC.
To Prove: ABCD is a parallelogram ie, AB || DC and AD || BC
Construction: Join A and C
Proof: In ΔABC and ΔCDA
AB = DC [Given]
AD = BC [Given]
And AC = AC [Common]
∴ ΔABC ≅ ΔCDA [By SSS]
⇒ ∠1 = ∠3 [By CPCT]
And ∠2 = ∠4 [By CPCT]
But these are alternate angles and whenever alternate angles are equal, the lines are parallel.
∴ AB || DC and AD || BC
⇒ ABCD is a parallelogram.
Hence, proved.

Theorem 4.
In a parallelogram, opposite angles are equal.

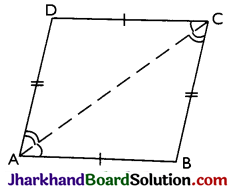
Proof:
Given: A parallelogram ABCD in which AB || DC and AD || BC.
To Prove: Opposite angles are equal i.e. ∠A = ∠C and ∠B = ∠D
Construction: Draw diagonal AC.
Proof: In ΔABC and ΔCDA
∠BAC = ∠DCA [Alternate angles]
∠BCA = ∠DAC [Alternate angles]
AC = AC [Common]
∴ ΔABC ≅ ΔCDA [By ASA]
⇒ ∠B = ∠D [By CPCT]
Similarly, we can prove that
∠A = ∠C Hence, proved.

Theorem 5.
If in a quadrilateral, each pair of opposite angles is equal, then it is a parallelogram.
Proof:
Given: A quadrilateral ABCD in which opposite angles are equal. i.e., ∠A = ∠C and ∠B = ∠D
To prove: ABCD is a parallelogram i.e.,
AB || DC and AD || BC
Proof: Since the sum of the angles of quadrilateral is 360°
⇒ ∠A + ∠B + ∠C+ ∠D = 360°
⇒ ∠A + ∠D + ∠A + ∠D = 360°
[∵ ∠A = ∠C and ∠B = ∠D]
⇒ 2∠A + 2∠D = 360°
⇒ ∠A + ∠D = 180°
[∵ The sum of interior angles on the same side of transversal AB is 180°]
⇒ AB || DC
Similarly, ∠A + ∠B + ∠C + ∠D = 360°
⇒ ∠A + ∠B + ∠A + ∠B = 360°
[∵ ∠A = ∠C and ∠B = ∠D]
⇒ 2∠A + 2∠B = 360°
⇒ ∠A + ∠B = 180°
[∵ The sum of interior angles on the same side of transversal AB is 180°]
∴ AD || BC
So, AB || DC and AD || BC
⇒ ABCD is a parallelogram.
Hence, proved.

Theorem 6.
The diagonal of a parallelogram bisect each other.

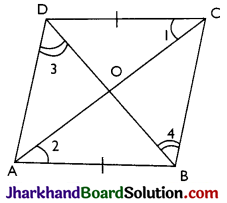
Proof:
Given: A parallelogram ABCD. Its diagonals AC and BD intersect each other at point O..
To Prove: Diagonals AC and BD bisect each other i.e., OA = OC and OB = OD.
Proof: In ΔAOB and ΔCOD
∵ AB || DC and BD is a transversal.
∴ ∠ABO = ∠CDO [Alternate angles]
∵ AB || DC and AC is a transversal line.
∴ ∠BAO = ∠DCO [Alternate angles]
And, AB = DC
⇒ ΔAOB ≅ ΔCOD [By ASA]
⇒ OA = OC and OB = OD [By CPCT]
Hence, proved.

Theorem 7.
If the diagonals of a quadrilateral bisect each other, then it is a parallelogram.

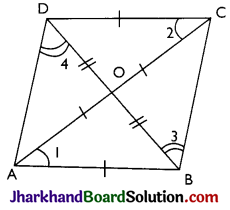
Proof:
Given: A quadrilateral ABCD whose diagonals AC and BD bisect each other at point O.
i.e., OA = OC and OB = OD
To prove: ABCD is a parallelogram i.e..
AB || DC and AD || BC.
Proof: In ΔAOB and ΔCOD
OA = OC [Given]
OB = OD [Given]
And, ∠AOB = ∠COD [Vertically opposite angles]
⇒ ΔAOB ≅ ΔCOD [By SAS]
⇒ ∠1 = ∠2 [By CPCT]
But these are alternate angles and whenever alternate angles are equal, the lines are parallel.
∴ AB is parallel to DC ie., AB || DC Similarly,
ΔAOD ≅ ΔCOB [By SAS]
⇒ ∠3 = ∠4
But these are also alternate angles
⇒ AD || BC
AB || DC and AD || BC
⇒ ABCD is parallelogram.
Hence, proved.

Theorem 8.
A quadrilateral is a parallelogram, if a pair of opposite sides is equal and parallel.

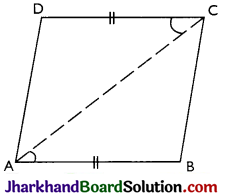
Proof:
Given: A quadrilateral ABCD in which AB = DC and AB || DC.
To Prove: ABCD is a parallelogram, i.e. AB || DC and AD || BC.
Construction: Join A and C.
Proof: Since AB is parallel to DC and AC is transversal
∠BAC = ∠DCA [Alternate angles]
AB = DC [Given]
And AC = AC [Common]
⇒ ΔBAC ≅ ΔDCA [By SAS]
⇒ ∠BCA = ∠DAC [By CPCT]
But these are alternate angles and whenever alternate angles are equal, the lines are parallel.
⇒ AD || BC
Now, AB || DC (given) and AD || BC
[Proved above]
⇒ ABCD is a parallelogram
Hence, proved.

Remarks:
In order to prove that given quadrilateral is parallelogram, we can prove any one of the following.

• Opposite angles of the quadrilateral are equal, or
• Diagonals of the quadrilateral bisect each other, or
• A pair of opposite sides is parallel and is of equal length, or
• Opposite sides are equal.
• Every diagonal divides the parallelogram into two congruent triangles.

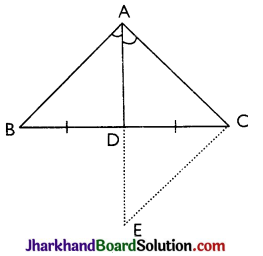
Mid-Point Theorem
Statement: In a triangle, the line segment joining the mid-points of any two sides is parallel to the third side and is half of it.

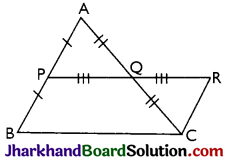
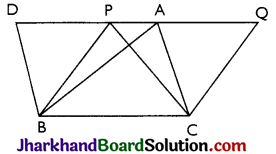
Given: A triangle ABC in which P is the mid-point of side AB and Q is the mid-point of side AC.
To Prove: PQ is parallel to BC and is half of it
i.e., PQ || BC and PQ = \(\frac{1}{2}\)BC
Construction: Produce PQ upto point such that PQ = QR. Join R and C.
Proof: In ΔAPQ and ΔCRQ
PQ = QR [By construction]
AQ = QC [Given]
And, ∠AQP = ∠CQR [Vertically opposite angles]
⇒ ΔAPQ ≅ ΔCRQ [By SAS]
⇒ AP = CR [By CPCT]
And, ∠APQ = ∠CRQ [By CPCT]
But, ∠APQ and ∠CRQ are alternate angles and we know, whenever the alternate angles are equal, the lines are parallel.
⇒ AP || CR
⇒ AB || CR
⇒ BP || CR
Given, P is mid-point of AB
⇒ AP = BP
⇒ CR = BP [As, AP = CR]
Now, BP = CR and BP || CR
⇒ BCRP is a parallelogram.
[When any pair of opposite sides are equal and parallel, the quadrilateral is a parallelogram]
BCRP is a parallelogram and opposite sides of a parallelogram are equal and parallel.
∴ PR = BC and PR || BC
Since, PQ = QR
⇒ PQ = \(\frac{1}{2}\)PR = \(\frac{1}{2}\)BC [AS, PR = BC]
Also, PQ || BC [As, PR || BC]
∴ PQ || BC and PQ = \(\frac{1}{2}\)BC
Hence, proved.

Converse of the Mid-Point Theorem
Statement: The line drawn through the midpoint of one side of a triangle parallel to the another side bisects the third side.

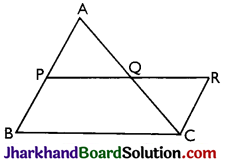
Given: A triangle ABC in which is the midpoint of side AB and PQ is parallel to BC.
To prove: PQ bisects the third side AC i.e., AQ = QC.
Construction: Through C, draw CR parallel to BA, which meets PQ produced at point R.
Proof: Since, PQ || BC i.e., PR || BC [Given]
CR || BA i.e., CR || BP [By construction]
∴ Opposite sides of quadrilateral PBCR are parallel.
⇒ PBCR is a parallelogram
⇒ BP = CR
Also, BP = AP [As Pis mid-point of AB]
∴ CR = AP (As CR = BP)
Now, AB || CR and AC is transversal, ∠PAQ = ∠ROQ [Alternate angles]
Also, AB || CR and PR is transversal, ∠APQ = ∠CRQ [Alternate angles]
In ΔAPQ and ΔCRQ
CR = AP, ∠PAQ = ∠RCQ and ∠APQ = ∠CRQ
⇒ ΔAPQ ≅ ΔCRO [By ASA]
⇒ AQ = QC Hence, proved.

Students should go through these JAC Class 9 Maths Notes Chapter 5 Introduction to Euclid’s Geometry will seemingly help to get a clear insight into all the important concepts.

JAC Board Class 9 Maths Notes Chapter 5 Introduction to Euclid’s Geometry

Introduction:
The credit for introducing geometrical concepts goes to the distinguished Greek mathematician ‘Euclid’ who is known as the “Father of Geometry” and the word geometry comes from the Geek words ‘geo’ which means “Earth’ and ‘Metreon’ which means ‘measure’.

Basic Concepts In Geometry:
A point, a ‘line’ and a plane are the basic concepts to be used in geometry.
→ Axioms:
The statement that is taken to be true without proof, to serve as a premise for further reasoning and arguments, are called axioms.

Euclid’s Definitions:

• A point is that which has no part.
• A line is breadthless length.
• The ends of a line segment are points.
• A straight line is that which has length only.
• A surface is that which has length and breadth only.
• The edges of surface are lines.
• A plane surface is a surface which lies evenly with the straight lines on itself.

Euclid’s Five Postulates:
→ A straight line may be drawn from any one point to any other point.
→ A terminated line or a line segment can be produced infinitely.

→ A circle can be drawn with any centre and of any radius.
→ All right angles are equal to one another.
→ If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines, if produced infinitely, meet on that side on which the sum of angles is less than two right angles.

Important Axioms:
→ A line is the collection of infinite number of points.
→ Through a given point, infinite lines can be drawn.

→ Given two distinct points, there is one and only one line that contains both the points.
→ If P is a point not lying on a line l, then one and only one line can be drawn through P which is parallel to l.

→ Two distinct lines cannot have more than one point in common.

→ Two lines which are both parallel to the same line, are parallel to each other. i.e. IF l || n, m || n ⇒ l || m.

Some Important Definitions:
→ Collinear points: Three or more points are said to be collinear if there is a line which contains all of them.

→ Concurrent lines: Three or more lines are said to be concurrent if there is a point which lies on all of them.

→ Intersecting lines: Two lines are intersecting if they have a common point. The common point is called the “point of intersection”.

→ Parallel lines: Two lines I and m in a plane are said to be parallel lines if they do not have a common point.

→ Line segment: Given two points A and B on a line l, the connected part (segment) of the line with end points at A and B is called the line segment AB.

→ Interior point of a line segment: A point R is called an interior point of a line segment PQ if R lies between Pand O but Ris neither P nor Q.

→ Congruence of line segment: Two line segments AB and CD are congruent if trace copy of one can be superposed on the other so as to cover it completely and exactly in this case we write AB ≅ CD. In other words we can say two lines are congruent if their lengths are same.
→ Distance between two points: The distance between two points P and Q is the length of the line segment PO.
→ Ray: Directed line segment is called a ray. If AB is a ray, then it is denoted by \(\overrightarrow{\mathrm{AB}}\). Point A is called initial point of ray.

→ Opposite rays: Two rays AB and AC are said to be opposite rays if they are collinear and point A is the only common point of the two rays and A lies in between B and C.

Theorem 1.
If l, m, n are lines in the same plane such that l intersects m and n || m, then l also intersects n.
Answer:
Given: Three lines l, m, n in the same plane such that intersects m and n || m.
To prove: Lines land n are intersecting lines.

Proof: Let l and n be non intersecting lines. Then. l || n. But, n || m [Given]
∴ l || n and n || m
⇒ l || m
⇒ l and m are non-intersecting lines.
This is a contradiction to the hypothesis that I and m are intersecting lines. So our supposition is wrong.
Hence, l intersects line n.

Theorem 2.
If lines AB, AC, AD and AE are parallel to a line l, then points A, B, C, D and E are collinear.
Answer:
Given: Lines AB, AC, AD and AE are parallel to a line l.
To prove: A, B, C, D, E are collinear.
Proof: Since AB, AC, AD and AE are all parallel to a line l. Therefore point A is outside l and lines AB, AC, AD, AE are drawn through A and each line is parallel to l.
But by parallel lines axiom, one and only one line can be drawn through the point A outside a line l and parallel to it.
This is possible only when A, B, C, D and E all lie on the same line. Hence, A, B, C, D and E are collinear.

JAC Jharkhand Board Class 9th Maths Solutions in Hindi & English Medium

JAC Board Class 9th Maths Solutions in English Medium

JAC Class 9 Maths Chapter 1 Number Systems

• Chapter 1 Number Systems Ex 1.1
• Chapter 1 Number Systems Ex 1.2
• Chapter 1 Number Systems Ex 1.3
• Chapter 1 Number Systems Ex 1.4
• Chapter 1 Number Systems Ex 1.5
• Chapter 1 Number Systems Ex 1.6

JAC Class 9 Maths Chapter 2 Polynomials

• Chapter 2 Polynomials Ex 2.1
• Chapter 2 Polynomials Ex 2.2
• Chapter 2 Polynomials Ex 2.3
• Chapter 2 Polynomials Ex 2.4
• Chapter 2 Polynomials Ex 2.5

JAC Class 9 Maths Chapter 3 Coordinate Geometry

• Chapter 3 Coordinate Geometry Ex 3.1
• Chapter 3 Coordinate Geometry Ex 3.2
• Chapter 3 Coordinate Geometry Ex 3.3

JAC Class 9 Maths Chapter 4 Linear Equations in Two Variables

• Chapter 4 Linear Equations in Two Variables Ex 4.1
• Chapter 4 Linear Equations in Two Variables Ex 4.2
• Chapter 4 Linear Equations in Two Variables Ex 4.3
• Chapter 4 Linear Equations in Two Variables Ex 4.4

JAC Class 9 Maths Chapter 5 Introduction to Euclid’s Geometry

• Chapter 5 Introduction to Euclid’s Geometry Ex 5.1
• Chapter 5 Introduction to Euclid’s Geometry Ex 5.2

JAC Class 9 Maths Chapter 6 Lines and Angles

• Chapter 6 Lines and Angles Ex 6.1
• Chapter 6 Lines and Angles Ex 6.2
• Chapter 6 Lines and Angles Ex 6.3

JAC Class 9 Maths Chapter 7 Triangles

• Chapter 7 Triangles Ex 7.1
• Chapter 7 Triangles Ex 7.2
• Chapter 7 Triangles Ex 7.3
• Chapter 7 Triangles Ex 7.4
• Chapter 7 Triangles Ex 7.5

JAC Class 9 Maths Chapter 8 Quadrilaterals

• Chapter 8 Quadrilaterals Ex 8.1
• Chapter 8 Quadrilaterals Ex 8.2

JAC Class 9 Maths Chapter 9 Areas of Parallelograms and Triangles

• Chapter 9 Areas of Parallelograms and Triangles Ex 9.1
• Chapter 9 Areas of Parallelograms and Triangles Ex 9.2
• Chapter 9 Areas of Parallelograms and Triangles Ex 9.3
• Chapter 9 Areas of Parallelograms and Triangles Ex 9.4

JAC Class 9 Maths Chapter 10 Circles

• Chapter 10 Circles Ex 10.1
• Chapter 10 Circles Ex 10.2
• Chapter 10 Circles Ex 10.3
• Chapter 10 Circles Ex 10.4
• Chapter 10 Circles Ex 10.5
• Chapter 10 Circles Ex 10.6

JAC Class 9 Maths Chapter 11 Constructions

• Chapter 11 Constructions Ex 11.1
• Chapter 11 Constructions Ex 11.2

JAC Class 9 Maths Chapter 12 Heron’s Formula

• Chapter 12 Heron’s Formula Ex 12.1
• Chapter 12 Heron’s Formula Ex 12.2

JAC Class 9 Maths Chapter 13 Surface Areas and Volumes

• Chapter 13 Surface Areas and Volumes Ex 13.1
• Chapter 13 Surface Areas and Volumes Ex 13.2
• Chapter 13 Surface Areas and Volumes Ex 13.3
• Chapter 13 Surface Areas and Volumes Ex 13.4
• Chapter 13 Surface Areas and Volumes Ex 13.5
• Chapter 13 Surface Areas and Volumes Ex 13.6
• Chapter 13 Surface Areas and Volumes Ex 13.7
• Chapter 13 Surface Areas and Volumes Ex 13.8
• Chapter 13 Surface Areas and Volumes Ex 13.9

JAC Class 9 Maths Chapter 14 Statistics

• Chapter 14 Statistics Ex 14.1
• Chapter 14 Statistics Ex 14.2
• Chapter 14 Statistics Ex 14.3
• Chapter 14 Statistics Ex 14.4

JAC Class 9 Maths Chapter 15 Probability

• Chapter 15 Probability Ex 15.1

JAC Board Class 9th Maths Solutions in Hindi Medium

JAC Class 9 Maths Chapter 1 संख्या पद्धति

• Chapter 1 संख्या पद्धति Ex 1.1
• Chapter 1 संख्या पद्धति Ex 1.2
• Chapter 1 संख्या पद्धति Ex 1.3
• Chapter 1 संख्या पद्धति Ex 1.4
• Chapter 1 संख्या पद्धति Ex 1.5
• Chapter 1 संख्या पद्धति Ex 1.6

JAC Class 9 Maths Chapter 2 बहुपद

• Chapter 2 बहुपद Ex 2.1
• Chapter 2 बहुपद Ex 2.2
• Chapter 2 बहुपद Ex 2.3
• Chapter 2 बहुपद Ex 2.4
• Chapter 2 बहुपद Ex 2.5

JAC Class 9 Maths Chapter 3 निर्देशांक ज्यामिति

• Chapter 3 निर्देशांक ज्यामिति Ex 3.1
• Chapter 3 निर्देशांक ज्यामिति Ex 3.2
• Chapter 3 निर्देशांक ज्यामिति Ex 3.3

JAC Class 9 Maths Chapter 4 दो चरों वाले रैखिक समीकरण

• Chapter 4 दो चरों वाले रैखिक समीकरण Ex 4.1
• Chapter 4 दो चरों वाले रैखिक समीकरण Ex 4.2
• Chapter 4 दो चरों वाले रैखिक समीकरण Ex 4.3
• Chapter 4 दो चरों वाले रैखिक समीकरण Ex 4.4

JAC Class 9 Maths Chapter 5 युक्लिड के ज्यामिति का परिचय

• Chapter 5 युक्लिड के ज्यामिति का परिचय Ex 5.1
• Chapter 5 युक्लिड के ज्यामिति का परिचय Ex 5.2

JAC Class 9 Maths Chapter 6 रेखाएँ और कोण

• Chapter 6 रेखाएँ और कोण Ex 6.1
• Chapter 6 रेखाएँ और कोण Ex 6.2
• Chapter 6 रेखाएँ और कोण Ex 6.3

JAC Class 9 Maths Chapter 7 त्रिभुज

• Chapter 7 त्रिभुज Ex 7.1
• Chapter 7 त्रिभुज Ex 7.2
• Chapter 7 त्रिभुज Ex 7.3
• Chapter 7 त्रिभुज Ex 7.4
• Chapter 7 त्रिभुज Ex 7.5

JAC Class 9 Maths Chapter 8 चतुर्भुज

• Chapter 8 चतुर्भुज Ex 8.1
• Chapter 8 चतुर्भुज Ex 8.2

JAC Class 9 Maths Chapter 9 समान्तर चतुर्भुज और त्रिभुजों के क्षेत्रफल

• Chapter 9 समान्तर चतुर्भुज और त्रिभुजों के क्षेत्रफल Ex 9.1
• Chapter 9 समान्तर चतुर्भुज और त्रिभुजों के क्षेत्रफल Ex 9.2
• Chapter 9 समान्तर चतुर्भुज और त्रिभुजों के क्षेत्रफल Ex 9.3
• Chapter 9 समान्तर चतुर्भुज और त्रिभुजों के क्षेत्रफल Ex 9.4

JAC Class 9 Maths Chapter 10 वृत्त

• Chapter 10 वृत्त Ex 10.1
• Chapter 10 वृत्त Ex 10.2
• Chapter 10 वृत्त Ex 10.3
• Chapter 10 वृत्त Ex 10.4
• Chapter 10 वृत्त Ex 10.5
• Chapter 10 वृत्त Ex 10.6

JAC Class 9 Maths Chapter 11 रचनाएँ

• Chapter 11 रचनाएँ Ex 11.1
• Chapter 11 रचनाएँ Ex 11.2

JAC Class 9 Maths Chapter 12 हीरोन का सूत्र

• Chapter 12 हीरोन का सूत्र Ex 12.1
• Chapter 12 हीरोन का सूत्र Ex 12.2

JAC Class 9 Maths Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन

• Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन Ex 13.1
• Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन Ex 13.2
• Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन Ex 13.3
• Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन Ex 13.4
• Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन Ex 13.5
• Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन Ex 13.6
• Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन Ex 13.7
• Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन Ex 13.8
• Chapter 13 पृष्ठीय क्षेत्रफल एवं आयतन Ex 13.9

JAC Class 9 Maths Chapter 14 सांख्यिकी

• Chapter 14 सांख्यिकी Ex 14.1
• Chapter 14 सांख्यिकी Ex 14.2
• Chapter 14 सांख्यिकी Ex 14.3
• Chapter 14 सांख्यिकी Ex 14.4

JAC Class 9 Maths Chapter 15 प्रायिकता

• Chapter 15 प्रायिकता Ex 15.1